Boyle’s Law

Boyle's Law is one of the fundamental principles in the study of gases, and it explains the relationship between the pressure-volume of a gas if the temperature remains constant. That simply means that the law, which was first introduced by Robert Boyle in the 17th century, states that the pressure of a given measure of gas is inversely proportional to its volume if the temperature remains constant. This means that as the volume of a gas decreases, its pressure increases, and vice versa.

This Story also Contains

1. Boyle's Law
2. Some Solved Examples
3. Summary

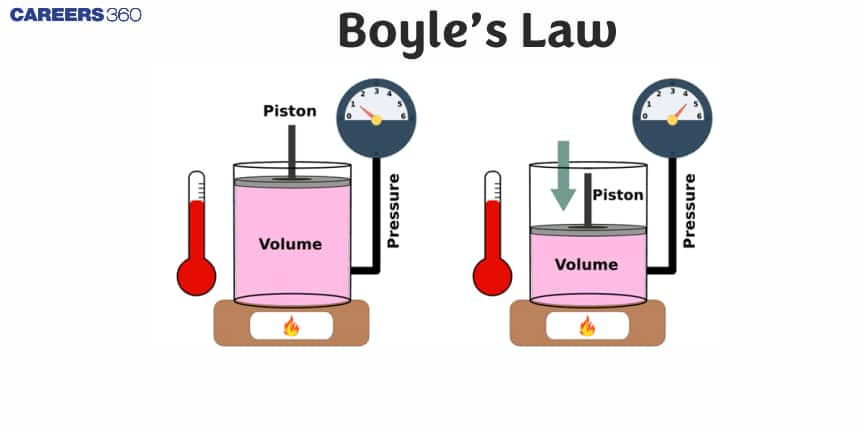
Boyle's Law

An Anglo-Irish scientist 'Robert Boyle' in 1662 gave the pressure-volume relationship of a gas. He made some experiments based on which he concluded that "At constant temperature, the pressure of a fixed amount of gas varies inversely with the volume of the gas." That means if the pressure is doubled, the volume is halved.

An Anglo-Irish scientist 'Robert Boyle' in 1662 gave the pressure-volume relationship of a gas. He made some experiments on the basis of which he concluded that "At constant temperature, the pressure of a fixed amount of gas varies inversely with the volume of the gas." That means if the pressure is doubled, the volume is halved.

The Boyle's law may be expressed mathematically as
$\mathrm{P} \propto \frac{1}{\mathrm{~V}},($ at constant T and n$)$
or $\mathrm{V} \propto \frac{1}{\mathrm{P}},($ at constant T and n$)$
Where,
$\mathrm{T}=$ temperature, $\mathrm{P}=$ pressure of the gas
$\mathrm{n}=$ number of moles of a gas and $\mathrm{V}=$ volume of the gas
$
\Rightarrow \mathrm{V}=\mathrm{k}_1 \frac{1}{\mathrm{P}}
$

k₁ is the proportionality constant whose value depends upon the following factors.

1. Amount of gas

2. Temperature

On rearranging the above equation we can write

$\mathrm{PV}=\mathrm{k}_1$
i.e., 'PV' is constant at constant temperature and for a fixed amount of the gas. So, Boyle's law can also be stated as "At constant temperature, the product of pressure and volume of a fixed amount of a gas remains constant."
Now if the initial pressure and volume of a fixed amount of gas at constant temperature are P₁ and V₁, and after expansion, the new pressure of the gas is P₂ and volume occupied is V₂ then according to Boyle's law
$\begin{aligned} & \mathrm{P}_1 \mathrm{~V}_1=\mathrm{P}_2 \mathrm{~V}_2=\text { constant } \\ & \text { or } \frac{P_1}{P_2}=\frac{V_2}{V_1}\end{aligned}$

Relation between Density and Pressure

According to Boyle's law at constant temperature and constant mass
$V \propto \frac{1}{P}$, As T and mass are constant
$V \propto \frac{1}{d}$, Here d is the density
As $\mathrm{V}=$ Mass/density
so $\quad \frac{1}{d} \propto \frac{1}{P}$
that is, $\mathrm{d} \propto P$ or $\mathrm{d}=\mathrm{K} / \mathrm{P}$
or $\log _{10} P=\log _{10} 1 / V+\log _{10} K$
$
d_1 / P_1=d_2 / P_2
$
Various plots between P vs V
These plots are called Isotherms.

Physical Significance of Boyle's Law :

As discussed before, with increasing pressure, the density of the air increases at a constant temperature. This indicates that gases are compressible. The same effect can be seen in daily life. Air is denser at the sea level and as the altitude increases air pressure decreases, which means air now becomes less denser. So, less oxygen molecules occupy the same volume. Therefore oxygen in the air becomes insufficient for normal breathing, As a result, altitude sickness occurs with symptoms like headache, and uneasiness. That is why mountaineers have to carry oxygen cylinders with them in case of emergency to restore normal breathing.

Recommended topic video on( Boyle's Law)

Some Solved Examples

Example 1: Why Boyle’s law cannot be used to calculate the volume of gas that is changed from the initial state to the final state during adiabatic expansion?

1)Because temperature is increased in adiabatic expansion

2) Because temperature is decreased in adiabatic expansion

3)Because temperature is constant in adiabatic expansion

4)Nothing can be said

Solution

Boyle’s law is applicable only for the processes when the temperature is constant. In adiabatic expansion, the temperature of the gas is lowered. Thus, Boyle’s law cannot be used in this case.
Hence, the answer is the option (2).

Example 2: For a particular gas in a cylinder of 10L, the pressure of a gas is 2 atm. If the pressure of the gas decreases up to 50%, then find the volume (in L) of gas at a constant temperature.

1) 20

2)24

3)18

4)15

Solution

Conditions we have given,
n = constant and T = constant
Now,
$P_1=2 \mathrm{~atm}$ and $V_1=10 L$$P_2=2-\left(\frac{2 \times 50}{100}\right)=1 \mathrm{~atm}$

Now, for $V_2$ we will use Boyle’s law as given below
$\Rightarrow P_1 V_1=P_2 V_2$
$\Rightarrow 2 \times 10=1 \times V_2$
$\Rightarrow V_2=20 L$
Hence, the answer is the option (1).

Example 3: At constant temperature, in a given mass of ideal gas -

1)The ratio of pressure and volume always remains constant

2)Volume always remains constant

3)Pressure always remains constant

4) The product of pressure and volume always remains constant

Solution

According to Boyle's law, at constant temperature and moles, Pressure is inversely proportional to the volume of gas.
P₁V₁ = P₂V₂ i.e PV = Constant

Hence, the answer is the option (4).

Example 4: Two flasks of equal volume are connected by a narrow tube (negligible volume), all at 27º C, and contain 0.70 moles of H₂ at 0.5 atm. One flask is then immersed into a bath at 127º C, while the other remains at 27º C. The number of moles of H₂ in flask 1 and flask 2 are:

1) Moles in flask 1 = 0.4, Moles in flask 2 = 0.3

2) Moles in flask 1 = 0.2, Moles in flask 2 = 0.3

3) Moles in flask 1 = 0.3, Moles in flask 2 = 0.2

4) Moles in flask 1 = 0.4, Moles in flask 2 = 0.2

Solution

To find the number of moles of H₂ in flask 1 and flask 2,

We can use PV = nRT

Two flasks of equal Volume, Let Vol. of each flask be 'V' L,

Total volume = V+V = 2V

Now. PV = nRT

So,

$0.5 \times {V}=0.7\times{R} \times 300$${~V}=420 {R}$

In the 2nd case, the pressure will be the same in both flasks, and the sum of moles of gas will be 0.7. But Volume will be half 420R/2=210R

Now,

Flask 1-

PV=aRT

P X 210R=a X R X 300

a = 0.7P

Flask2-

PV=bRT

P X 210 R = b X R X 400

b = 0.525P

So,

a+b=1.225P=0.7

P=0.571atm

Now,

a = 0.7 X 0.571 = 0.399 = 0.4 moles

b = 0.525 X 0.571 = 0.299 = 0.3 moles

Hence, the answer is the option (1).

Example 5: At constant temperature, in a given mass of ideal gas -

1)The ratio of pressure and volume always remains constant

2)Volume always remains constant

3)Pressure always remains constant

4) The product of pressure and volume always remains constant

Solution

According to Boyle's law at constant temperature and moles, Pressure is inversely proportional to the volume of gas.

P₁V₁ = P₂V₂

i.e PV = Constant

Hence, the answer is the option (4).

Summary

The inverse relationship between the pressure and volume of a gas if the temperature remains constant is known as Boyle's law. Formulated by Robert Boyle, it states that the greater the volume, the smaller the pressure, and vice versa, so long as the temperature remains constant. Mathematically, this may be expressed as, proving that pressure times volume is constantly equal to the same value for the amount of gas under consideration. Boyle's Law forms the basis for gas behavior, such as within confined spaces.