Derivation of Ideal Gas Equation - Definition, Formula, Limitations, FAQs

The ideal gas equation is usually called the ideal gas law. It contains in one mathematical expression the basic principles relating to pressure, volume, temperature, and number of moles of gas. This formula is always written as PV=nRT, where P = pressure, V = volume, n = number of moles, R = universal gas constant, and T = Temperature in Kelvin. It is an integrated law that has Boyle’s Law , Charles’ Law , Gay Lussac’s Law , and all such laws combined into a single equation.

This Story also Contains

1. Ideal Gas Equation
2. Some Solved Examples
3. Summary

Also check-

• NCERT Exemplar Class 11th Chemistry Solutions
• NCERT Exemplar Class 12th Chemistry Solutions
• NCERT Exemplar Solutions for All Subjects

Ideal Gas Equation

The ideal gas equation is an equation that is followed by the ideal gases. A gas that would obey Boyle's and Charles's laws under all the conditions of temperature and pressure is called an ideal gas.
As discussed the behavior of gases is described by certain laws such as Avogadro's Law, Boyle's Law, and Charles' Law.

According to Avogadro's Law; V∝n(P and T constant ) According to Boyle's Law; V∝1P ( T and n constant) According to Charles' Law; V∝T(P and n constant) Combining the three laws; we get:

V∝nTPV=RnTP

' R ' is the proportionality constant. On rearranging the above equation we get the following:
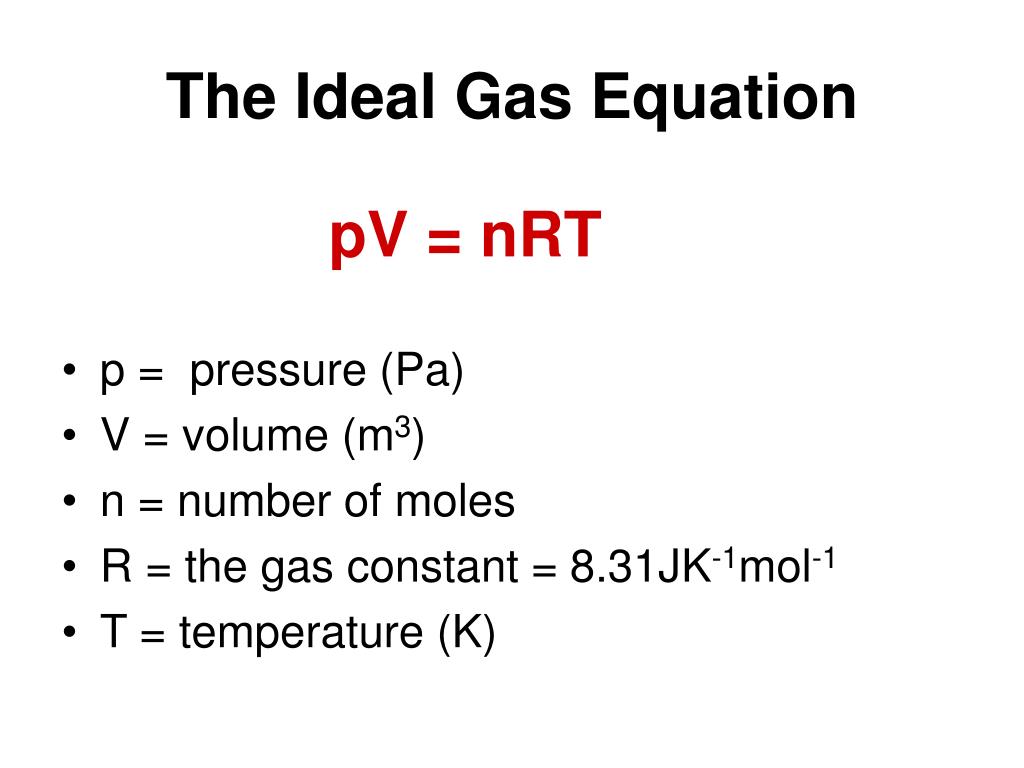
PV=nRT

This is the ideal gas equation as it is obeyed by the hypothetical gases called ideal gases under all conditions

Universal Gas Constant or Ideal Gas ConstantR or S : Molar gas constant or universal gas constant Values of R=0.0821lit, atm, K−1, mol−1
=8.314 joule K−1 mol−1=8.314×107ergK−1 mol−1=2calK−1 mol−1

For a single molecule, the gas constant is known as Boltzmann constant ( k ) and unit (m2kgs−2 K−1)
$
k=RN0=1.38×10−3 J/deg− abs / molecule =1.38×10−16erg/ deg-abs / molecule
$

Also read :

• NCERT notes Class 11 Chemistry Chapter 2 Structure of Atom
• NCERT solutions for Class 11 Chemistry Chapter 2 Structure of Atom
• NCERT Exemplar Class 11 Chemistry Solutions Chapter 2 Structure of Atom

Combined gas law

Boyle's and Charles' Law can be combined to give a relationship between the three variables P, V, and T. The initial temperature, pressure, and volume of a gas are T1,P1 and V1. With the change in either of the variables, all three variables change to T2,P2, and V2. Then we can write:

P1V1T1=nR and P2V2T2=nR
Combining the above equations we get

P1V1T1=P2V2T2=nR
The above relation is called the combined gas law.

Density and Molar Mass of a Gaseous Substance

Ideal gas equation is PV=nRT
On rearranging the above equation, we get
nV=PRTn(N0. of moles )= Given mass (m) Molar mass (M)

Putting the value of ' n ' from equation (iii) in equation (ii), we get:
mMV=PRT

We know that density (d) is mass (m) per unit volume (V)
d=mV

Replacing mV in eq. (iv) with d (density), then equation (iv) becomes:

dM=PRT

Rearranging the above equation, we get M=dRTP

The above equation gives the relation between the density and molar mass of a gaseous substance

Recommended topic video on(Derivation of Ideal Gas Equation)

Some Solved Examples

Example 1: A refrigeration tank holding 5 liters of gas with molecular formula C2Cl2 F4 at 298 Kand 3 atm pressure developed a leak. When the leak was discovered and repaired, the tank had lost 76 g of the gas. What was the pressure of the gas remaining in the tank at 298 K?

1) 0.83

2)1.23

3)0.67

4)2.23

Solution

First, understand the question.

there is a tank holding 5 liter of gas with molecular formula C₂Cl₂ F4 at 298K at pressure 3 atm

after leaking 76 g of gas lost

We have to find the pressure of the gas remaining in the tank at 298K.

Now,

given some gas is lost in grams, so we need to find the initial quantity in grams from PV=nRT and n = weight/molar mass

then we will have the quantity in grams of remaining gas.

Then we have to convert it into moles and find p from PV=nRT.

According to the ideal gas equation, we have:

pV = nRT

Thus, n=3×50.082×298=0.613moles

Thus, the total weight of the gas originally present = 0.613 x 171g
= 105g
Now, 76g of the gas is already lost, thus the remaining gas:
= 105 - 76 = 29g
Thus, total moles of the gas remaining = 29/171 = 0.17 moles
Again, according to the ideal gas equation, we have:
PV = nRT

Thus, P=0.17×0.082×2985=0.83 atm

Therefore, Option(1) is correct.

Example 2: Two identical flasks contain gases A and B at the same temperature. If density of A=3 g/dm3 and that of B=1.5 g/dm3and the molar mass of A=12 of B , the ratio of pressure exerted by gases is :

1)PAPB=2

2)PAPB=1

3) PAPB=4

4)PAPB=3

Solution

As we learned in Ideal Gas Law in terms of density -

PM=dRT

- wherein
where
d - density of gas
P - Pressure
R - Gas Constant
T - Temperature
M - Molar Mass

PA=3RTMA;PB=1.5RTMBPAPB=2MBMA=2×2MAMA=4 $;$

Example 3: NaClO3 is used, even in spacecraft, to produce O2. The daily consumption of pure O2 by a person is 492L at 1 atm,300 K . How much amount of NaClO3 , in grams, is required to produce O2 for the daily consumption of a person at 1 atm,300 K ? _______.

NaClO3( s)+Fe(s)→O2( g)+NaCl(s)+FeO(s)

R=0.082 L atm mol−1 K−1

1) 2130

2)2140

3)2456

4)2458

Solution

moles of NaClO3 = moles of O2

moles of O2=PVRT=1×4920.082×300=20ml

Mass of NaClO3=20×106.5=2130 g

Example 4: The volume occupied by 4.75 g of acetylene gas at 50^oC and 740 mmHg pressure is _______L. (Rounded off to the nearest integer)

[Given R = 0.0826 L atm K^-1 mol ^-1]

1) 5

2)5.2

3)6

4)7

Solution

T=50∘C=323.15 KP=740 mm of Hg=740760 atm V=? moles (n)=4.7526 V=4.7526×0.0821×323.15740×760 V=4.97≃5Lit

Answer ; 5

Example 5: A car tyre is filled with nitrogen gas at 35 psi and 27∘C. It will burst of pressure exceeds 40 psi. The temperature in ∘C at which the car tyre will burst is ______ (Rounded off to the nearest integer)

1) 70

2)81

3)85

4)78

Solution

T₁ = 27^oC = 273 + 27 K = 300 K

P1 T1=P2 T235300=40 T2 T2=40×30035 T2=342.86 K T2=69.85∘C≃70∘C

Hence, the answer is (70).

Summary

The ideal gas equation is based on the assumption that the particles of the gas are always in constant, random motion and that their interaction with one another is only through elastic collisions, thus exerting negligible intermolecular forces. This idealization serves to simplify the really complex nature of real gases and hence makes the calculations easier and predictions more at hand. However, the formula becomes much less accurate for real gases under very high pressure or extremely low-temperature conditions, where ideal behavior deviates. The ideal gas equation has immense importance that cuts across a number of scientific and industrial fields.

Also read -

• NCERT Solutions for Class 11 Chemistry
• NCERT Solutions for Class 12 Chemistry
• NCERT Solutions for All Subjects

Related Topics link

• NMR Spectroscopy
• Proton Neutron Discovery
• Protons
• Proton Neutron Discovery
• Atomic number mass number