Faraday’s Laws of Electrolysis

Michael Faraday's work on electrolysis led to the formulation of two fundamental laws that state that the amount of chemical change (such as the amount of substance deposited or dissolved) at an electrode during electrolysis is directly proportional to the quantity of electric charge passed through the electrolyte. Faraday's Second Law of Electrolysis (1834): This law states that the amounts of different substances altered at an electrode by the same quantity of electricity are proportional to their equivalent weights.

JEE Main 2025: Chemistry Formula | Study Materials | High Scoring Topics | Preparation Guide

JEE Main 2025: Syllabus | Sample Papers | Mock Tests | PYQs | Study Plan 100 Days

NEET 2025: Syllabus | High Scoring Topics |  PYQs

This Story also Contains

1. Faraday’s Laws of Electrolysis
2. Some Solved Examples
3. Summary

Faraday’s Laws of Electrolysis

Faraday's laws of electrolysis are fundamental principles in electrochemistry that relate to the amount of substance transformed during electrolysis to the electric charge passed through the electrolyte. Faraday gives two laws which are the Faraday First Law and the Faraday Second Law

Faraday's First Law of Electrolysis

The amount of chemical change (or substance transformed) at an electrode during electrolysis is directly proportional to the total electric charge passed through the electrolyte.

According to Faraday's first law, "The amount of substance or quantity of chemical reaction at the electrode is directly proportional to the quantity of electricity passed into the cell".

$\begin{aligned} & \text { W or } \mathrm{m} \propto \mathrm{q} \\ & \mathrm{W} \propto \text { it } \\ & \mathrm{W}=\mathrm{Zit} \\ & \mathrm{Z}=\frac{\mathrm{M}}{\mathrm{nf}}=\frac{\text { Eq.wt }}{96500} \\ & \mathrm{Z}=\text { Electrochemical equivalent } \\ & \mathrm{M}=\text { Molar Mass } \\ & \mathrm{F}=96500 \mathrm{C} \\ & \mathrm{n}=\text { Number of electrons transfered } \\ & \mathrm{q}=\text { amount of charge utilized }\end{aligned}$

Faraday's Second Law of Electrolysis

The amounts of different substances transformed or deposited by the same quantity of electricity passing through an electrolyte are proportional to their equivalent weights. In other words, for a given charge, the mass of a substance transformed is inversely proportional to its equivalent weight.

Electrochemical equivalent is the amount of the substance deposited or liberated by one-ampere current passing for one second (that is, one coulomb of charge.)
One gram equivalent of any substance is liberated by one faraday.

$\begin{aligned} & \text { Eq. Wt. }=\mathrm{Z} \times 96500 \\ & \frac{\mathrm{W}}{\mathrm{E}}=\frac{\mathrm{q}}{96500} \\ & \mathrm{w}=\frac{\mathrm{E} \cdot \mathrm{q}}{96500} \\ & \mathrm{~W}=\frac{\text { Eit }}{96500}\end{aligned}$

As w = a x l x d that is, area x length x density
Here a = area of the object to be electroplated
d = density of metal to be deposited
l = thickness of layer deposited
Hence from here, we can predict charge, current strength, time, thickness of deposited layer etc.

NOTE: One faraday is the quantity of charge carried by one mole of electrons.

$\begin{aligned} & 1 \mathrm{~F}=1.6 \times 10^{-19} \times 6.023 \times 10^{23} \\ & \simeq 96500 \text { Coulombs }\end{aligned}$

Recommended topic video on (Faraday’s Laws of Electrolysis)

Some Solved Examples

Example.1

1. A current of 10.0 A flows for 2.00 h through an electrolytic cell containing a molten salt of metal X. This results in the decomposition of 0.250 mol of metal X at the cathode. The oxidation state of X in the molten salt is : (F= 96,500 C)

1)1+

2)2+

3) (correct)3+

4)4+

Solution

i = 10 A, t = 2hr

No. of moles $=\frac{I t}{96500 \times(n-\text { factor })}$

$\begin{aligned} & \therefore \text { moles of } \mathrm{e}^{-}=\frac{10 \times 2 \times 60 \times 60}{96500 \times \mathrm{n}-\text { factor }} \\ & \therefore 0.25=\frac{10 \times 2 \times 60 \times 60}{96500 \times \mathrm{X}} \\ & \therefore 0.75=0.25 \times(X) \\ & \Rightarrow X=3 \\ & \therefore \text { Metal } \mathrm{X} \text { is present in the form of } \mathrm{X}^{3+}\end{aligned}$

Hence, the answer is the option (3).

Example.2

2. How many electrons would be required to deposit 6.35 g of copper at the cathode during the electrolysis of an aqueous solution of copper sulphate ? (Atomic mass of copper = 63.5 u, N_A=Avogadro’s constant) :

1)$\frac{N_A}{20}$

2)$\frac{N_A}{10}$

3) (correct)$\frac{N_A}{5}$

4)$\frac{N_A}{2}$

Solution

According to the reaction:

$\mathrm{Cu}^{2+}+2 e^{-} \rightarrow \mathrm{Cu}$

We require 2 moles of electrons or 2N_A electrons to deposit 1 mol or 63.5 g of Cu.

So, 6.35 g of Cu, requires $\frac{2 \mathrm{~N}_{\mathrm{A}}}{10}$ electrons.

After simplifying.

$\frac{2 \mathrm{~N}_{\mathrm{A}}}{10}=\frac{\mathrm{N}_{\mathrm{A}}}{5}$

Hence, the answer is the option(3)

Example.3

3. Aluminium oxide may be electrolysed at 1000°C to furnish aluminium metal ( At. Mass = 27 amu,1 Faraday= 96,500 Coulombs ) The cathode reaction is

$A l^{3+}+3 e^{-} \rightarrow A l^0$

To prepare 5.12 kg of aluminium metal by this method would require

1) (correct)$5.49 \times 10^7 \mathrm{C}$ of electricity

2)$1.83 \times 10^7 \mathrm{C}$ of electricity

3)$5.49 \times 10^4 \mathrm{C}$ of electricity

4)$5.49 \times 10^1 \mathrm{C}$ of electricity

Solution

$\begin{aligned} & \text { Moles of } \mathrm{Al}=\frac{5.12 \times 1000}{27} \\ & \approx 190 \\ & \therefore \text { Moles of } \mathrm{e}^{-}=3 \times 190 \therefore \text { Total charge }=3 \times 190 \times 96500 \\ & \simeq 5.49 \times 10^7 \mathrm{C}\end{aligned}$

Hence, the answer is the option (1).

Example.4

4. A solution of $\mathrm{Ni}\left(\mathrm{NO}_3\right)_2$ is electrolysed between platinium electrodes using 1 faraday electricity. How many mole of Ni will be deposited at the cathode?

1) (correct)0.5

2)0.05

3)1

4)0.25

Solution

The reaction : $\mathrm{Ni}\left(\mathrm{NO}_3\right)_2 \rightarrow \mathrm{Ni}^{2+}+2 \mathrm{NO}_3^{-}$ at cathode $: \mathrm{Ni}^{2+}+2 \mathrm{e}^{-} \rightarrow \mathrm{Ni}$
$\begin{aligned} & 2 \text { mole of } \mathrm{e}^{-} \text {are required for } 1 \text { mole of } \mathrm{Ni} \\ & \therefore \quad 0.5 \mathrm{~mole} \text { of } \mathrm{Ni} \text { is deposited by } 1 \mathrm{~mole} \text { of electron }\end{aligned}$
Hence, the answer is the option (1).

Example.5

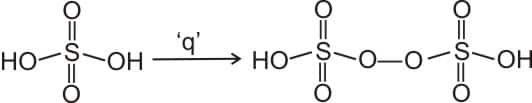
5. The product obtained from the electrolytic oxidation of acidified sulphate solutions, is :

1)$\mathrm{HSO}_4^{-}$

2) (correct)$\mathrm{HO}_3 \mathrm{SOOSO}_3 \mathrm{H}$

3)${ }_{)} \mathrm{HO}_2 \mathrm{SOSO}_2 \mathrm{H}$

4)$\mathrm{HO}_3 \mathrm{SOSO}_3 \mathrm{H}$

Solution

As we have learnt,

Electrolytic oxidation of acidified sulphate solution from peroxydisulphuric acid.

Hence, the correct answer is option (2).

Summary

Faraday's laws allow the calculations of the amount of substance that will be deposited or dissolved during electrolysis, based on the amount of electric charge passed through the electrolyte. Electroplating and Metallurgy in these laws are essential in industries for electroplating, where they help control the thickness and quality of metal coatings on surfaces. Battery Design The understanding of electrolysis helps in designing more efficient batteries and fuel cells, as it relates to the movement of ions and the overall electrochemical reactions involved.