Relation between Kp and Kc

The equilibrium constants ( K_p ) and ( K_c ) are fundamental concepts in chemical equilibrium, describing the relationship between the concentrations or partial pressures of reactants and products in a chemical reaction at equilibrium. In chemical thermodynamics, the equilibrium constants ( K_p ) and ( K_c ) provide crucial information about the position of equilibrium for a reaction.

NEET 2025:  Mock Test Series | Syllabus | High Scoring Topics | PYQs

JEE Main: Study Materials | High Scoring Topics | Preparation Guide

JEE Main: Syllabus | Sample Papers | Mock Tests | PYQs

This Story also Contains

1. Relation Between Kp And Kc
2. Some Solved Examples
3. Summary

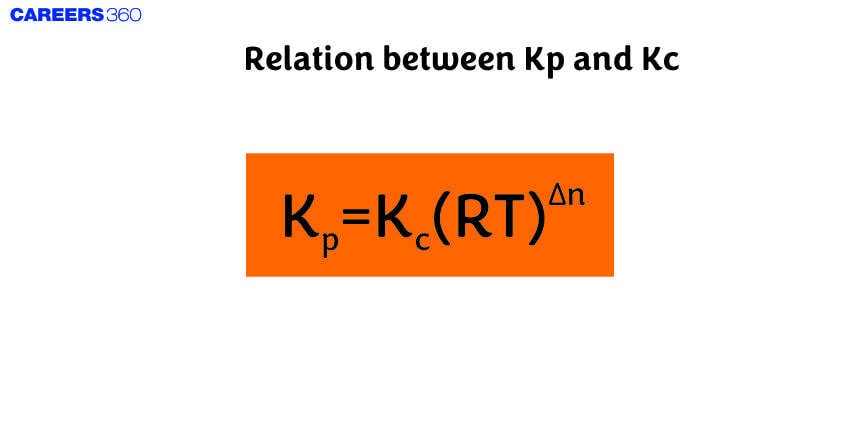
K_c is the equilibrium constant expressed in terms of the concentrations of reactants and products, while ( K_p ) is expressed in terms of the partial pressures of gases. The relationship between ( Kp ) and ( Kc ) is particularly important for understanding how changes in pressure and temperature affect chemical equilibria.

The specific relationship between ( Kp ) and ( Kc ) was derived later as the field of chemical thermodynamics evolved. The equilibrium constants ( K_p ) and ( K_c ) are extremely helpful in various ways. They provide insight into the extent to which a reaction will proceed to form products or remain as reactants. A large value of ( K_c ) or ( K_p ) indicates a reaction that favors the formation of products, while a small value suggests a reaction that favors the reactants.

Relation Between K_p And K_c

Let us suppose we react:
${\mathrm{n}}_1\mathrm{A}(\mathrm{g})+{\mathrm{n}}_2\mathrm{B}(\mathrm{g})\leftrightharpoons {\mathrm{n}}_3\mathrm{C}(\mathrm{g})+{\mathrm{n}}_4\mathrm{D}(\mathrm{g})$

The equilibrium constant ${\mathrm{K}}_{\mathrm{c}}$ for this reaction is given as:
${\mathrm{K}}_{\mathrm{c}}=\frac{[\mathrm{C}{]}^{{\mathrm{n}}_3}[\mathrm{D}{]}^{{\mathrm{n}}_4}}{[\mathrm{A}{]}^{{\mathrm{n}}_1}[\mathrm{B}{]}^{{\mathrm{n}}_2}}$

For the reaction:
${\mathrm{n}}_1\mathrm{A}(\mathrm{g})+{\mathrm{n}}_2\mathrm{B}(\mathrm{g})\leftrightharpoons {\mathrm{n}}_3\mathrm{C}(\mathrm{g})+{\mathrm{n}}_4\mathrm{D}(\mathrm{g})$

The equilibrium constant ${\mathrm{K}}_{\mathrm{p}}$ is given as:
$K_p=\frac{{\left(P_C\right)}^{n_3}{\left(P_D\right)}^{n_4}}{{\left(P_A\right)}^{n_1}{\left(P_B\right)}^{n_2}}$

Now, from the Ideal gas Equation
$\mathrm{PV}=\mathrm{nRT}$

$\begin{array}{rl}& \mathrm{P}=\frac{\mathrm{n}}{\mathrm{V}}\mathrm{RT}\\ & \mathrm{P}=\mathrm{CRT}\end{array}$

Putting the value of $P$ in terms of $C$ in the expression for $K_P$
$\begin{array}{rl}& {\mathrm{K}}_{\mathrm{p}}=\frac{[\mathrm{C}{]}^{{\mathrm{n}}_3}(\mathrm{RT}{)}^{{\mathrm{n}}_3}[\mathrm{D}{]}^{{\mathrm{n}}_4}(\mathrm{RT}{)}^{{\mathrm{n}}_4}}{[\mathrm{A}{]}^{{\mathrm{n}}_1}(\mathrm{RT}{)}^{{\mathrm{n}}_1}[\mathrm{B}{]}^{{\mathrm{n}}_2}(\mathrm{RT}{)}^{{\mathrm{n}}_2}}\\ & {\mathrm{K}}_{\mathrm{P}}=\frac{[\mathrm{C}{]}^{{\mathrm{n}}_3}[\mathrm{D}{]}^{{\mathrm{n}}_4}}{[\mathrm{A}{]}^{{\mathrm{n}}_1}[\mathrm{B}{]}^{{\mathrm{n}}_2}}[\mathrm{RT}{]}^{{\mathrm{n}}_3+{\mathrm{n}}_4)-({\mathrm{n}}_1+{\mathrm{n}}_2)}\end{array}$

Putting $\mathrm{\Delta }{\mathrm{n}}_{\mathrm{g}}=({\mathrm{n}}_3+{\mathrm{n}}_4)-({\mathrm{n}}_1+{\mathrm{n}}_2)$, we have
${\mathrm{K}}_{\mathrm{P}}={\mathrm{K}}_{\mathrm{C}}(\mathrm{RT}{)}^{\mathrm{\Delta }{\mathrm{n}}_5}$

It can be seen that:
- When $\mathrm{\Delta }{\mathrm{ng}}_{\mathrm{g}}=0$, then ${\mathrm{K}}_{\mathrm{P}}={\mathrm{K}}_{\mathrm{C}}$
. When $\mathrm{\Delta }{\mathrm{ng}}_{\mathrm{g}}>0$, then ${\mathrm{K}}_{\mathrm{P}}>{\mathrm{K}}_{\mathrm{Q}}$
When $\mathrm{\Delta }{\mathrm{n}}_{\mathrm{g}}<0$, then ${\mathrm{K}}_{\mathrm{P}}<{\mathrm{K}}_{\mathrm{C}}$

Recommended topic video on(Relation between Kp and Kc)

Some Solved Examples

Example 1. An amount of solid NH₄HS is placed in a flask already containing ammonia gas at a certain temperature and 0.50 atm pressure. Ammonium hydrogen sulphide decomposes to yield NH₃ and H₂S gases in the flask. When the decomposition reaction reaches equilibrium, the total pressure in the flask rises to 0.85 atm. The equilibrium constant forNH₄HS decomposition at this temperature is

1)0.30

2)0.18

3) 0.17

4)0.11 (correct)

$$\begin{array}{rl}& \text{ Solution }\\ & \begin{array}{lc}& {\mathrm{NH}}_4\mathrm{HS}(\mathrm{s})\rightleftharpoons {\mathrm{NH}}_3(\mathrm{g})+{\mathrm{H}}_2\mathrm{S}(\mathrm{g})\\ \text{ Initial pressure }& 0& 0.5& 0\\ \text{ At eq. }& 0& 0.5+\mathrm{x}& \mathrm{x}\end{array}\end{array}$$

Total pressure = 0.5 + 2x = 0.84

x = 0.17 atm

Now,
$\begin{array}{rl}& {\mathrm{K}}_{\mathrm{p}}={\mathrm{pNH}}_3\times {\mathrm{pH}}_2\mathrm{S}\\ & {\mathrm{K}}_{\mathrm{p}}=(0.5+0.17)(0.17)=0.11{\mathrm{atm}}^2\end{array}$

Example 2. Two solids dissociate as follows

$\begin{array}{rl}& A(s)\rightleftharpoons B(g)+C(g);K_{p1}=xat{m}^2\\ & D(s)\rightleftharpoons C(g)+E(g);K_{p2}=yat{m}^2\end{array}$

The total pressure when both the solids dissociate simultaneously is :

1) $(x+y)\mathrm{atm}2)$
2) $\sqrt{x+y}\mathrm{atm}$
3) $x^2+y^{2}atm$
4) $($ correct) $2(\sqrt{x+y})\mathrm{atm}$

Solution

Relation between pressure and concentration -
$PV=nRT$
or $P=\frac{n}{V}RT$
or $P=CRT$
$R=0.0831$ bar inter $/\mathrm{mol}K$

- wherein

P is pressure in Pa. C is concentration in mol/liter. T is the temperature in kelvin

As we have learned in total pressure at equilibrium
$\begin{array}{rl}& A_{(s)}\rightleftharpoons B_{(g)}+C_{(g)}{K}_p={\text{ xatm }}^2\\ & D_{(s)}\rightleftharpoons C_{(g)}+E_{(g)}K{p}_2={\text{ yatm }}^2\\ & p_1+p_2{p}_2\\ & K{p}_1=p_1(p_1+p_2)\text{ Kp }{p}_2=p_2(p_1+p_2)\\ & K{p}_2=p_2{(p_1+p_2)}^2\\ & x+y={\left(p_{1+}{p}_2\right)}^2\\ & \left(p_{1+}{p}_2\right)=\sqrt{x+y}\\ & P_{\text{total }}=P_b+P_c+P_e\\ & =2\left(p_{1+}{p}_2\right)=2\sqrt{x+y}\end{array}$

Hence, the answer is the option (4).

Example 3. Consider the reaction ${\mathrm{N}}_2(\mathrm{g})+3{\mathrm{H}}_2(\mathrm{g})\rightleftharpoons 2{\mathrm{NH}}_3(\mathrm{g})$. The equilibrium constant of the above reaction is $K_p$. If pure ammonia is left to dissociate, the partial pressure of ammonia at equilibrium is given by ( Assume that $P_{N{H}_3}<<p_{\text{total }}$ at equilibrium )

$\begin{array}{rl}& \text{ 1) (correct) }\frac{3^{\frac{3}{2}}{K}_p^{\frac{1}{2}}{P}^2}{16}\\ & \text{ 2) }\frac{3^{\frac{3}{2}}{K}_p^{\frac{1}{2}}{P}^2}{4}\\ & \text{ 3) }\frac{K_p^{\frac{1}{2}}{P}^2}{4}\\ & \text{ 4) }\frac{K_P^{\frac{1}{2}}{P}^2}{16}\end{array}$

Solution
${\mathrm{N}}_2+3{\mathrm{H}}_2\rightleftharpoons 2{\mathrm{NH}}_3\text{ Equilibrium Constant }=K_p$

Now,
$\begin{array}{rl}& 2{\mathrm{NH}}_3\rightleftharpoons {\mathrm{N}}_2+3{\mathrm{H}}_2;K_p^{\prime }=\frac{1}{K_p}\\ & P_{\text{total }}=P=P_{{\mathrm{N}}_2}+P_{{\mathrm{H}}_2}+P_{{\mathrm{NH}}_3}\end{array}$

Due to $P_{{\mathrm{NH}}_3}<<P_{\text{Total }}$
${\mathrm{P}}_{\text{total }}\approx {\mathrm{P}}_{{\mathrm{N}}_2}+{\mathrm{P}}_{{\mathrm{H}}_2}$

Total moles are 4,1 of ${\mathrm{NH}}_3$, and 3 of ${\mathrm{H}}_2$.
Partial Pressure of $\mathrm{A}=\frac{\text{ moles of }\mathrm{A}}{\text{ Total moles }}\times$ Total Pressure

$\begin{array}{rl}& {\mathrm{P}}_{{\mathrm{N}}_2}=\frac{1}{4}\times \mathrm{P}\text{ and }{\mathrm{P}}_{{\mathrm{H}}_2}=\frac{3}{4}\times \mathrm{P}\\ & \frac{1}{K_P}=\frac{P_{N_2}{\left(P_{H_3}\right)}^3}{{\left(P_{N_3}\right)}^2}=\frac{\left(\frac{P}{4}\right){\left(\frac{3P}{4}\right)}^3}{{\left(P_{N{H}_3}\right)}^2}\\ & {\left(P_{N{H}_3}\right)}^2=\frac{3^3{P}^4}{4^4}{K}_p\end{array}$

Example 4. For the reaction, ${\mathrm{CO}}_{(\mathrm{g})}+{\mathrm{Cl}}_{2(\mathrm{g})}\rightleftharpoons {\mathrm{COCl}}_{2(\mathrm{g})}$ the value of $\frac{{\mathrm{K}}_{\mathrm{p}}}{{\mathrm{K}}_{\mathrm{c}}}$ is equal to

1) $($ correct) $\frac{1}{RT}$
2)RT
3) $\sqrt{\mathrm{RT}}$
4) 1.0

Solution

Relation between $Kp$ and $K_c-K_p=K_c(RT{)}^{\mathrm{△}n}$
Now, for the given reaction, ${\mathrm{CO}}_{(\mathrm{g})}+{\mathrm{Cl}}_{2(\mathrm{g})}\rightleftharpoons {\mathrm{COCl}}_{2(\mathrm{g})}$
$\begin{array}{rl}& \mathrm{\Delta }\mathrm{n}=-1\\ & \therefore {\mathrm{K}}_{\mathrm{p}}={\mathrm{K}}_{\mathrm{c}}(\mathrm{RT}{)}^{-1}\end{array}$

Thus $\frac{{\mathrm{K}}_{\mathrm{p}}}{{\mathrm{K}}_{\mathrm{c}}}=\frac{1}{\mathrm{RT}}$

Hence, the answer is the option (1).

Example 5. For the reaction,
${\mathrm{SO}}_{2(\mathrm{g})}+\frac{1}{2}{\mathrm{O}}_{2(\mathrm{g})}\rightleftharpoons {\mathrm{SO}}_{3(\mathrm{g})}$ if ${\mathrm{K}}_{\mathrm{P}}={\mathrm{K}}_{\mathrm{C}}(\mathrm{RT}{)}^{\mathrm{x}}$ where the symbols have the usual meaning then the value of x is

1) -1
2) (correct) $-\frac{1}{2}$
3) $\frac{1}{2}$
4) 1

Solution

We know that

Relation between Kp and Kc -
${\mathrm{K}}_{\mathrm{p}}={\mathrm{K}}_{\mathrm{c}}(\mathrm{RT}{)}^{\mathrm{\Delta }\mathrm{n}}$

According to the data given in the question
${\mathrm{K}}_{\mathrm{C}}(\mathrm{RT}{)}^{\mathrm{\Delta }\mathrm{n}}={\mathrm{K}}_{\mathrm{C}}(\mathrm{RT}{)}^{\mathrm{x}}$

For the given reaction
$\begin{array}{rl}& {\mathrm{SO}}_2(\mathrm{g})+\frac{1}{2}{\mathrm{O}}_2(\mathrm{g})\rightleftharpoons {\mathrm{SO}}_3(\mathrm{g})\\ & \mathrm{\Delta }\mathrm{n}=1-\frac{3}{2}=-\frac{1}{2}\end{array}$

Hence, the value of $x$ is $-\frac{1}{2}$

Hence, the answer is an option (2).

Summary

The relationship between ( K_c ) (the equilibrium constant for concentration) and ( K_p ) (the equilibrium constant for partial pressures) is crucial in understanding chemical equilibria, especially for reactions involving gases. This relation has various benefits such as predicting reaction behavior: Knowing the values of ( Kc ) and ( Kp ) helps to predict the direction and extent of a reaction under varying conditions.