Reversible, Irreversible, Polytropic Process

A reversible process in thermodynamics is a hypothetical process in which a system undergoes a change of state to a given final state such that the process can be reversed and that change of state made nil—a net change nil, in both the system and its surroundings. Reversible processes occur at an infinitely slow pace since a system is considered to be in a condition of complete equilibrium at all instances of time during the process. They are primarily used for theoretical purposes and produce maximum efficiency among the limiting values for actual processes.

This Story also Contains

1. Reversible or Quasi-Static Process
2. Irreversible Process
3. Polytropic Process
4. Some Solved Examples
5. ∴Q=−w=Summary

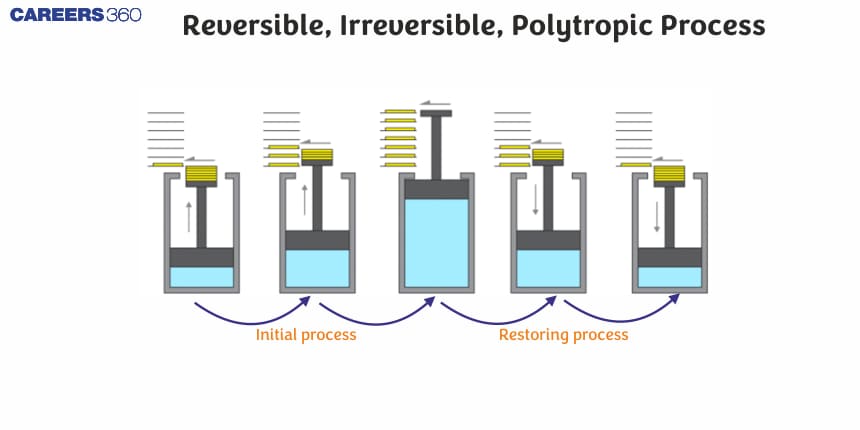
Reversible or Quasi-Static Process

It is carried out in such a way that the system remains in a state of equilibrium. All changes occurring at any part of the process will be exactly reversed when the change in carried out in the opposite direction.

• It involves slow changes during operation.

• This process may occur in any direction.

• Here driving force and opposing force differ with each other by a very small value.

Irreversible Process

Here the direction of change can not be reversed by small changes in variables. All processes occurring naturally are reversible

• It involves fast changes during operation.

• It is a unidirectional process.

• Here, driving and opposing forces differ by a large amount.

Polytropic Process

PV^m = constant is known as a general or polytropic process

If m= 0, the process is constant pressure.

If m = 1, the process is isothermal.

If m = $\gamma$ , the process is adiabatic.

Recommended topic video on (Reversible, Irreversible, Polytropic Process)

Some Solved Examples

Example 1: Mixing alcohol with the water is :

1)Reversible Process

2) Irreversible Process

3)Quasi-static Process

4)None of the above

Solution

An irreversible process is a process in which after the process has been completed in the forward and reverse orders, the system fails to return to the initial state. As water and alcohol can’t separate themselves, hence it is an irreversible process.
Hence, the answer is the option (2).

Example 2: In an irreversible process in which only Pressure-Volume is taking place at constant T and P the change in Gibbs free energy (dG) and change in entropy (dS) are related as :

1) dS is negative and dG positive $(dS){)}_{V,E}>0,(dG{)}_{T,P}>0$
2) dS is positive and dG is negative $(dS){)}_{V,E}>0,(dG{)}_{T,P}<0$

3) dS is negative and dG is negative $(dS){)}_{V,E}<0,(dG{)}_{T,P}<0$

4) Both are positive $(dS){)}_{V,E}=0,(dG{)}_{T,P}=0$

Solution

An irreversible process is a spontaneous process, so for spontaneity change in entropy (dS) must be positive and the change in Gibbs free energy (dG) should be negative.
Hence, the answer is the option (2).

Example 3: The irreversible process is due to

1)Lack of equilibrium

2)Involvement of dissipative forces

3) Both (1) and (2)

4)None of the above

Solution

Irreversible Process - The process is carried out in such a manner that the system is in thermodynamic equilibrium only at the initial & final stage of the process but not at the intermediate stage. In the case of any Irreversible Process at the intermediate stages of the process, the different state functions such as pressure, temperature, etc. are not defined. Both (1) and (2) are major reasons for irreversibility.

Hence, the answer is the option (3).

Example 4: Any series of operations so carried out that at the end, the system is back to its initial state is called

1)Boyle's Process

2)Adiabatic cycle

3) Cyclic process

4)Reversible process

Solution

In a cyclic process, a series of operations are carried out such that at the end of the series of processes, the system is back to its initial state.

Hence, the answer is the option (3).

$\therefore Q=-w=$Summary

Reversible processes are idealized ones in which changes are so gradual that the system is in all instances in thermodynamic equilibrium. During such a process, there are no dissipative effects, such as friction or unrestrained expansion. Reversibility implies that the system and the surroundings may be taken back to their original states without a net change. This concept may be used to define upper-efficiency limits for engines and other thermodynamic systems, even though reversibility is unattainable in practice. The main characteristics are that it involves infinitesimal changes, no production of entropy, and maximum workout. Examples include quasi-static processes and isothermal expansions/compressions in ideal gases. Reversible processes serve as benchmarks for assessing the performance of real, irreversible processes.

Irreversible processes are natural processes exemplified by spontaneous changes, friction, turbulence, unrestrained expansion, etc., which lead to entropy production. Work output from such processes is smaller than from their reversible counterparts since it is impossible to return the system and surroundings to their original states spontaneously, that is, without external intervention. Irreversibility is inherent in all real processes. Common examples include natural heat transfer, free expansion of gases, and real engine cycles. Irreversible processes point out the practical limitation in energy conversion and efficiency. Understanding them plays a crucial role in the design of systems that would help minimize losses of energy while maximizing performance, recognizing that some amount of irreversibility is always present in any real application.