Application of Even-Odd Properties in Definite Integrals

Integration is one of the important parts of Calculus, which applies to measuring the change in the function at a certain point. Mathematically, it forms a powerful tool by which slopes of functions are determined, the maximum and minimum of functions found, and problems on motion, growth, and decay, to name a few. These integration concepts have been broadly applied in branches of mathematics, physics, engineering, economics, and biology.

JEE Main 2025: Sample Papers | Mock Tests | PYQs | Study Plan 100 Days

JEE Main 2025: Maths Formulas  | Study Materials

JEE Main 2025: Syllabus | Preparation Guide | High Scoring Topics 

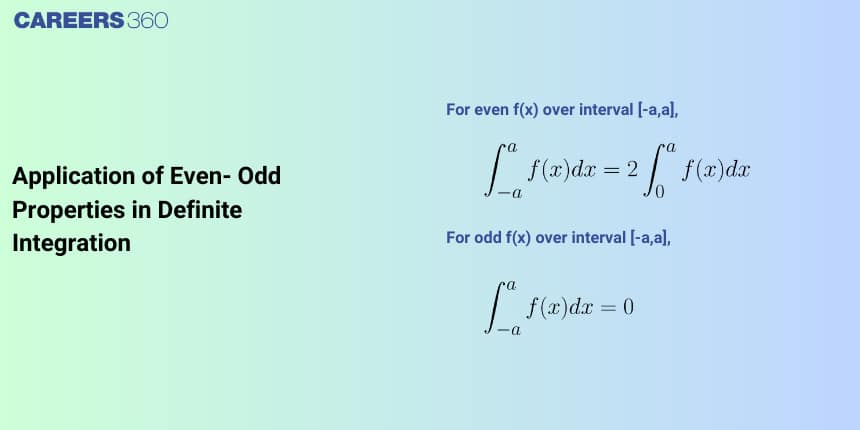
Application of Even-Odd Properties in Definite Integration

Definite integration calculates the area under a curve between two specific points on the x-axis.

Let f be a function of x defined on the closed interval [a, b]. F be another function such that $\frac{d}{d x}(F(x))=f(x)$ for all x in the domain of f, then $\int_a^b f(x) d x=[F(x)+c]_a^b=F(b)-F(a)$is called the definite integral of the function f(x) over the interval [a, b], where a is called the lower limit of the integral and b is called the upper limit of the integral.

Definite integrals have properties that relate to the limits of integration.

Property 1

$\int_{-a}^a f(x) d x=\left\{\begin{array}{ccc}0, & \text { if } f \text { is an odd function } & \text { i.e. } f(-x)=-f(x) \\ 2 \int_0^a f(x) d x, & \text { if } f \text { is an even function } & \text { i.e. } f(-x)=f(x)\end{array}\right.$

Proof:

$\begin{aligned} \int_{-\mathrm{a}}^{\mathrm{a}} \mathrm{f}(\mathrm{x}) \mathrm{dx} & =\underbrace{\int_{-a}^0 f(x) d x}_{x=-t}+\int_0^{\mathrm{a}} \mathrm{f}(\mathrm{x}) \mathrm{dx} \\ & =\int_a^0 f(-t)(-d t)+\int_0^a f(x) d x \\ & =\int_0^a f(-x)(d x)+\int_0^a f(x) d x \\ & =\left\{\begin{array}{cc}-\int_0^a f(x) d x+\int_0^a f(x) d x, & \text { if } \mathrm{f}(\mathrm{x}) \text { is odd } \\ \int_0^a f(x) d x+\int_0^a f(x) d x, & \text { if } \mathrm{f}(\mathrm{x}) \text { is even }\end{array}\right. \\ & =\left\{\begin{array}{cc}0, & \text { if } f \text { is an odd function } \\ 2 \int_0^a f(x) d x, & \text { if } f \text { is an even function }\end{array}\right.\end{aligned}$

Proof using Graph

The graph of the odd function is symmetric about the origin, as shown in the above figure

So, if $\int_0^{\mathrm{a}} \mathrm{f}(\mathrm{x}) \mathrm{dx}=\alpha$ then, $\int_{-\mathrm{a}}^0 \mathrm{f}(\mathrm{x}) \mathrm{dx}=-\alpha$

$
\therefore \quad \int_{-a}^a f(x) d x=0
$

The graph of the even function is symmetric about the y-axis, as shown in the above figure

$\begin{array}{ll}\text { So, } & \int_{-a}^0 \mathrm{f}(\mathrm{x}) \mathrm{dx}=\int_0^{\mathrm{a}} \mathrm{f}(\mathrm{x}) \mathrm{dx}=\alpha \\ \therefore & \int_{-a}^{\mathrm{a}} \mathrm{f}(\mathrm{x}) \mathrm{dx}=2 \alpha=2 \int_0^{\mathrm{a}} \mathrm{f}(\mathrm{x}) \mathrm{dx}\end{array}$

Corollary:

$\int_0^{2 a} f(x) d x=\left\{\begin{array}{cc}2 \int_0^a f(x) d x, & \text { if } f(2 a-x)=f(x) \\ 0, & \text { if } f(2 a-x)=-f(x\end{array}\right.$

Property 9

If f(x) is a periodic function with period T, then the area under f(x) for n periods would be n times the area under f(x) for one period, i.e.

$\int_0^{\mathrm{nT}} \mathrm{f}(\mathrm{x}) \mathrm{dx}=\mathrm{n} \int_0^{\mathrm{T}} \mathrm{f}(\mathrm{x}) \mathrm{dx}$

Proof:

Graphical Method

f(x) is a periodic function with period T. Consider the following graph of function f(x).

The graph of the function is the same in each of the interval (0, T), (T, 2T), (2T, 3T) ……..

So,

$\begin{aligned} \int_0^{\mathrm{nT}} \mathrm{f}(\mathrm{x}) \mathrm{d} & =\text { total shaded area } \\ & =\mathrm{n} \times(\text { area in the interval }(0, \mathrm{~T})) \\ & =\mathrm{n} \int_0^{\mathrm{T}} \mathrm{f}(\mathrm{x}) \mathrm{dx}\end{aligned}$

Property 10

$\int_a^{a+n T} f(x) d x=\int_0^{a T} f(x) d x=n \int_0^T f(x) d x$

Proof:

$\begin{aligned} & \text { Let, } \begin{aligned} & \mathrm{I}=\int_{\mathrm{a}}^{\mathrm{a}+\mathrm{nT}} \mathrm{f}(\mathrm{x}) \mathrm{dx} \\ &=\int_{\mathrm{a}}^0 \mathrm{f}(\mathrm{x}) \mathrm{dx}+\int_0^{\mathrm{nT}} \mathrm{f}(\mathrm{x}) \mathrm{dx}+\underbrace{\int_{n T}^{a+n T} f(x) d x}_{x=y+n T} \\ & \Rightarrow \mathrm{dx}=\mathrm{dy} \text { and when } \mathrm{x}=\mathrm{nT} \text { then } \mathrm{y}=0 \text { and } \mathrm{x}=\mathrm{a}+\mathrm{nT}, \mathrm{y}=\mathrm{a} \\ &=\int_{\mathrm{a}}^0 \mathrm{f}(\mathrm{x}) \mathrm{dx}+\int_0^{\mathrm{nT}} \mathrm{f}(\mathrm{x}) \mathrm{dx}+\int_0^{\mathrm{a}} \mathrm{f}(\mathrm{y}) \mathrm{dy} \\ &=\mathrm{n} \int_0^{\mathrm{nT}} \mathrm{f}(\mathrm{x}) \mathrm{dx} \\ & {\left[\because \int_0^{\mathrm{a}} \mathrm{f}(\mathrm{y}) \mathrm{dy}=\int_0^{\mathrm{a}} \mathrm{f}(\mathrm{x}) \mathrm{dx} \text { and } \int_0^{\mathrm{a}} \mathrm{f}(\mathrm{x}) \mathrm{dx}=-\int_{\mathrm{a}}^0 \mathrm{f}(\mathrm{x}) \mathrm{dx}\right] }\end{aligned}\end{aligned}$

Property 11

$
\int_{\mathrm{a}+\mathrm{nT}}^{\mathrm{b}+\mathrm{nT}} \mathrm{f}(\mathrm{x}) \mathrm{dx}=\int_{\mathrm{a}}^{\mathrm{b}} \mathrm{f}(\mathrm{x}) \mathrm{dx}
$

Property 12

$
\int_{\mathrm{mT}}^{\mathrm{nT}} f(x) d x=(n-m) \int_0^{\mathrm{T}} f(x) d x
$

Where ‘T’ is the period and m and n are Integers

Recommended Video Based on Application of Even-Odd Properties in Definite Integration

Solved Example Based on Application of Even-Odd Properties in Definite Integration

Example 1: $\int_{-3 \pi / 2}^{-\pi / 2}\left[(x+\pi)^3+\cos ^2(x+3 \pi)\right] d x$ is equal to

1) $\frac{\pi^4}{32}$
2) $\frac{\pi^4}{32}+\frac{\pi}{2}$
3) $\frac{1}{2}$
4) $\frac{\pi}{2}-1$

Solution

As learnt in concept

Properties of definite integration -

If $f(x)$ is an EVEN function of x: then integral of the function from - a to a is the same as twice the integral of the same function from o to a.

$\int_{-a}^a f(x) d x=2\left\{\int_o^a f(x) d x\right\}$

wherein

Check even function $f(-x)=f(x)$ and symmetrical about y axis.

$
I=\int_{\frac{-3 \pi}{2}}^{-\frac{\pi}{2}}\left[(x+\pi)^3+\cos ^2(x+3 \pi)\right] d x
$

Put $x+\pi=t$

$
\begin{aligned}
& I=\int_{-\frac{\pi}{2}}^{\frac{\pi}{2}}\left[t^3+\cos ^2 t\right] d t \\
& I=2 \int_0^{\frac{\pi}{2}} \cos ^2 t d \\
& =\int_0^{\frac{\pi}{2}}[1+\operatorname{Cos} 2 t] d t \\
& {[t]_0^{\frac{\pi}{2}}+\left[\frac{\operatorname{Sin} 2 t}{2}\right]_0^{\frac{\pi}{2}}} \\
& \frac{\pi}{2}+0
\end{aligned}
$

Example 2: $\int_{-\pi}^\pi \frac{2 x(1+\sin x)}{1+\cos ^2 x} d x$ is

1) $\pi^2 / 4$
2) $\pi^2$
3) 0
4) $\pi / 2$

Solution

As we learnt in

Properties of definite integration -

If $f(x)$ is an EVEN function of x: then integral of the function from - a to a is the same as twice the integral of the same function from o to a.

$\int_{-a}^a f(x) d x=2\left\{\int_o^a f(x) d x\right\}$

- wherein

Check even function $f(-x)=f(x)$ and symmetrical about y axis.

And,

Properties of Definite Integration -

If $f(x)$ is an odd function of then integral of the function from -a to a is ZERO

$\int_{-a}^a f(x) d x=0$
- wherein

Check

Odd function $f(-x)=-f(x)$

And,

Properties of Definite Integration -

$\int_0^{2 a} f(x) d x=\int_0^a[f(x)+f(-x)] d x$

$=\left\{\begin{array}{cc}2 \int_0^a f(x) d x & \text { if } f(2 a-x)=f(x) \\ 0 & \text { if } f(2 a-x)=-f(x)\end{array}\right.$

- $I=\int_{-\pi}^\pi \frac{2 x(1+\sin x) d x}{1+\cos ^2 x}$

$I=\int_0^\pi\left(\frac{2 x(1+\sin x)-2 x+2 x \sin x}{\left(1+\cos ^2 x\right)}\right) d x$

$\begin{aligned} & I=\int_0^\pi \frac{4 x \sin x}{\left(1+\cos ^2 x\right)} d x \\ & I=\int_0^{\frac{\pi}{2}} \frac{4 \sin x}{1+\cos ^2 x}(x+\pi-x) d x \\ & I=4 \pi \int_0^{\frac{\pi}{2}} \frac{\sin x d x}{1+\cos ^2 x} \\ & I=4 \pi\left[\tan ^{-1}(\cos x)\right]^\pi \\ & I=4 \pi\left[0+\frac{\pi}{4}\right]=\pi^2\end{aligned}$

Example 3: The value of the integral $\int_{\frac{-\pi}{2}}^{\frac{\pi}{2}} \sin ^4 x\left[1+\log \left[\frac{2+\sin x}{2-\sin x}\right]\right] d x$ is :

1) 0
2) $\frac{3}{4}$
3) $\frac{3}{8} \pi$
4) $\frac{3}{16} \pi$

Solution

As we learned

Properties of definite integration -

If $f(x)$ is an EVEN function of x: then integral of the function from - a to a is the same as twice the integral of the same function from o to a.

$\int_{-a}^a f(x) d x=2\left\{\int_0^a f(x) d x\right\}$

- wherein

Check even function $f(-x)=f(x)$ and symmetrical about y axis.

Check

Odd function $f(-x)=-f(x)$

$\int_{-\pi}^{\frac{1}{2}} \sin ^4 x\left(1+\log \left(\frac{2+\sin x}{2-\sin x}\right)\right) d x$

$=2 \int_0^{\frac{\pi}{2}} \sin ^4 x d x+2 \int_0^{\frac{x}{2}} \sin ^4 x \log \frac{2+\sin x}{2-\sin x} d x$

Since value of $\int_0^{\frac{5}{2}} \sin ^4 x \log \frac{2+\sin x}{2-\sin x} d x=0$ (Odd function)

So,

$\begin{aligned} & \sin ^4 x=\frac{1}{4}(1-\cos 2 x)^2 \\ & \sin ^4 x=\frac{1}{4}\left(1+\cos ^2 2 x-2 \cos 2 x\right) \\ & \sin ^4 x=\frac{1}{4}\left(1+\frac{1+\cos 4 x}{2}-2 \cos 2 x\right)\end{aligned}$

$\begin{aligned} & I=2 \cdot \int_0^{\frac{\pi}{2}} \sin ^4(x) d x \\ & I=2 \cdot \int_0^{\frac{\pi}{2}} \frac{1}{4}\left(\frac{3+\cos (4 x)-8 \cos (2 x)}{2}\right) d x \\ & =\frac{3 \pi}{8}\end{aligned}$

Example 4: $\int_{-\Pi / 2}^{\Pi / 2} \frac{\cos x}{1+e^x} d x$ is equal to?

1) $1$

2) $2 $

3) $-1$

4) $-2$

Solution

As we learnt

Properties of definite integration -

If $f(x)$ is an EVEN function of x: then integral of the function from - a to a is the same as twice the integral of the same function from o to a.

$\int_{-a}^a f(x) d x=2\left\{\int_0^a f(x) d x\right\}$

- wherein

Check even function $f(-x)=f(x)$ and symmetrical about y axis.

$\begin{aligned} & I=\int_{-\pi / 2}^{\pi / 2} \frac{\cos x}{1+e^x} d x=\int_0^{\pi / 2}\left(\frac{\cos x}{1+e^x}+\frac{\cos (-x)}{1+e^x}\right) d x=\int_0^{\pi / 2} \cos x d x= \\ & {[\sin x]_0^{\pi / 2}=1}\end{aligned}$

Example 5: $\int_{-1}^1 \frac{\log \left(x+\sqrt{1+x^2}\right)}{x+\log \left(x+\sqrt{1+x^2}\right)}(f(x)-f(-x)) d x$ is euqal to?

1) $0$

2) $2 \int_0^1 \frac{\log \left(x+\sqrt{1+x^2}\right)}{x+\log \left(x+\sqrt{1+x^2}\right)}(f(x)-f(-x)) d x$

3) $2 f(x)$

4) none of these

Solution

As we learnt

Properties of definite integration -

If $f(x)$ is an EVEN function of x: then integral of the function from - a to a is the same as twice the integral of the same function from o to a.

$\int_{-a}^a f(x) d x=2\left\{\int_o^a f(x) d x\right\}$

- wherein

Check even function $f(-x)=f(x)$ and symmetrical about y axis.

$\operatorname{Let} \phi(x)=\frac{\log \left(x+\sqrt{1+x^2}\right)}{x+\log \left(x+\sqrt{1+x^2}\right)} ; g(x)=(f(x)-f(-x))$

f(x) is an even function $\int_{-1}^1(\phi(x) \cdot g(x)) d x=0$

g(x) is an odd function.

Summary

Definite integration is a powerful tool in calculus that allows us to calculate the area under a curve between two specific points. Even-odd integration is useful when the function is symmetric around the x-axis or y-axis, It provides a deeper understanding of mathematical ideas paramount for later developments in many scientific and engineering disciplines.