Application of Inequality in Definite Integration

Definite Integration is one of the important parts of Calculus, which applies to measuring the change in the function at a certain point. Mathematically, it forms a powerful tool by which slopes of functions are determined, the maximum and minimum of functions found, and problems on motion, growth, and decay, to name a few. These concepts of integration have been broadly applied in branches of mathematics, physics, engineering, economics, and biology.

JEE Main 2025: Sample Papers | Mock Tests | PYQs | Study Plan 100 Days

JEE Main 2025: Maths Formulas  | Study Materials

JEE Main 2025: Syllabus | Preparation Guide | High Scoring Topics 

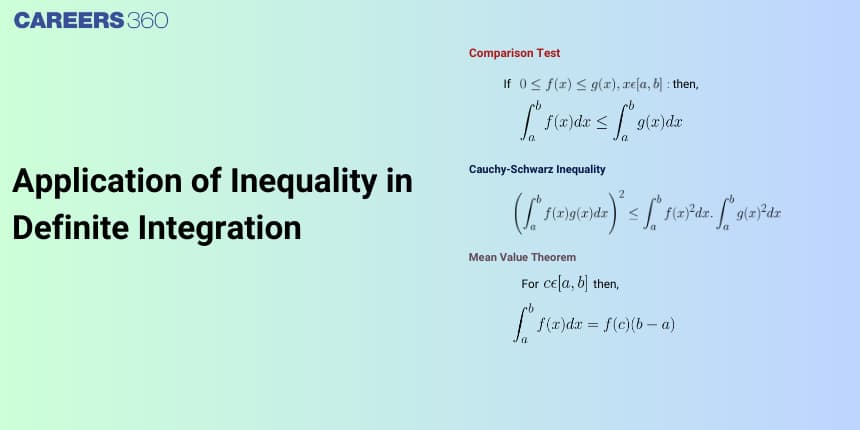
In this article, we will cover the concept Applicatons of Definite Integration. This concept falls under the broader category of Calculus, which is a crucial Chapter in class 12 Mathematics. It is not only essential for board exams but also for competitive exams like the Joint Entrance Examination (JEE Main), and other entrance exams such as SRMJEE, BITSAT, WBJEE, BCECE, and more. Over the last ten years of the JEE Main exam (from 2013 to 2023), thirty questions have been asked on this concept, including one in 2016, one in 2018, five in 2019, two in 2021, five in 2022, and sixteen in 2023.

Definite Integration

Definite integration is used to calculate the value of function over a specific interval.

Let f be a function of x defined on the closed interval [a, b] and F be another function such that $\frac{d}{d x}(F(x))=f(x)$ for all $x$ in the domain of $f$, then

$\int_a^b f(x) d x=[F(x)+c]_a^b=F(b)-F(a)$ is called the definite integral of the function $f(x)$ over the interval $[a, b]$, where $a$ is called the lower limit of the integral and $b$ is called the upper limit of the integral.

Applications of inequalities in Definite Integration

Inequalities gives relationship between two expressions that are not equal to one another. Symbols denoting the inequalities are <, >, ≤, ≥, and ≠.

Inequalities are used in definite integration to show compare to functions.

Results with inequalities in definite integration

1. If $m \leq f(x) \leq M$ for all $x$ in the interval $[a, b]$, then:

$
m(b-a) \leq \int_a^b f(x) d x \leq M(b-a)
$

2. Mean Value Theorem

If $f(x)$ is continuous on $[a, b]$, there exists a $c \in[a, b]$ such that:

$
f(c)=\frac{1}{b-a} \int_a^b f(x) d x
$

3. If $f(x) \geq g(x)$ for all $x \in[a, b]$, then:

$
\int_a^b f(x) d x \geq \int_a^b g(x) d x
$

This result is used to compare two functions.

4. For convex functions $\phi(x)$ and a probability distribution $p(x)$, Jensen's inequality states that:

$
\phi\left(\int_a^b f(x) d x\right) \leq \int_a^b \phi(f(x)) d x
$

5. Minkowski's Inequality

For $f(x)$ and $g(x)$ both in $L^p$ space:

$
\left(\int_a^b|f(x)+g(x)|^p d x\right)^{1 / p} \leq\left(\int_a^b|f(x)|^p d x\right)^{1 / p}+\left(\int_a^b|g(x)|^p d x\right)^{1 / p}
$

Recommended Video Based on Applications of Inequalities in Definite Integration

Solved Examples based on Applications of inequalities in Definite Integration

Example 1: Which of the following is a perfect way to write an integral?
$
\begin{aligned}
& \text { 1) } \int_1^3 f(x) \\
& \int_5^8 f(x) d y
\end{aligned}
$

3) $\int_7^9 f(x) d x$

$
{ }_4 \int_7^5 f(x) d x
$

Solution

As we have learnt,

Definite integration -

When $f(x)$ is integrated in a continuous limit, $(a, b)$: a, and b are known as the limit of integration.

wherein

Where a < x < b

In (A), dx is not written.

In (B), the variables are not the same.

In (D), the lower variable is greater than the upper variable.

Hence, the answer is the option 3.

Example 2: Which of the following is NOT a definite integral?
$
\int_{-5}^5 f(x) d x
$

2) $\int g(y) d y$

$
\int_3 \int_0^5 d u
$

4) $\int_5^6 0 d y$

Solution

As we have learned,

Definite integration -

When $f(x)$ is integrated in a continuous limit, $(a, b)$: a, and b are known as the limit of integration.

wherein

Where$a<x<b$

The upper and lower limits are not specified.

Hence, the answer is the option 2.

Example 3: Which of the following is NOT true?

(1) $\int_a^b f(x) d x=F(b)-F(a)$
$\int_0^0 f(x) d x=-F(a$
$\int_0^a f(x) d x=F(a)-F(b)$
4) $\int_a^a f(x) d x=0$

Solution

As we have learned,

lower and upper limit -

$\int_a^b f(x) d x=(F(x))_a^b$

$=F(b)-F(a)$

wherein

Where a is lower and b is the upper limit.

$\int_0^a f(x) d x=F(0)-F(a)$

and F(0) may or may not be equal to 0

Hence, the answer is the option (2).

Example 4: If $\int_a^b f(x) d x=F(b)-F(a)$; then which of the following is NOT true?

1) "a" s the lower limit

2) "b" is the upper limit

3) $\int_a^b f(x) d x=\int_b^a f(x) d x$

4) If $F(b)=F(a) ; \int_a^b f(x) d x=0$

Solution

As we have learnt,

lower and upper limit -

$\begin{array}{r}\int_a^b f(x) d x=(F(x))_a^b \\ =F(b)-F(a)\end{array}$

wherein

Where a is the lower and b is the upper limit.

$\int_a^b f(x) d x \neq \int_h^a f(x) d x$

Hence, the answer is the option 3.

Summary

Definite integration is a powerful tool in calculus that allows us to calculate the area under a curve between two specific points. Inequalites in defintie integration is used to compare two integrals which are not equal. It provides a deeper understanding of mathematical ideas paramount for later developments in many scientific and engineering disciplines.