Approximations and Errors using Derivatives: Definition and Examples

Approximation is an important concept in calculus. It is used to estimate the values of functions at a given point. An approximate model is used to make the calculations easier. The approximations can also be used if inadequate information prevents the use of exact demonstrations. These concepts of approximation have been broadly applied in branches of mathematics, physics, engineering, economics, and biology.

JEE Main 2025: Sample Papers | Mock Tests | PYQs | Study Plan 100 Days

JEE Main 2025: Maths Formulas  | Study Materials

JEE Main 2025: Syllabus | Preparation Guide | High Scoring Topics 

This Story also Contains

1. Approximation
2. Approximations and Errors Using Derivatives
3. ERROR

In this article, we will cover the concept of the approximation. This topic falls under the broader category of Calculus, which is a crucial chapter in Class 11 Mathematics. This is very important not only for board exams but also for competitive exams, which even include the Joint Entrance Examination Main and other entrance exams: SRM Joint Engineering Entrance, BITSAT, WBJEE, and BCECE. A total of one question has been asked on this topic in JEE Main from 2013 to 2023 in 2013.

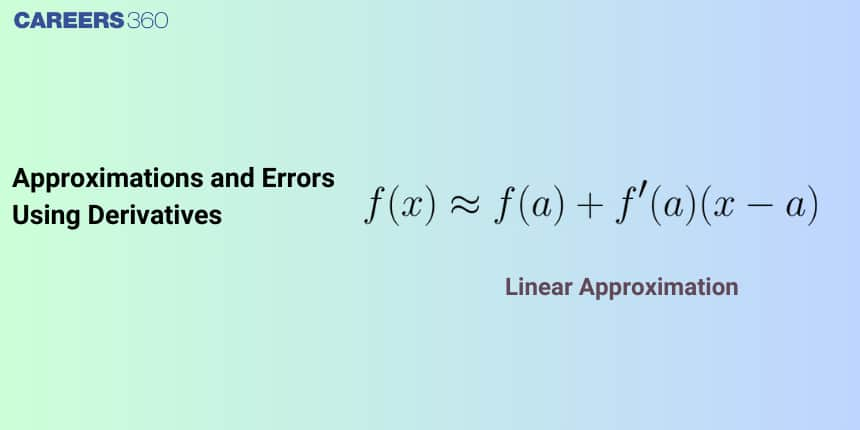
Approximation

Where $fx$ is the negative value of $f(x)$. It may be positive or negative.
It gives the approximate value of any $\mathrm{f}(\mathrm{x})$ at $x=x_{\circ }$. We break $x_{\circ }$ to $x+\delta x$ Such that $(x+\delta x)=f(x)+f^{\prime }(x)\cdot \delta x$
ex $:\sqrt{25.5}$ we take $f(x)=\sqrt{x},x=25$ and $\delta x=0.5$
we will use differentials to approximate values of certain quantities.
Let $f:D\to R,D\subset R$, be a given function, and let $y=f(x)$. Let $\mathrm{\Delta }x$ denote a small increment in x . Recall that the increment in y corresponding to the increment in $x$, denoted by $\mathrm{\Delta }y$, is given by $\mathrm{\Delta }y=f(x$ $+\mathrm{\Delta }x)-f(x)$. We define the following
(i) The differential of x , denoted by dx , is defined by $\mathrm{dx}=\mathrm{\Delta }\mathrm{x}$.
(ii) The differential of $y$, denoted by $dy$, is defined by $dy=f^{\prime }(x)dx$ or

$dy=\left(\frac{dy}{dx}\right)\mathrm{\Delta }x$

Approximations and Errors Using Derivatives

Let the function, $y=f(x)$, be a function of $x$
As we have derived derivatives earlier,

$\frac{dy}{dx}=\underset{\mathrm{\Delta }x\to 0}{lim}\frac{\mathrm{\Delta }y}{\mathrm{\Delta }x}=\underset{\mathrm{\Delta }x\to 0}{lim}\frac{f(x+\mathrm{\Delta }x)-f(x)}{\mathrm{\Delta }x}$

$\mathrm{\Delta }x$ is a small change in $x$ and the corresponding change in $y$ is $\mathrm{\Delta }y$
As in the figure, point $Q$ moves closer to point $P$ on the curve, then dy is a good approximation of $\mathrm{\Delta }y$.

$\begin{array}{rl}& \frac{dy}{dx}=\frac{\mathrm{\Delta }y}{\mathrm{\Delta }x}=\frac{f(x+\mathrm{\Delta }x)-f(x)}{\mathrm{\Delta }x}\\ \therefore & \mathbf{f}(\mathbf{x}+\mathrm{\Delta }\mathbf{x})=\mathbf{f}(\mathbf{x})+\mathrm{\Delta }\mathbf{x}\cdot \frac{\mathbf{d}\mathbf{y}}{\mathbf{d}\mathbf{x}}\end{array}$

ERROR

Absolute Error

$\mathrm{\Delta }\mathrm{x}$ or $dx$ is called absolute error in $x$.

Relative Error

$\frac{\mathrm{\Delta }\mathrm{x}}{\mathrm{x}}$ or $\frac{dx}{\mathrm{x}}$ is called the relative error in $x$

Percentage Error

$\frac{\mathrm{\Delta }\mathrm{x}}{\mathrm{x}}\cdot 100$ or $\frac{dx}{\mathrm{x}}\cdot 100$ is called the percentage error in $x$.

Recommended Video Based on Condition of Approximation

Solved Examples Based on Condition of Approximation:

Example 1: Let $f(1)=-2$ and $f^{\prime }(x)⩾4.2$ for $1\le x\le 6$ The possible value of $f(6)$ lies in the interval:
1) $[1,2\}$
2) $[13,\mathrm{\infty })$
3) $[14,\mathrm{\infty })$
4) $[19,\mathrm{\infty })$

Solution:

Given $f(1)=-2$ and $f^{\prime }(x)⩾4.2$ for $1\le x\le 6$
Consider $f^{\prime }(x)=\frac{f(x+h)-f(x)}{h}$

$\Rightarrow f(x+h)-f(x)=f^{\prime }(x)\cdot h\ge (4.2)h$
So, $f(x+h)\ge f(x)+(4.2)h$
put $x=1$ and $h=5$,
we get

$\begin{array}{rl}& f(6)\ge f(1)+5(4.2)\\ & \Rightarrow f(6)\ge 19\end{array}$
Hence $f(6)$ lies in $[19,\mathrm{\infty })$
Hence, the answer is option 4.

Example 2: Approximate value of $\sqrt{400.1}$ will be
1) 20.0025
2) 20.01
3) 20.001
4) 20.0035

Solution

Let $y=f(x)=\sqrt{x}$
With a small change $\mathrm{\Delta }x$ in x , there will be a small change in y i.e., $\mathrm{\Delta }y$

Now,

$\begin{array}{rl}& y=\sqrt{x}\Rightarrow \frac{dy}{dx}=\frac{1}{2\sqrt{x}}\Rightarrow dy=\frac{dx}{2\sqrt{x}}\\ & \Rightarrow \mathrm{\Delta }y=\frac{\mathrm{\Delta }x}{2\sqrt{x}}...(i)\end{array}$
For a given question, let $\mathrm{x}=400,x+\mathrm{\Delta }x=400.1\Rightarrow \mathrm{\Delta }x=0.1$
Also $\mathrm{y}=\sqrt{400}=20$

$\begin{array}{rl}& \therefore \mathrm{\Delta }y=\frac{0.1}{2\sqrt{400}}=0.1/40=0.0025(Using(i))\\ & \therefore y+\mathrm{\Delta }y=20+0.0025=20.0025\end{array}$

Hence, the answer is the option 1.

Example 3: Approximate value of $(999{)}^{\frac{1}{3}}$ equals
1) 9.96
2) 9.99
3) 10
4) 9.997

Solution

Let $\mathrm{y}=\mathrm{f}(\mathrm{x})=x^{1/3}$
With a small change $\mathrm{\Delta }x$ in x , there will be a small change $\mathrm{\Delta }y$ in y
Now,

$\begin{array}{rl}& y=x^{1/3}\Rightarrow \frac{dy}{dx}=\frac{1}{3x^{2/3}}\\ & \frac{dy}{dx}=\frac{1}{3x^{2/3}}\Rightarrow \mathrm{\Delta }y=\frac{\mathrm{\Delta }x}{3x^{2/3}}\end{array}$
For the given question, $\mathrm{x}=1000,x+\mathrm{\Delta }x=999\Rightarrow \mathrm{\Delta }x=-1$
Also $y=(1000{)}^{\frac{1}{3}}=10$
Now using (i)

$\begin{array}{rl}& \therefore \mathrm{\Delta }y=\frac{-1}{3\ast (1000{)}^{\frac{2}{3}}}=-0.00333\\ & \therefore y+\mathrm{\Delta }y=10-0.0033=9.99667\approx 9.997\end{array}$
Hence, the answer is the option 4.