Area of Parallelogram (Definition, Formulas & Examples)

What is the Area of a Parallelogram?

The area of a parallelogram is the measure of the region enclosed within its four sides. It represents the amount of two-dimensional space covered by the parallelogram and is expressed in square units such as $cm^2$, $m^2$, or $km^2$. Understanding the area of a parallelogram is an important part of geometry and mensuration and is frequently tested in school examinations, SSC, Banking, CUET, CAT, Railways, Defence, and other competitive exams.

Area of Parallelogram Meaning in Simple Words

In simple words, the area of a parallelogram tells us how much surface is covered inside the shape.

For example, if a parallelogram-shaped garden occupies a certain piece of land, its area tells us the size of the land covered by that garden.

Like rectangles and squares, the area of a parallelogram is measured in square units.

Definition of Area of a Parallelogram

The area of a parallelogram is defined as the product of its base and the corresponding perpendicular height.

Mathematically,

$A=b\times h$

where:

• $b$ = base of the parallelogram
• $h$ = perpendicular height

This formula gives the exact amount of space enclosed within the parallelogram.

Area of Parallelogram Formula

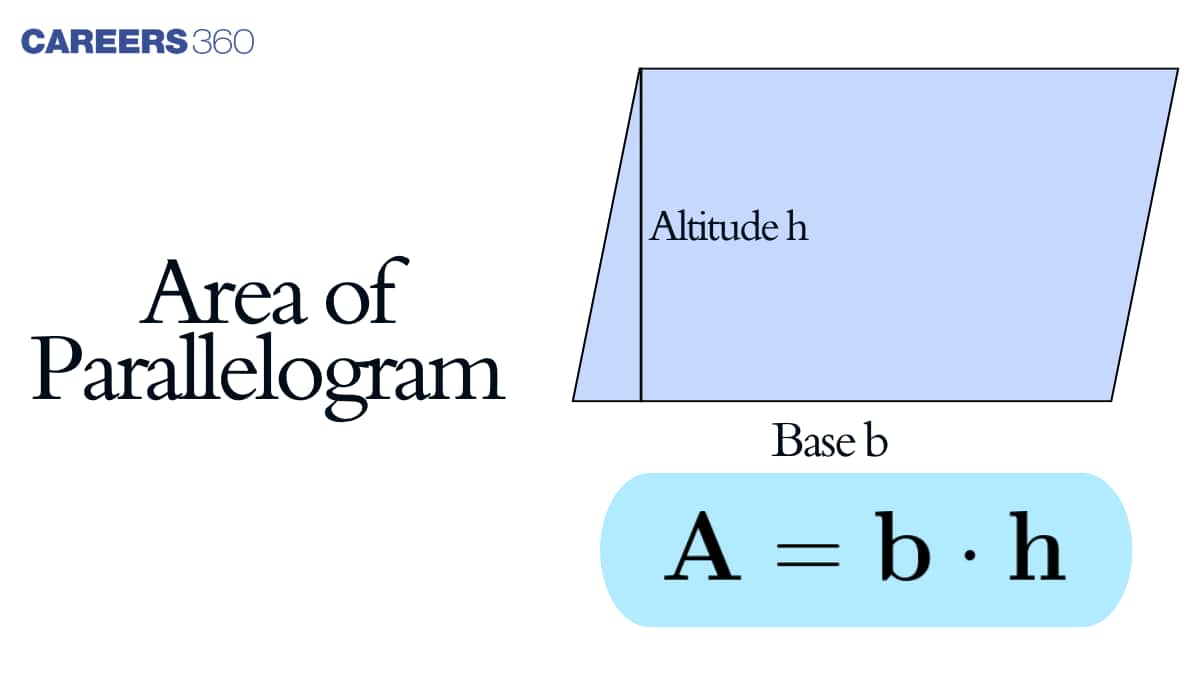
If we wish to find the area of parallelogram, we have to multiply the base of the perpendicular of parallelogram by its height. We must remember certain points that the base and the height of the parallelogram are always perpendicular to each other, which means they form an angle of 90 degrees wherever they meet or intersect, whereas the lateral side of the parallelogram is not perpendicular to the base, hence angle measuring 90 degrees is not formed at their meeting point. The formula for area of parallelogram is given as:

Area $=b \times h$ Square units

Where "b" = base and " $h$ " = height

How to find Area of Parallelogram?

Area of parallelogram can be calculated using its base and height. Apart from that, the area of parallelogram can also be calculated if its two diagonals are known along with any of their intersecting angles or if the length of the parallel sides is known, along with any of the angles between the sides. Following are the ways to find the area of parallelogram depending upon which sides are unknown to us and which are known:

• When the base and height of the parallelogram are given
• When height is not given
• When diagonals are given

Area of Parallelogram Using Sides

If the base and height of a parallelogram are known, its area can be calculated directly using the standard area formula. This is the most commonly used method in geometry and mensuration problems.

The area of a parallelogram is given by:

$A=b\times h$

where:

• $b$ = base of the parallelogram
• $h$ = perpendicular height

Example

Find the area of a parallelogram whose base is 10 cm and height is 5 cm.

Solution:

Given,

Base $=10\ \text{cm}$

Height $=5\ \text{cm}$

Using the formula:

Area of parallelogram $= b\times h$

$=10\times5$

$=50\ \text{cm}^2$

Hence, the area of the parallelogram is $50\ \text{cm}^2$.

Area of Parallelogram Without Height

Sometimes the perpendicular height of a parallelogram is not given. In such cases, the area can be calculated using trigonometry if the lengths of two adjacent sides and the included angle are known.

The formula is:

$A=ab\sin x$

where:

• $a$ = length of one side
• $b$ = length of the adjacent side
• $x$ = angle between the two sides

This formula is particularly useful in trigonometry and coordinate geometry problems.

Area of Parallelogram Using Diagonals

The area of a parallelogram can also be calculated using its diagonals and the angle between them.

Suppose:

• $d_1$ = first diagonal
• $d_2$ = second diagonal
• $y$ = angle between the diagonals

Then,

$A=\frac{1}{2}d_1d_2\sin y$

This method is useful when the lengths of the diagonals are known instead of the base and height.

Area Formulas for a Parallelogram

Meaning of Variables

Area of Parallelogram Using Vectors

When the sides of a parallelogram are represented as vectors, the area can be determined using vector algebra.

Let:

• $\vec a$ and $\vec b$ be two adjacent sides of the parallelogram.

Then the area is equal to the magnitude of their cross product.

Formula Using Side Vectors

The area of the parallelogram is:

$A=|\vec a\times\vec b|$

where $|\vec a\times\vec b|$ denotes the magnitude of the cross product of vectors $\vec a$ and $\vec b$.

Area Using Diagonal Vectors

Let: $d_1=\vec a+\vec b$ and $d_2=\vec b-\vec a$

Then, $d_1\times d_2=(\vec a+\vec b)\times(\vec b-\vec a)$

Expanding,

$= \vec a\times\vec b-\vec a\times\vec a+\vec b\times\vec b-\vec b\times\vec a$

Since, $\vec a\times\vec a=0$ and $\vec b\times\vec b=0$

we get, $=\vec a\times\vec b-\vec b\times\vec a$

Using the property: $\vec a\times\vec b=-\vec b\times\vec a$

Therefore, $d_1\times d_2=2(\vec a\times\vec b)$

Thus, $\vec a\times\vec b=\frac{1}{2}(d_1\times d_2)$

Hence, the area of the parallelogram in terms of diagonals is:

$A=\frac{1}{2}|d_1\times d_2|$

Perimeter and Area of a Parallelogram

The area and perimeter of a parallelogram are related through its side lengths.

Area Formula

The area of a parallelogram is:

$A=bh$

where:

• $b$ = base
• $h$ = height

Perimeter Formula

The perimeter of a parallelogram is:

$P=2(a+b)$

where:

• $a$ and $b$ are adjacent sides

Expressing Area in Terms of Perimeter

From the perimeter formula:

$P=2(a+b)$

Dividing both sides by 2:

$\frac{P}{2}=a+b$

Therefore,

$b=\frac{P}{2}-a$

Substituting this value into the area formula:

$A=bh$

$A=\left(\frac{P}{2}-a\right)h$

This gives the area of a parallelogram in terms of its perimeter, side length, and height.

Why the Area Formula is Important?

The area formula of a parallelogram is important because it helps calculate the size of various geometric and real-world objects.

Importance of Area of Parallelogram

• Used in geometry and mensuration.
• Helps compare sizes of geometric figures.
• Useful in architecture and construction.
• Used in land measurement and surveying.
• Forms the basis for area formulas of other quadrilaterals.
• Frequently appears in board exams and competitive examinations.
• Important for engineering and design calculations.

Real-Life Applications of Parallelograms

Parallelogram-shaped structures are commonly found in daily life and engineering designs.

Examples

• Slanted windows often form parallelograms.
• Bridge structures use parallelogram frameworks.
• Decorative floor patterns frequently contain parallelogram tiles.

Properties of a Parallelogram

A parallelogram has several unique geometric properties that distinguish it from other quadrilaterals.

Opposite Sides of a Parallelogram

In a parallelogram:

• Opposite sides are parallel.
• Opposite sides are equal in length.

If $ABCD$ is a parallelogram, then:

$AB=CD$ and $BC=AD$

Opposite Angles of a Parallelogram

The opposite angles of a parallelogram are equal.

For parallelogram $ABCD$:

$\angle A=\angle C$

$\angle B=\angle D$

Also, adjacent angles are supplementary.

$\angle A+\angle B=180^\circ$

Diagonals of a Parallelogram

The diagonals of a parallelogram bisect each other.

If diagonals $AC$ and $BD$ intersect at point $O$, then:

$AO=OC$

$BO=OD$

However, unlike a rectangle or square, the diagonals are generally not equal.

Height and Base of a Parallelogram

The area of a parallelogram depends on its base and perpendicular height.

Base

The side chosen as the reference side is called the base.

Height

The perpendicular distance between two parallel sides is called the height.

A common mistake is using the slant side instead of the perpendicular height.

Only the perpendicular height should be used in area calculations.

Area of Parallelogram Formula

Several formulas can be used to calculate the area of a parallelogram depending on the available information.

Standard Area Formula

The most commonly used formula is:

A=bh

where:

• $A$ = area
• $b$ = base
• $h$ = perpendicular height

Formula Using Base and Height

If the base and corresponding height are known, simply multiply them.

Example

Base $=12\ cm$

Height $=5\ cm$

Area $=12\times5=60\ cm^2$

Formula Using Trigonometry

If two sides and the included angle are known, the area can be calculated using trigonometry.

$A=ab\sin\theta$

where:

• $a$ and $b$ are adjacent sides
• $\theta$ is the included angle

Example

$a=8\ cm$

$b=6\ cm$

$\theta=30^\circ$

Area $=8\times6\times\sin30^\circ$

Area $=48\times\frac{1}{2}$

Area $=24\ cm^2$

Meaning of Base and Height in the Formula

The area depends on the perpendicular height and not on the slant side length.

Derivation of Area of Parallelogram

The area formula can be derived using basic geometric concepts.

Derivation Using Rectangle Concept

Consider a parallelogram with base $b$ and height $h$.

If a triangular portion from one side is cut and moved to the opposite side, the figure transforms into a rectangle.

The resulting rectangle has:

• Length $=b$
• Width $=h$

Area of rectangle:

$=b\times h$

Therefore,

Area of parallelogram $=b\times h$

Derivation Using Geometry

A parallelogram can be divided into triangles.

By rearranging these triangles, a rectangle with the same base and height is obtained.

Since area remains unchanged during rearrangement:

Area of parallelogram $=b\times h$

Visual Explanation of the Formula

The area depends entirely on:

• Base length
• Perpendicular height

If the height increases while the base remains fixed, the area increases.

If the height decreases, the area decreases.

How to Find the Area of a Parallelogram?

Finding the area is straightforward when the required dimensions are known.

Step-by-Step Method

Step 1

Identify the base.

Step 2

Find the corresponding perpendicular height.

Step 3

Apply the formula:

$A=b\times h$

Step 4

Substitute the values.

Step 5

Simplify and write the answer in square units.

Using Base and Height

Example

Base $=15\ cm$

Height $=8\ cm$

Area $=15\times8$

Area $=120\ cm^2$

Using Side Lengths and Angle

When height is not given but two sides and the included angle are known:

Use:

$A=ab\sin\theta$

Example

$a=10\ cm$

$b=8\ cm$

$\theta=60^\circ$

Area $=10\times8\times\sin60^\circ$

Area $=80\times\frac{\sqrt3}{2}$

Area $=40\sqrt3\ cm^2$

Example 1

Find the area of a parallelogram with base 14 cm and height 9 cm.

Area $=14\times9$

Area $=126\ cm^2$

Example 2

Find the area of a parallelogram with sides 12 cm and 7 cm and included angle $30^\circ$.

Area $=12\times7\times\sin30^\circ$

Area $=84\times\frac{1}{2}$

Area $=42\ cm^2$

Area of Special Parallelograms

Several common quadrilaterals are special forms of parallelograms.

Area of a Rectangle

A rectangle is a parallelogram with all angles equal to $90^\circ$.

Formula:

$A=l\times b$

Area of a Rhombus

A rhombus is a parallelogram with all sides equal.

Formula:

$A=\frac{1}{2}d_1d_2$

where $d_1$ and $d_2$ are diagonals.

Area of a Square

A square is a parallelogram with equal sides and right angles.

Formula:

$A=s^2$

where $s$ is the side length.

Relationship with Parallelogram Formula

All these shapes follow the same fundamental area concept.

Applications of Area of Parallelogram

The area of a parallelogram has numerous practical applications.

Applications in Geometry

Used for:

• Area calculations
• Geometric proofs
• Coordinate geometry problems

Applications in Architecture

Architects use parallelogram area formulas while designing:

• Roof structures
• Building facades
• Decorative patterns

Applications in Engineering

Engineers use area calculations in:

• Structural analysis
• Material estimation
• Mechanical design

Applications in Land Measurement

Surveyors often divide irregular plots into parallelogram-shaped sections to simplify area calculations.

Important Results and Identities

• Opposite sides are equal and parallel.
• Opposite angles are equal.
• Diagonals bisect each other.
• Area depends on base and perpendicular height.
• Parallelograms with the same base and height have equal areas.
• A rectangle and a parallelogram having the same base and height have the same area.

These concepts form the foundation of area of parallelogram formulas, mensuration, quadrilateral geometry, and competitive exam mathematics.

Best Books for Area of Parallelogram

The following books help build a strong understanding of parallelograms, geometry concepts, and mensuration formulas frequently used in examinations.

Shortcut Tips and Tricks for Area of Parallelogram

Understanding a few key relationships can help solve area of parallelogram questions quickly and accurately.

Important Formula Table

This formula sheet summarizes the most important formulas related to the area of a parallelogram and related figures.

Solved Examples based on Area of a Parallelogram

For more such examples refer Area of parallelogram worksheet.

List of Topics Related to Area of Parallelogram

Understanding the area of a parallelogram becomes easier when you study related geometry and mensuration topics. The following concepts help strengthen your understanding of area formulas, properties of quadrilaterals, and problem-solving techniques commonly asked in school and competitive examinations.