Bernoulli Trials and Binomial Distribution

Probability is a part of mathematics that deals with the likelihood of different outcomes. It plays an important role in estimating the outcome or predicting the chances of that event. Bernoulli's equation is a special type of first-order non-linear differential equation that plays an important role in various fields such as physics, engineering, and economics. Some major applications of the Bernoulli equation are used in fluid dynamics, biology, and economics.

JEE Main 2025: Sample Papers | Mock Tests | PYQs | Study Plan 100 Days

JEE Main 2025: Maths Formulas  | Study Materials

JEE Main 2025: Syllabus | Preparation Guide | High Scoring Topics 

Bernoulli Equation

An equation of the form

$
\frac{d y}{d x}+P(x) \cdot y=Q(x) \cdot y^n
$

Where $P(x)$ and $Q(x)$ are a function of $x$ only, is known as Bernoulli's equation.
(If we put $\mathrm{n}=0$, then the equation is in linear form.)
To reduce Eq (i) into linear form, divide both sides by $y^n$,

$
\begin{aligned}
& \quad \frac{1}{y^n} \cdot \frac{d y}{d x}+\frac{P(x)}{y^{n-1}}=Q(x) \\
& \text { or } \quad y^{-n} \cdot \frac{d y}{d x}+y^{1-n} \cdot P(x)=Q(x)
\end{aligned}
$

Putting $y^{1-n}=t$, converts this equation into a linear equation in $t$, which can then be solved

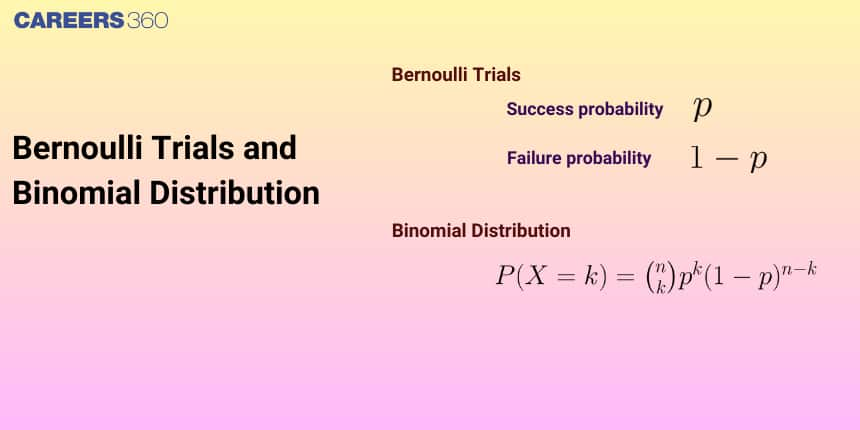
Bernoulli Trials

Trials of a random experiment are called Bernoulli trials, if they satisfy the following conditions

1. There are a fixed number of trials. Think of trials as repetitions of an experiment. The letter $n$ denotes the number of trials.

2. The $n$ trials are independent and are repeated using identical conditions.

3. There are only two possible outcomes, called "success" and "failure," for each trial. The letter p denotes the probability of a success on any one trial, and q denotes the probability of a failure on any one trial. $p + q = 1$

For example, randomly guessing at a true-false statistics question has only two outcomes. If a success is guessing correctly, then a failure is guessing incorrectly. Suppose Joe always guesses correctly on any statistics true-false question with a probability $p = 0.6.$ Then, $q = 0.4.$ This means that for every true-false statistics question Joe answers, his probability of success $(p = 0.6)$ and his probability of failure $(q = 0.4)$ remain the same. So guessing one question is considered a trial. If he guesses $n$ different questions, means the trial is repeated $n$ times and $p = 0.6$ remains the same for each trial.

Binomial Distribution

A binomial distribution with $n$-Bernoulli trials and probability of success in each trial as $p$, is denoted by $B(n, p)$. The probability

$
P(X=r)={ }^n C_r p^r \cdot q^{n-r}
$

Where $P(X=r)$ is the probability of $X$ successes in $n$ trials when the probability of success in ANY ONE TRIAL is $p$. And of course $q=(1-p)$ and is the probability of a failure in any one trial.

In the experiment, the probability of
- At least " $r$ " successes,

$
P(X \geq r)=\sum_{\lambda=r}^n{ }^n C_\lambda p^\lambda \cdot q^{n-\lambda}
$

- At most "r" successes,

$
\mathrm{P}(\mathrm{X} \leq \mathrm{r})=\sum_{\lambda=0}^{\mathrm{r}}{ }^{\mathrm{n}} \mathrm{C}_\lambda \mathrm{p}^\lambda \cdot \mathrm{q}^{\mathrm{n}-\lambda}
$

The mean, $\mu$, and variance, $\sigma^2$, for the binomial probability distribution are $\mu=\mathrm{np} \quad$ and $\quad \sigma^2=\mathrm{npq}$
The standard deviation, $\sigma$, is then $\sigma=\sqrt{n p q}$.

Solved Examples Based on Bernoulli Trials and Binomial Distribution

Example 1: The probability that a student is not a swimmer is $\frac{1}{5}$. The probability that out of 5 students exactly 4 are swimmer is
1) $\left(\frac{4}{5}\right)^3$
2) $\left(\frac{4}{5}\right)^4$
3) ${ }^5 \mathrm{C}_4\left(\frac{4}{5}\right)^4$
4) none of these

Solution
Binomial Theorem on Probability -
If an experiment is repeated n times under similar conditions we say that n trials of the experiment have been made.
Let $E$ be an event.
$P=$ the Probability of occurrence of event $E$ in one trial.
$q=1-p=$ probability of nonoccurrence of event $E$ in one trial such that $p+q=1$
$x=$ number of successes.

|Let $p=$ probability that a student selected at random is a swimmer $=1-\frac{1}{5}=\frac{4}{5}$

The probability that exactly 4 students are swimmers is

$
{ }^5 C_4 p^4 q=5\left(\frac{4}{5}\right)^4 \frac{1}{5}=\left(\frac{4}{5}\right)^4
$
Hence, the answer is the option (2).

Example 2: If the probability of hitting a target by a shooter, in any shot, is $\frac{1}{3}$, then the minimum number of independent shots at the target required by him so that the probability of hitting the target at least once is greater than $\frac{5}{6}$, is:
1) 6
2) 5
3) 4
4) 3

Solution
Binomial Theorem on Probability -
If an experiment is repeated n times under similar conditions we say that n trials of the experiment have been made.
Let E be an event.
$P=$ the Probability of occurrence of event $E$ in one trial.
$q=1-p=$ probability of nonoccurrence of event $E$ in one trial such that $p+q=1$
$\mathrm{x}=$ number of successes.
Binomial Theorem on Probability -
Then
$
\begin{aligned}
& P(X=r) \quad \text { or } P(r) \\
& ={ }^n C_{\Upsilon} \cdot P^r \cdot q^{n-r}
\end{aligned}
$
From the concept

$
\begin{aligned}
& 1-{ }_0^n \mathrm{C}\left(\frac{1}{3}\right)^0\left(\frac{2}{3}\right)^n>\frac{5}{6} \\
& \frac{1}{6}>\left(\frac{2}{3}\right)^n \\
& =>0.16>\left(\frac{2}{3}\right)^n \\
& n_{\min }=5
\end{aligned}
$
Hence, the answer is the option (2).

Example 3: One hundred identical coins each with probability $p$ of showing heads are tossed once. If $0<p<1$ and the probability of heads showing on 50 coins is equal to that of heads showing on 51 coins, the value of $p$ is
1) $\frac{1}{2}$
2) $\frac{51}{101}$
3) $\frac{49}{101}$
4) none of these

Solution

Binomial Theorem on Probability -

Then

$
\begin{aligned}
& P(X=r) \quad \text { or } P(r) \\
& ={ }^n C_{\Upsilon} \cdot P^r \cdot q^{n-r}
\end{aligned}
$
Let $X$ be the number of coins showing heads.
Then $X$ follows a binomial distribution with parameters $n=100$ and $p$.

$
\begin{aligned}
& \text { since } P(X=50)=P(X=51) \\
& \quad \Rightarrow \frac{100!}{50!50!} \cdot \frac{51!49!}{100!}=\frac{p}{1-p} \\
& \Rightarrow \frac{51}{50}=\frac{p}{1-p} \\
& \Rightarrow 51-51 p=50 p \\
& \Rightarrow p=\frac{51}{101}
\end{aligned}
$
Hence, the answer is the option (2).

Example 4: South African cricket captain lost the toss of a coin 13 times out of 14 . The chance of this happening was:
1) $\frac{7}{2^{13}}$
2) $\frac{1}{2^{13}}$
3) $\frac{13}{2^{14}}$
4) $\frac{13}{2^{13}}$

Solution
As we have learned in
Binomial Theorem on Probability -
Then

$
\begin{aligned}
& P(X=r) \text { or } P(r) \\
& ={ }^n C_{\Upsilon} \cdot P^r \cdot q^{n-r}
\end{aligned}
$

$
P={ }^{14} C_{13}\left(\frac{1}{2}\right)^{13}\left(\frac{1}{2}\right)^{14-13}=14 \times \frac{1}{2^{13}} \frac{1}{2}=\frac{7}{2^{13}}
$
Hence, the answer is the option (1).

Example 5: For the initial screening of an admission test, a candidate is given fifty problems to solve. If the probability that the candidate can solve any problem is $\frac{4}{5}$, then the probability that he is unable to solve less than two problems is:
1) $\frac{201}{5}\left(\frac{1}{5}\right)^{49}$
2) $\frac{316}{25}\left(\frac{4}{}\right)5^{48}$
3) $\frac{54}{5}\left(\frac{4}{5}\right)^{49}$
4) $\frac{164}{25}\left(\frac{1}{5}\right)^{48}$

Solution
Binomial Theorem on Probability
Then

$
\begin{aligned}
& P(X=r) \quad \text { or } P(r) \\
& ={ }^n C_{\Upsilon} \cdot P^r \cdot q^{n-r}
\end{aligned}
$

Probability of Solving the problems out of 50 problems $=\frac{4}{5}$

$
\Rightarrow P(\text { not solving })=\frac{1}{5}
$
The probability that he is unable to solve less than two problems is :
$P$ (zero correct ) $+P$ (one correct)

$
\left(\frac{1}{5}\right)^{50}+{ }^{50} \mathrm{C}_1\left(\frac{4}{5}\right)^{49}\left(\frac{1}{5}\right)^1
$

(using Binominal Probability)

$
\frac{54}{5}\left(\frac{4}{5}\right)^{49}
$
Hence, the answer is option (3).

Summary
Bernoulli Trials and Binomial Distribution provide tools to solve complex situations and analyze the likelihood of an event. These methods are widely used in real-life applications providing insights and solutions to problems. Mastery of these concepts can help in solving gaining deeper insights and contributing meaningfully to real-life problems.