DeMoivre's Theorem - Definition, Proof, Uses and Examples

Edited By Komal Miglani | Updated on Jul 02, 2025 08:06 PM IST

This theorem was developed by French mathematician Abraham de Moivre. It helps to connect complex numbers with trigonometry. It also helps in finding the powers and the roots of a complex number when they are expressed in Euler form. The main applications of De-moivre's theorem are to find powers of complex numbers, and roots of complex numbers, derive various trigonometric identities, and help in signal processing.

This Story also Contains

De-moivre's Theorem
De Moivre’s Theorem Proof
Solved Examples Based on De-Moivre's Theorem

DeMoivre's Theorem - Definition, Proof, Uses and Examples

In this article, we will cover the concept of the De-moivre's theorem. This concept falls under the broader category of complex numbers and quadratic equations, a crucial Chapter in class 11 Mathematics. It is not only essential for board exams but also for competitive exams like the Joint Entrance Examination (JEE Main), and other entrance exams such as SRMJEE, BITSAT, WBJEE, BCECE, and more.

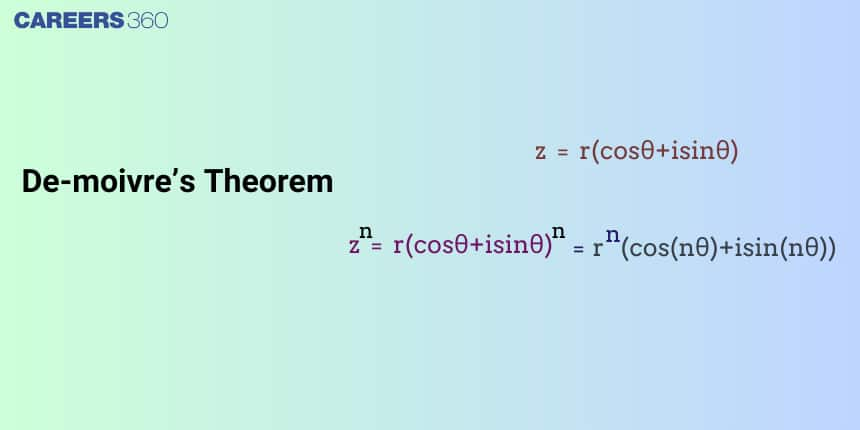
De-moivre's Theorem

One of the fundamental theorems of complex numbers, De Moivre's theorem is utilised to address a variety of complex number problems. The solution of trigonometric functions with numerous angles is another common use of this theorem. "De Moivre's Identity" and "De Moivre's Formula" are other names for DeMoivre's Theorem. The name of this theorem originates from the name of its creator, the renowned mathematician De Moivre.

De Moivre’s Formula for complex numbers is, for any real value of x,

$(\cos x+i \cdot \sin x)^n=\cos (n x)+i \cdot \sin (n x)$

De Moivre’s Theorem Proof

De-moivre’s theorem is based on the Euler form representation of complex numbers.

According to De-moivre’s theorem

1. If $\mathrm{n} \in \mathbb{I}$ (integers), $\theta \in \mathbb{R}$ and $i=\sqrt{-1}$, then
$
(\cos \theta+i \sin \theta)^{\mathbf{n}}=\cos \mathbf{n} \theta+i \sin \mathbf{n} \theta
$

Proof:
We know that, $\mathrm{e}^{i \theta}=\cos \theta+i \sin \theta$
now,
$
\begin{aligned}
& \Rightarrow\left(\mathrm{e}^{i \theta}\right)^{\mathrm{n}}=(\cos \theta+i \sin \theta)^{\mathrm{n}} \\
& \Rightarrow \mathrm{e}^{i(\mathrm{n} \theta)}=(\cos \theta+i \sin \theta)^{\mathrm{n}} \\
& \Rightarrow \cos \mathrm{n} \theta+i \sin \mathrm{n} \theta=(\cos \theta+i \sin \theta)^n
\end{aligned}
$

2. If $\theta_1, \theta_2, \theta_3 \ldots \ldots ., \theta_{\mathrm{n}} \in \mathbb{R}$, then
$
\begin{gathered}
\left(\cos \theta_1+i \sin \theta_1\right)\left(\cos \theta_2+i \sin \theta_2\right)\left(\cos \theta_3+i \sin \theta_3\right) \ldots \ldots \ldots \ldots \ldots\left(\cos \theta_{\mathrm{n}}+i \sin \theta_{\mathrm{n}}\right) \\
=\cos \left(\theta_1+\theta_2+\theta_3+\ldots \ldots .+\theta_{\mathrm{n}}\right)+i \sin \left(\theta_1+\theta_2+\theta_3+\ldots \ldots+\theta_{\mathrm{n}}\right)
\end{gathered}
$

Proof:

$\theta_1, \theta_2, \theta_3 \ldots \ldots \ldots . \theta_{\mathrm{n}} \in \mathbb{R}$ and $\mathrm{i}=\sqrt{-1}$, then
by Euler's formula $\mathrm{e}^{\mathrm{i} \theta}=\cos \theta+\mathrm{i} \sin \theta$

$
\begin{aligned}
& \left(\cos \theta_1+\mathrm{i} \sin \theta_1\right)\left(\cos \theta_2+\mathrm{i} \sin \theta_2\right)\left(\cos \theta_3+\mathrm{i} \sin \theta_3\right) \ldots \ldots \\
& \ldots \ldots \ldots\left(\cos \theta_{\mathrm{n}}+\mathrm{i} \sin \theta_{\mathrm{n}}\right)=\mathrm{e}^{\mathrm{i} \theta_1} \cdot \mathrm{e}^{\mathrm{i} \theta_2} \cdot \mathrm{e}^{\mathrm{i} \theta_3} \cdot \ldots \ldots \ldots . \cdot \mathrm{e}^{\mathrm{i} \theta_{\mathrm{n}}} \\
& \left.=\mathrm{e}^{\mathrm{i}\left(\theta_1+\theta_2+\theta_3+\ldots\right.}+\ldots+\theta_{\mathrm{n}}\right) \\
& =\cos \left(\theta_1+\theta_2+\theta_3+\ldots \ldots+\theta_{\mathrm{n}}\right)+\mathrm{i} \sin \left(\theta_1+\theta_2+\theta_3+\ldots \ldots . .+\theta_{\mathrm{n}}\right)
\end{aligned}
$

Note: If $n$ is a rational number that is not an integer and $n=p / q$, where $\operatorname{HCF}(p, q)=1$ and $q>0$, then $z^n$ will have $q$ values. One of the values will be $\cos n+i \sin n$.

1. $(\cos \theta-\mathrm{i} \sin \theta)^{\mathrm{n}}=\cos \mathrm{n} \theta-\mathrm{i} \sin \mathrm{n} \theta$

2. $(\sin \theta+\mathrm{i} \cos \theta)^{\mathrm{n}}=(\mathrm{i})^{\mathrm{n}}(\cos \mathrm{n} \theta-\mathrm{i} \sin \mathrm{n} \theta)$

(by taking i common first)

3. $(\sin \theta-\mathrm{i} \cos \theta)^{\mathrm{n}}=(-\mathrm{i})^{\mathrm{n}}(\cos \mathrm{n} \theta+\mathrm{i} \sin \mathrm{n} \theta)$

(by taking i common first)

4. $(\cos \theta+i \sin \phi)^n \neq \cos \mathrm{n} \theta+\mathrm{i} \sin \mathrm{n} \phi$

Solved Examples Based on De-Moivre's Theorem

Example 1: Let $a=\cos \alpha+i \sin \alpha, b=\cos \beta+i \sin \beta, c=\cos \gamma+i \sin \gamma$ and $\frac{a^2}{b^2}+\frac{b^2}{c^2}+\frac{c^2}{a^2}=1$ then $\cos (2 \alpha-2 \beta)+\cos (23-2 \gamma)+\cos (2 \gamma-2 a)$ equals

1) 0

2) 1

3) 2

4) 3

Solution

As we learned in

De Moivre's Theorem -

$(\cos \theta+i \sin \theta)^n=\cos n \theta+i \sin n \theta \forall n \in I$

Now,

$\begin{aligned} & a^2=\cos 2 \alpha+i \sin 2 \alpha=e^{i 2 a} \\ & b^2=\cos 2 \beta+i \sin 2 \beta=e^{i 2 \beta} \\ & c^2=\cos 2 \gamma+i \sin 2 \gamma=e^{i 2 \gamma}\end{aligned}$

Given
$
\begin{aligned}
& \frac{a^2}{b^2}+\frac{b^2}{c^2}+\frac{c^2}{a^2}=1 \\
& \Rightarrow \frac{e^{12 \gamma}}{e^{128}}+\frac{e^{128}}{e^{12 \gamma}}+\frac{e^{12 \gamma}}{e^{i 2 a}}=1 \\
& \Rightarrow e^{i 2(a-\beta)}+e^{12(3-\gamma)}+e^{12(7-a)}=1
\end{aligned}
$
$
\begin{aligned}
& \Rightarrow \cos (2 \alpha-2 \beta)+i \sin (2 \alpha-2 \beta)+\cos (2 \beta-2 \gamma)+i \sin (2 \beta-2 \gamma)+ \\
& \cos (2 \gamma-2 \alpha)+i \sin (2 \gamma-2 \alpha)=1
\end{aligned}
$

Comparing real and imaginary parts

$\begin{aligned} & \Rightarrow \cos (2 \alpha-2 \beta)+\cos (2 \beta-2 \gamma)+\cos (2 \gamma-2 \alpha)=1 \text { and } \sin (2 \alpha-2 \beta)+ \\ & \sin (2 \beta-2 \gamma)+\sin (2 \gamma-2 \alpha)=0\end{aligned}$

Hence, the answer is the option 2.

Example 2: Let p. $\mathrm{q} \in \mathbb{R}$ and $(1-\sqrt{3} i)^{200}=2^{199}(p+i q), i=\sqrt{-1}$
Then $p+q+q^2$ and $p-q+q^2$ are roots of the equation

1) $x^2-4 x-1=0$

2) $x^2-4 x+1=0$

3) $x^2+4 x-1=0$

4) $x^2+4 x+1=0$

Solution

$\begin{aligned} & (1-\sqrt{3} i)^{200}=2^{199}(p+i q) \\ & \Rightarrow 2^{200} \operatorname{cis}\left(\frac{-\pi}{3}\right)^{200}=2^{199}(p+i q) \\ & \Rightarrow 2^{200}\left(\operatorname{cis}\left(-\frac{200 \pi}{3}\right)\right)=2^{199}(p+i q)\end{aligned}$

$\begin{aligned} & \Rightarrow 2\left(\operatorname{cis}\left(-66 \pi-\frac{2 \pi}{3}\right)\right)=(p+i q) \\ & \Rightarrow 2\left[\operatorname{cis}\left(\frac{-2 \pi}{3}\right)\right]=(p+i q) \\ & \Rightarrow 2\left[\frac{-1}{2}-\frac{\sqrt{3} i}{2}\right]=(p+i q) \\ & \Rightarrow p=-1, q=-\sqrt{3}\end{aligned}$
$
\begin{aligned}
& \text { Now } \\
& \alpha=p+q+q^2=2-\sqrt{3} \\
& \beta=p-q+q^2=2+\sqrt{3}
\end{aligned}
$

req. quad is $x^2-4 x+1=0$

Hence, the answer is the option 2.

Example 3: The value of $\left(\frac{1+\sin \frac{2 \pi}{9}+i \cos \frac{2 \pi}{9}}{1+\sin \frac{2 \pi}{9} i \cos \frac{2 \pi}{9}}\right)^3$ is

1)$-\frac{1}{2}(\sqrt{3}-i)$

2) $-\frac{1}{2}(1-i \sqrt{3})$

3) $\frac{1}{2}(1-i \sqrt{3})$

4) $\frac{1}{2}(\sqrt{3}+i)$

Solution

$\begin{aligned} & \frac{\pi}{2}-\frac{2 \pi}{9} \\ & =\frac{95-4 \pi}{18}=\frac{5 \pi}{18} \\ & \Rightarrow \frac{1+\cos \frac{5 \pi}{18}+i \sin \frac{5 \pi}{18}}{1+\cos \frac{5 \pi}{18}-i \sin \frac{5 \pi}{18}}\end{aligned}$

$\begin{aligned} & =\frac{2 \cos ^2 \frac{5 \pi}{36}+2 i \sin \frac{5 \pi}{36} \cdot \cos \frac{5 \pi}{36}}{2 \cos ^2 \frac{5 \pi}{36}-2 i \sin \frac{5 \pi}{36} \cos \frac{5 \pi}{36}} \Rightarrow\left(\frac{\mathrm{e}^{i \frac{5 \pi}{36}}}{\mathrm{e}^{-i \frac{5 \pi}{36}}}\right)^3 \\ & =e^{\left(\frac{5 \pi}{18}\right)^3}=e^{i\left(\frac{5 \pi}{6}\right)} \\ & \left(\cos \frac{5 \pi}{6}+i \sin \frac{5 \pi}{6}\right)=-\frac{\sqrt{3}}{2}+\frac{i}{2}\end{aligned}$

Hence, the answer is the option 1.

Example 4: The complex number $z=\frac{i-1}{\cos \frac{\pi}{3}+i \sin \frac{\pi}{3}}$ is equal to :

1) $\sqrt{2} i\left(\cos \frac{5 \pi}{12}-i \sin \frac{5 \pi}{12}\right)$
2) $\sqrt{2}\left(\cos \frac{\pi}{12}+\sin \frac{\pi}{12}\right)$
3) $\sqrt{2}\left(\cos \frac{5 \pi}{12}+i \sin \frac{5 \pi}{12}\right)$
4) $\cos \frac{\pi}{12}-1 \sin \frac{\pi}{12}$

Solution

$Z=\frac{i-1}{\cos \frac{\pi}{3}+i \sin \frac{\pi}{3}}$
$\mathrm{i}-1=\sqrt{2}\left(\frac{-1}{\sqrt{2}}+\frac{\mathrm{i}}{\sqrt{2}}\right)=\sqrt{2} \cdot \mathrm{e}^{\mathrm{i} \frac{3 \pi}{4}}$
$z=\frac{\sqrt{2} \cdot e^{i \frac{3 \pi}{4}}}{e^{i \cdot \frac{3 \pi}{4}}}$
$=\sqrt{2} \cdot \mathrm{e}^{\mathrm{i}\left(\frac{3 \pi}{4}-\frac{\pi}{3}\right)}$
$=\sqrt{2} \mathrm{e}^{\frac{5 \pi}{12} \mathrm{i}}$
$=\sqrt{2}\left(\cos \left(\frac{5 \pi}{12}\right)+\mathrm{i} \sin \left(\frac{5 \pi}{12}\right)\right)$
Hence, the answer is the option (3).

Summary

One of the fundamental theorems of complex numbers, De Moivre's theorem is utilized to address a variety of complex number problems. The solution of trigonometric functions with numerous angles is another common use of this theorem. "De Moivre's Identity" and "De Moivre's Formula" are other names for DeMoivre's Theorem.

Frequently Asked Questions (FAQs)

1. What is De Moivre’s Theorem?

De Moivre’s theorem is the basic theorem used in complex numbers for solving various problems. The De Moivre’s theorem states that, $(\cos x+i \sin x)^n=\cos (n x)+i \sin (n x)$

2. What are the uses of De Moivre’s Theorem?

The various uses of De Moivre’s theorem include the solving of complex roots, finding the power of the complex number, and others.

3. Does De Moivre’s Theorem work for Non-Integer Powers?

No, De Moivre’s formula does not work for non-integer powers. The result for non-negative integers is the multiple-value different from the original results.

4. Who invented De Moivre’s Theorem?

De Moivre’s theorem was first introduced by the French mathematician Abraham De Moivre.

5. What is De Moivre's Theorem and why is it important in complex number theory?

De Moivre's Theorem is a powerful formula that relates complex numbers to trigonometry. It states that for any real number x and integer n, (cos x + i sin x)^n = cos(nx) + i sin(nx). This theorem is important because it simplifies the process of raising complex numbers to powers and finding roots of complex numbers, making many calculations in complex analysis much easier.

6. How does De Moivre's Theorem connect trigonometry and complex numbers?

De Moivre's Theorem creates a bridge between trigonometry and complex numbers by expressing the powers of complex numbers in polar form using trigonometric functions. It shows that when you raise a complex number in its trigonometric form (cos θ + i sin θ) to a power n, the result is another complex number with an angle that's n times the original angle.

7. Can you explain the intuition behind De Moivre's Theorem?

Intuitively, De Moivre's Theorem tells us that when we multiply complex numbers in polar form, we add their angles. When we raise a complex number to a power n, we're essentially multiplying it by itself n times. Therefore, we're adding its angle to itself n times, which is equivalent to multiplying the angle by n. This is why (cos θ + i sin θ)^n = cos(nθ) + i sin(nθ).

8. What are the limitations of De Moivre's Theorem?

While De Moivre's Theorem is very powerful, it has some limitations. It only applies to complex numbers in the form cos θ + i sin θ (i.e., complex numbers with a magnitude of 1). For other complex numbers, we need to factor out the magnitude before applying the theorem. Also, in its basic form, it only works for integer powers, though it can be extended to rational powers.

9. How can De Moivre's Theorem be used to find roots of complex numbers?

De Moivre's Theorem can be used to find nth roots of complex numbers by expressing the complex number in polar form (r(cos θ + i sin θ)), taking the nth root of the magnitude r, and dividing the angle θ by n. This gives one root. To find all n roots, we add multiples of 2π/n to the angle, giving us n equally spaced points on a circle in the complex plane.

10. How is De Moivre's Theorem proved?

De Moivre's Theorem is typically proved using mathematical induction. The base case (n=1) is trivial. Then, assuming the theorem holds for some k, we prove it holds for k+1 by multiplying both sides of the equation for k by (cos θ + i sin θ) and using trigonometric addition formulas. This establishes the theorem for all positive integers, and it can be extended to negative integers and rational numbers.

11. What's the relationship between De Moivre's Theorem and Euler's formula?

De Moivre's Theorem is closely related to Euler's formula, e^(iθ) = cos θ + i sin θ. In fact, De Moivre's Theorem can be derived from Euler's formula. If we raise both sides of Euler's formula to the power n, we get (e^(iθ))^n = e^(inθ) = cos(nθ) + i sin(nθ), which is essentially De Moivre's Theorem.

12. How can De Moivre's Theorem help in solving polynomial equations?

De Moivre's Theorem is particularly useful in solving certain types of polynomial equations, especially those of the form x^n = a, where a is a complex number. By expressing a in polar form and using the theorem, we can find all n complex roots of the equation relatively easily.

13. How does De Moivre's Theorem relate to the binomial theorem?

De Moivre's Theorem can be used in conjunction with the binomial theorem to derive formulas for cos(nθ) and sin(nθ) in terms of powers of cos θ and sin θ. This connection provides another way to prove trigonometric multiple angle formulas.

14. What's the connection between De Moivre's Theorem and trigonometric identities?

De Moivre's Theorem can be used to derive many trigonometric identities. For example, by expanding (cos θ + i sin θ)^n using the binomial theorem and equating real and imaginary parts, you can derive formulas for cos(nθ) and sin(nθ) in terms of powers of cos θ and sin θ.

15. How does De Moivre's Theorem relate to the concept of complex logarithms?

De Moivre's Theorem is closely related to complex logarithms. The complex logarithm is the inverse of the complex exponential function, and De Moivre's Theorem can be seen as a special case of the exponential function. Understanding this relationship helps in grasping the multivalued nature of complex logarithms.

16. Can De Moivre's Theorem be used for complex numbers not on the unit circle?

While De Moivre's Theorem is typically stated for complex numbers on the unit circle (i.e., with magnitude 1), it can be extended to any complex number. For a complex number z = r(cos θ + i sin θ), we have z^n = r^n(cos(nθ) + i sin(nθ)). This extension is crucial for applying the theorem to a wider range of problems.

17. Can De Moivre's Theorem be used to simplify calculations involving complex matrices?

Yes, De Moivre's Theorem can simplify calculations with certain types of complex matrices, particularly rotation matrices. For 2x2 rotation matrices in the complex plane, the theorem provides a simple way to compute powers of these matrices, which is useful in computer graphics and other applications involving repeated rotations.

18. How does De Moivre's Theorem help in understanding the behavior of dynamical systems?

In the study of dynamical systems, De Moivre's Theorem is useful for analyzing systems with periodic or quasi-periodic behavior. It helps in understanding how complex eigenvalues of a system's transition matrix relate to its long-term behavior, particularly in terms of rotations in phase space.

19. How does De Moivre's Theorem relate to the concept of complex power series?

De Moivre's Theorem is closely related to complex power series. It provides a way to understand how certain power series behave when evaluated at complex numbers. For example, the series expansions of cos(z) and sin(z) for complex z can be derived using De Moivre's Theorem and Euler's formula.

20. Can De Moivre's Theorem be used to simplify calculations in spherical trigonometry?

While not directly applicable to spherical trigonometry, De Moivre's Theorem can be useful in certain calculations involving spherical coordinates. By expressing spherical harmonics in terms of complex exponentials, the theorem can simplify some computations in fields like geodesy and astronomy that use spherical trigonometry.

21. How does De Moivre's Theorem help in understanding the concept of fractional calculus?

In fractional calculus, which deals with derivatives and integrals of non-integer order, De Moivre's Theorem plays a role in defining and understanding complex-order derivatives. The theorem helps in extending the concept of differentiation to complex powers, which is crucial in this field.

22. How can De Moivre's Theorem be used to solve systems of trigonometric equations?

De Moivre's Theorem can be a powerful tool for solving systems of trigonometric equations. By converting the trigonometric expressions to complex exponential form using Euler's formula and applying the theorem, some systems of equations become more manageable and can be solved more easily.

23. Can De Moivre's Theorem be used to simplify calculations involving quaternions?

While De Moivre's Theorem itself doesn't directly apply to quaternions (which are 4-dimensional), a similar principle can be derived for quaternions. Understanding the complex number case through De Moivre's Theorem provides insight into how this principle works for higher-dimensional number systems like quaternions.

24. How does De Moivre's Theorem help in understanding the behavior of complex dynamical systems?

In the study of complex dynamical systems, De Moivre's Theorem is useful for analyzing periodic points and their stability. It helps in understanding how points rotate around fixed points under iteration, which is crucial in determining the long-term behavior of these systems.

25. What's the connection between De Moivre's Theorem and the theory of character sums in number theory?

In number theory, De Moivre's Theorem is related to the theory of character sums, particularly those involving roots of unity. The theorem helps in evaluating certain types of character sums and in understanding their properties, which has applications in areas like cryptography and coding theory.

26. How can De Moivre's Theorem be used to derive identities for hyperbolic functions?

While De Moivre's Theorem is typically associated with trigonometric functions, it can also be used to derive identities for hyperbolic functions. By using the relationship between complex exponentials and hyperbolic functions (e^x = cosh x + sinh x), similar identities for powers of hyperbolic functions can be derived.

27. What role does De Moivre's Theorem play in the theory of elliptic functions?

In the theory of elliptic functions, which are complex functions with two periods, De Moivre's Theorem helps in understanding the behavior of these functions under composition and iteration. It's particularly useful in studying the periodicity and symmetry properties of elliptic functions.

28. How does De Moivre's Theorem simplify trigonometric calculations?

De Moivre's Theorem can simplify complex trigonometric calculations, especially those involving powers of sines and cosines. For example, to calculate cos 3θ, we can use the theorem to expand (cos θ + i sin θ)^3 and then equate the real parts. This is often easier than using multiple angle formulas in trigonometry.

29. Can De Moivre's Theorem be used for non-integer powers?

Yes, De Moivre's Theorem can be extended to non-integer powers, including fractional and even irrational powers. For fractional powers, it's particularly useful in finding roots of complex numbers. For irrational powers, the result is still valid but may not have a simple geometric interpretation.

30. How does De Moivre's Theorem relate to the complex exponential function?

De Moivre's Theorem is closely related to the complex exponential function. In fact, it can be seen as a special case of the more general exponential law (e^a)^n = e^(an) when applied to complex numbers. This connection helps unify exponential, trigonometric, and complex number theories.

31. What are some practical applications of De Moivre's Theorem?

De Moivre's Theorem has numerous practical applications. It's used in signal processing, electrical engineering for analyzing alternating currents, in computer graphics for rotations, in quantum mechanics for describing spin states, and in many areas of pure mathematics like number theory and complex analysis.

32. What's the geometric interpretation of De Moivre's Theorem?

Geometrically, De Moivre's Theorem describes rotation in the complex plane. When you raise a complex number to a power n, you're rotating its angle n times around the origin. This gives a beautiful visual representation of complex number multiplication and exponentiation.

33. Can De Moivre's Theorem be used for complex roots of unity?

Yes, De Moivre's Theorem is particularly useful for finding and understanding complex roots of unity. The nth roots of unity are given by e^(2πik/n) = cos(2πk/n) + i sin(2πk/n) for k = 0, 1, ..., n-1. This formula comes directly from De Moivre's Theorem.

34. How does De Moivre's Theorem help in understanding the behavior of complex functions?

De Moivre's Theorem helps in understanding how complex functions behave under exponentiation. It shows that raising a complex number to a power results in a rotation and scaling in the complex plane, which is crucial for analyzing the behavior of complex analytic functions.

35. How does De Moivre's Theorem help in understanding the periodicity of complex exponentials?

De Moivre's Theorem helps explain why complex exponentials are periodic. Since cos θ + i sin θ = cos(θ + 2π) + i sin(θ + 2π), raising both sides to the nth power shows that the complex exponential function has a period of 2π/n. This insight is fundamental in many areas of mathematics and physics.

36. What role does De Moivre's Theorem play in Fourier analysis?

De Moivre's Theorem is fundamental to Fourier analysis. It allows us to express periodic functions as sums of sines and cosines (or complex exponentials), which is the basis of Fourier series. The theorem helps in understanding how these components combine and how the coefficients relate to the original function.

37. How can De Moivre's Theorem be used to separate real and imaginary parts of complex powers?

De Moivre's Theorem provides a straightforward way to separate the real and imaginary parts of complex powers. For (cos θ + i sin θ)^n, the real part is cos(nθ) and the imaginary part is sin(nθ). This separation is often crucial in solving problems involving complex numbers.

38. What's the significance of De Moivre's Theorem in the theory of angular momentum in quantum mechanics?

In quantum mechanics, De Moivre's Theorem is crucial for understanding angular momentum. It's used in the mathematical description of spin states and in calculating how these states change under rotations. The theorem helps explain the quantization of angular momentum in quantum systems.

39. How does De Moivre's Theorem relate to the concept of winding numbers in complex analysis?

De Moivre's Theorem is closely related to the concept of winding numbers in complex analysis. The theorem essentially describes how many times a complex number winds around the origin when raised to a power. This connection is important in understanding the behavior of complex functions and in the theory of contour integrals.

40. What's the connection between De Moivre's Theorem and the roots of unity filter in signal processing?

De Moivre's Theorem is fundamental to the roots of unity filter in signal processing. This filter uses the complex roots of unity (given by De Moivre's Theorem) to separate a signal into its frequency components. Understanding the theorem helps in grasping how this filter operates and why it's effective.

41. How can De Moivre's Theorem be used to derive formulas for trigonometric functions of multiple angles?

De Moivre's Theorem provides an elegant way to derive formulas for trigonometric functions of multiple angles. By expanding (cos θ + i sin θ)^n using the binomial theorem and equating real and imaginary parts, you can obtain expressions for cos(nθ) and sin(nθ) in terms of powers of cos θ and sin θ.

42. What role does De Moivre's Theorem play in the theory of cyclotomic fields in abstract algebra?

In abstract algebra, De Moivre's Theorem is crucial in the study of cyclotomic fields, which are formed by adjoining roots of unity to the rational numbers. The theorem helps in understanding the structure of these fields, their Galois groups, and their applications in number theory.

43. What's the significance of De Moivre's Theorem in the theory of Riemann surfaces?

In the theory of Riemann surfaces, De Moivre's Theorem helps in understanding the behavior of multi-valued complex functions. It's particularly useful in visualizing how these functions behave under analytic continuation around branch points, which is crucial in defining and working with Riemann surfaces.

44. What role does De Moivre's Theorem play in the study of fractals?

In the study of fractals, particularly those generated by complex functions, De Moivre's Theorem is useful in understanding how certain transformations affect the fractal shape. It helps explain the self-similarity and rotational symmetry observed in many complex-plane fractals, such as the Mandelbrot set.

45. How does De Moivre's Theorem relate to the concept of phase in signal processing?

In signal processing, De Moivre's Theorem is crucial for understanding phase relationships. It shows how the phase of a complex signal changes when the signal is raised to a power, which is important in many signal processing applications, including modulation and demodulation techniques.