Derivative as Rate Measure: Definition, Formula, Examples

Derivative as a rate measurement is an important concept in calculus. It is useful in understanding the rate of a change in the function. The existence of a derivative at a point implies that the function has a specific rate of change at that point. These concepts of derivatives have been broadly applied in branches of mathematics, physics, engineering, economics, and biology.

JEE Main 2025: Sample Papers | Mock Tests | PYQs | Study Plan 100 Days

JEE Main 2025: Maths Formulas  | Study Materials

JEE Main 2025: Syllabus | Preparation Guide | High Scoring Topics 

In this article, we will cover the concept of the Derivative as Rate Measure. This topic falls under the broader category of Calculus, which is a crucial chapter in Class 11 Mathematics. This is very important not only for board exams but also for competitive exams, which even include the Joint Entrance Examination Main and other entrance exams: SRM Joint Engineering Entrance, BITSAT, WBJEE, and BCECE. A total of eight questions have been asked on this topic in JEE Main from 2013 to 2023, two questions in 2013, one question in 2020, one question in 2021, three in 2022, and one in 2023.

Change Measurement:

$
\text { Where } \frac{dR}{dt} \text { means the Rate of change of radius. }
$

The rate of any variable concerning time is rate measurement. This means according to small changes in time how much other factors change is rate measurement:

$
\begin{aligned}
& \Rightarrow \frac{d x}{d t}, \frac{d y}{d t}, \frac{d R}{d t},(\text { linear }), \frac{d a}{d t} \\
& \Rightarrow \frac{d S}{d t}, \frac{d A}{d t}(\text { Area })
\end{aligned}
$
$
\begin{aligned}
& \Rightarrow \frac{d V}{d t}(\text { Volume }) \\
& \Rightarrow \frac{d V}{V} \times 100(\text { percentage change in volume })
\end{aligned}
$

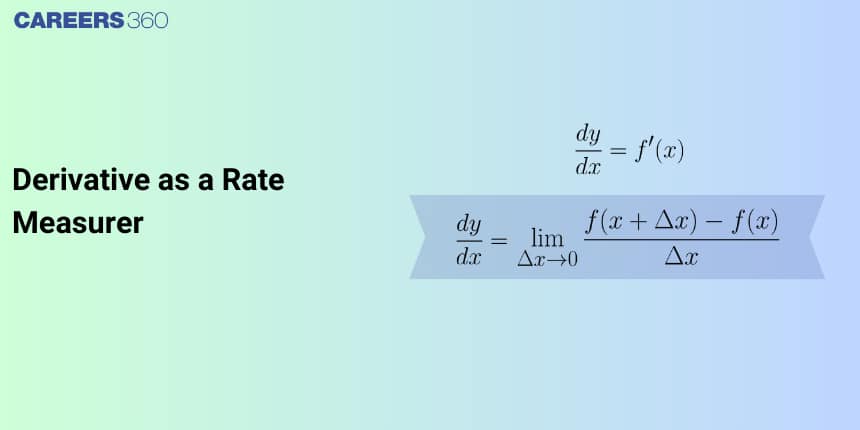
Recall that by the derivative $\frac{d s}{d t}$, we mean the rate of change of distance $s$ with respect to the time $t$. In a similar fashion, whenever one quantity $y$ varies with another quantity $x$, satisfying some rule $y=f(x)$, then $\frac{d y}{d x}$ (or $f^{\prime}(x)$ ) represents the rate of change of $y$ with respect to $x$ and $\left.\frac{d y}{d x}\right]_{x=x_0}$ (or $f^{\prime}\left(x_0\right)$ ) represents the rate of change of $y$ with respect to $x$ at $x=x_0$.

Further, if two variables $x$ and $y$ are varying with respect to another variable $t$, i.e., if $x=f(t)$ and $y=g(t)$, then by Chain Rule
$
\frac{d y}{d x}=\frac{d y}{d t} / \frac{d x}{d t}, \text { if } \frac{d x}{d t} \neq 0
$

Thus, the rate of change of $y$ concerning $x$ can be calculated using the rate of change of $y$ and that of $x$ both concerning $t$.

The rate of change of $y$ concerning $x$ can be calculated using the rate of change of $y$ and that of $x$ both concerning $t$ whenever one quantity $y$ varies with another quantity x , satisfying some rule $\mathrm{y}=\mathrm{f}(\mathrm{x})$, then $\frac{d y}{d x}$ (or $f^{\prime}(x)$ )represents the rate of change of $y$ for $x$.

Recall that by the derivative $\frac{d s}{d t}$, we mean the rate of change of distance s concerning the time $t$.

Derivative as Rate Measure

If two related quantities are changing over time, the rates at which the quantities change are related. For example, if a balloon is being filled with air, both the radius of the balloon and the volume of the balloon are increasing.

If a variable quantity $y$ depends on and varies with a quantity $x$, then the rate of change of y for x is $\frac{d y}{d x}$.

A rate of change concerning time is simply called the rate of change.
For example, the rate of change of displacement (s) of an object w.r.t. time is velocity $(\mathrm{v})$.

$
v=\frac{d s}{d t}
$

Illustration:

Consider balloon example again, a spherical balloon is being filled with air at a constant rate of $2 \mathrm{~cm}^3 / \mathrm{sec}$. How fast is the radius increasing when the radius is 3 cm ?

The volume of a sphere of radius $r$ centimeters is,

$
V=\frac{4}{3} \pi r^3 \mathrm{~cm}^3
$
Since the balloon is being filled with air, both the volume and the radius are functions of time. Therefore, $t$ seconds after beginning to fill the balloon with air, the volume of air in the balloon is

$
V(t)=\frac{4}{3} \pi[r(t)]^3 \mathrm{~cm}^3
$
Differentiating both sides of this equation with respect to time and applying the chain rule, we see that the rate of change in the volume is related to the rate of change in the radius by the equation

$
\begin{aligned}
\frac{d}{d t}(V(t)) & =\frac{4}{3} \pi \frac{d}{d t}\left([r(t)]^3\right) \mathrm{cm}^3 \\
V^{\prime}(t) & =4 \pi[r(t)]^2 r^{\prime}(t)
\end{aligned}
$
The balloon is filled with air at a constant rate of $2 \mathrm{~cm}^3 / \mathrm{sec}$. So, $\mathrm{V}^{\prime}(\mathrm{t})=2$

$
\begin{aligned}
& \mathrm{cm}^3 / \mathrm{sec} \\
& \therefore \quad 2 \mathrm{~cm}^3 / \mathrm{sec}=\left(4 \pi[r(t)]^2 \mathrm{~cm}^2\right) \cdot r^{\prime}(t) \\
& \Rightarrow \quad r^{\prime}(t)=\frac{1}{2 \pi[r(t)]^2} \mathrm{~cm} / \mathrm{sec}
\end{aligned}
$
When the radius $\mathrm{r}=3 \mathrm{~cm}$

$
\Rightarrow \quad r^{\prime}(t)=\frac{1}{18 \pi} \mathrm{cm} / \mathrm{sec}
$

Recommended Video Based on Derivative as Rate Measure

Solved Examples Based On Derivative as Rate Measure:

Example 1: If the surface area of a sphere of radius $r$ is increasing uniformly at the rate $8 \mathrm{~cm}^2 / \mathrm{s}$, then the rate of change of its volume is :
1) Constant
2) Proportional to $\sqrt{r}$
3) Proportional to $r^2$
4) Proportional to $r$

Solution

$
\begin{aligned}
& \mathrm{V}=\frac{4}{3} \pi r^3 \Rightarrow \frac{d \mathrm{~V}}{d t}=4 \pi r^2 \cdot \frac{d r}{d t} \\
& \mathrm{~S}=4 \pi r^2 \Rightarrow \frac{d \mathrm{~S}}{d t}=8 \pi r \cdot \frac{d r}{d t} \\
& \Rightarrow 8=8 \pi r \frac{d r}{d t} \Rightarrow \frac{d r}{d t}=\frac{1}{\pi r}
\end{aligned}
$
Putting the value of $\frac{d r}{d t}$ in (i), we get $\frac{d \mathrm{~V}}{d t}=4 \pi r^2 \times \frac{1}{\pi r}=4 r$
$\Rightarrow \frac{d \mathrm{~V}}{d t}$ is proportional to $r$.

Hence, the answer is option 4.

Example 2: A spherical balloon is being inflated at the rate of $35 \mathrm{cc} / \mathrm{min}$. The rate of increase in surface area( in $\mathrm{cm}^2 / \mathrm{min}$.) of the balloon when its diameter is 14 cm , is :
1) 10
2) $\sqrt{10}$
3) 100
4) $10 \sqrt{10}$

Solution

Volume of the spherical ballon is, $\mathrm{V}=\frac{4}{3} \pi r^3$

$
\begin{aligned}
& \frac{d \mathrm{~V}}{d t}=\frac{4}{3} \cdot \pi \cdot 3 r^2 \cdot \frac{d r}{d t} \\
& 35=4 \pi r^2 \cdot \frac{d r}{d t} \text { or } \frac{d r}{d t}=\frac{35}{4 \pi r^2}
\end{aligned}
$
Now, surface area of sphere is, $S=4 \pi r^2$

$
\begin{aligned}
& \frac{d S}{d t}=(4 \pi) 2 r \frac{d r}{d t} \\
& \frac{d \mathrm{~S}}{d t}=8 \pi r \times \frac{35}{4 \pi r^2}=\frac{70}{r}
\end{aligned}
$
Now, diameter $=14 \mathrm{~cm}, r=7$

$
\therefore \quad \frac{d \mathrm{~S}}{d t}=10
$
Hence, the answer is option 1.

Example 3: The population $\mathrm{P}=\mathrm{P}(\mathrm{t})$ at time ' $t$ ' of ascertain species follows the differential equation. Then the time at which the population becomes zero:
1) $\log _e 18$
2) $\frac{1}{2} \log _e 18$
3) $\log _e 9$
4) $2 \log _e 18$

Solution

$
\begin{aligned}
& \frac{\mathrm{dP}(\mathrm{t})}{\mathrm{dt}}=\frac{\mathrm{P}(\mathrm{t})-900}{2} \\
& \int_0^t \frac{\mathrm{dP}(\mathrm{t})}{\mathrm{P}(\mathrm{t})-900}=\int_0^{\mathrm{t}} \frac{\mathrm{dt}}{2} \\
& \{\ell \ln |\mathrm{P}(\mathrm{t})-900|\}_0^{\mathrm{t}}=\left\{\frac{\mathrm{t}}{2}\right\}_0^{\mathrm{t}} \\
& \operatorname{\ell n}|\mathrm{P}(\mathrm{t})-900|-\ln |\mathrm{P}(0)-900|=\frac{\mathrm{t}}{2} \\
& \ln |\mathrm{P}(\mathrm{t})-900|-\ln 50=\frac{\mathrm{t}}{2}
\end{aligned}
$
Let at $t=t_1, P(t)=0$ hence

$
\begin{aligned}
& \ln |\mathrm{P}(\mathrm{t})-900|-\ln 50=\frac{\mathrm{t}_1}{2} \\
& \mathrm{t}_1=2 \ell \mathrm{n} 18
\end{aligned}
$
Hence, the answer is the option 4.

Example 4: The surface area of a balloon of spherical shape being inflated, increases at a constant rate. If initially, the radius of the balloon is 3 units and after 5 seconds, it becomes 7 units, then its radius after 9 seconds is:
1) $9$
2) $10$
3) $11$
4) $12$

Solution

Let r: radius

$
\begin{aligned}
& \mathrm{s}=4 \pi \mathrm{r}^2 \\
& \frac{\mathrm{ds}}{\mathrm{dt}}=8 \pi \mathrm{r}\left(\frac{\mathrm{dr}}{\mathrm{dt}}\right)=\lambda \text { (constant) } \\
& 4 \pi \mathrm{r}^2=\lambda \mathrm{t}+\mathrm{c} \\
& \text { at } \mathrm{t}=0, \mathrm{r}=3 \quad \Rightarrow \quad \mathrm{c}=36 \pi \\
& \text { at } \mathrm{t}=5, \mathrm{r}=7 \quad \Rightarrow \quad \lambda=32 \pi \\
& \therefore \lambda^2=8 \mathrm{t}+9 \\
& \therefore \text { at } \mathrm{t}=9 \\
& \quad \mathrm{r}^2=81 \\
& \mathrm{r}=9 \mathrm{unit}
\end{aligned}
$
Hence, the answer is the option 1.

Example 5: Water is being filled at the rate of $1 \mathrm{~cm}^3 / \mathrm{sec}_{\text {in }}$ in a right circular conical vessel ( vertex downwards ) of height 35 cm and diameter 14 cm . When the height of the water level is 10 cm , the rate ( in $\mathrm{cm}^2 / \mathrm{sec}$ ) at which the wet conical surface area of the vessel increases is:
1) 5
2) $\frac{\sqrt{21}}{5}$
3) $\frac{\sqrt{26}}{5}$
4) $\frac{\sqrt{26}}{10}$

Solution

Let the volume of the cone be $\mathrm{V} \mathrm{cm}{ }^3$
Given $\frac{\mathrm{dV}}{\mathrm{dt}}=1 \mathrm{~cm}^3 / \mathrm{sec}, \mathrm{h}=35 \mathrm{~cm}, \mathrm{r}=7 \mathrm{~cm}$
i.e $\frac{\mathrm{h}}{\mathrm{r}}=5$

We know for a cone $\mathrm{l}^2=\mathrm{r}^2+\mathrm{h}$
Lateral Surface area,

$
\begin{aligned}
& \mathrm{S}=\pi \mathrm{r} \sqrt{\mathrm{r}^2+\mathrm{h}^2} \\
& \mathrm{~S}=\pi \frac{\mathrm{h}}{5} \sqrt{\frac{\mathrm{h}^2}{25}+\mathrm{h}^2}=\pi \frac{\sqrt{26}}{25} \mathrm{~h}^2 \\
& \mathrm{~V}=\frac{1}{3} \pi \mathrm{r}^2 \mathrm{~h} \frac{1}{3} \pi\left(\frac{\mathrm{h}}{5}\right)^2 \mathrm{~h}=\frac{\pi}{75} \mathrm{~h}^3 \\
& \Rightarrow \frac{\mathrm{dV}}{\mathrm{dt}}=\frac{\pi}{25} \mathrm{~h}^2 \frac{\mathrm{dh}}{\mathrm{dt}} \Rightarrow \frac{\pi}{25} \mathrm{~h}^2 \frac{\mathrm{dh}}{\mathrm{dt}}=1 \\
& \Rightarrow \frac{\mathrm{dh}}{\mathrm{dt}}=\frac{25}{\pi \mathrm{h}^2}
\end{aligned}
$

$
\begin{aligned}
& \frac{\mathrm{ds}}{\mathrm{dt}}=\frac{\pi \sqrt{26}}{25} \times 2 \mathrm{~h} \frac{\mathrm{dh}}{\mathrm{dt}}=\frac{2 \sqrt{26}}{\mathrm{~h}} \\
& \left(\frac{\mathrm{ds}}{\mathrm{dt}}\right)_{\mathrm{h}=10}=\frac{\sqrt{26}}{5}
\end{aligned}
$
Hence, the answer is the option (3).

Summary:

Rate measurement is an important topic in mathematics that tells us the degree of rat change following the input. This concept is crucial as it involves calculating the rate of change of variables such as displacement, volume, and surface area. We can solve real-world problems with the help of this topic.

FAQs:

1) What is rate measurement?

Solution: The rate of any variable concerning time is rate measurement.
2) What is derivative?

Solution: The instantaneous rate of change of a function concerning the independent variable is called the derivative.
3) What are common types of derivatives used in Rate Measurement?

Solution: Common types of derivatives used in Rate Measurement are velocity, acceleration, and growth rate.
4) What is rate of change of $y$ for $x$ ?

Solution: If a variable quantity y depends on and varies with a quantity x , $\frac{d y}{d x}$
5) What is differentiation?

Solution: The process of finding the derivative of a function is called differentiation