Differentiability and Existence of Derivative: Difference, Examples

Differentiability is an important concept in calculus. It is useful in understanding the rate of a change in the function. The existence of a derivative at a point implies that the function has a specific rate of change at that point. These concepts of differentiability have been broadly applied in branches of mathematics, physics, engineering, economics, and biology.

JEE Main 2025: Sample Papers | Mock Tests | PYQs | Study Plan 100 Days

JEE Main 2025: Maths Formulas  | Study Materials

JEE Main 2025: Syllabus | Preparation Guide | High Scoring Topics 

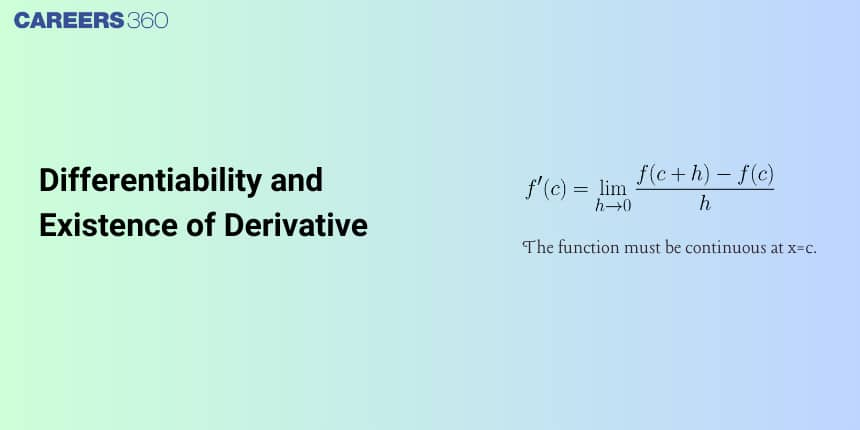
In this article, we will cover the concept of the differentiability and existence of Derivatives. This topic falls under the broader category of Calculus, a crucial chapter in Class 11 Mathematics. This is very important not only for board exams but also for competitive exams, which even include the Joint Entrance Examination Main and other entrance exams: SRM Joint Engineering Entrance, BITSAT, WBJEE, and BCECE. A total of ten questions have been asked on this topic in JEE Main from 2013 to 2023, one question in 2018, three questions in 2019, two questions in 2020, one in 2021, and three in 2023.

Differentiability and Existence of Derivative

Derivative: The instantaneous rate of change of a function with respect to the independent variable is called derivative. Let $f(x)$ be a given function of one variable and $\Delta x$ denotes a number ( positive or negative) to be added to the number $x$.

Let $\Delta f$ denotes the corresponding change of f , then

$
\Delta f=f(x+\Delta x)-f(x)
$

if $\frac{\Delta f}{\Delta x}$ approaches a limit as $\Delta x$ approaches to zero, this limit is the derivative of $f$ at the point $x$. The derivative of a function $f$ is denoted by symbols such as $f^{\prime}(x), \frac{\mathrm{d} f}{\mathrm{~d} x}, \frac{\mathrm{d}}{\mathrm{d} x} f(x)$ or $\frac{\mathrm{d} f(x)}{\mathrm{d} x}$.
$\Rightarrow \frac{\mathrm{d} f}{\mathrm{~d} x}=\lim\limits_{\Delta x \rightarrow 0} \frac{\Delta f}{\Delta x}=\lim\limits_{\Delta x \rightarrow 0} \frac{f(x+\Delta x)-f(x)}{\Delta x}$ the derivative evaluated at a point a is written $f^{\prime}(a),\left(\frac{\mathrm{d} f(x)}{\mathrm{d} x}\right)_{x=a},\left(f^{\prime}(x)\right)_{x=a}$ etc.

Geographical representation of Derivative

Where ( $\mathrm{x}, \mathrm{y}$ ) on the curve and $\mathrm{M}_{\mathrm{T}}$ is tangent at ( $\mathrm{x}, \mathrm{y}$ ).
Let $P$ be any point $(x, y)$ on the curve $y=f(x)$ and $Q$ is a point in the neighborhood of $P$ on either side of $P$. such that the co-ordinate of the point $Q$ are
$(x+\delta x, y+\delta y)$ satisfying the curve $\mathrm{y}=\mathrm{f}(\mathrm{x})$
$\therefore M_T=$ slope of tangent
$=\lim\limits_{\delta x \rightarrow 0} \frac{(y+\delta y)-y}{(x+\delta x)-x}=\lim\limits_{\delta x \rightarrow 0} \frac{\delta y}{\delta x}$
$\therefore \quad M_T=\left(\frac{d y}{d x}\right)$ at $(x, y)$
The process of finding the derivative of a function is called differentiation. We also use the phrase differentiate $f(x)$ with respect to $x$ to mean find $f$ '(x)

Whenever we defined derivative, we had put a caution provided the limit exists. Now the natural question is; what if it doesn't? The question is quite pertinent and so is its answer. If $\lim\limits_{h \rightarrow 0} \frac{f(c+h)-f(c)}{h}$ does not exist, we say that $f$ is not differentiable at c. In other words, we say that a function f is differentiable at a point c in its domain if both $\lim\limits_{h \rightarrow 0^{-}} \frac{f(c+h)-f(c)}{h}$ and

$
\lim\limits_{h \rightarrow 0^{+}} \frac{f(c+h)-f(c)}{h}
$

are finite and equal.

Differentiability:The derivative of a function $f(x)$ at point $a$ is defined by$
f^{\prime}(x) \text { at } \mathrm{x}=\text { a i.e, } f^{\prime}(a)=\lim\limits_{h \rightarrow 0} \frac{f(a+h)-f(a)}{h} \text { or } \lim\limits_{x \rightarrow a} \frac{f(x)-f(a)}{x-a}
$

provided that the limit exists.
If $\lim\limits_{h \rightarrow 0} \frac{f(a+h)-f(a)}{h}$ does not exists, we say that the function $\mathrm{f}(\mathrm{x})$ is not differentiable at $x=a$.

Or, we can say that a function $f(x)$ is differentiable at a point ' $a$ ' in its domain if limit of the function $f^{\prime}(x)$ exists at $x=a$.
i.e.$
\text { Right Hand Derivative }=\mathrm{RHD}=R f^{\prime}(a)=\lim\limits_{h \rightarrow 0} \frac{f(a+h)-f(a)}{h}
$ and$
\text { Left Hand Derivative }=\mathrm{LHD}=L f^{\prime}(a)=\lim\limits_{h \rightarrow 0} \frac{f(a-h)-f(a)}{-h}
$ are finite and equal.
(Both the left-hand derivative and the right-hand derivative are finite and equal.)

$R f^{\prime}(a)=L f^{\prime}(a)$ is the condition for differentiability at $x=a$

$
\lim\limits_{h \rightarrow 0} \frac{f(a+h)-f(a)}{h}=\lim\limits_{h \rightarrow 0} \frac{f(a-h)-f(a)}{-h}
$

Geometrical meaning of Right-hand derivative

Let $A(a, f(a))$ and $B(a+h, f(a+h))$ be two points very near to each other on the curve $y=f(x)$. Using the slope of a line formula, we get

Slope of $A B=\frac{f(a+h)-f(a)}{(a+h)-a}$
Now apply $\lim \mathrm{h} \rightarrow 0$ on both sides to get :

$
\lim\limits_{h \rightarrow 0}(\text { slope of chord } A B)=\lim\limits_{h \rightarrow 0} \frac{f(a+h)-f(a)}{h}
$
Right hand derivative

$
=R\left[f^{\prime}(a)\right]=\lim\limits_{h \rightarrow 0}(\text { slope of chord } A B)
$
As $h \rightarrow 0, B \rightarrow A$ on a curve, $a+h \rightarrow a$ on the $x$-axis, and $f(a+h) \rightarrow f(a)$ on the $y$-axis.

When $h$ is infinitely small, chord $A B$ almost becomes tangent drawn at A towards the right.

Hence the geometrical significance of the right-hand derivative is that it represents the slope of the tangent drawn at A towards the right.

Geometrical meaning of Left-hand derivative

Let $A(a, f(a))$ and $C(a-h, f(a-h))$ be two points very near to each other on the curve $y=f(x)$. Using the slope of a line formula, we get

Slope of $A C=\frac{f(a-h)-f(a)}{(a-h)-a}$
Now apply $\lim \mathrm{h} \rightarrow 0$ on both sides to get :

$
\lim\limits_{h \rightarrow 0}(\text { slope of chord } A C)=\lim\limits_{h \rightarrow 0} \frac{f(a-h)-f(a)}{-h}
$
Left hand derivative

$
=L\left[f^{\prime}(a)\right]=\lim\limits_{h \rightarrow 0}(\text { slope of chord } A C)
$
As $\mathrm{h} \rightarrow 0, \mathrm{C} \rightarrow \mathrm{A}$ on the curve, $\mathrm{a}-\mathrm{h} \rightarrow \mathrm{a}$ on the x -axis and $\mathrm{f}(\mathrm{a}-\mathrm{h}) \rightarrow \mathrm{f}(\mathrm{a})$ on the $y$-axis.

When $h$ is infinitely small, chord $A C$ almost becomes tangent drawn at $A$ towards the left.

Hence the geometrical significance of the left-hand derivative is that it represents the slope of the tangent drawn at $A$ towards the left.

Geometrical meaning of the existence of derivative

We know that the derivative of a function exists at $x=a$, if $L\left[f^{\prime}(a)\right]=R\left[f^{\prime}(a)\right]$
1. The slope of the tangent drawn at $A$ towards the left = slope of the tangent drawn at $A$ towards the right
2. The same tangent line towards left and right: meaning a unique tangent at the point
3. Smooth curve around $x=a$

Recommended Video Based on Tangents to the Curve at a Point

Solved Examples Based On Tangents to the curve at a point:

Example 1: Let $S$ be the set of all points in $(-\pi, \pi)$ at which the function, $f(x)=\min \{\sin x, \cos x\}$ is not differentiable. Then S is a subset of which of the following?
[JEE Main 2019]
1) $\left\{-\frac{3 \pi}{4},-\frac{\pi}{2}, \frac{\pi}{2}, \frac{3 \pi}{4}\right\}$
2) $\left\{-\frac{3 \pi}{4},-\frac{\pi}{4}, \frac{3 \pi}{4}, \frac{\pi}{4}\right\}$
3) $\left\{-\frac{\pi}{4}, 0, \frac{\pi}{4}\right\}$
4) $\left\{-\frac{\pi}{2},-\frac{\pi}{4}, \frac{\pi}{4}, \frac{\pi}{2}\right\}$

Solution

Differentiability -
Let $\mathrm{f}(\mathrm{x})$ be a real valued function defined on an open interval ( $\mathrm{a}, \mathrm{b})$ and $x \in(\mathrm{a}$, b). Then the function $\mathrm{f}(\mathrm{x})$ is said to be differentiable at $x_{\circ}$ if

$
\lim\limits_{h \rightarrow 0} \frac{f\left(x_0+h\right)-f\left(x_0\right)}{\left(x_0+h\right)-x_0}
$

or $\lim\limits_{x \rightarrow x_0} \frac{f(x)-f\left(x_0\right)}{x-x_0}$
-Hence number of points $f(x)$ is non-differentiable are 2 which are $\frac{-3 \pi}{4}$ and $\frac{\pi}{4}$

Example 2: Let $f(x)=\left\{\begin{array}{cc}\max \left\{|x|, x^2\right\}, & |x| \leq 2 \\ 8-2|x| & 2<|x| \leq 4 \text { Let } \mathrm{S} \text { be the }\end{array}\right.$ set of points in the interval $(-4,4)$ at which f is not differentiable. Then S : [JEE Main 2019]
1) is an empty set
2) equals $\{-2,-1,0,1,2\}$
3) equals $\{-2,-1,1,2\}$
4) equals $\{-2,2\}$

Solution

Condition for differentiability -
A function $\mathrm{f}(\mathrm{x})$ is said to be differentiable at $x=x_{\circ}$ if $R f^{\prime}\left(x_{\circ}\right)$ and $L f^{\prime}\left(x_{\circ}\right)$ both exist and are equal otherwise non differentiable

Geometrical interpretation of Derivative -
Let $P$ be any point $(x, y)$ on the curve $y=f(x)$ and $Q$ is a point in the neighbourhood of $P$ on either side of $P$. such that the co-ordinate of the point Q are
$(x+\delta x, y+\delta y)$ satisfying the curve $\mathrm{y}=\mathrm{f}(\mathrm{x})$
$\therefore M_T=$ slope of tangent

$
\begin{aligned}
& =\lim\limits_{\delta x \rightarrow 0} \frac{(y+\delta y)-y}{(x+\delta x)-x}=\lim\limits_{\delta x \rightarrow 0} \frac{\delta y}{\delta x} \\
& \therefore \quad M_T=\left(\frac{d y}{d x}\right) \text { at }(x, y)
\end{aligned}
$

- wherein

Where ( $x, y$ ) on the curve and $M_T$ is tangent at ( $x, y$ ).
$
f(x)=\left\{\begin{array}{ccc}
8+2 x, & & -4 \leq x<-2 \\
x^2, & & -2 \leq x \leq-1 \\
|x|, & & -1<x<1 \\
x^2, & & 1 \leq x \leq 2 \\
8-2 x, & & 2<x \leq 4
\end{array}\right.
$

$f(x)$ is not differentiable at $x=-2,-1,0,1,2$.

Example 3: Let $
S=\left\{(\lambda, \mu) \epsilon \mathbf{R} \times \mathbf{R}: f(t)=\left(|\lambda| e^{|t|}-\mu\right) \cdot \sin (2|t|), t \in \mathbf{R},\right\}
$

is a differentiable function. Then S is a subset of: [JEE Main 2018]
1) $\mathbf{R} \times[0, \infty]$
2) $[0, \infty] \times \mathbf{R}$
3) $\mathbf{R} \times[-\infty, 0]$
4) $[-\infty, 0] \times \mathbf{R}$

Solution

As we learned
-Condition for differentiable -
A function $\mathrm{f}(\mathrm{x})$ is said to be differentiable at $x=x_{\circ}$ if $R f^{\prime}\left(x_{\circ}\right)$ and $L f^{\prime}\left(x_{\circ}\right)$ both exist and are equal otherwise nondifferentiable

$
\begin{aligned}
& f(t)=\left(|\lambda| e^{|t|}-\mu\right) \sin (2|t|) \\
& =\left(|\lambda| e^t-\mu\right) \sin 2 t \quad t>0 \\
& =\left(|\lambda| e^{-t}-\mu\right) \sin 2 t \quad t<0
\end{aligned}
$

$f(t)$ is differentiable at $t=0$

$
\mathrm{LHD}=\mathrm{RHD}
$
$
\begin{aligned}
& |\lambda| \sin 2(0)+\left(|\lambda| e^{\circ}-\mu\right) 2 \cos 0=|\lambda| \sin 2(0)-2 \cos (0)\left(\lambda e^{-0}-\mu\right) \\
& \Rightarrow 4(|\lambda|-\mu)=0 \Rightarrow|\lambda|=\mu \\
& S \equiv(\lambda, \mu)=\{\lambda \equiv R, \mu \equiv(0, \infty)\}
\end{aligned}
$

S is a subset of $R \times(0, \infty)$
Hence, the answer is the option 1 .

Example 4: Let K be the set of all real values of x where the function $f(x)=\sin |x|-|x|+2(x-\pi) \cos |x|$ is not differentiable. Then the set K is equal to: [JEE Main 2019]
1) $\{0\}$
2) $\phi$ (an empty set)
3) $\{\pi\}$
4) $\{0, \pi\}$

Solution

Condition for differentiability -
A function $\mathrm{f}(\mathrm{x})$ is said to be differentiable at $x=x_{\circ}$ if $R f^{\prime}\left(x_{\circ}\right)$ and $L f^{\prime}\left(x_{\circ}\right)$ both exist and are equal otherwise non differentiable

Differentiability -
Let $\mathrm{f}(\mathrm{x})$ be a real valued function defined on an open interval $(\mathrm{a}, \mathrm{b})$ and $x \epsilon(\mathrm{a}$, b). Then the function $\mathrm{f}(\mathrm{x})$ is said to be differentiable at $x_{\circ}$ if

$
\begin{aligned}
& \lim\limits_{h \rightarrow 0} \frac{f\left(x_0+h\right)-f\left(x_0\right)}{\left(x_0+h\right)-x_0} \\
& \text { or } \lim\limits_{x \rightarrow x_0} \frac{f(x)-f\left(x_0\right)}{x-x_0}
\end{aligned}
$
Checking differentiability at $x=0$
for $x>0$,

$
\begin{aligned}
& f(x)=\sin x-x+2(x-\pi) \cos x \\
& f^{\prime}(x)=\cos x-1+2 \cos x-2(x-\pi) \sin x \\
& \mathrm{RHD}=f^{\prime}(0+)=1-1+2-2(-\pi) \cdot 0=2
\end{aligned}
$

for $x<0$,

$
\begin{aligned}
& f(x)=-\sin x+x+2(x-\pi) \cos x \\
& f^{\prime}(x)=-\cos x+1+2 \cos x-2(x-\pi) \sin x \\
& \text { LHD }=f^{\prime}(0-)=-1+1+2-2(-\pi) \cdot 0=2 \\
& \because \text { LHD }=\text { RHD }
\end{aligned}
$

differentiable at $x=0 \Rightarrow>$ differentiable everywhere
Hence, the answer is the option 2.

Example 5: Suppose differential function $f(x)$ satisfies the identity $f(x+y)=f(x)+f(y)+x y^2+x^2 y$, for all real x and y . If $\lim\limits_{x \rightarrow 0} \frac{(x)}{x}=1$ then $\mathrm{f}(3)$ is equal to: [JEE Main 2020]
1) 8
2) 10
3) 12
4) 14

Solution

Since, $\lim\limits_{x \rightarrow 0} \frac{f(x)}{x}$ exist $\Rightarrow f(0)=0$
Now, $f^{\prime}(x)=\lim\limits_{h \rightarrow 0} \frac{f(x+h)-f(x)}{h}$

$
\begin{aligned}
& =\lim\limits_{h \rightarrow 0} \frac{f(h)+x h^2+x^2 h}{h}(\text { take } y=h) \\
& =\lim\limits_{h \rightarrow 0} \frac{f(h)}{h}+\lim\limits_{h \rightarrow 0}(x h)+x^2 \\
& \Rightarrow f^{\prime}(x)=1+0+x^2 \\
& \Rightarrow f^{\prime}(3)=10
\end{aligned}
$
Hence, the answer is the option 2.

Summary

The derivative is an important concept of the limits. Differentiation of a function at a point represents the slope of the tangent to the graph of the function at that point. With the help of differentiation, we can find the rate of change of one quantity for another. The concept of differentiation is the cornerstone on which the development of calculus rests.