Differentiation of Determinants

A determinant is a special number that can be determined from a matrix. For a determinant to exist, matrix A must be a square matrix. The determinant of the matrix is denoted by det A or |A|. Finding the derivate of the determinants is important to analyze the behaviour of the determinants with respect to the parameter. In real life, we can use determinant in graphic designing, and gaming. Determinants also help us in taking necessary steps in business.

JEE Main: Study Materials | High Scoring Topics | Preparation Guide

JEE Main: Syllabus | Sample Papers | Mock Tests | PYQs

This Story also Contains

1. Determinants
2. Differentiation
3. Differentiation of Determinants
4. Solved Examples Based on Differentiation of Determinant
5. Summary

In this article, we will learn the properties of Differentiation of Determinants. This category falls under the broader category of matrices, a crucial Chapter in class 12 Mathematics. It is not only essential for board exams but also for competitive exams like the Joint Entrance Examination(JEE Main) and other entrance exams such as SRMJEE, BITSAT, WBJEE, BCECE, and more. A total of one question has been asked on this topic in 2022.

Determinants

The determinant of a matrix A is a number that is calculated from the matrix. For a determinant to exist, matrix A must be a square matrix. The determinant of the matrix is denoted by $\det A$ or $|A|$.

Properties of Determinant:

1. The value of the determinant remains unchanged if its rows and columns are interchanged.
2. If any two rows or two columns of a determinant are interchanged, then the sign of the determinant changes but the numerical value remains unaltered.
3. If there is an interchange of rows or columns twice, then the value of the determinant remains the same.
4. If any two rows (or columns) of a determinant are identical (all corresponding elements are the same), then the value of the determinant is zero.

Differentiation

The rate of change of a quantity $y$ concerning another quantity $x$ is called the derivative or differential coefficient of $y$ concerning $x$. Differentiation is a derivative of an independent variable's value that can be used to calculate characteristics in an independent variable per unit change.

Derivatives of some basic functions

1. $\frac{d}{dx}($ constant $)=0$

2. $\frac{d}{dx}\left({\mathbf{x}}^{\mathbf{n}}\right)=\mathbf{n}{\mathbf{x}}^{\mathbf{n}-1}$

3. $\frac{d}{dx}\left({\mathbf{a}}^{\mathrm{x}}\right)={\mathbf{a}}^{\mathrm{x}}{\log }_{\mathrm{e}}\mathbf{a}$

4. $\frac{d}{dx}\left({\mathrm{e}}^{\mathrm{x}}\right)={\mathrm{e}}^{\mathrm{x}}{\log }_{\mathrm{e}}\mathrm{e}={\mathrm{e}}^{\mathrm{x}}$

5. $\frac{d}{dx}({\log }_{\mathrm{e}}|\mathbf{x}|)=\frac{\mathbf{1}}{\mathbf{x}},\mathbf{x}\ne 0$

6. $\frac{d}{dx}({\log }_{\mathbf{a}}|\mathbf{x}|)=\frac{1}{\mathbf{x}{\log }_{\mathrm{e}}\mathbf{a}},\mathbf{x}\ne 0$

7. $\frac{d}{dx}(\sin (\mathbf{x}))=\cos (\mathbf{x})$

8. $\frac{d}{dx}(\cos (\mathbf{x}))=-\sin (\mathbf{x})$

9. $\frac{d}{dx}(\tan (\mathbf{x}))={\sec }^2(\mathbf{x})$

10. $\frac{d}{dx}(\cot (\mathbf{x}))=-{\csc }^2(\mathbf{x})$

11. $\frac{d}{dx}(\sec (\mathbf{x}))=\sec (\mathbf{x})\tan (\mathbf{x})$

12. $\frac{d}{dx}(\csc (\mathbf{x}))=-\csc (\mathbf{x})\cot (\mathbf{x})$

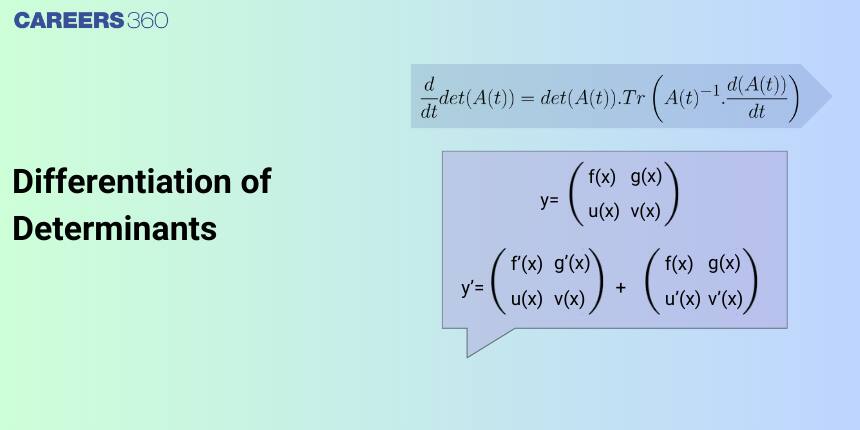
Differentiation of Determinants

To differentiate a determinant, we differentiate one row or column at a time, keeping the other row or column unchanged.

Consider a $2\times 2$ matrix,

$\mathrm{\Delta }=\left|\begin{array}{ll}{y}_{11}& y_{12}\\ y_{21}& y_{22}\end{array}\right|$, where $y_{ij}$ is a function of $x$ for all $i,j$

$\mathrm{\Delta }=y_{11}\cdot y_{22}-y_{12}\cdot y_{21}$

differentiating w.r.t. $x$ we get

$\begin{array}{rl}\mathrm{\Delta }& ={\left(y_{11}\right)}^{\prime }\cdot y_{22}+y_{11}\cdot {\left(y_{22}\right)}^{\prime }-{\left(y_{12}\right)}^{\prime }\cdot y_{21}-y_{12}\cdot {\left(y_{21}\right)}^{\prime }\\ & =[{\left(y_{11}\right)}^{\prime }\cdot y_{22}-{\left(y_{12}\right)}^{\prime }\cdot y_{21}]+[y_{11}\cdot {\left(y_{22}\right)}^{\prime }-y_{12}\cdot {\left(y_{21}\right)}^{\prime }]\\ & =\left|\begin{array}{cc}{\left(y_{11}\right)}^{\prime }& {\left(y_{12}\right)}^{\prime }\\ y_{21}& y_{22}\end{array}\right|+\left|\begin{array}{cc}{y}_{11}& y_{12}\\ {\left(y_{21}\right)}^{\prime }& {\left(y_{22}\right)}^{\prime }\end{array}\right|\end{array}$
Thus, for

$\mathrm{\Delta }=\left|\begin{array}{l}{R}_1\\ R_2\end{array}\right|,{\mathrm{\Delta }}^{\prime }=\left|\begin{array}{c}{\left(R_1\right)}^{\prime }\\ R_2\end{array}\right|+\left|\begin{array}{c}{R}_1\\ {\left(R_2\right)}^{\prime }\end{array}\right|$

We can also differentiate column-wise.
Similarly, if a three-order determinant is given,

$\begin{array}{rl}& \mathrm{\Delta }=\left|\begin{array}{lll}f(x)& g(x)& h(x)\\ p(x)& q(x)& r(x)\\ u(x)& v(x)& w(x)\end{array}\right|,\text{ then }\\ & {\mathrm{\Delta }}^{\prime }=\frac{d}{dx}(\mathrm{\Delta })=\left|\begin{array}{lll}{f}^{\prime }(x)& g^{\prime }(x)& h^{\prime }(x)\\ p(x)& q(x)& r(x)\\ u(x)& v(x)& w(x)\end{array}\right|+\left|\begin{array}{ccc}f(x)& g(x)& h(x)\\ p^{\prime }(x)& q^{\prime }(x)& r^{\prime }(x)\\ u(x)& v(x)& w(x)\end{array}\right|\\ & +\left|\begin{array}{ccc}f(x)& g(x)& h(x)\\ p(x)& q(x)& r(x)\\ u^{\prime }(x)& v^{\prime }(x)& w^{\prime }(x)\end{array}\right|\end{array}$

Also if $\mathrm{\Delta }=\left|\begin{array}{ccc}f(x)& g(x)& h(x)\\ a& b& c\\ p& q& r\end{array}\right|$, where, $\mathrm{a},\mathrm{b},\mathrm{c},\mathrm{p},\mathrm{q}$ and r are constant, then

${\mathrm{\Delta }}^{\prime }=\left|\begin{array}{ccc}{f}^{\prime }(x)& g^{\prime }(x)& h^{\prime }(x)\\ a& b& c\\ p& q& r\end{array}\right|$

and $\frac{d^n}{d{x}^n}(\mathrm{\Delta })=\left|\begin{array}{ccc}\frac{d^n}{dx}(f(x))& \frac{d^n}{dx}(g(x))& \frac{d^n}{dx}(h(x))\\ a& b& c\\ p& q& r\end{array}\right|$

Recommended Video Based on Differentiation of Determinant

Solved Examples Based on Differentiation of Determinant

Example 1: Let , $f(x)=\left|\begin{array}{ccc}\sin x& \cos x& 1\\ \cos x& -\sin x& 2\\ \sin x& \cos x& 3\end{array}\right|$ then $f^{\prime }(x)=$

1) $0$
2) $-1$
3) $1$
4) $2$

Solution:

Differentiating column-wise,

$f^{\prime }(x)=\left|\begin{array}{ccc}\cos x& \cos x& 1\\ -\sin x& -\sin x& 2\\ \cos x& \cos x& 3\end{array}\right|+\left|\begin{array}{ccc}\sin x& -\sin x& 1\\ \cos x& -\cos x& 2\\ \sin x& -\sin x& 3\end{array}\right|+\left|\begin{array}{ccc}\sin x& \cos x& 0\\ \cos x& -\sin x& 0\\ \sin x& \cos x& 0\end{array}\right|$

(1st and 2nd determinants are 0 as columns are proportional)

$\therefore f^{\prime }(x)=0+0+0=0$

Hence, the answer is the option 1.

Example 2: Let $f(x)={\left|\begin{array}{lll}{x}^3& 1& 3\\ x^4& 2& 4\\ x^2& 3& 2\end{array}\right|}_{\text{, then }}{f}^{\prime }(1)=$

1) $0$
2) $1$
3) $2$
4) $3$

Solution:

Differentiating column-wise,

$\begin{array}{rl}& f^{\prime }(x)=\left|\begin{array}{ccc}3x^2& 1& 3\\ 4x^3& 2& 4\\ 2x& 3& 2\end{array}\right|+\left|\begin{array}{lll}{x}^3& 0& 3\\ x^4& 0& 4\\ x^2& 0& 2\end{array}\right|+\left|\begin{array}{lll}{x}^3& 1& 0\\ x^4& 2& 0\\ x^2& 3& 0\end{array}\right|\\ & f^{\prime }(1)=\left|\begin{array}{lll}3& 1& 3\\ 4& 2& 4\\ 2& 3& 2\end{array}\right|+0+0\\ & =0+0+0(\because C_1=C_3)\\ & =0\end{array}$

Hence, the answer is the option 1.

Example 3: Let $\mathrm{f}(\mathrm{x})=\left|\begin{array}{ccc}a& -1& 0\\ ax& a& -1\\ a{x}^2& ax& a\end{array}\right|,\mathrm{a}\in \mathbb{R}$ . Then the sum of the squares of all the values of $a$, for which $2{\mathrm{f}}^{\prime }(10)-{\mathrm{f}}^{\prime }(5)+100=0$, is?

1) $117$
2) $106$
3) $125$
4) $136$

Solution:

$\begin{array}{rl}f(x)=a& \left|\begin{array}{ccc}1& -1& 0\\ x& a& -1\\ x^2& ax& a\end{array}\right|=a(1(a^2+ax)+1(ax+x^2)]\\ & =a{x}^2+2a^{2}x+a^3=a(x+a{)}^2.\\ & f^{\prime }(x)=2a(x+a)\\ & f^{\prime }(10)=2a(10+a)=2a^2+20a\\ & f^{\prime }(5)=2a(5+a)=2a^2+10a.\\ & \Rightarrow f^{\prime }(10)-f^{\prime }(5)+100=0\end{array}$

$\begin{array}{rl}& \Rightarrow (2a^2+20a)-2a^2-10a+100=0\\ & \Rightarrow 2a^2+30a+100=0\\ & \Rightarrow a^2+15a+50=0\\ & \Rightarrow a=-5,-10\\ & \text{ sum of squares }=5^2+{10}^2=125\end{array}$

Hence, the answer is the option (3).

Example 4: Let

$f(x)=\left|\begin{array}{ccc}{\sin }^{2}x& -2+{\cos }^{2}x& \cos 2x\\ 2+{\sin }^{2}x& {\cos }^{2}x& \cos 2x\\ {\sin }^{2}x& {\cos }^{2}x& 1+\cos 2x\end{array}\right|,x\in [0,\pi ]$

Then the maximum value of $f(x)$ is equal to $$

1) $6$
2) $4$
3) $2$
4) $1$

Solution:

$\begin{array}{rl}& R_2\to R_2-R_1\text{ and }{R}_3\to R_3-R_1\\ & \begin{array}{rl}f(x)& =\left|\begin{array}{ccc}{\sin }^{2}x& -2+{\cos }^{2}x& \cos 2x\\ 2& 2& 0\\ 0& 2& 1\end{array}\right|\\ & ={\sin }^{2}x(2-0)+(2-{\cos }^{2}x)(2)+\cos 2x(4)\\ & =2{\sin }^{2}x+4-2{\cos }^{2}x+4\cos 2x\\ & =4+2({\sin }^{2}x-{\cos }^{2}x)+4\cos 2x\\ & =4+2\cos 2x\\ \Rightarrow f^{\prime }(x)& =-2\cdot 2\sin 2x=0\\ & \Rightarrow \sin 2x=0\\ & \Rightarrow 2x=0,\pi ,2\pi \\ & \Rightarrow x=0,\frac{\pi }{2},\pi \end{array}\end{array}$
$\begin{array}{rl}& f(0)=4+2=6\\ & f\left(\frac{\pi }{2}\right)=4-2=2\\ & f(\pi )=4+2=6\end{array}$
$\text{ Maximum }=6$

Hence, the answer is (6).

Example 5: $f(x)=\left|\begin{array}{ccc}2{\cos }^{4}x& 2{\sin }^{4}x& 3+{\sin }^{2}2x\\ 3+2{\cos }^{4}x& 2{\sin }^{4}x& {\sin }^{2}2x\\ 2{\cos }^{4}x& 3+2{\sin }^{4}x& {\sin }^{2}2x\end{array}\right|$, then $\frac{1}{5}$ $f^{\prime }(0)$ is equal to

1) $0$
2) $1$
3) $2$
4) $6$

Solution:

$\begin{array}{rl}& f(x)=\left|\begin{array}{ccc}2{\cos }^{4}x& 2{\sin }^{4}x& 3+{\sin }^{2}2x\\ 3+2{\cos }^{4}x& 2{\sin }^{4}x& {\sin }^{2}2x\\ 2{\cos }^{4}x& 3+2{\sin }^{4}x& {\sin }^{2}2x\end{array}\right|\\ & {\mathrm{f}}^{\prime }(0)=0+0+0=0\\ & {\mathrm{f}}^{\prime }(0)=0\\ & \frac{1}{5}\cdot {\mathrm{f}}^{\prime }(0)=0\end{array}$

Hence, the answer is the option (1).

Summary

Differentiation of determinants is an important concept to monitor its behaviour. Knowing about determinants and their properties is very crucial as it helps us know the whether inverse of the matrix exists or not. It also helps us to find the value of determinants in simpler ways. The properties of determinants offer powerful tools in linear algebra for analyzing systems of equations, transformations, and geometric interpretations.