First Derivative Test - Examples, Steps, Applications

First Derivative Test is an important concept in calculus. It is useful in understanding the rate of a change in the function. The existence of a derivative at a point implies that the function has a specific rate of change at that point. These concepts of derivatives have been broadly applied in branches of mathematics, physics, engineering, economics, and biology.

JEE Main 2025: Sample Papers | Mock Tests | PYQs | Study Plan 100 Days

JEE Main 2025: Maths Formulas  | Study Materials

JEE Main 2025: Syllabus | Preparation Guide | High Scoring Topics 

This Story also Contains

1. First Derivative Test
2. First Derivative Test to Get Extrema
3. Discontinuous Function
4. Non-Differentiable Function
5. Global Maxima and Minima in $[a, b]$
6. Global Maxima and Minima in $(a, b)$
7. Solved Examples Based on First Derivative Test
8. Summary

This article is about the concept of first derivative test which falls under the broader category of Calculus, which is a crucial chapter in class 11. It is not only essential for board exams but also for competitive exams like the Joint Entrance Examination (JEE Main), and other entrance exams such as SRMJEE, BITSAT, WBJEE, BCECE, and more.

First Derivative Test

The first derivative test is used to test a function's monotonicity, focusing on a particular poin in its domain and the local extrema on a domain.

Monotonicity:

A function is said to be monotonic if it is either increasing or decreasing in its entire domain. By a monotonic function $f$ in an interval $I$, we mean that $f$ is either increasing in the Given domain or decreasing in a given domain.

Critical Points:

Extremes of a function always lie on the critical points only. A critical point is a point belonging to the domain of the function such that either the function is non-differentiable at this point or the derivative of function at this point is zero.

Hence, a function $f(x)$ has a critical point at $x=a$, if $f^{\prime}(a)=0$ or $f^{\prime}(a)$ is not defined.

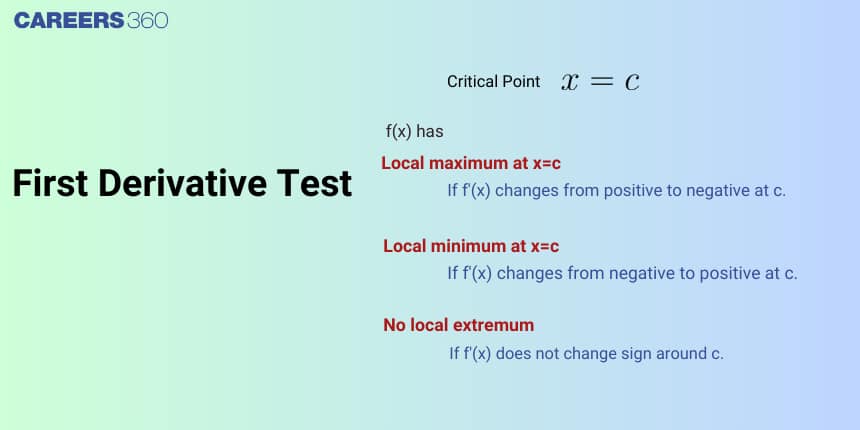
First Derivative Test to Get Extrema

(1) At critical point $x=a$

Let $y=f(x)$ be a differentiable function and $x=$ a be a critical point of $y=$ $\mathrm{f}(\mathrm{x})$.
1. If $f^{\prime}(x)$ changes from positive to negative at $x=a$, then $f$ has a Local Maxima at $\mathrm{x}=\mathrm{a}$.

2. If $f^{\prime}(x)$ changes from negative to positive at $x=a$, then $f$ has a Local Minima at $\mathrm{x}=\mathrm{a}$.

3. If $f^{\prime}(x)$ does not change any sign at $x=a$, then $f$ has neither Local maxima nor Local minima at $\mathrm{x}=\mathrm{a}$.

(2) At end points of the closed interval $[a, b]$
$f(x)$ is defined on the interval $[a, b]$ and if $f^{\prime}(x)<0$ for $x$ then $f(x)$ has a local maximum at $\mathrm{x}=\mathrm{a}$ and local minimum at $\mathrm{x}=\mathrm{b}$.

Again if $f^{\prime}(x)>0$ then $f(x)$ has a local minimum at $x=a$ and local maximum at $x=b$.

$\mathrm{n}^{\text {th }}$ derivative test

First find the value of $x$ such that $f^{\prime}(x)=0$, let at $x=a, f^{\prime}(x)=0$
Now, find $\mathrm{f}^{\prime \prime}(\mathrm{x})$ at $\mathrm{x}=\mathrm{a}$
1. If $f^{\prime \prime}(a)<0$, then $f(x)$ has local maximum at $x=a$
2. If $f^{\prime \prime}(a)>0$, then $f(x)$ has local minimum at $x=a$
3. If $f^{\prime \prime}(a)=0$

Then, find $f^{\prime \prime \prime}$ $(\mathrm{x})$ at $\mathrm{x}=\mathrm{a}$
If$f^{\prime \prime \prime}(a)$ $\neq 0$, then $f(x)$ has neither maximum nor minimum (inflection point) at $x=a$.

But, if $f^{\prime \prime \prime}(a)=0$, then find fourth derivative of $f(x)$ at $x=$ a, i.e. $f^{\text {iv }}(x)$ at $x=$ a.

If $f^{\text {iv }}(a)<0$, then $f(x)$ is maximum at $x=a$ and if $f^{\text {iv }}(a)>0$ then $f(x)$ is minimum at $\mathrm{x}=\mathrm{a}$ and so on.

Discontinuous Function

If function $f(x)$ is discontinuous at $x=a$ with $f(a)$ exists finitely

In this case, we use the most common definition of minima and maxima
I.e. if $f(a)>f(a-h)$ and $f(a)>f(a+h)$, then $x=a$ is the point of maxima and if $f(a)<f(a-h)$ and $f(a)<f(a+h)$, then $x=a$ is point of minima.

We can also use the graph of the function to decide the point of maxima or minima for such a function.

Non-Differentiable Function

When the function $f(x)$ is continuous at $x=a$ but $f(x)$ is not differentiable at $x=a$

In this case, we can check the change in the sign of the derivative in the neighborhood of $x=a$
i.e. if $f^{\prime}(a-h)>0$ and $f^{\prime}(a+h)<0$, then $x=a$ is point of maxima And if $f^{\prime}(a-h)<0$ and $f^{\prime}(a+h)>0$, then $x=a$ is the point of minima.

Global Maxima and Minima in $[a, b]$

Let $y=f(x)$ be a given function in the domain $D$ and let $[a, b] \subseteq D$. Then, the global maxima and minima of a function $f(x)$ in the closed interval $[a, b]$ are the greatest and least value of $f(x)$ in $[a, b]$ respectively.

Global maxima and minima in $[a, b]$ would always occur at critical points of the function $f(x)$ within the $[a, b]$ or at the endpoints of the interval.

First of all, we shall find out all the critical points of $f(x)$ on $(a, b)$ i.e. points where $f^{\prime}(x)=0$ or where $f(x)$ is non-differentiable or discontinuous.

Let $c_1, c_2, \ldots c_n$ are the critical points of $f(x)$ then we shall find the value of the function for all those critical points.

Let $f\left(c_1\right), f\left(c_2\right), \ldots, f\left(c_n\right)$ be the values of the function at critical points.
Let $M_1=\max \left\{f(a), f\left(c_1\right), f\left(c_2\right), \ldots, f\left(c_n\right), f(b)\right\}$ and

$
M_2=\min \left\{f(a), f\left(c_1\right), f\left(c_2\right), \ldots, f\left(c_n\right), f(b)\right\}
$

Then $\mathrm{M}_1$ is the Global Maximum or Absolute Maximum or the
Greatest value of the function and
$\mathrm{M}_2$ is the Global Minimum or Absolute Minimum or the Least value of the function.

Global Maxima and Minima in $(a, b)$

Let us consider a function $f$ given by $f(x)=x+2, x \in(0,1)$ Observe that the function is continuous on $(0,1)$ and neither has a maximum value nor a minimum value. Further, we may note that the function even has neither a local maximum value nor a local minimum value. However, if we extend the domain of $f$ to the closed interval $[0,1]$, then $f$ still may not have local maximum (minimum) values but it certainly does have maximum value $3=f(1)$ and minimum value $2=f(0)$. The maximum value $3$ of $f$ at $x=1$ is called the absolute maximum value (global maximum or greatest value) of $f$ on the interval $[0,1]$. Similarly, the minimum value $2$ of $f$ at $x=0$ is called the absolute minimum value (global minimum or least value) of $f$ on $[0,1]$.

Consider the graph given in Figure, of a continuous function defined on a closed interval $[a, d]$. Observe that the function $f$ has a local minimum at $x$ $=\mathrm{b}$ and the local minimum value is $\mathrm{f}(\mathrm{b})$. The function also has a local maxima at $x=c$ and the local maximum value is $f(c)$.
Also from the graph, it is evident that $f$ has an absolute maximum value $f (a)$ and an absolute minimum value $f (d)$. Further, note that the absolute maximum (minimum) value of $f$ is different from local maximum (minimum) value of $f$.

The method for obtaining the greatest and least values of $f(x)$ in $(a, b)$ is almost the same as the method used for obtaining the greatest and least values in $[a, b]$ however with caution.

Step 1

We do not take $f(a)$ and $f(b)$ into consideration in first step
Let $M_1=\max \left\{f\left(c_1\right), f\left(c_2\right), \ldots, f\left(c_n\right)\right\}$ and $M_2=\min \left\{f\left(c_1\right), f\left(c_2\right), \ldots, f\left(c_n\right)\right\}$

Step 2

If, $\quad \lim _{x \rightarrow a^{+}} f(x)>M_1$ or $\lim _{x \rightarrow b^{-}} f(x)>M_1$

Then $\mathrm{f}(\mathrm{x})$ does not possess global maxima
If none of these two is true then $M1$ is Global Maxima

And if,

$
\lim _{x \rightarrow a^{+}} f(x)<M_2 \text { or } \lim _{x \rightarrow b^{-}} f(x)<M_2
$

Then $f(x)$ does not possess global minima
If none of these two conditions are satisfied, then $M2$ is the global minima

For example,

This function has no global maxima and no global minima.

As a recap,

$\begin{array}{c ccc } \hline & \begin{array}{c}{\text { First order }} \\ {\text { derivative test }}\end{array}& \begin{array}{c}{\text { Second order }} \\ {\text { derivative test }}\end{array}&\begin{array}{c}{\text { Higher order }} \\ {\text { derivative test }}\end{array} \\ \hline\hline\\\text{Max}& \begin{array}{l}{f^{\prime}(a)=0} \\ {f^{\prime}(x) \text { changes }} \\ {\text { sign from }+\text { ve to }} \\ {\text { -ve as } x \text { crosses } a}\end{array} & \begin{array}{l}{f^{\prime}(a)=0} \\ {f^{\prime \prime}(a)<0}\end{array} & \begin{array}{c}{f^{\prime}(a)=0} \\ {f^{\prime}(a)=0} \\ {\vdots} \\ {f^{n-1}(a)=0} \\ {f^{n}(a)<0} \\ {\text { where } n \text { is even }} \\ {\text { (If } n \text { is odd, } x=a \text { is not }} \\ {\text { an extremum point; it is }} \\ {\text { a point of inflection) }}\end{array} \\ \\ \hline \end{array}$

$\begin{array}{c ccc } \hline\\\text{Min}& \begin{array}{l}{f^{\prime}(a)=0} \\ {f^{\prime}(x) \text { changes }} \\ {\text { sign from }-\text { ve to }} \\ {\text { +ve as } x \text { crosses } a}\end{array} & \begin{array}{l}{f^{\prime}(a)=0} \\ {f^{\prime \prime}(a)>0}\end{array} & \begin{array}{c}{f^{\prime}(a)=0} \\ {f^{\prime}(a)=0} \\ {\vdots} \\ {f^{n-1}(a)=0} \\ {f^{n}(a)>0} \\ {\text { where } n \text { is even }} \\ {\text { (If } n \text { is odd, } x=a \text { is not }} \\ {\text { an extremum point; it is }} \\ {\text { a point of inflection) }}\end{array} \\ \\ \hline \\ &\text{point of inflection}&&\begin{array}{l}{f^{\prime \prime}(x) \text { change sign }} \\ {\text { at } x=a}\end{array}\\\\\hline \end{array}$

Recommended Video Based on First Derivative Test

Solved Examples Based on First Derivative Test

Example 1: If the function $f$ given by $f(x)=x^3-3(a-2) x^2+3 a x+7$, for some $a \in R$ is increasing in $(0,1]$ and decreasing in $[1,5)$, then one root of the equation, $\frac{f(x)-14}{(x-1)^2}=0(x \neq 1)$ is:
1) $7$
2) $6$
3) $5$
4) $-7$

Solution
$
\begin{aligned}
& f^{\prime}(x)=3\left(x^2-2(a-2) x+a\right) \\
& f^{\prime}(1)=0 \\
& \Rightarrow a=5 \\
& \frac{f(x)-14}{(x-1)^2}=\frac{x^3-a x^2+15 x-7}{(x-1)^2}=x-7=0 \\
& \Rightarrow x=7
\end{aligned}
$

Hence, the answer is the option 1.

Example 2: The maximum value of the term independent of ' $t$ ' in the expansion of $\left(t x^{\frac{1}{5}}+\frac{(1-x)^{\frac{1}{10}}}{t}\right)^{10}$ where $x \in(0,1)$ is :
1) $
\frac{2.10!}{3 \sqrt{3}(5!)^2}
$

2) $
\frac{10!}{3(5!)^2}
$

3) $
\frac{10!}{\sqrt{3}(5!)^2}
$

4) $
\frac{2.10!}{3(5!)^2}
$

Solution

The term independent of $t$ will be the middle term due to exact same magnitude but opposite sign powers of $t$ in the binomial expression given

$
\begin{aligned}
& \text { so } \mathrm{T}_6={ }^{10} \mathrm{C}_5\left(\mathrm{tx}^{\frac{1}{5}}\right)^5\left(\frac{(1-\mathrm{x})^{\frac{1}{10}}}{\mathrm{t}}\right)^5 \\
& T_6=f(x)={ }^{10} \mathrm{C}_5(\mathrm{x} \sqrt{1-\mathrm{x}}) \\
& f^{\prime}(x)={ }^{10} C_5\left(\sqrt{1-x}-\frac{x}{2 \sqrt{1-x}}\right)={ }^{10} C_5\left(-\frac{3 x-2}{2 \sqrt{1-x}}\right)
\end{aligned}
$

for maximum $f^{\prime}(x)=0$

$
\begin{aligned}
& \Rightarrow x=\frac{2}{3} \\
& f^{\prime \prime}(x)=\frac{2-3 x}{4(1-x)^{\frac{3}{2}}}-\frac{3}{2 \sqrt{1-x}}=\frac{3 x-4}{4(1-x)^{\frac{3}{2}}} \\
& f^{\prime \prime}\left(\frac{2}{3}\right)<0
\end{aligned}
$

so $f(x)_{\max }={ }^{10} C_5\left(\frac{2}{3}\right) \cdot \frac{1}{\sqrt{3}}$
Hence, the answer is the option 1 .

Example 3: The function $f$ defined by $f(x)=x^3-3 x^2+5 x+7$ is: [JEE Main 2017]

1) increasing in $R$.

2) decreasing in $R$.

3) decreasing in $(0, ∞)$ and increasing in $(−∞, 0)$.

4) increasing in $(0, ∞)$ and decreasing in $(−∞, 0)$.

Solution

First Derivative Test to Get Extrema

First find the value of $x$ such that $f^{\prime}(x)=0$, let at $x=a, f^{\prime}(x)=0$

Now, find $f^{\prime}(x)$ at $x=a$.
1. If $f^{\prime}(a)<0$, then $f(x)$ is maximum at $x=a$
2. If $f^{\prime}(a)>0$, then $f(x)$ id minimum at $x=a$
3. If $f^{\prime}(a)=a$

Then, find $f^{\prime \prime \prime}(x)$ at $x=a$.
If $f^{\prime \prime \prime}(a) \neq 0$, then $f(x)$ has neither maximum nor minimum (inflection point) at $x=a$.

But, if $f^{\prime \prime}(a)=0$, then find fourth derivative of $f(x)$ at $x=a$, i.e. $f^{\text {iv }}(x)$ at $x=$ a.

If $f^{\text {iv }}(a)<0$, then $f(x)$ is maximum at $x=a$, and if $f^{\text {iv }}(a)>0$ then $f(x)$ is minimum at $\mathrm{x}=\mathrm{a}$, and so on.

$
\begin{aligned}
& f(x)=x^3-3 x^2+5 x+7 \\
& f^{\prime}(x)=3 x^2-6 x+5 \\
& D=(6)^2-4 \cdot 3 \cdot 5 \\
& =36-60<0
\end{aligned}
$

So,

$
f^{\prime}(x)>0 \quad \text { for } x \in R
$

Hence, the answer is the option 1.

Example 4: Let $
f(x)=\frac{x}{\sqrt{a^2+x^2}}-\frac{d-x}{\sqrt{b^2+(d-x)^2}}, x \in R
$

where $\mathrm{a}, \mathrm{b}$ and d are non-zero real constants. Then :[JEE Main 2019]
1) $f$ is neither increasing nor decreasing function of $x$
2) $\mathrm{f}^{\prime}$ is not a continuous function of $x$
3) $f$ is a decreasing function of $x$
4) $f$ is an increasing function of $x$

Solution

First Derivative Test to Get Extrema

First find the value of $x$ such that $f(x)=0$, let at $x=a, f(x)=0$

Now, find $f^{\prime}(x)$ at $x=a$.
1. If $f^{\prime}(a)<0$, then $f(x)$ is maximum at $x=a$
2. If $f^{\prime}(a)>0$, then $f(x)$ id minimum at $x=a$
3. If $f^{\prime}(a)=a$

Then, find $\mathrm{f}^{\prime \prime}(\mathrm{x})$ at $\mathrm{x}=\mathrm{a}$.
If $f^{\prime \prime \prime}(a) \neq 0$, then $f(x)$ has neither maximum nor minimum (inflection point) at $x=a$.

But, if $f^{\prime \prime \prime}(a)=0$, then find fourth derivative of $f(x)$ at $x=a$, i.e. $f^{\text {iv }}(x)$ at $x=$ a.

If $f^{\text {iv }}(a)<0$, then $f(x)$ is maximum at $x=a$ and if $f^{\mathrm{iv}}(a)>0$ then $f(x)$ is minimum at $x=a$ and so on.

$
f^{\prime}(x)=\frac{a^2}{\left(a^2+x^2\right)^{\frac{3}{2}}}+\frac{b^2}{\left(b^2+\left(d-x^2\right)\right)^{\frac{3}{2}}}>0 \vee x \varepsilon R
$

$f(x)$ is increasing function.
Hence, the answer is the option 4.

Example 5: Let $f:[0,2] \rightarrow \mathbb{R}$ be a twice differentiable function such that $f^{\prime \prime}(x)>0$, for all $x \in(0,2)$. If $\phi(x)=f(x)+f(2-x)$, then $\phi$ is: [JEE Main 2019]
1) decreasing on $(0,1)$ and increasing on $(1,2)$.
2) increasing on $(0,2)$.
3 ) increasing on $(0,1)$ and decreasing on $(1,2)$.
4) decreasing on $(0,2)$.

Solution

First Derivative Test to Get Extrema:

First find the value of $x$ such that $f(x)=0$, let at $x=a, f(x)=0$

Now, find $f^{\prime}(x)$ at $x=a$.
1. If $f^{\prime}(a)<0$, then $f(x)$ is maximum at $x=a$
2. If $f^{\prime}(a)>0$, then $f(x)$ id minimum at $x=a$
3. If $f^{\prime}(a)=a$

Then, find $\mathrm{f}^{\prime \prime}(\mathrm{x})$ at $\mathrm{x}=\mathrm{a}$.
If $f^{\prime \prime \prime}(a) \neq 0$, then $f(x)$ has neither maximum nor minimum (inflection point) at $x=a$.

But, if $f^{\prime \prime}(a)=0$, then find the fourth derivative of $f(x)$ at $x=a$, i.e. $f^{i v}(x)$ at $x$ $=\mathrm{a}$.

If $f^{\text {iv }}(a)<0$, then $f(x)$ is maximum at $x=a$, and if $f^{\text {iv }}(a)>0$ then $f(x)$ is minimum at $x=a$ and so on.

$f:[0,2] \rightarrow \mathbb{R}_{\text {twice differentiable }}$
Such that. $f^{\prime \prime}(x)>0 \quad \vee \quad x \epsilon(0,2)$
If $\phi(x)=f(x)+f(2-x)$ then $\phi$
for $\phi(x)$ to be increasing $\phi^1(x)>0$
$\phi^1(x)=f^1(x)-f^1(2-x)$
Since $f^{\prime \prime}(x)>0 \Rightarrow f^1(x)_{\text {in increasing }}$
So for $x \epsilon(0,1)$
$f^1(x)-f^1(2-x)<0$
hence $\phi^1(x)<0$
and for $x \epsilon(1,2)$
$f^1(x)-f^1(2-x)>0$
$\Rightarrow \phi^1(x)>0$
Hence, $\phi(a)$ is increasing.
Hence, the answer is the option 1.

Summary

Differentiation is an important concept of Calculus. Differentiation of a function at a point represents the slope of the tangent to the graph of the function at that point. With the help of differentiation, we can find the rate of change of one quantity for another. The concept of differentiation is the cornerstone on which the development of calculus rests. First derivative test is used to know about the monotonicy and the extreme points of the function.

FAQs:

1) What is derivative?

Solution: The rate of change of a quantity $y$ concerning another quantity x is called the derivative or differential coefficient of $y$ concerning $x$.

2) When to use first derivative test?

Solution: First derivative test are used to determine the critical points of the function.

3) What is a critical point?

Solution: Extremes of a function always lie on the critical points only.

4) Where do extremes of functions lie?

Solution: A critical point is a point belonging to the domain of the function such that either the function is non-differentiable at this point or the derivative of the function at this point is zero.

5) What is Global Maximum?

Solution: Global Maximum also known as Absolute Maximum, is the largest value in the function.