Integration by Partial Fractions - Definition, Formulas, Steps and Examples

Integration Using Partial Fractions is one of the important parts of Calculus, which applies to measuring the change in the function at a certain point. Mathematically, it forms a powerful tool by which slopes of functions are determined, the maximum and minimum of functions found, and problems on motion, growth, and decay, to name a few. These integration concepts have been broadly applied in branches of mathematics, physics, engineering, economics, and biology.

JEE Main 2025: Sample Papers | Mock Tests | PYQs | Study Plan 100 Days

JEE Main 2025: Maths Formulas  | Study Materials

JEE Main 2025: Syllabus | Preparation Guide | High Scoring Topics 

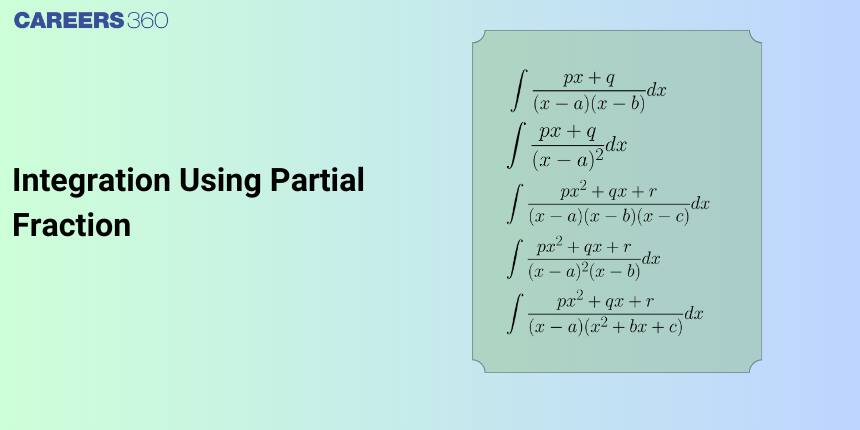
In this article, we will cover the concept of Integration Using Partial Fractions. This concept falls under the broader category of Calculus, a crucial Chapter in class 12 Mathematics. It is not only essential for board exams but also for competitive exams like the Joint Entrance Examination (JEE Main), and other entrance exams such as SRMJEE, BITSAT, WBJEE, BCECE, and more. Over the last ten years of the JEE Main exam (from 2013 to 2023), three questions have been asked on this concept, including one in 2017, one in 2021, and one in 2023.

Integration Using Partial Fraction

Integration is the reverse process of differentiation. In integration, we find the function whose differential coefficient is given. The rate of change of a quantity y concerning another quantity x is called the derivative or differential coefficient of y concerning x. Geometrically, the Differentiation of a function at a point represents the slope of the tangent to the graph of the function at that point.

A rational function is of the form P(x) / Q(x), where P(x) and Q(x) are polynomial function and Q(x) ≠ 0.

Partial fraction method is used to integrate rational functions.

Expression $\frac{P(x)}{Q(x)}$ can be decomposed into simpler rational expression that we can add or subtract to get the original rational function. This process is called partial fraction decomposition.

Partial fraction decomposition can be applied to a rational function $\frac{P(x)}{Q(x)}$ only if $\operatorname{deg}(\mathrm{P}(\mathrm{x}))<\operatorname{deg}(\mathrm{Q}(\mathrm{x}))$.

Illustration on how to approach to integrals of rational functions of the form $\int \frac{P(x)}{Q(x)} d x$ where, $\operatorname{deg}(\mathrm{P}(\mathrm{x})) \geq \operatorname{deg}(\mathrm{Q}(\mathrm{x}))$

$\int \frac{x^2+3 x+5}{x+1} d x$

since $\operatorname{deg}\left(x^2+3 x+5\right) \geq \operatorname{deg}(x+1)$, we perform long division to obtain

$\begin{aligned}
& \frac{x^2+3 x+5}{x+1}=x+2+\frac{3}{x+1} \\
& \begin{aligned}
\int \frac{x^2+3 x+5}{x+1} d x & =\int_{-}\left(x+2+\frac{3}{x+1}\right) d x \\
& =\frac{1}{2} x^2+2 x+3 \ln |x+1|+C
\end{aligned}
\end{aligned}$

If the rational function $\frac{P(x)}{Q(x)}$ is proper (i.e. $\operatorname{deg}(\mathrm{P}(\mathrm{x}))<\operatorname{deg}(\mathrm{Q}(\mathrm{x})$ )), then three cases arises

Case 1:
When denominator $(Q(x))$ is expressed as the product of the non-repeated linear factor
$\mathrm{Q}(\mathrm{x})$ can be factored as $\left(x-a_1\right)\left(x-a_2\right)\left(x-a_3\right) \ldots\left(x-a_n\right)$
then, we have

$\frac{P(x)}{Q(x)}=\frac{A_1}{\left(x-a_1\right)}+\frac{A_2}{\left(x-a_2\right)}+\cdots+\frac{A_n}{\left(x-a_n\right)}$

where, $\mathrm{A}_1, \mathrm{~A}_2, \mathrm{~A}_3, \ldots \ldots, \mathrm{A}_{\mathrm{n}}$ are constants and can be determined by equating the numerator on RHS to numerator on LHS and then substituting

$\mathrm{x}=\mathrm{a}_1, \mathrm{a}_2, \mathrm{a}_3 \ldots, \mathrm{a}_n$
Short cut method
Consider $x-a_1=0$ then, $x=a_1$, put his value of x in all the expression other than $x-a_1$ and so on for example :

$\frac{x^2+2}{x(x-1)(x-2)}=\frac{0+2}{x(0-1)(0-2)}+\frac{1+2}{1(x-1)(1-2)}+\frac{4+2}{2(2-1)(x-2)}$

Illustration 1: Evaluate $\int \frac{3 x+2}{x^3-x^2-2 x} d x$
since $\operatorname{deg}(3 x+2)<\operatorname{deg}\left(x^3-x^2-2 x\right)$, we begin by factoring the denominator of

$\frac{3 x+2}{x^3-x^2-2 x} \quad \frac{3 x+2}{x(x-2)(x+1)}=\frac{A}{x}+\frac{B}{x-2}+\frac{C}{x+1}$

We need to determine $A, B$ and $C$. Cross multiplying in the expression above, we obtain:

$3 x+2=A(x-2)(x+1)+B x(x+1)+C x(x-2)$

A, B, C can now be determined by

Option 1 : comparing coefficients of different powers of x and the constant terms on both sides.

Option 2: Since the relation that we just obtained should held true for all x, we substitute those values of x that would straight way give us the required values of A, B and C. These values are obviously the roots of Q(x).

$\begin{aligned}
\mathrm{x}=0 & \Rightarrow 2=\mathrm{A}(0-2)(0+1)+\mathrm{B}(0)+\mathrm{C}(0) \\
& \Rightarrow \mathrm{A}=-1 \\
\mathrm{x}=2 & \Rightarrow 8=\mathrm{A}(0)+\mathrm{B}(2)(2+1)+\mathrm{C}(0) \\
& \Rightarrow \mathrm{B}=\frac{4}{3} \\
\mathrm{x}=-1 & \Rightarrow-1=\mathrm{A}(0)+\mathrm{B}(0)+\mathrm{C}(-1)(-1-2) \\
& \Rightarrow \mathrm{C}=-\frac{1}{3}
\end{aligned}
$$

Now that we have the values of $\mathrm{A}, \mathrm{B}$, and C , we rewrite the original integral:

$\int \frac{3 x+2}{x^3-x^2-2 x} d x=\int\left(-\frac{1}{x}+\frac{4}{3} \cdot \frac{1}{(x-2)}-\frac{1}{3} \cdot \frac{1}{(x+1)}\right) d x$

Evaluating the integral gives us

$\int \frac{3 x+2}{x^3-x^2-2 x} d x=-\ln |x|+\frac{4}{3} \ln |x-2|-\frac{1}{3} \ln |x+1|+C$

Case 2:

When denominator (Q(x)) is expressed as the product of the linear factor such that some of them are repeating (Linear and repeated)

$\mathrm{Q}(\mathrm{x})$ can be factored as $(x-a)^k\left(x-a_1\right)\left(x-a_2\right)\left(x-a_3\right) \ldots . .\left(x-a_n\right)$ then, we have

$\frac{P(x)}{Q(x)}=\frac{A}{(x-a)}+\frac{A}{(x-a)^2}+\ldots+\frac{A^k}{(x-a)^k}+\frac{B_1}{\left(x-a_1\right)}+\frac{B_2}{\left(x-a_2\right)}+\cdots+\frac{B_n}{\left(x-a_n\right)}$

Case 3:

When some of the factors in denominator $(Q(x))$ are quadratic but non-repeating
Corresponding to each quadratic factor $a x^2+b x+c$, assume the partial fraction of the type

$\frac{A x+B}{a x^2+b x+c}$
of mass.

Recommended Video Based on Integration using Partial Function

Solved Examples Based On Integration using Partial Function:
Example 1: Integrate $\int \frac{x^3-x}{x^2+1} d x$

$1)^{x+\ln \left(x^2+1\right)+C}$

2) $\frac{x^2}{2}+\ln \left(x^2+1\right)+C$
3) $x-\ln \left(x^2+1\right)+C$
4) $\frac{x^2}{2}-\ln \left(x^2+1\right)+C$

Solution

$\begin{aligned}
& \int \frac{x\left(x^2-1\right) d x}{x^2+1} \\
& \begin{aligned}
\Rightarrow \int \frac{x\left(x^2+1\right)-2 x}{x^2+1} d x & =\int x d x-\int \frac{2 x}{x^2+1} d x \\
& =\frac{x^2}{2}-\ln \left(x^2+1\right)+C
\end{aligned}
\end{aligned}$

Hence, the answer is the option (4).

Example 2:Integrate $\int \frac{x^3+x^3+x}{x^2+1} d x$
1) $\frac{x^3}{3}+\ln \left(x^2+1\right)+C$
2) $\frac{x^4}{3}+\ln \left(x^2+1\right)+C$
3) $\frac{x^4}{4}+\frac{1}{2} \ln \left(x^2+1\right)+C$
4) none of these

Solution

$\begin{aligned}
\int \frac{x^5+x^3+x}{x^2+1} d x= & \int \frac{x^3\left(x^2+1\right)}{x^2+1} d x+\int \frac{x}{x^2+1} d x \\
& =\frac{x^4}{4}+\frac{1}{2} \ln \left(x^2+1\right)+C
\end{aligned}$

Hence, the answer is the option (3).

Example 4: If $f\left(\frac{3 x-4}{3 x+4}\right)=x+2, x \neq-\frac{4}{3}$ and $\int f(x) d x=A \log |1-x|+B x+C$ then the ordered pair (A, B ) is equal to: (where C is a constant of integration)
1) $\left(\frac{8}{3}, \frac{2}{3}\right)$
2) $\left(-\frac{8}{3}, \frac{2}{3}\right)$
3) $\left(-\frac{8}{3},-\frac{2}{3}\right)$
4) $\left(\frac{8}{3},-\frac{2}{3}\right)$

Solution

As learned in the concept

Rule of integration by Partial fraction -

Linear and non-repeated:

$\begin{aligned}
& \frac{P(x)}{Q(x)}=\frac{P(x)}{\left(x-\alpha_1\right)\left(x-\alpha_2\right) \cdots\left(x-\alpha_n\right)} \\
& \text { Let } \frac{P(x)}{Q(x)}=\frac{A}{\left(x-\alpha_1\right)}+\frac{B}{\left(x-\alpha_2\right)} \cdots
\end{aligned}$

Find $A, B \ldots$
By comparing $N^r$ and $P(x)$

$f\left(\frac{3 x-4}{3 x+4}\right)=x+2$

Put $\frac{3 x-4}{3 x+4}=y$

$\begin{aligned}
& =>3 x y+4 y=3 x-4 \\
& x=\frac{-4(y+1)}{3(y-1)} \\
& x=\frac{4(1+y)}{3(1-y)} \\
& f(y)=\frac{4(1+y)}{3(1-y)}+2 \\
& =\frac{10-2 y}{3(1-y)}
\end{aligned}$

$\begin{aligned}
& \therefore f(x)=\frac{2(5-x)}{3(1-x)} \\
& \therefore \frac{2}{3} \int\left(\frac{5-x}{1-x}\right) d x=\frac{2}{3} \int \frac{1-x+4}{(1-x)} d x \\
& =\frac{2}{3} x+\frac{8}{3} \ln |1-x|+C
\end{aligned}$

So, $A=8 / 3$ and $B=2 / 3$
Hence, the answer is the option 1.
Example 4: Find $\int \frac{6}{(x-1)(x+1)} d x$ :
1) $3 \ln (x+1)(x-1)+C$
2) $3 \ln (x+1) /(x-1)+C$
3) $3 \ln (x-1)-3 \ln (x+1)+C$

4) none of these

Solution

As we have learned

Rule of integration by Partial fraction -

Linear and non-repeated:
$
\begin{aligned}
& \frac{P(x)}{Q(x)}=\frac{P(x)}{\left(x-\alpha_1\right)\left(x-\alpha_2\right) \cdots\left(x-\alpha_n\right)} \\
& \text { Let } \frac{P(x)}{Q(x)}=\frac{A}{\left(x-\alpha_1\right)}+\frac{B}{\left(x-\alpha_2\right)} \cdots
\end{aligned}
$

Find $A, B$...
By comparing $N^x$ and $P(x)$

$I=\int \frac{6 d x}{(x-1)(x+1)}=\int\left(\frac{A}{(x-1)}+\frac{B}{(x+1)}\right) d x$

Thus $6=A(x+1)+B(x-1)$
On calculating $A=3, B=-3$
Thus $I=3 \ln (x-1)-3 \ln (x+1)+C$
Hence, the answer is the option (3).

Example 5: Find $\int \frac{d x}{x^2-4}$ :
1) $\ln \frac{x+2}{x-2}+C$
2) $1 / 2 \ln \frac{x-2}{x+2}+C$
3) $\frac{1}{4} \ln \left|\frac{x-2}{x+2}\right|+C$

4) none of these

Solution:

As we have learned

Rule of integration by Partial fraction -

Linear and non-repeated:
$\frac{P(x)}{Q(x)}=\frac{P(x)}{\left(x-\alpha_1\right)\left(x-\alpha_2\right) \cdots\left(x-\alpha_n\right)}$
Let $\frac{P(x)}{Q(x)}=\frac{A}{\left(x-\alpha_1\right)}+\frac{B}{\left(x-\alpha_2\right)} \cdots$
Find $A, B$...
By comparing $N^x$ and $P(x)$

$\begin{aligned}
& I=\int \frac{d x}{x^2-4}=\int \frac{d x}{(x-2)(x+2)} \\
& =-\frac{\ln (|x+2|)-\ln (|x-2|)}{4}+C \\
& =\frac{1}{4} \ln \left|\frac{x-2}{x+2}\right|+C
\end{aligned}$
Hence, the answer is the option (3).