Inverse Trigonometric Functions

Q: What is the value of x can be if sin(x) = 3 ?

First of all, to find the value of x we will inverse the equation in the form of sine of inverse x = sin⁻¹(3) this is not possible because the value of x in sine inverse can be between -1 and 1

Q: What is the weightage of inverse trigonometric function in jee mains

The total weightage of inverse trigonometric function is two percentage

Q: Is it important to study class 12 chapter two inverse trigonometric function?

Yes, this is a very essential topic because if you don't understand the ideas in this chapter, you won't be able to answer derivative and integration issues in this chapter.

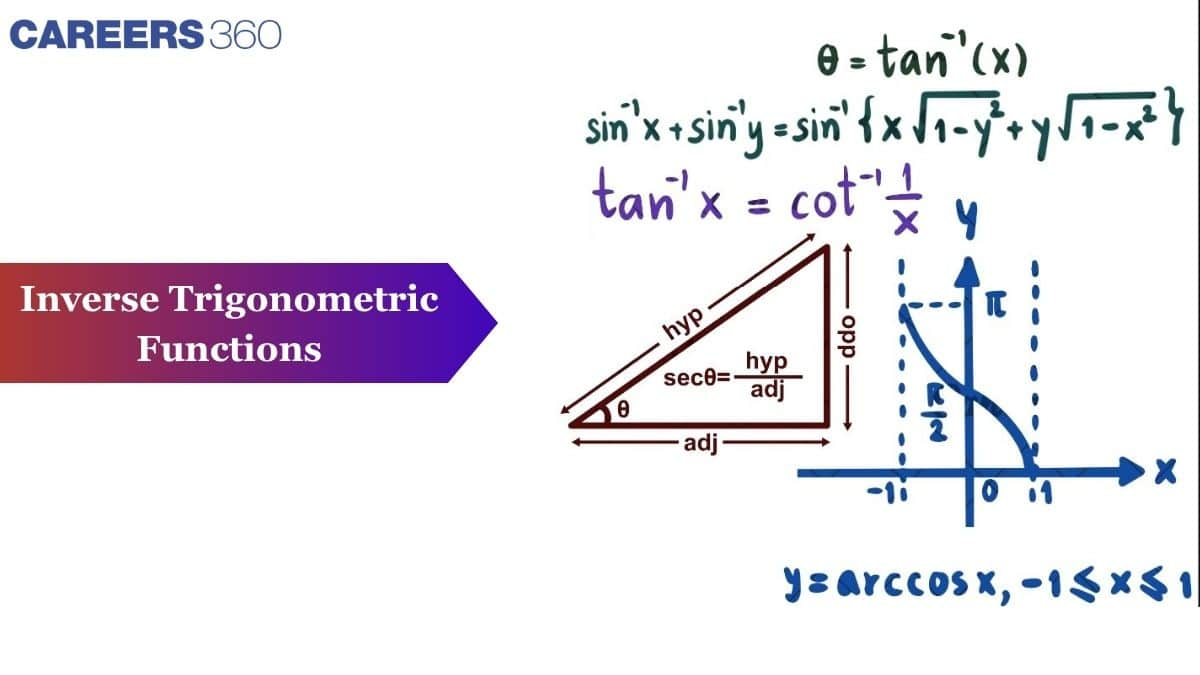
Q: What are the additional identities of inverse trigonometric functions?

sin⁻¹(x) + cos⁻¹(x) = ?2 tan⁻¹(x) + cot⁻¹(x) = ?2 cosec⁻¹(x) + sec⁻¹(x) = ?2

Q: What will happen if a same inverse trigonometric function is inside a inverse trigonometric function sin⁻¹(sin⁻¹(x)) like that ?

sin⁻¹(sin⁻¹(x)) is equal to x and this true for all the inverse trigonometric function sin⁻¹(sin⁻¹(x)) = x cos⁻¹(cos⁻¹(x))= x tan⁻¹(tan⁻¹(x))=x cot⁻¹(cot⁻¹(x))=x cosec⁻¹(cosec⁻¹(x))=x sec⁻¹(sec⁻¹(x))= x

Inverse Trigonometric Functions

Edited By Team Careers360 | Updated on Aug 14, 2023 04:44 PM IST

Introduction:
Class 12 Mathematics chapter Inverse Trigonometric functions dealing with inverse of trigonometric ratios, domain, range of inverse trigonometric functions have weightage not only in class 12th boards but its concept is used in JEE and other competitive exams. So the basic concepts should be clear and students should get a sufficient amount of practice for each class 12 maths inverse trigonometry solution through problem-solving. Inverse trigonometric functions' features aid in proving a distinct link between trigonometric entities such as sin x, cos x, tan x, cosec x, sec x, and cot x. Within the primary branches of the inverse trigonometric functions, the conclusions derived using these characteristics are valid. in the chapter, we have come across some of the basic formulas and we have also come to know about the domain and range of inverse trigonometric function The domain of a function refers to the range of values that may be plugged into it. This is the set of x values in a function like f(x). A function's range is the set of values that the function can take. This is the collection of values that the function produces when we enter x values. The y values are what you're looking for.

JEE Main 2025: Sample Papers | Mock Tests | PYQs | Study Plan 100 Days

JEE Main 2025: Maths Formulas | Study Materials

JEE Main 2025: Syllabus | Preparation Guide | High Scoring Topics

List of topics according to NCERT and JEE Main/NEET syllabus:

Basic Inverse trigonometric formulas
Domain and range of inverse trigonometric function
Addition identities of inverse trigonometric function
Addition identities of inverse trigonometric function
Other identities of inverse trigonometric function
Graphical representation of inverse trigonometric function
Derivative of inverse trigonometric function
Properties of inverse trigonometric function

Important concepts and Laws:

There are six basic diagrams of inverse trigonometric function which are based on how the inverse function will react when a negative input is inserted.

The first formula is on inverse of sine. What will happen if the input in the inverse sine function is negative.

sin⁻¹(-x) = -sin⁻¹(x)

Here, x belongs from -1 to 1

The second formula is on inverse of cosine. What will happen if the input in the inverse cosine function is negative.

cos⁻¹(-x) = π - cos⁻¹ (x)

Here, x belongs from -1 to 1

The third formula is on inverse of tangent. What will happen if the input in the inverse tangent function is negative.

tan⁻¹(-x) = -tan⁻¹ (x)

Here, x belongs to the real number

The fourth formula is on inverse of cotangent . What will happen if the input in the inverse cotangent function is negative.

cot⁻¹(-x) = π - cot⁻¹ (x)

Here , x belongs to real number

The fifth formula is on inverse of secant. What will happen if the input in the inverse secant function is negative.

sec⁻¹(-x) = π - sec⁻¹ (x)

Here, x belongs to greater than or equal to 1.

The sixth formula is on inverse of cosecant. What will happen if the input in the inverse cosecant function is negative.

cosec⁻¹(-x) = -cosec⁻¹ (x)

Now let us look at the domain and range of the inverse trigonometric function

sin⁻¹(x)

The domain of the function is -1 ≤ x ≤ 1

The range is -π / 2 ≤ y ≤ π / 2.

cos⁻¹(x)

The domain of the function is -1 ≤ x ≤ 1

The range is 0 ≤ y ≤ π.

tan⁻¹(x)

The domain of the function is -∞ < x < ∞.

the range is -π / 2 < y < π /2.

cot⁻¹(x)

The domain of this function is -∞ < x < ∞.

The value for range can also be expressed as 0 < y < π.

sec⁻¹(x)

The value of the domain can be (−∞,−1]∪[1,∞)

The value of the range can be [0,π2)∪(π2,π]

cosec⁻¹(x)

The domain can be (−∞,−1]∪[1,∞)

range, the value can be denoted by -π / 2 < y < π /2; y ≠ 0.

NCERT Notes Subject wise link:

Importance of inverse trigonometric Functions class 12:

In this chapter, students have learned about the functions which are developed by finding the inverse of trigonometric ratios, graphs of inverse trigonometric functions, their domains, range, etc. The chapters hold their relevance, as well as importance in 12th grade as well and the concepts that we study here, will help us to easily grasp the upcoming concepts that we will be studying for your competitive exams like JEE mains, JEE advanced, State Engineering Entrance Exams, etc. Also, its weightage in the 12th board exam makes it one of the most important chapters for those who want to score really well in the board exams. Class 12 Inverse Trigonometry chapter 2 has been prepared with an objective of an overall evolution of students’ concepts in a manner that the students understand all the class 12 maths inverse trigonometry solutions, theorems, formulas, and derivations quite effectively by linking them with their practical applications.

NCERT Solutions Subject wise link:

NCERT Exemplar Solutions Subject wise link:

Frequently Asked Questions (FAQs)

1. What is the value of x can be if sin(x) = 3 ?

First of all, to find the value of x we will inverse the equation in the form of sine of inverse x = sin⁻¹(3) this is not possible because the value of x in sine inverse can be between -1 and 1

2. What is the weightage of inverse trigonometric function in jee mains

The total weightage of inverse trigonometric function is two percentage

3. Is it important to study class 12 chapter two inverse trigonometric function?

Yes, this is a very essential topic because if you don't understand the ideas in this chapter, you won't be able to answer derivative and integration issues in this chapter.

4. What are the additional identities of inverse trigonometric functions?

sin⁻¹(x) + cos⁻¹(x) = ?2

tan⁻¹(x) + cot⁻¹(x) = ?2

cosec⁻¹(x) + sec⁻¹(x) = ?2

5. What will happen if a same inverse trigonometric function is inside a inverse trigonometric function sin⁻¹(sin⁻¹(x)) like that ?

sin⁻¹(sin⁻¹(x)) is equal to x and this true for all the inverse trigonometric function

sin⁻¹(sin⁻¹(x)) = x

cos⁻¹(cos⁻¹(x))= x

tan⁻¹(tan⁻¹(x))=x

cot⁻¹(cot⁻¹(x))=x

cosec⁻¹(cosec⁻¹(x))=x

sec⁻¹(sec⁻¹(x))= x