Logarithmic Differentiation

Differentiation is one of the important parts of Calculus, which applies to measuring the change in the function at a certain point. Mathematically, it forms a powerful tool by which slopes of functions are determined, the maximum and minimum of functions found, and problems on motion, growth, and decay, to name a few. These concepts of differentiation have been broadly applied in branches of mathematics, physics, engineering, economics, and biology.

JEE Main 2025: Sample Papers | Mock Tests | PYQs | Study Plan 100 Days

JEE Main 2025: Maths Formulas  | Study Materials

JEE Main 2025: Syllabus | Preparation Guide | High Scoring Topics 

This Story also Contains

1. Differentiation
2. Differentiation of some basic functions
3. Differentiation Using Logarithm
4. Solved Examples Based on Differentiation Using Logarithms
5. Summary

In this article, we will cover the concept of Differentiation using Logarithm. This concept falls under the broader category of Calculus, a crucial Chapter in class 12 Mathematics. It is not only essential for board exams but also for competitive exams like the Joint Entrance Examination (JEE Main), and other entrance exams such as SRMJEE, BITSAT, WBJEE, BCECE, and more.

Differentiation

The process of finding the derivative is called differentiation. Let $f$ be defined on an open interval $I \subseteq$ containing the point $x_0$, and suppose that $\lim _{\Delta x \rightarrow 0} \frac{f\left(x_0+\Delta x\right)-f\left(x_0\right)}{\Delta x}$ exists. Then $f$ is said to be differentiable at $x_0$ and the derivative of $f$ at $x_0$, denoted by $f^{\prime}\left(x_0\right)$, is given by

$$
f^{\prime}\left(x_0\right)=\lim _{\Delta x \rightarrow 0} \frac{\Delta y}{\Delta x}=\lim _{\Delta x \rightarrow 0} \frac{f\left(x_0+\Delta x\right)-f\left(x_0\right)}{\Delta x}
$$

For all $x$ for which this limit exists,
$f^{\prime}(x)=\lim _{\Delta x \rightarrow 0} \frac{\Delta y}{\Delta x}=\lim _{\Delta x \rightarrow 0} \frac{f(x+\Delta x)-f(x)}{\Delta x}$ is a function of $x$.

In addition to $f^{\prime}(x)$, other notations are used to denote the derivative of $y=f(x)$. The most common notations are $f^{\prime}(x), \frac{d y}{d x}, y^{\prime}, \frac{d}{d x}[f(x)], D_x[y]$ or $D y$ or $y_1$. Here $\frac{d}{d x}$ or $D$ is the differential operator.

Differentiation of some basic functions

1. $\frac{d}{d x}($ constant $)=0$

2. $\frac{d}{d x}\left(\mathbf{x}^{\mathbf{n}}\right)=\mathbf{n} \mathbf{x}^{\mathbf{n}-1}$

3. $\frac{d}{d x}\left(\mathbf{a}^{\mathrm{x}}\right)=\mathbf{a}^{\mathrm{x}} \log _{\mathrm{e}} \mathbf{a}$

4. $\quad \frac{d}{d x}\left(\mathrm{e}^{\mathrm{x}}\right)=\mathrm{e}^{\mathrm{x}} \log _{\mathrm{e}} \mathrm{e}=\mathrm{e}^{\mathrm{x}}$

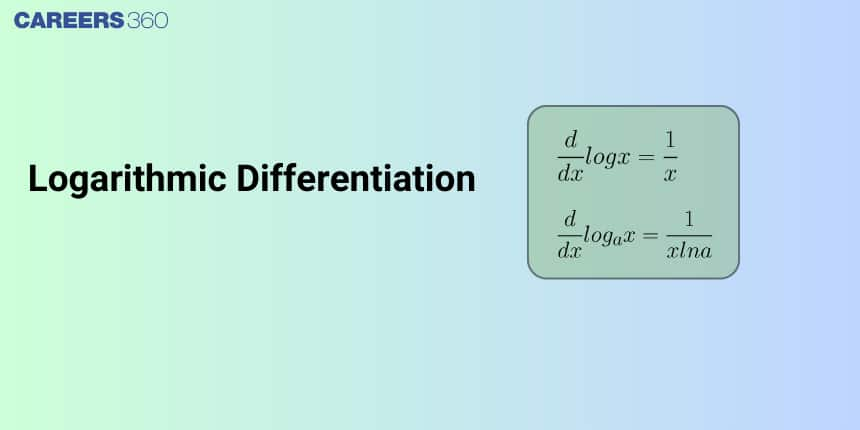
5. $\frac{d}{d x}\left(\log _{\mathrm{e}}|\mathbf{x}|\right)=\frac{\mathbf{1}}{\mathbf{x}}, \quad \mathbf{x} \neq 0$

6. $\quad \frac{d}{d x}\left(\log _{\mathbf{a}}|\mathbf{x}|\right)=\frac{1}{\mathbf{x} \log _{\mathrm{e}} \mathbf{a}}, \quad \mathbf{x} \neq 0$

7. $\frac{d}{d x}(\sin (\mathbf{x}))=\cos (\mathbf{x})$

8. $\frac{d}{d x}(\cos (\mathbf{x}))=-\sin (\mathbf{x})$

9. $\frac{d}{d x}(\tan (\mathbf{x}))=\sec ^2(\mathbf{x})$

10. $\frac{d}{d x}(\cot (\mathbf{x}))=-\csc ^2(\mathbf{x})$

11. $\frac{d}{d x}(\sec (\mathbf{x}))=\sec (\mathbf{x}) \tan (\mathbf{x})$

12. $\frac{d}{d x}(\csc (\mathbf{x}))=-\csc (\mathbf{x}) \cot (\mathbf{x})$

Differentiation Using Logarithm

Logarithm differentiation is used to find the derivative of some complex functions using logarithm. the method of differentiating functions by taking logs on both sides is called logarithm differentiation. This method is also known as Composite exponential function differentiation

1. Till now we have studied how to find derivatives of functions of the form $y=(g(x))^n$ (where $n$ is constant), as well as functions of the form $y=b^{g(x)}, b>0$ and $b \neq 1$ (where $b$ is a constant). But how to find the derivatives of functions of the form $y=[f(x)]^{g(x)}$ such as $y=x^x$ or $y=(\tan x)^{\sin (x)}$ ? These functions require a technique called logarithmic differentiation, which allows us to differentiate any function of the form $h(x)=[f(x)]^{g(x)}$

Let, $\quad y=(f(x))^{g(x)}$
Take $\log$ both side $\log y=g(x) \log f(x)$
Differentiate with respect to $x$

$
\begin{aligned}
& \frac{1}{y} \cdot \frac{d y}{d x}=g(x) \cdot \frac{1}{f(x)} \cdot \frac{d}{d x}(f(x))+\log f(x) \cdot \frac{d}{d x}(g(x)) \\
& \therefore \quad \frac{d y}{d x}=y\left[\frac{g(x)}{f(x)} \cdot \frac{d}{d x}(f(x))+\log f(x) \cdot \frac{d}{d x}(g(x))\right] \\
& \text { or } \quad \frac{\mathrm{dy}}{\mathrm{dx}}=(f(x))^{g(x)}\left[\frac{g(x)}{f(x)} \cdot \frac{d}{d x}(f(x))+\log f(x) \cdot \frac{d}{d x}(g(x))\right]
\end{aligned}
$

2. We can use logarithmic differentiation in cases where $y$ is made up of several factors in the numerator and denominator. Logarithm reduces the calculation time and effort in such cases.

Let, $\quad \mathrm{y}=\frac{\mathrm{f}_1(\mathrm{x}) \cdot \mathrm{f}_2(\mathrm{x}) \cdot \mathrm{f}_3(\mathrm{x}) \ldots}{\mathrm{g}_1(\mathrm{x}) \cdot \mathrm{g}_2(\mathrm{x}) \cdot \mathrm{g}_3(\mathrm{x}) \ldots}$
Take $\log$ both side

$
\begin{aligned}
\log y= & {\left[\log _e\left(f_1(x)\right)+\log _e\left(f_2(x)\right)+\log _e\left(f_3(x)\right)+\ldots\right] } \\
& \quad-\left[\log _e\left(g_1(x)\right)+\log _e\left(g_2(x)\right)+\log _e\left(g_3(x)\right)+\ldots\right]
\end{aligned}
$
Differentiating, w.r.t. $x$, we get

$
\begin{aligned}
& \frac{1}{y} \frac{d y}{d x}=\left[\frac{\left(f_1(x)\right)^{\prime}}{f_1(x)}+\frac{\left(f_2(x)\right)^{\prime}}{f_2(x)}+\frac{\left(f_3(x)\right)^{\prime}}{f_3(x)}+\ldots\right] \\
& -\left[\frac{\left(\mathrm{g}_1(\mathrm{x})\right)^{\prime}}{\mathrm{g}_1(\mathrm{x})}+\frac{\left(\mathrm{g}_2(\mathrm{x})\right)^{\prime}}{\mathrm{g}_2(\mathrm{x})}+\frac{\left(\mathrm{g}_3(\mathrm{x})\right)^{\prime}}{\mathrm{g}_3(\mathrm{x})}+\ldots\right]
\end{aligned}
$

Basic rules of Logarithms are:
- $\log A B=\log A+\log B$
- $\log A / B=\log A-\log B$
- $\log A^B=B \log A$
- $\log _B A=(\log A) /(\log B)$

Recommended Video Based on Differentiation Using Logarithms

Solved Examples Based on Differentiation Using Logarithms

Example 1: Let $y=\sin x^{\cos x}$, then $\frac{\mathrm{d} y}{\mathrm{~d} x}$ equals
1) $(\cos x)^{-\sin x}$
2) $(\cos x)(\sin x)^{-\cos x}$
3) $(\sin x)^{-\sin x}$
4) $(\sin x)^{\cos x}(\cos x \cdot \cot x-\sin x \cdot \log (\sin x))$

Solution:

$
y=(\sin x)^{\cos x}
$
Taking logarithms on both sides,

$
\begin{aligned}
\log _e y & =\log _e(\sin x)^{\mathrm{cos} x} \\
\Rightarrow & \log _e y=\cos x \log _e \sin x
\end{aligned}
$
Now differentiating both sides,

$
\begin{aligned}
& \frac{1}{y} \frac{d y}{d x}=\cos x \times \frac{\cos x}{\sin x}+(-\sin x) \log _e(\sin x) \\
& \Rightarrow \frac{d y}{d x}=y\left(\cos x \cdot \cot x-\sin x \cdot \log _e(\sin x)\right)
\end{aligned}
$

Hence, the answer is the option 4.

Example 2: Let $y=(x+1)(x+2)(x+3)(x+4)(x+5)$, then $\frac{\mathrm{d} y}{\mathrm{~d} x}$ at $x=0$ equals
1) $274$
2) $234$
3) $224$
4) $244$

Solution:

As we have learned,
Taking logarithms on both sides,

$
\log _e y=\log _e(x+1)+\log _e(x+2)+\log _e(x+3)+\log _e(x+4)+\log _e(x+5)
$
Now differentiating both sides,

$
\begin{aligned}
& \frac{1}{y} \frac{\mathrm{d} y}{\mathrm{~d} x}=\frac{1}{x+1}+\frac{1}{x+2}+\frac{1}{x+3}+\frac{1}{x+4}+\frac{1}{x+5} \\
& {\left[\frac{\mathrm{d} y}{\mathrm{~d} x}\right]_{(x=0)}=y(0)\left\{\frac{1}{1}+\frac{1}{2}+\frac{1}{3}+\frac{1}{4}+\frac{1}{5}\right\}=120+60+30+24=274[\text { As } y(0)=120]}
\end{aligned}
$

Hence, the answer is the option 1.

Example 3: Let $y=\frac{(x+1)^2(x+2)}{(x+3)}$, then $\frac{\mathrm{d} y}{\mathrm{~d} x}$ at $x=0$ equals
1) $\frac{9}{4}$
2) $\frac{4}{9}$
3) $\frac{9}{13}$
4) $\frac{13}{9}$

Solution:
Taking logarithms on both sides

$\ln y=2 \cdot \ln (x+1)+\ln (x+2)-\ln (x+3)$ Differentiating w.r.t. $\mathrm{x} \therefore \frac{1}{y} \frac{\mathrm{d} y}{\mathrm{~d} x}=\frac{2}{x+1}+\frac{1}{x+2}$

Hence, the answer is the option 4.

Example 4: $\frac{d}{d x} e^{x+2 \log x}$ is equal to?
1) $e^x\left(x^2-2 x\right)$
2) $e^x x^2(1+2 x)$
3) $e^x\left(x^2+3 x\right)$
4) $e^x\left(x^2+2 x\right)$

Solution:
Let $y=e^{x+2 \log x}$

$
\begin{aligned}
& \log _e y=x+2 \log _e x \\
& \frac{1}{y} \frac{d y}{d x}=1+\frac{2}{x} \\
& \frac{d y}{d x}=\frac{d}{d x} e^{x+2 \log x}=e^{x+2 \log x}\left(1+\frac{2}{x}\right)=e^x\left(x^2+2 x\right)
\end{aligned}
$

Hence, the answer is the option (4).

Example 5: $\frac{d}{d x} e^{x \cos x}$ is equal to?
1) $e^{x \cos x}(\cos x+x \sin x)$
2) $e^{x \cos x}(\sin x-x \cos x)$
3) $e^{x \cos x}(\sin x+x \cos x)$
4) $e^{x \cos x}(\cos x-x \sin x)$

Solution:
Let $y=e^{x \cos x}$

$
\begin{aligned}
& \log y=x \cos x \\
& \frac{1}{y} \frac{d y}{d x}=\cos x-x \sin x \\
& \frac{d y}{d x}=e^{x \cos x}(\cos x-x \sin x)
\end{aligned}
$

Hence, the answer is the option (4).

Summary

Logarithm differentiation is an important concept of Calculus used to find out the derivative of some complex functions by taking logs on both sides of the function. It provides a deeper understanding of mathematical ideas paramount for later developments in many scientific and engineering disciplines.