Many One Function - Definition, Formula and Examples

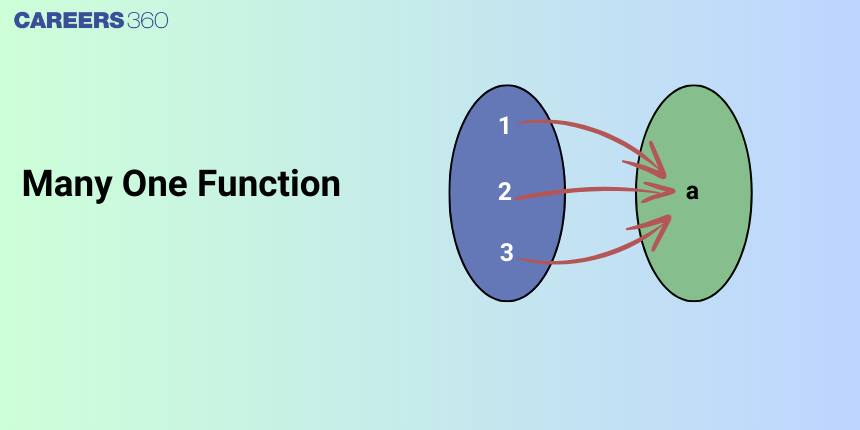
A many-one function, also known as a surjective function, is a function where each domain element is mapped to one or more elements in the codomain. In other words, two different elements in the domain map to the same element in the codomain. Understanding many-one functions is fundamental in various branches of mathematics, particularly in algebra and calculus.

JEE Main 2025: Sample Papers | Mock Tests | PYQs | Study Plan 100 Days

JEE Main 2025: Maths Formulas  | Study Materials

JEE Main 2025: Syllabus | Preparation Guide | High Scoring Topics 

This Story also Contains

1. What is many-one function?
2. Graph of Many to One Function
3. Properies of many-one funcion
4. Summary
5. Solved Examples Based on Many One Function

In this article, we will cover the concept of many-one functions. This concept falls under the broader category of relation and function. It is not only essential for board exams but also for competitive exams like the Joint Entrance Examination (JEE Main), and other entrance exams such as SRMJEE, BITSAT, WBJEE, BCECE, and more. Over the last ten years of the JEE Main exam (from 2013 to 2023), a total of one question have been asked on this concept, including one in 2023.

What is many-one function?

A function $f:X\to Y$ is called a many-one function if two or more elements of set $X$ have the same image in set $Y$,

Or we can say that if $f:X\to Y$ is many- one if it is not one-one function.

To check it graphically a line parallel to the x-axis cuts the curve at more than one point.

Both are many one, as in both there are two elements $x_2,x_3$ which corresponds to the same image $y_3$, i.e. $f\left(x_2\right)=f\left(x_3\right)=y_3$

Examples of many one function

Square function:
- Domain: All real numbers $(R)$
- Codomain: Non-negative real numbers ([0, $\mathrm{\infty })$ )
- Function: $f(x)=x^2$

Floor function:
- Domain: All real numbers $(R)$
- Codomain: All Integers $(Z)$
- Function: $f(x)=\lceil x\rceil$

Absolute value function
- Domain: All real numbers $(R)$
- Codomain: Non-negative real numbers ([0, =))
- Function: $f(x)=|x|$

Graph of Many to One Function

The graph of a many-to-one function doesn’t pass the horizontal line test for at least one point in its range. To check whether a function is many-one, we only have to draw a line parallel to the x-axis on the graph. If it intersects the graph at more than one point, the function is a many-one function.

Let’s consider an example of a many-one function, i.e., f(x) = x². As x² maps both 1 and -1 to 1, it is an example of a many-to-one function. You can see the graph for this function below:

Method to check many-one

Check whether the function is one-one or not. If the function is not one-one then it is a many-one function.

Properies of many-one funcion

The following is a list of some frequent properties of Many-One Function:

• An inverse function cannot exist for a many-to-one function.
• At least two components in the function's domain should share the same codomain value.
• A single output may result from numerous inputs. Distinct inputs (Domains) may yield identical results.
• The many-to-one function is also referred to as the onto function if each value in the range has an input picture.
• If there is just one codomain, the many one function can also be referred to as a constant function.
• There should always be more elements in the domain of many one functions than there are in the codomain.

Summary

Many-to-One or Many One Function is one of the various types of functions that represent relationships between different entities. As we know, a function is a very specific type of relation in which each input has a unique output. many to one function means When multiple elements are related to one element, or where multiple inputs can give the same output, that relation is known as Many to one function.

Recommended Video :

Solved Examples Based on Many One Function

Example 1: Find the domain and range of the many one function $f=\{(4,x),(5,x),(6,x),(7,y),(8,y),(9,z)\}$

Solution:

The given function is $f=\{(4,x),(5,x),(6,x),(7,y),(8,y),(9,z)\}$
Here we have:
Domain $=(4,5,6,7,8,9)$
Range $=(x,y,z)$

We observe that the elements 4, 5, and 6 in the domain are all connected to the same element 'x' in the range. Hence, this function, which connects multiple elements in the domain to a single element in the range, is a many-one function.

Therefore, the given function is a many-one function.

Example 2: Which of the following is a many-one function?

1) $f(x)=\log (x)$
2) $f(x)=2^x$
3) $f(x)=x^{10}$
4) None of these

Solution:

Graphs of the functions given in the options are

1) $y=\log (x)$

Clearly, one-one

2) $y=2^x$

Clearly. one-one

3) $y=x^{10}$

Many-one

Alternatively, this is a polynomial of even degree, so many-one

So, $\mathrm{f}(\mathrm{x})=x^{10}$ is a many-one function.

Hence, the answer is option 3.

Example 3: Which of the following functions is a many-one function?

1)

2)

3)

4) None of these

Solution:

For second option

we can have many horizontal lines that cut the graph at more than one point. So it is many one function

Example 4: Show that the function $f:R\to R$ defined by $f(x)=3x^2+5,\mathrm{\forall }x\in R$ is many one function.

Solution: Since $f(-1)=3\times (-1{)}^2+5=8$ and $f(1)=3\times (1{)}^2+5=8$, so the different elements -1 and 1 of the domain set $\mathrm{R}$ have the same image in the codomain set $\mathrm{R}$.

Therefore, $f$ is a many-one function. Hence proved.