Nature of Integral Part of Expression N = (a + sqrt b)^n

An algebraic expression consisting of only two terms is called a Binomial Expression. Expressions with a higher power are difficult to solve. In these cases, binomial theorem plays a major role. Binomial theorem is used for the expansion of a binomial expression with a higher degree. Mathematical induction is one of the techniques which can be used to prove a variety of mathematical statements which are formulated in terms of n, where n is a positive integer. Binomial theorem is proved using the concept of mathematical induction. Apart from mathematics, Binomial theorem is also used in various fields for statistical and financial data analysis.

JEE Main 2025: Sample Papers | Mock Tests | PYQs | Study Plan 100 Days

JEE Main 2025: Maths Formulas  | Study Materials

JEE Main 2025: Syllabus | Preparation Guide | High Scoring Topics 

This article is about a theorem to find the nature of integral part of an expression which falls under the topic Binomial theorem and its applications. This is one of the important topics for competitive exams.

Binomial Theorem

If $n$ is any positive number, then $(a + b)^n = \binom{n}{0} a^n + \binom{n}{1} a^{n - 1} b + \binom{n}{2} a^{n - 2} b^2 + \dots + \binom{n}{n - 1} a b^{n - 1} + \binom{n}{n} b^n $

Binomial Coefficient

The combination $\binom{n}{r}$ or ${ }^{\mathrm{n}} \mathrm{C}_{\mathrm{r}}$ occuring in the Binomial theorem is called a Binomial coefficient, where $\binom{n}{r}=C(n, r)={ }^n C_r=\frac{n!}{r!(n-r)!}$.

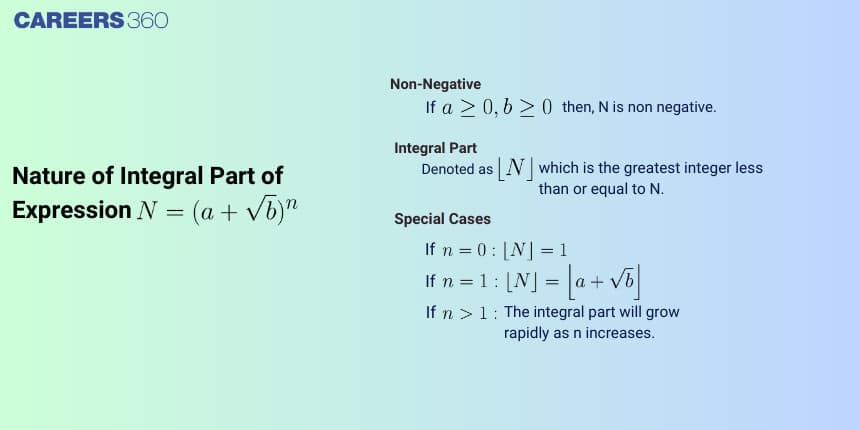
Nature of an integral part of the expression $N=(a+\sqrt{b})^n$

If the given expansion is in the form of $N=(a+\sqrt{b})^n \quad(n \in N)$

To find the integral part,

Step 1: Choose $N^{\prime}=(a-\sqrt{b})^n$ or $(\sqrt{b}-a)^n$ according as $a>\sqrt{b}$ or $\sqrt{b}>a$

Step 2: Use $N + N'$ or $N-N'$ such that result is an integer

I.e. $(a+\sqrt{b})^n+(a-\sqrt{b})^n$ or $(a+\sqrt{b})^n-(a-\sqrt{b})^n$ is an integer Step 3: Now use the concept greatest integer function and fractional part of a function, $\mathrm{N}=$ $I+f$, where $I$ is an integral part of $N$ i.e., $[N]$ and $f$ is a fractional part of $N$, i.e. $\{\mathrm{N}\}$.

For example, the integral part of $P=(3 \sqrt{3}+5)^{2 n+1}(n \in N)$ is an even number.

Now consider, $P^{\prime}=(3 \sqrt{3}-5)^{2 n+1}$ here $0<P^{\prime}<1$

Use, $\left.P-P^{\prime}=2\left[{ }^{2 n+1} C_1(3 \sqrt{3})^{2 n} 5^1+{ }^{2 n+1} C_3(3 \sqrt{3})^{2 n-2}(5)^3+\ldots \ldots\right]\right]$

$I+f-P^{\prime}=2 k(k \in N)$

$ (P=I+f) $

$-1<f-P^{\prime}<1$ but $f-P^{\prime}$ is an integer $\Rightarrow f-P^{\prime}=0 \Rightarrow I=2 k$

Hence, integral part of $P=(3 \sqrt{3}+5)^{2 n+1}(n \in N)$ is an even integer

Recommended Video

Solved Examples

Example 1: If $P=(7+4 \sqrt{3})^9$ and $P=\mathrm{I}+\mathrm{f}$, where I is the integer just less than P , then the value of $P(1-f)$ is equal to

1) $1 $

2) $2^n$

3) $2 $

4) $2^{2 n}$

Solution:

Now,

$P=(7+4 \sqrt{3})^9 $

Let $P^{\prime}=(7-4 \sqrt{3})^9 \quad \& \quad 0<7-4 \sqrt{3}<1$

Using Binomial addition for $P+P'$

$ P+P^{\prime}=2 \cdot\left[{ }^9 C_0 7^9+{ }^9 C_2 7^7 \cdot(4 \sqrt{3})^2+{ }^9 C_4 7^5 \cdot(4 \sqrt{3})^4 \cdots \cdots\right] $

and it is given that $P+I+f$, where $0<f<1$

Hence,

$ I+f+P^{\prime}=2 \cdot\left[{ }^9 C_0 7^9+{ }^9 C_2 7^7 \cdot(4 \sqrt{3})^2+{ }^9 C_4 7^5 \cdot(4 \sqrt{3})^4 \ldots \ldots\right] $

So, $I+f+P^{\prime}=$ even integer

Now it is clear that $I$ is an integer so $f+P^{\prime}$ should be an integer

$f$ lies between $(0,1)$ and $P^{\prime}$ also lies between $(0,1)$

hence, $f+P^{\prime} \in(0,2)$

But their sum is an integer and 1 is the only integer that lies between this interval

hence, $f+P^{\prime}=1$

$ P^{\prime}=1-f $

we need to calculate $P(1-f)$

$ \text { or } P(1-f)=P P^{\prime}=\left[(7+4 \sqrt{3})^9(7-4 \sqrt{3})^9\right] $

$P(1-f) =\left[(7+4 \sqrt{3})^9(7-4 \sqrt{3})^9\right] $

$ =[(7+4 \sqrt{3})(7-4 \sqrt{3})]^9 $

$ =[49-48]^9 $

$ =1^9=1 $

Hence, the answer is option 1.

Example 2: If $P=(10+3 \sqrt{11})^n$ and $P^{\prime}=(10-3 \sqrt{11})^n$, then which one of the following is not true?

1) $P P^{\prime}=1$

2) $P+P^{\prime}$ is an even integer

3) $P^{\prime}=1-f$ where, $f$ is the fractional part of $P$

4) $f+P^{\prime} \in(0,1)$

Solution:

Now

i) $P P^{\prime}=(10+3 \sqrt{11})^n(10-3 \sqrt{11})^n=\left(10^2-(3 \sqrt{11})^2\right)^n=1^n=1$

ii) $P+P^{\prime}=2\left[{ }^n C_0(10)^n+{ }^n C_2(10)^{n-2}(3 \sqrt{3})^2+{ }^n C_2(10)^{n-4}(3 \sqrt{3})^4 \cdots \cdots\right]$

hence, it is an even integer

iii) Let $P=[P]+f$, where $[\mathrm{P}]$ is the integer just less than P

Now, from (ii)

$ P+P^{\prime}=\text { even integer } $

$ P+P^{\prime}=[P]+f+P^{\prime}=\text { Integer }$

Example 3: If $x=(7+4 \sqrt{3})^{2 n}=[x]+f$, then $x(1-f)$ is equal to $\qquad$ .

1) $1 $

2) $2 $

3) $3 $

4) $0 $

Solution:

We have ${ }^{7-4 \sqrt{3}}=\frac{1}{7+4 \sqrt{3}}$

$\therefore 0<7-4 \sqrt{3}<1 \Rightarrow 0<(7-4 \sqrt{3} 3)^{2 n}<1 $

Let $F=(7-4 \sqrt{3})^{2 n}$, then

$ x+F=(7+4 \sqrt{3})^{2 n}+(7-4 \sqrt{3})^{2 n} $

$ =2\left[{ }^{2 n} C_0 7^{2 n}+{ }^{2 n} C_2 7^{2 n-2}(4 \sqrt{3})^2+{ }^{2 n} C_4 7^{2 n-4}(4 \sqrt{3})^4+\ldots+{ }^{2 n} C_{2 n}(4 \sqrt{3})^{2 n}\right] $

$=2 m$, where $m$ is some positive integer

$\Rightarrow[x]+f+\mathrm{F}=2 m $

$ \Rightarrow f+F=2 m-[x] $

Since, $0 \leq f<1$ and $0<F<1$, we get $0<f+\mathrm{F}<2$. Also, since $f+F$ is an integer, we must have $f+\mathrm{F}=1$

Thus, $x(1-f)=x F=(7+4 \sqrt{3})^{2 n}(7-4 \sqrt{ } 3)^{2 n}=(49-48)^{2 n}=1^{2 n}=1$

Hence, the answer is option (1).

Example 5: Let $R=(5 \sqrt{5}+11)^{2 n+1}$ and $f=R-[R]_{\text {where [.] denotes the greatest }}$ integer function. The value of $R . f$ is

1) $4^{2 n+1}$

2) $4^{2 n}$

3) $4^{2 n-1}$

4) $4^{-2 n}$

Solution:

Since $f=R-[R] \quad R=f+[R][5 \sqrt{5}+11]^{2 n+1}=f+[R]_{\text {where }[R] \text { is integer }}$

Now let $f^{\prime}=[5 \sqrt{5}-11]^{2 n+1}, 0<f^{\prime}<1$

$ f+[R]-f^{\prime}=[5 \sqrt{5}+11]^{2 n+1}-[5 \sqrt{5}-11]^{2 n+1}= $

$2\left[{ }^{2 n+1} C_1(5 \sqrt{5})^{2 n}(11)^1+{ }^{2 n+1} C_3(5 \sqrt{5})^{2 n-2}(11)^3+\ldots \ldots .\right] $

$ =2 \cdot(\text { Integer })=2 K(K \in N)=\text { Even integer } $

Hence

$f-f^{\prime}=$ even integer $-[R]$, but $-1<f-f^{\prime}<1$.
Therefore, $f-f^{\prime}=0 \quad \therefore f=f^{\prime}$

Hence R. $f=R \cdot f^{\prime}=(5 \sqrt{5}+11)^{2 n+1}(5 \sqrt{5}-11)^{2 n+1}=4^{2 n+1}$.

Hence, the answer is option (1).

Summary

An algebraic expression consisting of only two terms is called a Binomial Expression. Binomial theorem is used for the expansion of a binomial expression with a higher degree. Understanding the concepts of Binomial theorem could help us solve more complex problems.