Nature of Roots of Cubic Polynomial

The roots of cubic Polynomials are an important concept in mathematics. Polynomials are algebraic expressions that contain constants, variables, and exponents. Graphical analysis, numerical methods, and cubic polynomials provide valuable insights into the behaviour of algebraic equations.

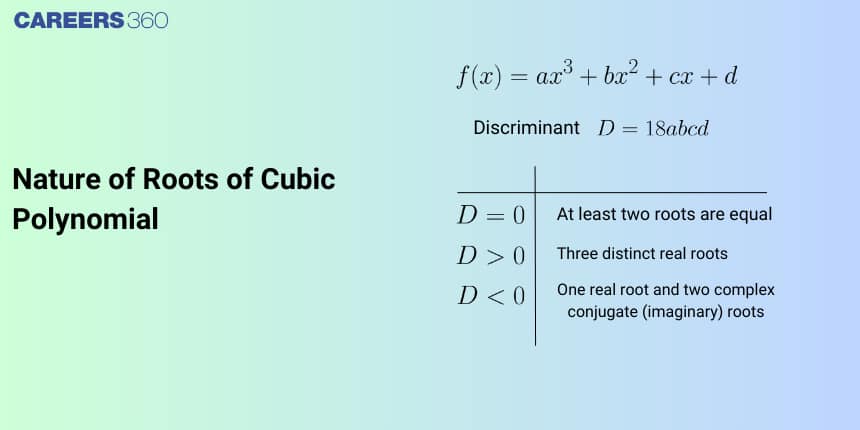
In this article, we will cover the concept of the Nature of the Roots of Cubic Polynomials. This is very important not only for board exams but also for competitive exams, which even include the Joint Entrance Examination Main and other entrance exams: SRM Joint Engineering Entrance, BITSAT, WBJEE, and BCECE. A total of three questions have been asked on this topic in JEE Main from 2013 to 2023, including one question in 2021, one in 2022, and one in 2023.

JEE Main 2025: Sample Papers | Mock Tests | PYQs | Study Plan 100 Days

JEE Main 2025: Maths Formulas  | Study Materials

JEE Main 2025: Syllabus | Preparation Guide | High Scoring Topics 

Nature of Roots of Cubic Polynomial

A cubic polynomial is a mathematical equation with power three. The polynomial crosses the $x$-axis at one point, and the other two roots are complex.
The roots of the cubic polynomial are the solution of the cubic equation. As the power is three the number of roots will also be three.
Let the cubic polynomial be $f(x)=a x^3+b x^2+c x+d$ and $f(x)=0$ is a cubic equation where $a, b, c$, and $d \in R$ and $a>0$.

Now, ${f}^{\prime}(x)=3 a x^2+b x+c$
Now, $\quad f^{\prime}(x)=a x^2+b x+c$
Let $D=4 a^2-12 b=4\left(a^2-3 b\right)$ be the discriminant of the equation $f^{\prime}(x)=0$

Now, we will have the following cases

Case 1
If $\mathrm{D}<0 \Rightarrow \mathrm{f}^{\prime}(\mathrm{x})>0 \forall \mathrm{x} \in \mathrm{R}$.
That means $f(x)$ would be an increasing function of x
Also, $\lim\limits _{x \rightarrow-\infty} f(x)=-\infty$ and $\lim\limits _{x \rightarrow \infty} f(x)=\infty$
Also, from the graph, it is clear that $f(x)$ cut the $x-$ axis only once.
Clearly $x_0>0$ if $d<0$, and $x_0<0$ if $d>0$

Case 2
If $D>0 \Rightarrow f^{\prime}(x)=0$ would have two real roots, say $x_1$ and $x_2$ let $\mathrm{x}_1<\mathrm{x}_2$

$
\begin{array}{ll}
\Rightarrow & f^{\prime}(x)=3 a\left(x-x_1\right)\left(x-x_2\right) \\
\Rightarrow & f^{\prime}(x)= \begin{cases}f^{\prime}(x)<0, & x \in\left(x_1, x_2\right) \\
f^{\prime}(x)=0, & x \in\left\{x_1, x_2\right\} \\
f^{\prime}(x)>0 & \left(-\infty, x_1\right) \cup\left(x_2, \infty\right)\end{cases}
\end{array}
$

Here, $\mathrm{x}=\mathrm{x}_1$ is point of local maxima and $\mathrm{x}=\mathrm{x}_2$ is point of local minima

Case 3
If $D=0 \Rightarrow \mathrm{f}^{\prime}(\mathrm{x})=3 \mathrm{a}\left(\mathrm{x}-\mathrm{x}_1\right)^2$
When, $x_1$ is root of $f^{\prime}(x)=0$, then $f(x)=a\left(x-x_1\right)^3+C$.
If $\mathrm{C}=0$, then $\mathrm{f}(\mathrm{x})=\mathrm{a}\left(\mathrm{x}-\mathrm{x}_1\right)^3$ has 3 equal roots if, $\mathrm{C} \neq 0$, then $\mathrm{f}(\mathrm{x})=0$ has one real root.

Thus, the graph of y = f(x) could have five possibilities as shown below:

(i)

(ii)

(iii)

(iv)

(v)

Conclusion:

a. If $f\left(x_1\right) f\left(x_2\right)>0, f(x)=0$ would have just one real root.
b. If $f\left(x_1\right) f\left(x_2\right)<0, f(x)=0$ would have three real and distinct roots.
c. If $f\left(x_1\right) f\left(x_2\right)=0, f(x)=0$ would have three real roots but one of the roots would be repeated.

Recommended Video Based on the Nature of Roots of Cubic Polynomial

Solved Examples Based on the Nature of Roots of Cubic Polynomial:

Example 1: Let a be an integer such that all real roots the polynomial $2 x^2+5 x^4+10 x^3+10 x^2+10 x+10$ lie in the interval $(\mathrm{a}, \mathrm{a}+1)$. Then, $|a|$ is equal to

1) 2

2) 4

3) 6

4) 5

Solution
$
\begin{aligned}
& \text { Let } 2 x^5+5 x^4+10 x^3+10 x^2+10 x+10=f(x) \\
& f(x)=x^5+x^5+5 x^4+10 x^3+10 x^2+5 x+10+5 x+9 \\
& f(x)=x^5+5 x+9+(x+1)^5 \\
& f^{\prime}(x)=5 x^4+5+0+5(x+1)^4>0
\end{aligned}
$

$\mathrm{f}(\mathrm{x})$ is an increasing function
Now $f(-2)=-34$ and $f(-1)=3$
Hence $f(x)$ has a root in $(-2,-1)$

So, $a=-2,|a|=2$.

Hence, the answer is the option 1.

Example 2: The number of distinct real roots of $x^4-4 x+1=0$ is :
1) 4
2) 2
3) 1
4) 0

Solution
$f(x)=x^4-4 x+1$
$f^{\prime}(x)=4 x^3-4=0$
$\Rightarrow \mathrm{x}=1$
$f^{\prime \prime}(x)=12 x^2, \quad f^{\prime \prime}(1): 12>0$
$\Rightarrow \mathrm{x}=1$ is a point of minima
$f(1): 1-4+1=-2$
For $\mathrm{x}<1, \quad \mathrm{f}(\mathrm{n})$ is decreasing and for $\mathrm{x}>1, \quad \mathrm{f}(\mathrm{x})$ is in increasing

$
\mathrm{f}(-\infty)=\infty, \quad \mathrm{f}(1)=-2, \quad \mathrm{f}(\infty)=\infty
$
From the intermediate value theorem, $f(x)$ will have 2 real roots one less than 1 and the other greater than 1.
Hence, the answer is the option (2).

Example 3: Let $\alpha, \beta, \gamma$, be the three roots of the equation $x^3+b x+c=0$ If $\beta \gamma=1=-\alpha$, then $b^3+2 c^3-3 \alpha^3-6 \beta^3-8 \gamma^3$ is
1) $\frac{155}{8}$
2) 21
3) 19
4) $\frac{169}{8}$

Solution

$
\begin{aligned}
& \beta \gamma=1 \\
& \alpha=-1 \\
& \text { Put } \alpha=-1 \\
& -1-b+c=0 \\
& c-b=1
\end{aligned}
$

also

$
\begin{aligned}
& \alpha \cdot \beta \cdot \gamma=-\mathrm{c} \\
& -1=-\mathrm{c} \Rightarrow \mathrm{c}=1 \\
& \therefore \mathrm{b}=0 \\
& \mathrm{x}^3+1=0 \\
& \alpha=-1, \beta=-\mathrm{w}, \gamma=-\mathrm{w}^2 \\
& \therefore \mathrm{b}^3+2 \mathrm{c}^3-3 \alpha^3-6 \beta^3-8 \gamma^3 \\
& 0+2+3+6+8=19
\end{aligned}
$

Summary

Cubic Equations are an important part of algebra and finding their roots is helpful in daily life for checking where the polynomials are zero. Understanding the roots of cubic polynomials combines algebraic theory and practical methods.