Periodic Function - Definition, Examples, Formula, Equations

A periodic function is a function that repeats its values at regular or certain intervals or periods. Periodic functions are fundamental in various branches of mathematics, physics, and engineering, especially in the study of waves, aerophysics, oscillations, and signal processing and many more. Understanding periodic functions is crucial in many scientific and engineering disciplines, where they model repeating phenomena and signals.

JEE Main 2025: Sample Papers | Mock Tests | PYQs | Study Plan 100 Days

JEE Main 2025: Maths Formulas  | Study Materials

JEE Main 2025: Syllabus | Preparation Guide | High Scoring Topics 

In this article, we will cover the concept of periodic function. This concept falls under the broader category of sets relation and function, a crucial Chapter in class 11 Mathematics. It is not only essential for board exams but also for competitive exams like the Joint Entrance Examination (JEE Main), and other entrance exams such as SRMJEE, BITSAT, WBJEE, BCECE, and more.

Periodic function

A relation from a set $A$ to a set $B$ is said to be a function from $A$ to $B$ if every element of set $A$ has one and only one image in set $B$. Now let us look into what is periodic function class 11.

What is Periodic Function Class 11?

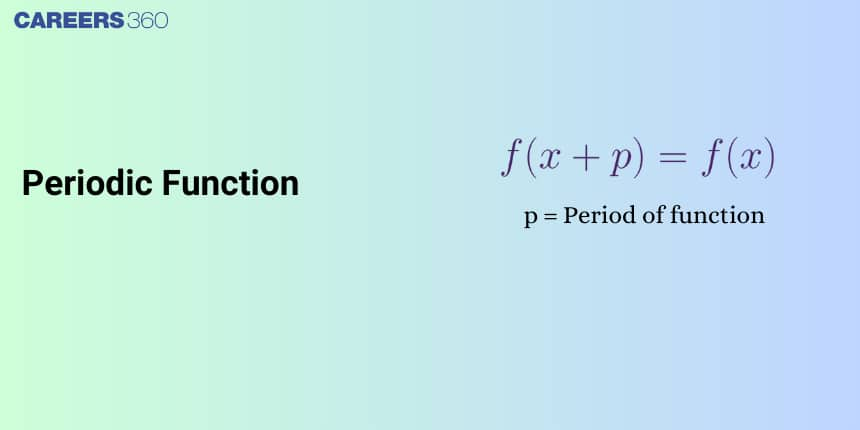
A function $f(x)$ is called a periodic function, if there exists a +ve real number $T$ such that $f(x+T)=f(x) \forall x$ belongs Domain of $f(x)$.

Here, $T$ is called the period of $f(x)$, where $T$ is the least $+v e$ value.

The fundamental period of a function is defined by the least value of the positive real number $P$, at which the function repeats itself.

$f(x + P) = f(x)$

Graphically we can say that if the graph repeats at a fixed interval, the function is said to be periodic and then its period is calculated by the width of that interval.

Eg.

Graph of $sin(x)$ is repeated at an interval of $2π$

Periods of Some Important Periodic Functions

The period of a function helps us to know the interval, after which the range of the periodic function repeats itself. The domain of a periodic function $f(x)$ includes of the real number values of $x$, the range of a periodic function is a limited set of values within an interval. The length of this repeating interval is called the period of the periodic function.

Some standard results of periodic function

Properties of the periodic function

The following properties gives us a deep understanding of the concepts of a periodic function and provide us with more clarity.

• The graph of a periodic function is symmetric and repeats itself along the $x$ axis.
• The domain of the periodic function includes all the real number values, and the range of the periodic function is defined for a fixed interval only.
• The period of a periodic function, is equal to the constant across the entire range of the function.
• If $f(x)$ is a periodic function with a period of $P$, then $\frac{1}{f(x)}$ will also be a periodic function with $P$.
• If $f(x)$ is a periodic function with a period of $P$, then $f(a x+b)$ is also a periodic function with a period of $\frac{\mathrm{P}}{|a|}$.
• If $f(x)$ is a periodic function with a period of $P$, then $a f(x)+b$ is also a periodic function with $P$.
• i) if $f(x)$ is periodic with period $T$, then
  1. $\operatorname{cf}(x)$ is periodic with period $T$
  2. $f(x+c)$ is periodic with period $T$
  3. $f(x) \pm c$ is periodic with period $T$, where $c$ is any constant.
  ii) if $f(x)$ is periodic with period $T$, then $k f(c x+d)$ has period $\frac{T}{|c|}$ i.e. period is only affected by the coefficient of $x$.

Some Important Periodic Functions

Euler's Formula: The periodic functions sine and cosine make up the complex number formula $e^{i x}=\cos k x+i \sin k x$ In this case, the two functions are periodic, and the periodic function represented by the euler's formula has a period of $\frac{2π}{k}$.

Jacobi Elliptic Functions: These functions have an elliptical graph. These elliptical forms result from the simultaneous involvement of two variables, such as the temperature and viscosity of the material. These functions are frequently employed to explain a pendulum's motion like that in physics and other related domains.

Periodic Function in Fourier Series: The Fourier series is a complex periodic function that is created by superimposing different periodic wave function series, made up of sine and cosine functions. We calculate the sum of series by assigning appropriate weight to the various terms involved in the given series. The series play great importance in analysis of various domains of physics like vibration analysis, electrical engineering, signal processing, etc.

Periodic Function in Complex analysis: Periodic function in complex analysis should satisfy the condition $f(z+T)=f(z)$ for all $z$ where $T$ is a nonzero complex constant called the period. If $T$ is samlest, then it is a fundamental period. The examples are exponential function $e^z$ with period $2 \pi i$ and trigonometric functions $\sin (z)$ and $\cos (z)$.

How to Find the Period of a Function?

Some methods to find the period are listed below:

1. Inspection:

   • For standard trigonometric functions like $sin⁡(x), cos⁡(x)$, and $tan⁡(x)$, the periods are well-known: $2π$ for sine and cosine, $π$ for tangent. So just by looking at the function,we recognise its period.

2. Algebraic Method:

   • For a function $f(x)$ defined by a formula, we usually set up the equation $f(x+T)=f(x)$ and solve for $T$, to get the desired output.

3. Graphical Method:

   • We plot the function and visually inspect the distance between repeating patterns to identify the period.

Periodic Function Examples in Real Life

Periodic function examples in real life include the motion of a pendulum, the change of day and night, the change in the seasons, the rhythm of the heartbeat, and a lot more. Periodic function examples in real life include those that repeat in regular intervals.

Solved Examples Based On the Periodic Functions

Example 1: What is the period of $f(x)=\cos (\cos x)+\cos (\sin x)$ ?
Solution:
The period of $\cos (\cos x)$ is $\pi$ and it is an even function.
The period of $\cos (\sin x)$ is $\pi$ and it is an even function
Thus, the period of $f(x)$ is $\frac{1}{2}($ LCM of $\pi, \pi)=\frac{\pi}{2}$
Hence, the answer is $\frac{\pi}{2}$.

Example 2: What is the period of $\tan (5x)$?

Solution:

As we have learned in Properties of the Periodic function

If $f(x)$ is periodic with period $T$, then $k f(c x+d)$ has a period $\frac{T}{|c|}$

Now,

As the period of $tan(x)$ is $π$, the period of $tan(5x)$ is $\frac{π}{5}$.

Hence, the answer is $\frac{π}{5}$.

Example 3: Let $f(x)$ be a differentiable function for all $x \in R$. The derivative of $f(x)$ is an even function. If the period of $f(2 x)$ is 1 , then the value of $f(2)+f(4)-f(6)-f(8)$ is
Solution:

$\begin{aligned}
& f(2 x)=f(2 x+1) \\
& f(x)=f\left(x+\frac{1}{2}\right)
\end{aligned}$

So period of $f(x)_{\text {is }} \frac{1}{2}$

$\begin{aligned}
& f(4)=f\left(2+\frac{1}{2} \times 4\right) \\
& f(6)=f\left(2+\frac{1}{2} \times 8\right) \\
& f(8)=f\left(2+\frac{1}{2} \times 12\right)
\end{aligned}$

So $f(2)=f(4)=f(6)=f(8)$
So value of $f(2)+f(4)-f(6)=f(8)=0$

Hence the answer is $0$.

Example 4: The function $f(x)=|\sin 4 x|+|\cos 2 x|$, is a periodic function with a period:

Solution:

As we have learned,
- If period of $f(x)$ is $T$, then period of $f(c x+d)$ is $\frac{T}{|c|}$
- If period of $f(x)$ is $T1$ and period of $g(x)$ is T2, then period for $f(x)+$ $\mathrm{g}(\mathrm{x})$ is the lcm of $T1$ and $T2$
- Period of $|\sin (\mathrm{x})|$ and $|\cos (\mathrm{x})|$ is $\pi$

Now,
As period of $|\sin (x)|$ is $\pi$, so period of $|\sin (4 x)|$ is $\frac{\pi}{4}$, and
As period of $|\cos (\mathrm{x})|$ is $\pi$, period of $|\cos (2 \mathrm{x})|$ is $\frac{\pi}{2}$
So period of $|\sin (4 x)|+|\cos (2 x)|$ is the $\mid c m$ of $\frac{\pi}{4}$ and $\frac{\pi}{2}$, which is $\frac{\pi}{2}$.
Hence, the answer is $\frac{\pi}{2}$.

Example 5: What is the period of $\frac{\sin x}{2}+\cos 2 x$ ?
Solution:

As we have learned
Period of a Trigonometric Ratio -
Period of $\sin x$ and $\cos x$ is $2 \pi$
- wherein

$\sin (2 n \pi+x)=\sin x$
$\cos (2 n \pi+x)=\cos x$
period of $\frac{\sin x}{2}$ is $4 \pi$
period of $\cos 2 x$ is $\frac{2 \pi}{2}=\pi$
Thus period $=4 \pi$
Hence, the answer is $4 \pi$.

List of Topics Related to Periodic Functions