Permutation vs Combination: Definition and Formulas
Permutations and combinations are the ways to select certain objects from a group of objects to form subsets with or without replacement. Permutations involve the arranging of objects i.e. to place them in a specific order among themselves. In combinations, we select the object a few objects out of the given objects. In real life, we use permutations for arranging people, digits, colours, and combinations for the selection of menus, food, clothes, and subjects.
JEE Main: Study Materials | High Scoring Topics | Preparation Guide
JEE Main: Syllabus | Sample Papers | Mock Tests | PYQs
In this article, we will learn about the difference between Permutations and combinations. This topic falls under the broader category of Permutations and combinations, which is a crucial chapter in Class 11 Mathematics. This is very important not only for board exams but also for competitive exams, which even include the Joint Entrance Examination Main and other entrance exams: SRM Joint Engineering Entrance, BITSAT, WBJEE, and BCECE.
Permutation
A permutation is an arrangement of all or part of a set of objects, with regard to the order of the arrangement.
Arranging n objects in r places (Same as arranging n objects taken r at a time) is equivalent to filling r places from n things.
Formula of permutation
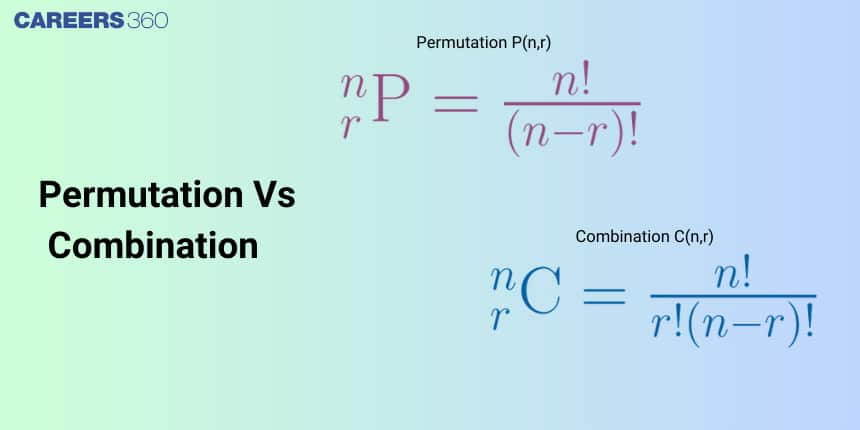
The number of ways of arranging n objects taken r at a time $={ }^n P_r$
Combination
The meaning of combination is selection. The notation of selecting r objects from n given object is ${ }^{\mathrm{n}} \mathrm{C}_{\mathrm{r}}$
Formula of combination
$\begin{aligned} & { }^n C_r \times r!={ }^n P_r \\ & { }^n C_r=\frac{{ }^n P_r}{r!} \\ & { }^n C_r=\frac{n!}{(n-r)!r!}\end{aligned}$
Relation between Permutation and combination
${ }^n C_r \times r!={ }^n P_r$
Permutations Vs Combinations
Always remember, in an arrangement, the order is always important. Whereas, in Combination, the order is not important.
Consider the following examples-
1. Selecting a team of 11 from 16 players - Selection
Drawing a batting line-up of 11 from 16 players - Arrangement
2. Selecting 3 students out of 10 students who will receive scholarships of the same value - Selection
Selecting 3 students out of 10 students who will receive scholarships of Rs. 500, Rs. 1000 and Rs. 2000 - Arrangement.
Difference Between Permutation and Combination
The difference between the Permutations and combinations is given below.
| Permutations | Combinations |
| Arranging people, digits, numbers, alphabets, letters, and colours | Selection of menu, food, clothes, subjects, team. |
| Picking a team captain, pitcher and shortstop from a group. | Picking three team members from a group. |
| Picking first, second and third place winners. | Picking three winners. |
| Picking two favourite colours, in order, from a colour brochure. | Picking two colours from a colour brochure. |
Applications of Permutation and Combination
Some applications of permutation and combinations are given below:
1) Passwords and Security
When you set a password, you select characters from a set of possibilities, such as letters, numbers, and symbols. The more options you have for each character position, the more potential combinations there are for your password.
2) Travel Itineraries and Planning
Planning a vacation often involves looking at all the places you want to visit and figuring out the best sequence to do so, which is a practical application of permutations (if travel time between locations matters) and combinations (if you’re just selecting destinations to visit without a set order).
3) Sports Teams Line-ups
Consider a baseball team manager thinking about the batting order. The sequence in which players bat is crucial and can be determined using permutations because the order matters.
4) Seating Arrangements and Event Planning
A seating plan is a very important element of the planning process regardless of whether you are organizing a theatre performance, a wedding reception, or a conference. Every single individual who is attending the event is directly impacted by how well the seating system works. The number of possibilities created by permutations and combinations makes it easier for the planners to bring each guest to that exact spot where they belong.
5) Fashion and Design
Fashion designers and interior decorators use permutations and combinations to predict trends and create novel designs
Recommended Video Based on Permutations vs Combinations:
Solved Example Based on Permutations Vs Combinations
Example 1: If ${ }^{2 n} C_3:{ }^n C_3: 10: 1$,then the ratio $\left(n^2+3 n\right):\left(n^2-3 n+4\right)$ is : [JEE MAINS 2023]
Solution
$\begin{aligned} & \frac{{ }^{2 n} C_3}{{ }^n C_3}=10 \Rightarrow \frac{2 \mathrm{n}!(\mathrm{n}-3)!}{(2 \mathrm{n}-3)!\mathrm{n}!}=10 \\ & \frac{2 \mathrm{n}(2 \mathrm{n}-1)(2 n-2)}{\mathrm{n}(\mathrm{n}-1)(\mathrm{n}-2)}=10 \\ & \frac{4(2 \mathrm{n}-1)}{\mathrm{n}-2}=10 \Rightarrow 8 \mathrm{n}-4=10 \mathrm{n}-20 \\ & 2 \mathrm{n}=16 \\ & \quad \frac{\mathrm{n}^2+3 \mathrm{n}}{\mathrm{n}^2-3 \mathrm{n}+4} \\ & =\frac{64+24}{64-24+4}=\frac{88}{44}=2\end{aligned}$
Hence, the answer is 2: 1
Example 2: The number of different ways in which five ‘alike dashes’ and eight ‘alike dots’ can be arranged, using only seven of these ‘dashes’ & ‘dots’ is
Solution
The number of ways of filling $n$ distinct objects at $r$ places $=n(n-1)(n-2) \ldots(n-r+1)=$ $=\frac{n(n-1)(n-2) \ldots(n-r+1)(n-r)!}{(n-r)!}=\frac{n!}{(n-r)!}={ }^n p_r=n .^{n-1} p_{r-1}$
Where $r \leq n$ and $r \geq 0$
${ }^7 C_2+{ }^7 C_3+{ }^7 C_4+7 C_5+7 C_6+7 C_7=2^7-8=120$
Hence, the answer is 120
Example 3: All the five-digit numbers $N=a b c d e$ having property $a<b<c<d<e$ are arranged in the increasing order of their magnitude. The $97^{\text {th }}$ number in the list does not contain the digit:
Solution: All the possible numbers are
$
\left.{ }^9 \mathrm{C}_5 \text { (none containing the digit } 0\right)=126
$
Total number starting with 1
$
\left.={ }^8 \mathrm{C}_4=70 \quad \text { (using } 2,3,4,5,6,7,8,9\right)
$
1_ _ _ _ _
Total numbers starting with 23
$={ }^6 \mathrm{C}_3=20 \quad$ (using $\left.4,5,6,7,8,9\right)$
23 _ _ _ _
Total numbers starting with $245={ }^4 C_2=6$ (using $\left.6,7,8,9\right)$
245 _ _ _
$97^{\text {th }}$ number $=24678$
Hence, the answer is 5
Example 4: A seven-digit number is in the form of abcdefg ( $g, f, e, \ldots .$. etc. are digits at units, tens, hundred places....etc), where $a<b<c<d>e>f>g \mathrm{a}, \mathrm{b}, \mathrm{c}, \mathrm{e}, \mathrm{f}, \mathrm{g}$ are different digits. The number of such numbers is:
Solution: Case (i): zero not taken
Now we have to select seven digits from 1,2,3,4,5,6,7,8,9 so ways are ${ }^9 C_7$
From 7 digits select the largest digits as $d$ and from the remaining 6 , we can select three digits $a, b, c$ in ${ }^6 C_3$ ways.
Hence number of such numbers are $={ }^9 \mathrm{C}_7 \cdot{ }^6 \mathrm{C}_3$
Case (ii): zero taken then
0 must be in last place, then the number of such numbers are ${ }^9 \mathrm{C}_6 \cdot{ }^5 \mathrm{C}_3$
$
\begin{aligned}
{ }^9 C_7 \cdot{ }^6 C_3+{ }^9 C_6 \cdot{ }^5 C_3 & ={ }^9 C_2 \cdot{ }^6 C_3+{ }^9 C_3 \cdot C_3 \\
& =1560
\end{aligned}
$
Hence, the answer is 1560
Example 5: A 6-digit number is to be formed using the digits 0-9, where repetition is allowed. How many different numbers can be formed if the number must be divisible by 2 and have exactly 3 even digits?
Solution: To calculate the number of different 6-digit numbers that can be formed using the digits $0-9$, where repetition is allowed, and the number must be divisible by 2 with exactly 3 even digits, we can consider the following:
Since the number must be divisible by 2 , the last digit must be even. We have 5 even digits $(0,2,4,6,8)$ to choose from for the last digit.
For the remaining 5 digits, we need to select 2 even digits and 3 odd digits. We have 5 even digits and 5 odd digits to choose
from, so we can select the 2 even digits in $\mathrm{C}(5,2)$ ways and the 3 odd digits in $\mathrm{C}(5,3)$ ways.
Once we have selected the digits, we can arrange them in the remaining 5 positions in 5 ! ways.
Therefore, the total number of different 6-digit numbers that can be formed with the given conditions is:
$
C(5,2) \times C(5,3) \times 5!\times 5=10 \times 10 \times 120 \times 5=60,000
$
Hence, the answer is 60,000
Also Read
13 Feb'25 06:36 PM
13 Feb'25 06:33 PM
13 Feb'25 06:31 PM
13 Feb'25 06:30 PM
13 Feb'25 06:26 PM
13 Feb'25 06:10 PM
13 Feb'25 05:04 PM
13 Feb'25 04:53 PM
13 Feb'25 04:47 PM
11 Oct'24 10:00 AM
