Properties of Determinants

A determinant is a special number that can be determined from a matrix. For a determinant to exist, matrix A must be a square matrix. The determinant of the matrix is denoted by det A or |A|. In real life, we can use determinant in graphic designing, and gaming. Determinants also help us in taking necessary steps in business.

JEE Main 2025: Sample Papers | Mock Tests | PYQs | Study Plan 100 Days

JEE Main 2025: Maths Formulas  | Study Materials

JEE Main 2025: Syllabus | Preparation Guide | High Scoring Topics 

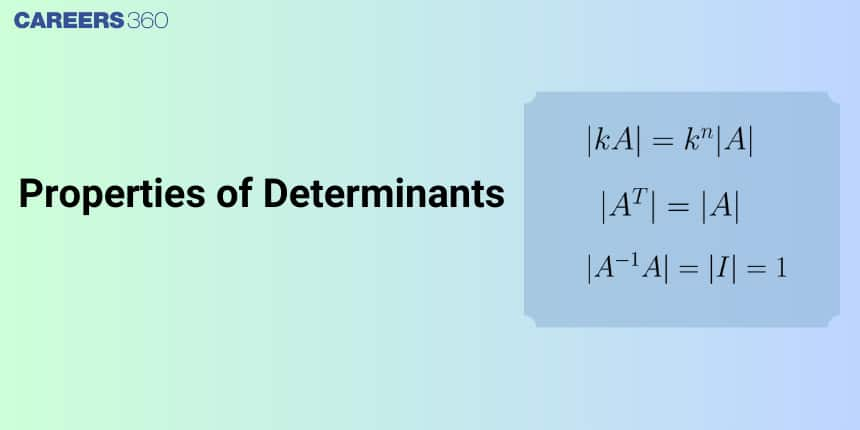
In this article, we will learn the properties of determinants. This category falls under the broader category of matrices, which is a crucial Chapter in class 12 Mathematics. It is not only essential for board exams but also for competitive exams like the Joint Entrance Examination(JEE Main) and other entrance exams such as SRMJEE, BITSAT, WBJEE, BCECE, and more. A total of thirteen question have been asked on this topic including one in 2018, four in 2019, three in 2020, four in 2021, one in 2022, three in 2023.

What are Determinants?

The determinant of a matrix A is a number which is calculated from the matrix. For a determinant to exist, matrix A must be a square matrix. The determinant of the matrix is denoted by det A or |A|.

How to find the Determinant of a Matrix?

For $2 \times 2$ matrices
$
\mathrm{A}=\left[\begin{array}{ll}
a_1 & a_2 \\
b_1 & b_2
\end{array}\right]
$
then $\operatorname{det} \mathrm{A}$ is :
$
|\mathrm{A}|=\left|\begin{array}{ll}
a_1 & a_2 \\
b_1 & b_2
\end{array}\right|=\mathrm{a}_1 \times \mathrm{b}_2-\mathrm{a}_2 \times \mathrm{b}_1
$

For a $3 \times 3$ matrix determinant can be calculated in the following way :
let $\mathrm{A}=\left[\begin{array}{lll}a_1 & a_2 & a_3 \\ b_1 & b_2 & b_3 \\ c_1 & c_2 & c_3\end{array}\right]$
then we find $\operatorname{det} \mathrm{A}$ in following way
$
|A|=a_1\left(b_2 \cdot c_3-b_3 \cdot c_2\right)-a_2\left(b_1 \cdot c_3-c_1 b_3\right)+a_3\left(b_1 c_2-b_2 c_1\right)
$

This same process we follow to evaluate the determinant of the matrix of any order. Notice that we start the first term with the +ve sign then the 2nd with the -ve sign and the 3rd again +ve sign, this sign sequence is followed for any order of matrix.

This whole process is row-dependent, the same process can be done using columns, which means we can select an element along a column delete their row and column compute the determinant of left out matrix, and then multiply it with the element that we select. And we will get the same result as we get while doing the whole process along the row.

Properties of Determinants

Property 1: Interchange Property

The value of the determinant remains unchanged if its rows and columns are interchanged.

For example,

Let, $\Delta=\left|\begin{array}{lll}a_1 & a_2 & a_3 \\ b_1 & b_2 & b_3 \\ c_1 & c_2 & c_3\end{array}\right|$
Expanding along the first row, we get
$
\begin{aligned}
& \Delta=\mathrm{a}_1\left|\begin{array}{ll}
b_2 & b_3 \\
c_2 & c_3
\end{array}\right|-\mathrm{a}_2\left|\begin{array}{ll}
b_1 & b_3 \\
c_1 & c_3
\end{array}\right|+\mathrm{a}_3\left|\begin{array}{ll}
b_1 & b_2 \\
c_1 & c_2
\end{array}\right| \\
& \Delta=\mathrm{a}_1\left(\mathrm{~b}_2 \mathrm{c}_3-\mathrm{b}_3 \mathrm{c}_2\right)-\mathrm{a}_2\left(\mathrm{~b}_1 \mathrm{c}_3-\mathrm{b}_3 \mathrm{c}_1\right)+\mathrm{a}_3\left(\mathrm{~b}_1 \mathrm{c}_2-\mathrm{b}_2 \mathrm{c}_1\right)
\end{aligned}
$

By interchanging the rows and columns of $\Delta$, we get the determinant
$
\Delta^{\prime}=\left|\begin{array}{lll}
a_1 & b_1 & c_1 \\
a_2 & b_2 & c_2 \\
a_3 & b_3 & c_3
\end{array}\right|
$

Expanding $\Delta^{\prime}$ along first column, we get
$
\begin{aligned}
& \Delta^{\prime}=\mathrm{a}_1\left(\mathrm{~b}_2 \mathrm{c}_3-\mathrm{b}_3 \mathrm{c}_2\right)-\mathrm{a}_2\left(\mathrm{~b}_1 \mathrm{c}_3-\mathrm{b}_3 \mathrm{c}_1\right)+\mathrm{a}_3\left(\mathrm{~b}_1 \mathrm{c}_2-\mathrm{b}_2 \mathrm{c}_1\right) \\
& \Delta=\Delta^{\prime}
\end{aligned}
$

Property 2: Switching Property

If any two rows or two columns of a determinant are interchanged, then the sign of the determinant changes but the numerical value remains unaltered.

For example

Let, $\Delta=\left|\begin{array}{lll}a_1 & a_2 & a_3 \\ b_1 & b_2 & b_3 \\ c_1 & c_2 & c_3\end{array}\right|$
Expanding along the first row, we get
$
\begin{aligned}
& \Delta=\mathrm{a}_1\left|\begin{array}{ll}
b_2 & b_3 \\
c_2 & c_3
\end{array}\right|-\mathrm{a}_2\left|\begin{array}{ll}
b_1 & b_3 \\
c_1 & c_3
\end{array}\right|+\mathrm{a}_3\left|\begin{array}{ll}
b_1 & b_2 \\
c_1 & c_2
\end{array}\right| \\
& \Delta=\mathrm{a}_1\left(\mathrm{~b}_2 \mathrm{c}_3-\mathrm{b}_3 \mathrm{c}_2\right)-\mathrm{a}_2\left(\mathrm{~b}_1 \mathrm{c}_3-\mathrm{b}_3 \mathrm{c}_1\right)+\mathrm{a}_3\left(\mathrm{~b}_1 \mathrm{c}_2-\mathrm{b}_2 \mathrm{c}_1\right)
\end{aligned}
$

Interchanging the first and third rows, the new determinant obtained is given by
$
\Delta^{\prime}=\left|\begin{array}{lll}
c_1 & c_2 & c_3 \\
b_1 & b_2 & b_3 \\
a_1 & a_2 & a_3
\end{array}\right|
$

Expanding along the third row, we get
$
\begin{aligned}
\Delta^{\prime} & =a_1\left(c_2 b_3-c_3 b_2\right)-a_2\left(c_1 b_3-c_3 b_1\right)+a_3\left(b_2 c_1-b_1 c_2\right) \\
& =-\left[a_1\left(b_2 c_3-b_3 c_2\right)-a_2\left(b_1 c_3-b_3 c_1\right)+a_3\left(b_1 c_2-b_2 c_1\right)\right] \\
\Delta & =-\Delta^{\prime}
\end{aligned}
$

Property 3: If there is an interchange of rows or columns twice, then the value of the determinant remains the same.

If $\Delta_n$ is the determinant obtained by $\mathrm{n}$ such successive operations, then
$
\Delta_n=\left\{\begin{array}{cc}
-\Delta, & \text { if } \mathrm{n} \text { is odd } \\
\Delta, & \text { if } \mathrm{n} \text { is even }
\end{array}\right.
$

Property 4: Proportionality (Repetition) Property

If any two rows (or columns) of a determinant are identical (all corresponding elements are the same), then the value of the determinant is zero.

For Example,

If we interchange the identical rows (or columns) of the determinant Δ, then by property 2, Δ changes its sign

Let, $\Delta=\left|\begin{array}{lll}a_1 & a_2 & a_3 \\ b_1 & b_2 & b_3 \\ a_1 & a_2 & a_3\end{array}\right|=-\left|\begin{array}{lll}a_1 & a_2 & a_3 \\ b_1 & b_2 & b_3 \\ a_1 & a_2 & a_3\end{array}\right| \quad$ (interchanging row 1 and row 3) $=-\Delta$
[By property 2]
$
\begin{aligned}
2 \Delta & =0 \\
\Delta & =0
\end{aligned}
$

If we interchange the identical rows (or columns) of the determinant Δ, then by property 2, Δ changes its sign

Property 5: Scalar Multiple Property

If each element of a row (or a column) of a determinant is multiplied by a constant k, then the value of the determinant is multiplied by k.

For example

Let, $\Delta=\left|\begin{array}{lll}a_1 & a_2 & a_3 \\ b_1 & b_2 & b_3 \\ c_1 & c_2 & c_3\end{array}\right|$
and $\Delta^{\prime}$ be the determinant obtained by multiplying the elements of the first row by $\mathrm{k}$.
$
\Delta^{\prime}=\left|\begin{array}{ccc}
k a_1 & k a_2 & k a_3 \\
b_1 & b_2 & b_3 \\
c_1 & c_2 & c_3
\end{array}\right|
$

Expanding along the first row, we get
$
\begin{aligned}
\Delta^{\prime} & =\mathrm{ka}_1\left(\mathrm{~b}_2 c_3-b_3 c_2\right)-\mathrm{ka}_2\left(\mathrm{~b}_1 \mathrm{c}_3-\mathrm{b}_3 \mathrm{c}_1\right)+\mathrm{ka}_3\left(\mathrm{~b}_1 \mathrm{c}_2-\mathrm{b}_2 \mathrm{c}_1\right) \\
& =\mathrm{k}\left[\mathrm{a}_1\left(\mathrm{~b}_2 \mathrm{c}_3-\mathrm{b}_3 \mathrm{c}_2\right)-\mathrm{a}_2\left(\mathrm{~b}_1 \mathrm{c}_3-\mathrm{b}_3 \mathrm{c}_1\right)+\mathrm{a}_3\left(\mathrm{~b}_1 \mathrm{c}_2-\mathrm{b}_2 \mathrm{c}_1\right)\right] \\
\Delta^{\prime} & =\mathrm{k} \Delta
\end{aligned}
$

Hence,
$
\left|\begin{array}{ccc}
k a_1 & k a_2 & k a_3 \\
b_1 & b_2 & b_3 \\
c_1 & c_2 & c_3
\end{array}\right|=\mathrm{k}\left|\begin{array}{lll}
a_1 & a_2 & a_3 \\
b_1 & b_2 & b_3 \\
c_1 & c_2 & c_3
\end{array}\right|
$

Note:

1. By this property, we can take out any common factor from any one row or any one column of a given determinant.
2. If the corresponding elements of any two rows (or columns) of a determinant are proportional (in the same ratio), then the determinant value is zero.

Property 6: Sum Property

If every element of some row or column of a determinant is expressed as the sum of two (or more) terms, then the determinant can be expressed as the sum of two (or more) determinants

For example

$
\left|\begin{array}{ccc}
a_1+x & a_2+y & a_3+z \\
b_1 & b_2 & b_3 \\
c_1 & c_2 & c_3
\end{array}\right|=\left|\begin{array}{ccc}
a_1 & a_2 & a_3 \\
b_1 & b_2 & b_3 \\
c_1 & c_2 & c_3
\end{array}\right|+\left|\begin{array}{ccc}
x & y & z \\
b_1 & b_2 & b_3 \\
c_1 & c_2 & c_3
\end{array}\right|
$

Proof:
$
\mathrm{LHS}=\left|\begin{array}{ccc}
a_1+x & a_2+y & a_3+z \\
b_1 & b_2 & b_3 \\
c_1 & c_2 & c_3
\end{array}\right|
$

Expanding along the first row, we get
$
\begin{aligned}
& \Delta=\left(\mathrm{a}_1+\mathrm{x}\right)\left(\mathrm{b}_2 \mathrm{c}_3-\mathrm{b}_3 \mathrm{c}_2\right)-\left(\mathrm{a}_2+\mathrm{y}\right)\left(\mathrm{b}_1 \mathrm{c}_3-\mathrm{b}_3 \mathrm{c}_1\right)+\left(\mathrm{a}_3+\mathrm{z}\right)\left(\mathrm{b}_1 \mathrm{c}_2-\mathrm{b}_2 \mathrm{c}_1\right) \\
& =\mathrm{a}_1\left(\mathrm{~b}_2 \mathrm{c}_3-\mathrm{b}_3 \mathrm{c}_2\right)-\mathrm{a}_2\left(\mathrm{~b}_1 \mathrm{c}_3-\mathrm{b}_3 \mathrm{c}_1\right)+\mathrm{a}_3\left(\mathrm{~b}_1 \mathrm{c}_2-\mathrm{b}_2 \mathrm{c}_1\right) \\
& \quad+\mathrm{x}\left(\mathrm{b}_2 \mathrm{c}_3-\mathrm{b}_3 \mathrm{c}_2\right)-\mathrm{y}\left(\mathrm{b}_1 \mathrm{c}_3-\mathrm{b}_3 \mathrm{c}_1\right)+\mathrm{z}\left(\mathrm{b}_1 \mathrm{c}_2-\mathrm{b}_2 \mathrm{c}_1\right) \\
& =\left|\begin{array}{lll}
a_1 & a_2 & a_3 \\
b_1 & b_2 & b_3 \\
c_1 & c_2 & c_3
\end{array}\right|+\left|\begin{array}{ccc}
x & y & z \\
b_1 & b_2 & b_3 \\
c_1 & c_2 & c_3
\end{array}\right|
\end{aligned}
$

Property 7: Property of Invariance

If to each element of any row or column of a determinant, the equimultiples of corresponding elements of other rows (or columns) are added, then the value of the determinant remains the same, i.e., the value of the determinant remains the same if we apply the operation

$
\mathrm{R}_{\mathrm{i}} \rightarrow \mathrm{R}_{\mathrm{i}}+\mathrm{kR}_{\mathrm{j}} \text { or } \mathrm{C}_{\mathrm{i}} \rightarrow \mathrm{C}_{\mathrm{i}}+\mathrm{kC}_{\mathrm{j}}
$

Explanation,
Let, $\Delta=\left|\begin{array}{lll}a_1 & a_2 & a_3 \\ b_1 & b_2 & b_3 \\ c_1 & c_2 & c_3\end{array}\right|$ and $\Delta^{\prime}=\left|\begin{array}{ccc}a_1+k c_1 & a_2+k c_2 & a_3+k c_3 \\ b_1 & b_2 & b_3 \\ c_1 & c_2 & c_3\end{array}\right|$ Here, $\Delta^{\prime}$ is obtained by $R_1 \rightarrow R_1+k R_3$
we can write $\Delta^{\prime}$ as
$
\begin{aligned}
& \text { we can write } \Delta^{\prime} \text { as } \\
& \begin{aligned}
\Delta^{\prime} & =\left|\begin{array}{lll}
a_1 & a_2 & a_3 \\
b_1 & b_2 & b_3 \\
c_1 & c_2 & c_3
\end{array}\right|+\left|\begin{array}{ccc}
k c_1 & k c_2 & k c_3 \\
b_1 & b_2 & b_3 \\
c_1 & c_2 & c_3
\end{array}\right| \\
& =\left|\begin{array}{lll}
a_1 & a_2 & a_3 \\
b_1 & b_2 & b_3 \\
c_1 & c_2 & c_3
\end{array}\right|+\mathrm{k}\left|\begin{array}{lll}
c_1 & c_2 & c_3 \\
b_1 & b_2 & b_3 \\
c_1 & c_2 & c_3
\end{array}\right| \\
& =\Delta+\mathrm{k} \cdot 0
\end{aligned}
\end{aligned}
$
hence, $\Delta^{\prime}=\Delta$

Property 8: Triangle Property

If each element of a determinant above or below one the principal diagonal of a determinant is zero, then the value of the determinant is the product of the diagonal elements.

I.e.

$\left|\begin{array}{lll}a & f & g \\ 0 & b & h \\ 0 & 0 & c\end{array}\right|=\left|\begin{array}{lll}a & 0 & 0 \\ f & b & 0 \\ g & h & c\end{array}\right|=\left|\begin{array}{lll}a & 0 & 0 \\ 0 & b & 0 \\ 0 & 0 & c\end{array}\right|=\mathrm{abc}$

Property 9: Factor Property

If a determinant D becomes 0 for x = α, then (x - α) is a factor of Δ.

For example,

If $\Delta=\left|\begin{array}{ccc}x & x^2 & x^3 \\ 4 & 16 & 64 \\ 5 & 9 & 11\end{array}\right|$
When, $\mathrm{x}=4$ the value of $\Delta$ becomes 0 $\because$ at $\mathrm{x}=4, \mathrm{R}_1$ and $\mathrm{R}_2$ are identical. and at $\mathrm{x}=0, \Delta=0$, because all element of $\mathrm{R}_1$ becomes 0 hence, $(x-0)$ and $(x-4)$ are the factors of $\Delta$.

Property 10: All-zero Property

If all the elements of a row or column are zero, then the determinant is zero.

Singular and non-singular matrix:

A square matrix is called a singular matrix if its determinant is 0 otherwise it is called a non-singular matrix. Let's say A is a square matrix then it is singular if |A| = 0, otherwise, it will be non-singular if |A| ≠ 0.

Summary

Knowing about determinants and their properties is very crucial as it helps us know the whether inverse of the matrix exists or not. It also helps us to find the value of determinants in simpler ways. The properties of determinants offer powerful tools in linear algebra for analyzing systems of equations, transformations, and geometric interpretations.

Recommended Video :

Solved Examples Based on Properties of Determinant

Example 1: Let $\mathrm{P}$ and $\mathrm{p}+2$ be prime numbers and let $
\Delta=\left|\begin{array}{ccc}
\mathrm{p}! & (\mathrm{p}+1)! & (\mathrm{p}+2)! \\
(\mathrm{p}+1)! & (\mathrm{p}+2)! & (\mathrm{p}+3)! \\
(\mathrm{p}+2)! & (\mathrm{p}+3)! & (\mathrm{p}+4)!
\end{array}\right|
$. Then the sum of the maximum values of $\alpha$ and $\beta$ such that $\mathrm{P}^\alpha$ and $(\mathrm{p}+2)^\beta$ divide $\Delta$, is
[JEE MAINS 2022]

Solution:
$
\begin{aligned}
& \Delta=\left|\begin{array}{lll}
\mathrm{P}! & (\mathrm{P}+1)! & (\mathrm{P}+2)! \\
(\mathrm{P}+1)! & (\mathrm{P}+2)! & (\mathrm{P}+3)! \\
(\mathrm{P}+2)! & (\mathrm{P}+3)! & (\mathrm{P}+4)!
\end{array}\right| \\
& \Delta=\mathrm{P})(\mathrm{P}+1)!(\mathrm{P}+2)!\left|\begin{array}{lll}
1 & 1 & 1 \\
\mathrm{P}+1 & \mathrm{P}+2 & \mathrm{P}+3 \\
(\mathrm{P}+2)(\mathrm{P}+1) & (\mathrm{P}+3)(\mathrm{P}+2) & (\mathrm{P}+4)(\mathrm{P}+3)
\end{array}\right| \\
& \Delta=2 \mathrm{P}!(\mathrm{P}+1)!(\mathrm{P}+2)!
\end{aligned}
$
which is divisible by $\mathrm{p}^\alpha \&(\mathrm{p}+2)^\beta$
$
\therefore \alpha=3, \beta=1
$

Hence, the required answer is 4

Example 2: If $\left[\begin{array}{ccc}x-4 & 2 x & 2 x \\ 2 x & x-4 & 2 x \\ 2 x & 2 x & x-4\end{array}\right]=(A+B x)(x-A)^2 \quad$ then the ordered pair $(A, B)$ is equal to :
[JEE MAINS 2018]

Solution:

Property of determinant

If a determinant becomes 0 for $x=a$, then $(x-a)$ is a factor of $D$, in other words, if two rows ( or two columns ) become identical for $x=a$, Then $(x-a)$ is a factor of $D$
we can put values of $x=0$ in both the sides $\left[\begin{array}{ccc}-4 & 0 & 0 \\ 0 & -4 & 0 \\ 0 & 0 & -4\end{array}\right]=A\left(-A^2\right)$
$
(-4)^3=A^3 \Rightarrow A=-4
or $x=4$
$
\begin{aligned}
& {\left[\begin{array}{lll}
0 & 8 & 8 \\
8 & 0 & 8 \\
8 & 8 & 0
\end{array}\right]=(A+4 B)(4-A)^2} \\
& -8\left(-8^2\right)+8\left(8^2\right)=(4 B-4)\left(8^2\right)=16 \times 8^2=(4 B-4) 8^2 \\
& B=5
\end{aligned}

(-4,5)

Hence, the required answer is (-4,5)

Example 3: Let $A=\left[a_{i j}\right]$ and $B=\left[b_{i j}\right]$ be two $3 \times 3$ real matrices such that $b_{i j}=(3)^{(i+j-2)} a_{j i}$, where, $\mathrm{i}, \mathrm{j}=1,2,3$. if the determinant of $\mathrm{B}$ is 81 , then the determinant of $\mathrm{A}$ is :
[JEE MAINS 2020]

Solution:
$
\begin{aligned}
& |B|=\left|\begin{array}{lll}
b_{11} & b_{12} & b_{13} \\
b_{21} & b_{22} & b_{23} \\
b_{31} & b_{32} & b_{33}
\end{array}\right| \\
& |B|=\left|\begin{array}{lll}
3^0 a_{11} & 3^1 a_{12} & 3^2 a_{13} \\
3^1 a_{21} & 3^2 a_{22} & 3^3 a_{23} \\
3^2 a_{31} & 3^3 a_{32} & 3^4 a_{33}
\end{array}\right|
\end{aligned}
$

Taking Common $3^2$ from $R_3$ and 3 from $R_2$
$
|B|=3^3\left|\begin{array}{ccc}
3^0 a_{11} & 3^1 a_{12} & 3^2 a_{13} \\
3^0 a_{21} & 3^1 a_{22} & 3^2 a_{23} \\
3^0 a_{31} & 3^1 a_{32} & 3^2 a_{33}
\end{array}\right|
$

Taking Common $3^2$ from $C_3$ and 3 from $C_2$
$
\Rightarrow 81=3^3 \cdot 3 \cdot 3^2|\mathrm{~A}| \Rightarrow 3^4=3^6|\mathrm{~A}| \Rightarrow|\mathrm{A}|=\frac{1}{9}
$

Hence, the required answer is $\frac{1}{9}$

Example 4: Let $
\mathrm{A}=\left(\begin{array}{ccc}
{[x+1]} & {[x+2]} & {[x+3]} \\
{[x]} & {[x+3]} & {[x+3]} \\
{[x]} & {[x+2]} & {[x+4]}
\end{array}\right)
$ where $[t]$ denotes the greatest integer less than or equal to $t$. If $\operatorname{det}(\mathrm{A})=192$, then the set of values of $x$ is the interval :
[JEE MAINS 2023]

Solution:
We know that $[x+I]=[x]+I$ for $I \in$ Integer
$
\begin{aligned}
& \operatorname{det}(A)=\left|\begin{array}{ccc}
{[x]+1} & {[x]+2} & {[x]+3} \\
{[x]} & {[x]+3} & {[x]+3} \\
{[x]} & {[x]+2} & {[x]+4}
\end{array}\right|=192 \\
& R_2 \rightarrow R_2-R_1, R_3 \rightarrow R_3-R_2 \\
& \operatorname{det}(A)=\left|\begin{array}{ccc}
{[x]+1} & {[x]+2} & {[x]+3} \\
-1 & 1 & 0 \\
0 & -1 & 1
\end{array}\right|=192 \\
& \Rightarrow([x]+1)(1-0)-([x]+2)(-1-0)+([x]+3)(1-0)=192 \\
& \Rightarrow 3[x]+6=192 \Rightarrow 3[x]=186 \\
& \Rightarrow[x]=62 \Rightarrow x \in[62,63)
\end{aligned}
$

Hence, the required answer is $[62,63)$

Example 5: If $\left|\begin{array}{ccc}x+1 & x & x \\ x & x+\lambda & x \\ x & x & x+\lambda^2\end{array}\right|=\frac{9}{8}(103 x+81)$,then $\lambda, \frac{\lambda}{3}$ are the roots of the equation
[JEE MAINS 2023]

Solution:
$
\begin{aligned}
& \left|\begin{array}{ccc}
x+1 & x & x \\
x & x+d & x \\
x & x & x+d^2
\end{array}\right|=\frac{9}{8}(103 x+81) \\
& \text { Put } \mathrm{x}=0 \\
& \left|\begin{array}{ccc}
1 & 0 & 0 \\
0 & \lambda & 0 \\
0 & 0 & \lambda^2
\end{array}\right|=\frac{9}{8} \times 81 \\
& \lambda^3=\frac{9^3}{8} \\
& \lambda=\frac{9}{2} \\
& \frac{\lambda}{3}=\frac{9}{2 \times 3} \Rightarrow \frac{3}{2} \\
& \frac{\lambda}{3}=\frac{3}{2} \\
& 4 x^2-24 x+27=0
\end{aligned}
$

Hence, the required answer is $\frac{3}{2}, \frac{9}{2}$