Straight Lines - Definition, Equations, Properties and Examples
Imagine drawing a road map or planning the path of a bridge — the paths you draw can be represented by straight lines in mathematics. Understanding straight line equations helps in calculating the slope of a straight line, finding the distance between two points on a line, and determining the angle between two straight lines. For students preparing for exams like JEE, mastering the equation of straight line in various forms, including point-slope form of a straight line, is crucial. Knowing the important formulas for straight lines makes solving problems faster and more accurate. In this article, we will explore all key concepts, formulas, and applications of straight lines for easy learning and exam preparation in mathematics.
This Story also Contains
- What is a Straight Line?
- Types of Straight Lines
- Slope of a Straight Line
- Types of Slopes
- Equation of a straight line
- Angle Between Two Straight Lines
- Important Formulae for Straight Lines
- List of Topics related to straight lines according to NCERT/JEE MAIN
- Important Books and Resources for Straight Lines
- Practice Questions based on Straight Lines
What is a Straight Line?
A straight line is an infinite length extending in both directions. Every equation of the first degree in $x, \text{and} \space y$ represents a straight line. The general equation of a straight line is given as $ax+b y+c=0$ where $a, b,$ and $c$ are real numbers and at least one of $a$ and $b$ is non-zero.
Types of Straight Lines
The types of straight lines based on its position and relation with other lines are mentioned below:
Types of Straight Lines | Description | Illustration |
Horizontal Line | Lines that extend from left to right or right to left horizontally are called horizontal lines. Horizontal lines are parallel to the $x $-axis and perpendicular to the $y$-axis. |
|
Vertical Line | Lines that extend from top to bottom or bottom to top vertically are called vertical lines. Vertical lines are perpendicular to $x$-axis and parallel to $y$-axis. |
|
Oblique or Slant Line | Lines that are drawn slantingly are called oblique lines. Obliques lines are also called slanting lines. |
|
Line Segment | The infinite length extending in both directions between two points is called a line segment. |
|
Ray | A line starting from one point and extending infinitely in the other direction is called a ray. |
|
Parallel Lines | Lines that extend on either side without meeting each other with a constant distance are called parallel lines. |
|
Intersecting Lines | Lines that pass through each other are called the intersecting lines. The point at which the lines pass is called the point of intersection. |
|
Perpendicular Lines | Two lines intersecting each other at an angle of $90^\circ$ is called a perpendicular line. |
|
Properties of Straight Lines
The properties of straight lines include,
The length of the straight line is infinite
A straight line gives the shortest distance between two points
Straight lines do not have any curves or bends.
Straight lines are one-dimensional
A straight line does not have area or volume
Slope of a Straight Line
In mathematics, the change of the $y$-axis coordinate with respect to the change of the $x$-axis coordinate is called the Slope of a line. It is the ratio of vertical change to horizontal change between two points on a line. It measures the steepness and direction of the line. The slope of a straight line is denoted by $m$.
The slope of a line with given two points $(x_1,y_1)$ and $(x_2,y_2)$ is $m = \frac{y_2-y_1}{x_2-x_1}$
The slope of a line with a given angle $\theta$ is $ m = \tan \theta $
The slope of a line from the equation of the line of the form $y=mx+c$ is the coefficient of the variable $x$, which is $m$.
Types of Slopes
Slope of a straight line can be of different types.
Uphill slopes
Downhill slopes
Horizontal slopes
Vertical slopes
Uphill Slopes
The slope of a straight line extending upwards from left to right is called the uphill slope. It is also called a positive slope as the calculated slope will yield a positive value. In this type of slope, when the value of $x$ increases, the value of $y$ also increases.
Downhill Slope
The slope of the straight line extending downwards from left to right is called the downhill slope. It is also called a negative slope as the calculated slope will yield a negative value. In this type of slope, when the value of $x$ increases, the value of $y$ decreases.
Horizontal Slope
The slope of a horizontal line is called the horizontal slope. This is also called a zero slope as the calculated slope will yield the value $0$. In this type of slope, when the value of $x$ increases, the value of $y$ remains the same. That is, the line remains flat.
Vertical Slope
The slope of a vertical line is called the vertical slope. This is also called an infinite slope as the slope is undefined. In this type of slope, when the value of $y$ increases, the value of $x$ remains the same. That is, the line will be straight up or down.
Notes:
The slope of a horizontal line is $0$
The slope of a vertical line is undefined
The slope of parallel lines $l_1$ and $l_2$ with slopes $m_1$ and $m_2$ is equal (i.e) $m_1 = m_2$
The product of the slope of two perpendicular lines $l_1$ and $l_2$ with slopes $m_1$ and $m_2$ is $1$ (i.e) $m_1.m_2 = 1$
Equation of a straight line
The equation of straight line in various forms are point-slope form, slope-intercept form, standard form, etc. These forms help us understand how to find the equation of a straight line with the given information. Let’s discuss each form in detail.
Equation of Horizontal and Vertical lines
Horizontal Line: A horizontal line has a slope of zero. Its equation is of the form $y = c$, where $c$ is the $y$-coordinate of any point on the line.
Vertical Line: A vertical line has an undefined slope. Its equation is of the form $x = a$, where $a$ is the $x$-coordinate of any point on the line.
Example: $y=2$ is a horizontal line and $x=4$ is a vertical line.

Point-slope form of Straight line
The point-slope form is used to determine the equation of a straight line passing through a point $(x_1,y_1)$ and having slope $m$. The point-slope form of straight line is given by $y-y_1=m(x-x_1)$

Example: The straight line equations with $m = 2$ and passing through $(3,4)$ is
$(y-4)=2(x-3)$ ⇒ $2x-y=2$
Two points form of a line
The straight line equations passing through $(x_1,y_1)$ and $(x_2,y_2)$ is given by
$\frac{x-x_1}{x_1-x_2}=\frac{y-y_1}{y_1-y_2}$

Example: The equation of a line passing through $(1,2)$ and $(3,5)$ is
$\frac{x-1}{1-2}=\frac{y-3}{3-5}$
⇒ $2x-2=y-3$
⇒ $2x-y+1=0$
Slope intercept form of a line
If a line is given with its slope m and its $y$-intercept. Say, a line intersects the $y$-axis at the point $(0, c)$. Using the point-slope form of straight line, we have $y - c = m (x - 0) ⇒ y = mx + c$, where $c$ is the $ y$-intercept. This is called the slope-intercept form of a line.

Example: The equation of a line with slope $m=3$ and $y$-intercept $4$ is $y=3x+4$.
Intercept form of a line
The intercept form is used when the intercepts on the $x$-axis and $y$-axis are known. It is given by: $\frac{x}{a}+\frac{y}{b}=1$, where $a$ is the $x$-intercept and $b$ is the $y$-intercept.
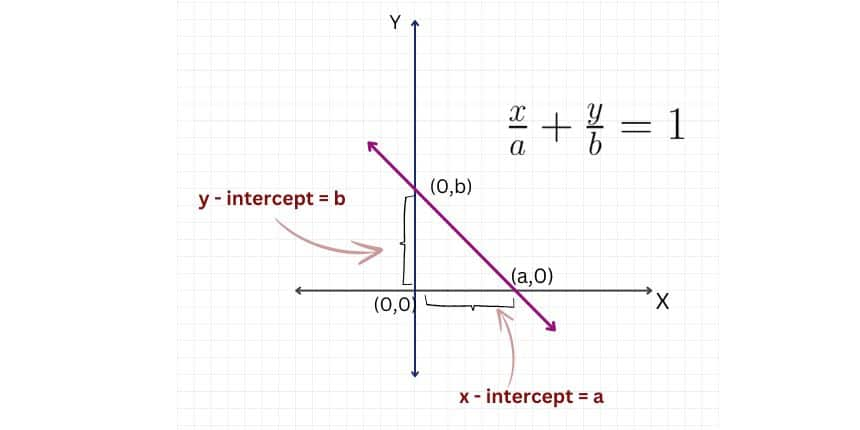
Example: For $x$-intercept as $4$ and $y$-intercept as $5$, the equation of line becomes $\frac{x}{4}+\frac{y}{5}=1$
The normal form of a line
The normal form of a line’s equation involves the perpendicular (normal) distance from the origin to the line. It is given by: $x\cos\alpha + y\sin\alpha=p$
Where $p$ is the length of the perpendicular from the origin to the line, and $\alpha$ is the angle between this perpendicular and the positive $x$-axis.

Example: For $p=5$ and $\alpha=30°$, the equation of the line becomes
$x\cos 30° + y\sin 30° = 5$ ⇒ $\sqrt{3}x+y=10$
Standard form of a line
The standard form of a straight line is given by $ax + by + c = 0$, where $a, b, c$ are real numbers.
Example: The equation of a line in standard form is $2x + 3y + 5 = 0$.
Angle Between Two Straight Lines
The angle($θ$) between two lines with slopes $m_1$ and $m_2$ can be found by using the formula:
$\tan θ = \frac{m_1-m_2}{1+m_1m_2}$
If the value of $\frac{m_1-m_2}{1+m_1m_2}$ is positive, then the angle between the lines is acute.
If the value of $\frac{m_1-m_2}{1+m_1m_2}$ is negative, then the angle between the lines is obtuse.
Distance between Two Points on a Line
The distance between two points on a line $(x_1,y_1)$ and $(x_2,y_2)$ is $d=\sqrt{\left(x_2-x_1\right)^2+\left(y_2-y_1\right)^2}$
Important Formulae for Straight Lines
This section provides all the important formulae for straight lines in one place, including slope, distance, angle, and various forms of line equations.
| Concept | Formula | Description |
|---|---|---|
| General equation of a line | $ax+by+c=0$ | $a,b,c \in \mathbb{R}$, not both $a$ and $b$ zero |
| Slope of a line | $m=-\dfrac{a}{b}$ (from $ax+by+c=0$) | If $b \neq 0$ |
| Slope between two points | $m=\dfrac{y_2-y_1}{x_2-x_1}$ | Provided $x_1 \neq x_2$ |
| Slope-intercept form | $y=mx+c$ | $m$ = slope, $c$ = y-intercept |
| Point-slope form | $y-y_1=m(x-x_1)$ | Line through $(x_1,y_1)$ with slope $m$ |
| Two-point form | $y-y_1=\dfrac{y_2-y_1}{x_2-x_1}(x-x_1)$ | Line through $(x_1,y_1)$ and $(x_2,y_2)$ |
| Intercept form | $\dfrac{x}{a}+\dfrac{y}{b}=1$ | $a,b$ = x- and y-intercepts |
| Normal form | $x\cos\alpha+y\sin\alpha=p$ | $p$ = perpendicular distance from origin, $\alpha$ = angle with x-axis |
| Condition for parallel lines | Slopes equal, $m_1=m_2$ | |
| Condition for perpendicular lines | $m_1 \cdot m_2=-1$ | Product of slopes = $-1$ |
| Equation of line parallel to given line $ax+by+c=0$ through $(x_1,y_1)$ | $ax+by+d=0$ | Use same $a,b$, find $d$ with point substitution |
| Equation of line perpendicular to $ax+by+c=0$ through $(x_1,y_1)$ | $bx-ay+d=0$ | Swap coefficients with sign change |
| Equation of angle bisectors of lines $a_1x+b_1y+c_1=0$, $a_2x+b_2y+c_2=0$ | $\dfrac{a_1x+b_1y+c_1}{\sqrt{a_1^2+b_1^2}}=\pm \dfrac{a_2x+b_2y+c_2}{\sqrt{a_2^2+b_2^2}}$ | Gives both bisectors |
List of Topics related to straight lines according to NCERT/JEE MAIN
This section outlines the key topics under straight lines, as per NCERT and JEE Main syllabus. These topics will help you gain better conceptual clarity.
Important Books and Resources for Straight Lines
Here you will find recommended books and resources that explain straight line concepts, equations, and problem-solving techniques with clarity.
| Book Title | Author / Publisher | Description |
|---|---|---|
| NCERT Mathematics Class 11 | NCERT | Official textbook with thorough coverage of straight lines topic. |
| Mathematics for Class 11 | R.D. Sharma | Clear explanations and extensive solved examples on straight lines. |
| Objective Mathematics | R.S. Aggarwal | Comprehensive MCQs and practice problems on straight lines. |
| Arihant All-In-One Mathematics | Arihant | Complete topic-wise coverage including straight lines. |
| Coordinate Geometry | S.L. Loney | Classic book with challenging problems on straight lines. |
NCERT Resources
This section highlights the NCERT textbook chapters that introduce straight lines and build conceptual understanding for Class 11 and competitive exams.
NCERT Maths Notes for Class 11th Chapter 10 - Straight Lines
NCERT Maths Solutions for Class 11th Chapter 10 - Straight Lines
NCERT Maths Exemplar Solutions for Class 11th Chapter 10 - Straight Lines
NCERT Subjectwise Resources
This section provides NCERT exemplar solutions, detailed notes, and step-by-step solutions to strengthen your preparation.
Practice Questions based on Straight Lines
Here you will get a collection of practice problems to apply straight line formulas, improve accuracy, and boost exam readiness.
Frequently Asked Questions (FAQs)
The general equation of a straight line in a plane is $ax + by + c = 0$, where $a$, $b$, and $c$ are real constants and not both $a$ and $b$ are zero.
The slope of a line represents its steepness and is given by $m = \frac{y_2 - y_1}{x_2 - x_1}$ if two points on the line are $(x_1, y_1)$ and $(x_2, y_2)$.
The main forms are:
Slope-intercept form: $y = mx + c$
Point-slope form: $y - y_1 = m(x - x_1)$
Two-point form: $y - y_1 = \frac{y_2 - y_1}{x_2 - x_1}(x - x_1)$
Intercept form: $\frac{x}{a} + \frac{y}{b} = 1$
General form: $ax + by + c = 0$
Two lines are parallel if their slopes are equal ($m_1 = m_2$).
Two lines are perpendicular if the product of their slopes is $-1$ ($m_1 \cdot m_2 = -1$).
The slope of a straight line can be calculated using the formulas,
- The slope of a line with given two points $(x_1,y_1)$ and $(x_2,y_2)$ is $m = \frac{y_2-y_1}{x_2-x_1}$
- The slope of a line with a given angle $\theta$ is $ m = \tan \theta $
- The slope of a line from the equation of the line of the form $y=mx+c$ is the coefficient of the variable $x$, which is $m$.
.png)
.png)





