Sum of Binomial Coefficients

The Sum of Binomial Coefficient is an important concept of algebra that helps to expand the expressions. A Binomial is an expression with two terms. It is difficult to solve the powers manually therefore this expression makes it simpler to solve. This theorem is widely used in real-life applications in mathematics including calculus etc.

JEE Main 2025: Sample Papers | Mock Tests | PYQs | Study Plan 100 Days

JEE Main 2025: Maths Formulas  | Study Materials

JEE Main 2025: Syllabus | Preparation Guide | High Scoring Topics 

Binomial Expression:

An algebraic expression consisting of only two terms is called a Binomial Expression

$
e g \cdot(a+b)^2,\left(\sqrt{x}+\frac{k}{x^2}\right)^5,(x+9 y)^{-2 / 3}
$

If we wanted to expand $(x+y)^{52}$, we might multiply $(x+y)$ by itself fifty-two times. This could take hours!
But if we examine some simple expansions, we can find patterns that will lead us to a shortcut for finding more complicated binomial expansions.

$
\begin{aligned}
& (x+y)^2=x^2+2 x y+y^2 \\
& (x+y)^3=x^3+3 x^2 y+3 x y^2+y^3 \\
& (x+y)^4=x^4+4 x^3 y+6 x^2 y^2+4 x y^3+y^4
\end{aligned}
$

On examining the exponents, we find that with each successive term, the exponent for x decreases by 1 and the exponent for y increases by $1$ . The sum of the two exponents is $n$ for each term.
Also the coefficients for $(x+y)^n$ are equal to $\binom{n}{0},\binom{n}{1},\binom{n}{2}, \ldots,\binom{n}{n}$ where, $\binom{n}{r}=C(n, r)={ }^n C_r=\frac{n!}{r!(n-r)!}$

These patterns lead us to the Binomial Theorem, which can be used to expand any binomial expression.

Binomial Theorem

If $n$ is any positive integer, then

$ (a + b)^n = \binom{n}{0} a^n + \binom{n}{1} a^{n - 1} b + \binom{n}{2} a^{n - 2} b^2 + \dots + \binom{n}{n - 1} a b^{n - 1} + \binom{n}{n} b^n $

Binomial Coefficient

The combination $\binom{n}{r}$ or ${ }^{\mathrm{n}} \mathrm{C}_{\mathrm{r}}$ occuring in the Binomial theorem is called a Binomial coefficient, where $\binom{n}{r}=C(n, r)={ }^n C_r=\frac{n!}{r!(n-r)!}$.

Some Standard Expansions on Sum of Binomial Coefficients:

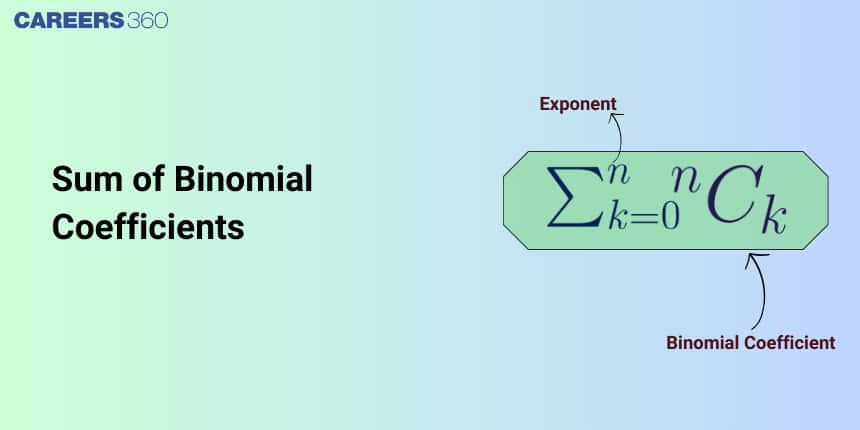
1. Sum of Binomial Coefficients

$
\begin{aligned}
& C_0+\mathrm{C}_1+C_2+C_3+\ldots \ldots+C_n=2^n \\
& \text { or } \sum_{r=0}^n{ }^n C_r=2^n
\end{aligned}
$

2. Sum of Binomial coefficients with alternate sign

The sim of Binomial coefficeints with alternate sign are zero as the terms $T_k$ and $T_{n-k}$ are equal.

$
\begin{aligned}
& C_0-\mathrm{C}_1+C_2-C_3+\ldots \ldots+(-1)^n C_n=0 \\
& \text { or } \quad \sum_{r=0}^n(-1)^r{ }^n C_r=0
\end{aligned}
$

3. Sum of the Binomial coefficients of the odd terms / Sum of the Binomial coefficients of the even terms

The sum of the binomial coefficients of the odd terms = Sum of the binomial coefficients of the even terms

$
\text { I.e. } C_1+C_3+C_5 \ldots \ldots=C_0+C_2+C_4+\ldots \ldots=2^{n-1}
$
solve and analyze real-life complex problems.

Recommended Video Based on Sum of Binomial Coefficients:

Solved Examples Based on Sum of Binomial Coefficients

Example 1: Let $S_n=1+q+q^2+\ldots \ldots \ldots+q^n$ and $T_n=1+\left(\frac{q+1}{2}\right)+\left(\frac{q+1}{2}\right)^2+\ldots \ldots \ldots \ldots+\left(\frac{q+1}{2}\right)^n$ where q is a real numer and $q \neq 1$ ${ }_{\text {If }}{ }^{101} C_1+{ }^{101} C_2 \cdot S_1+\ldots \ldots . .+{ }^{101} C_{101} \cdot S_{100}=\alpha T_{100}$ then $\alpha$ is equal to :
1) 200
2) $2^{100}$
3) 2021
4) $2^{99}$

Solution
Sum of Binomial Coefficients

$
C_0+C_1+C_2+C_3+----+C_n=2^n
$
$\begin{aligned}
&T_n=1+\left(\frac{q+1}{2}\right)+\left(\frac{q+1}{2}\right)^2+\cdots+\left(\frac{q+1}{2}\right)^n=\frac{\left(\frac{q+1}{2}\right)^{n+1}-1}{\left(\frac{q+1}{2}\right)-1}\\
&\text { Also, given, }\\
& { }^{101} \mathrm{C}_1+{ }^{101} \mathrm{C}_2 S_1+\ldots \ldots \ldots \ldots+{ }^{101} \mathrm{C}_{101} S_{100}=\alpha T_{100} \\
& \alpha T_{100}=\sum_{r=1}^{101}{ }^{101} C_r \cdot S_{r-1} \\
& \alpha T_{100}=\sum_{r=1}^{101}{ }^{101} C_r\left[\frac{q^r-1}{q-1}\right] \\
& \alpha T_{100}=\frac{1}{q-1}\left(\sum_{r=1}^{101}{ }^{101} C_r\left(q^r-1\right)\right) \\
& \alpha T_{100}=\frac{1}{q-1}\left((1+q)^{101}-1-\left(2^{101}-1\right)\right) \\
& \alpha T_{100}=\frac{1}{q-1}\left((1+q)^{101}-2^{101}\right)
\end{aligned}$

$\begin{aligned}
& \alpha\left(\frac{\left(\frac{q+1}{2}\right)^{101}-1}{\left(\frac{q+1}{2}\right)-1}\right)=\frac{(1+q)^{101}-2^{101}}{q-1} \\
& \frac{2}{2^{101}} \alpha\left(\frac{(q+1)^{101}-2^{101}}{(q+1)-2}\right)=\frac{(1+q)^{101}-2^{101}}{q-1} \\
& \alpha\left(\frac{1}{2^{100}}\right)=1 \\
& \alpha=2^{100}
\end{aligned}$

Example 2: If the number of terms in the expansion of $\left(1-\frac{2}{x}+\frac{4}{y}\right)^n, x, y \neq 0$ is 28 , then the sum of the coefficients of all the terms in this expansion is
1) $64$
2) $2187$
3) $243$
4) $729$

Solution:

$
\left(1-\frac{2}{x}+\frac{4}{y}\right)^n
$
Using multinomial theorem, number of terms ${ }^{n+2} C_2=28$

$
\begin{aligned}
& \frac{(n+2)!}{2!((n+2)-2)!}=28 \\
& \frac{(n+2)(n+1) n!}{2!n!}=28 \\
& (n+2)(n+1)=56
\end{aligned}
$

Thus $n=6$
So, in $\left(1-\frac{2}{x}+\frac{4}{y}\right)^6$
Put $x=1, y=1$
We get sum of coefficients $=3^6=729$

Example 3: If the fractional part of the number $\frac{2^{403}}{15}$ is $\frac{k}{15}$, then k is equal to:
1) $6$
2) $ 8$
3) $4$
4) $14$

Solution
Sum of Binomial Coefficients

$
C_0+C_1+C_2+C_3+----+C_n=2^n
$
Now,
$2^{403}$ can be written as

$
2^{403}=2^3 \cdot 2^{400}=8\left(2^4\right)^{100}=8(15+1)^{100}
$

So,

$
\begin{aligned}
\Rightarrow \frac{8}{15}(15+1)^{100} & =\frac{8}{15}(15 \lambda+1) \\
& =8 \lambda+\frac{8}{15}
\end{aligned}
$

$\because 8 \lambda$ is integer and $\frac{8}{15}$ is fractional part
So, $k=8$

Example 4: What is the sum of the coefficient of the term $(\sqrt{2}-\sqrt[3]{3}+\sqrt[6]{5})^{10}$ ?
1) $3^{10}$
2) $2^{10}$
3) 1
4) None of the above

Solution
Series Involving Binomial Coefficients -
In the expansion of $(x+y+z)^n$ if we put $x=y=z=1$, then we get the sum of coefficients. In this case, $(1+1+1)^n=\underline{3^n}$.
Sum of the coefficient $\left(x_1+x_2+x_3\right)^n=(1+1+1)^n=3^n$
To determine the coefficient
Put $x_1=1, x_2=-1, x_3=1$ and $n=10$
$(1-1+1)^n=1^n=1$
option C is correct.

Example 5: If $C_r={ }^{25} \mathrm{C}_r$ and $C_0+5 \cdot C_1+9 \cdot C_2+\cdots \cdots+(101) \cdot C_{25}=2^{25} \cdot k$, then k is equal to $\qquad$
1) 15
2) 30
3) 51
4) 27

Solution
The general term for the given series is: $(4 \mathrm{r}+1) .{ }^{25} \mathrm{C}_{\mathrm{r}}$
Applying summation
是
$=4 \sum_{r=1}^{25} r \times{ }^{25} C_r+2^{25}$
$=4 \times 25 \cdot 2^{24}+2^{25}=50 \cdot 2^{25}+2^{25}=(50+1) 2^{25}=51 \cdot 2^{25}$
$k=51$
Hence, the answer is the option 3.