Sum of First n Terms of GP Formula

A geometric sequence is a sequence where the first term is non-zero and the ratio between consecutive terms is always constant. In real life, we use geometric progressions to calculate the size of exponential population growth, such as bacteria in a container.

In this article, we will cover the concept of the Sum of n term of Geometric Progression. This category falls under the broader category of Sequence and series, which is a crucial Chapter in class 11 Mathematics. It is not only essential for board exams but also for competitive exams like the Joint Entrance Examination(JEE Main) and other entrance exams such as SRMJEE, BITSAT, WBJEE, BCECE, and more. Questions based on this topic have been asked frequently in JEE Mains.

JEE Main 2025: Sample Papers | Mock Tests | PYQs | Study Plan 100 Days

JEE Main 2025: Maths Formulas  | Study Materials

JEE Main 2025: Syllabus | Preparation Guide | High Scoring Topics 

This Story also Contains

1. Geometric Progression
2. General Term of a GP
3. Geometric Mean
4. The sum of $ \text { n } $ term of a GP
5. The sum of an infinite GP
6. Application of GP
7. Solved Examples Based on the Sum of n term of GP

Geometric Progression

A geometric sequence is a sequence where the first term is non-zero and the ratio between consecutive terms is always constant. The 'constant factor' is called the common ratio and is denoted by ' $r$ '. $r$ is also a non-zero number.

The first term of a G.P. is usually denoted by $a$.

If $\mathrm{\mathit{a_1,a_2,a_3.....a_{n-1},a_n}}$is in geometric progression

$\mathrm{then,\;\mathit{r=\frac{a_2}{a_1}=\frac{a_3}{a_2}=....=\frac{a_n}{a_{n-1}}}}$

Eg,

• $2,6,18,54, \ldots .(a=2, r=3)$
• $4,2,1,1 / 2,1 / 4, \ldots .(a=4, r=1 / 2)$
• $-5,5,-5,5, \ldots \ldots . .(a=-5, r=-1)$

General Term of a GP

If ' $a$ ' is the first term and ' $r$ ' is the common ratio, then

$a_1=a=a r^{1-1}\left(1^{\text {st }}\right.$ term $)$

$a_2=a r=a r^{2-1}\left(2^{\text {nd }}\right.$ term $)$

$a_3=a r^2=a r^{3-1} \quad\left(3^{\text {rd }}\right.$ term $)$

$a_n=a r^{n-1}\left(\mathrm{n}^{\mathrm{th}}\right.$ term $)$

So, the general term or $n^{\text {th }}$ term of a geometric progression is $a_n=ar^{n-1}$

Geometric Mean

If three terms are in G.P., then the middle term is called the Geometric Mean (G.M.) of the other two numbers. So if $\mathrm{a}, \mathrm{b}$, and $
\mathrm{c} \text { are in G.P., then } \mathrm{b} \text { is } \mathrm{GM} \text { of } \mathrm{a} \text { and } \mathrm{c} \text {, }
$

If $a_1, a_2, a_3, \ldots ., a_n$ are n positive numbers, then the Geometric Mean of these numbers is given by $G=\sqrt[n]{a_1 \cdot a_2 \cdot a_3 \cdot \ldots . . \cdot a_n}$

If $a$ and $b$ are two numbers and $G$ is the $G M$ of $a$ and $b$. Then, $a, G, \underline{b}$ are in geometric progression.

Hence, $G=\sqrt{a \cdot b}$

$
\text { Insertion of n-Geometric Mean Between a and b }
$

Let $\mathrm{G}_1, \mathrm{G}_2, \mathrm{G}_3 \ldots, \mathrm{G}_{\mathrm{n}}$ be n geometric mean between two numbers a and b . Then, $a, \mathrm{G}_1, \mathrm{G}_2, \mathrm{G}_3 \ldots, \mathrm{G}_{\mathrm{n}}, b$ is an G.P. Clearly, this G.P. contains $\mathrm{n}+2$ terms.
now, $\mathrm{b}=(\mathrm{n}+2)^{\text {th }}$ term $=\mathrm{ar}^{\mathrm{n}+2-1}$
$\therefore r=\left(\frac{b}{a}\right)^{\frac{1}{n+1}}$
[where, $\mathrm{r}=$ common ratio]

$
\begin{aligned}
& \therefore \mathrm{G}_1=\mathrm{ar}, \mathrm{G}_2=\mathrm{ar}^2, \mathrm{G}_3=\mathrm{ar}^3, \ldots ., \mathrm{Ga}_{\mathrm{n}}=\mathrm{ar}^{\mathrm{n}} \\
& \Rightarrow \mathrm{G}_1=\mathrm{a}\left(\frac{\mathrm{b}}{\mathrm{a}}\right)^{\frac{1}{\mathrm{n}+1}}, \mathrm{G}_2=\mathrm{a}\left(\frac{\mathrm{b}}{\mathrm{a}}\right)^{\frac{2}{\mathrm{n}+1}}, \mathrm{G}_3=\mathrm{a}\left(\frac{\mathrm{b}}{\mathrm{a}}\right)^{\frac{3}{\mathrm{n}+1}} \ldots \ldots \\
& \mathrm{G}_{\mathrm{n}}=\mathrm{a}\left(\frac{\mathrm{b}}{\mathrm{a}}\right)^{\frac{\mathrm{n}}{\mathrm{a}+1}}
\end{aligned}
$

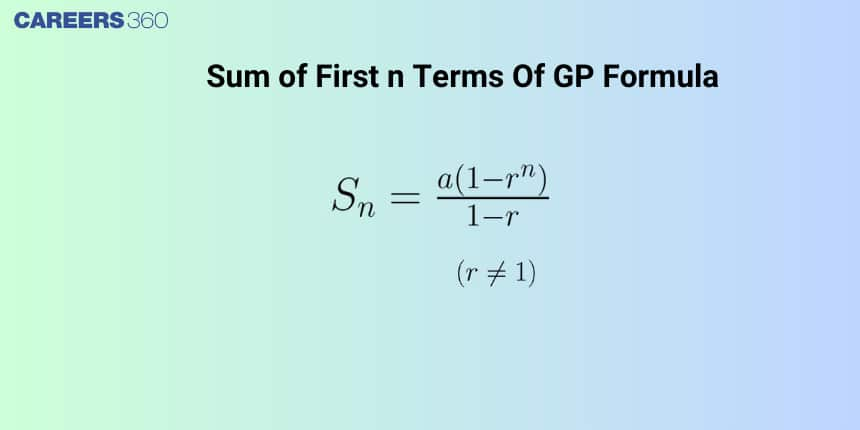
The sum of $ \text { n } $ term of a GP

Let $S_n$ be the sum of $n$ terms of the G.P. with the first term ' $a$ ' and common ratio ' $r$ '. Then

$
S_n = a + ar + ar^2 + \cdots + ar^{n-2} + ar^{n-1} \quad \text{(i)}
$

Multiply both sides by \( r \):

$
rS_n = ar + ar^2 + ar^3 + \cdots + ar^{n-1} + ar^n \quad \text{(ii)}
$

Subtract (ii) from (i):

$
S_n - rS_n = a - ar^n
$

Thus,

$
S_n = \frac{a - ar^n}{1 - r} = a \left( \frac{1 - r^n}{1 - r} \right)
$

$
\Rightarrow S_n = a \left( \frac{r^n - 1}{r - 1} \right)
$

The above formula does not hold for $r=1$
For $\mathrm{r}=1$, each of the n terms is equal to $a$, and thus the sum of $n$ terms of the G.P. is $S_n = na$.

$
\text { So, The sum of } n \text { terms of GP with first term 'a' and common ratio ' } r \text { ' is given by }
$

The sum of an infinite GP

Consider an infinite GP $a, a r, a r^2, a r^3, a r^4 \ldots \ldots \ldots \ldots \ldots \ldots \ldots$

If a is the first term and r is the common ratio of a G.P.

The last term is not known.

Then,

$
S_n = a \left( \frac{1 - r^n}{1 - r} \right) = \frac{a}{1 - r} - \frac{ar^n}{1 - r}
$

Let, $-1<r<1$, i.e. $|r|<1$, then

$\mathrm{\lim_{n\rightarrow \infty}r^n=0}$

[as this is infinite G.P., so $ n $ tends to infinity]

The sum of an infinite term of GP is given by

$\mathrm{S_{\infty}=\frac{a}{1-r}}$

$S_{\infty}$ is the sum of infinite terms of the G.P.Note: If $r \geq 1$, then the sum of an infinite G.P. tends to infinity.

Application of GP

• Using Geometric Progression, we can convert non-terminating numbers into a fraction.

To write a non-terminating repeating number in $
\text { p/q form: }
$

Example:

In this example, we will convert the non-terminating number into a fraction.

The value of 0.358585858585..........

$\begin{array}{l}{0.3 \overline{5} \overline{8}=0.358585858 \ldots \ldots \text { to } \infty} \\ {\Rightarrow 0.3+0.058+0.00058+0.0000058+\ldots \ldots} \\ {\Rightarrow \frac{3}{10}+\frac{58}{10^{3}}+\frac{58}{10^{5}}+\frac{58}{10^{7}}+\ldots \ldots \ldots \ldots} \\\\ {\Rightarrow \frac{3}{10}+\frac{58}{10^{3}}\left(1+\frac{1}{10^{2}}+\frac{1}{10^{4}}+\ldots .\right)} \\\\ {\Rightarrow \frac{3}{10}+\frac{58}{10^{3}}\left(\frac{1}{1-\frac{1}{10^{2}}}\right)} \\\\ {\Rightarrow \frac{355}{990}}\end{array} $

• The sum of the n-term of the series

$a+a a+a a a+a a a a+\ldots \ldots \ldots ., \forall a \in \mathbb{N}, 1 \leq a \leq 9$

With the use of GP, we can find the sum of the n term of the above GP

The sum of the $ n $ term of GP is given by

$\frac{a}{9}\left[\frac{10}{9}\left(10^{n}-1\right)-n\right]$

• The sum of the n-term of the series
  $0.\; a + 0.\; a a + 0.\; a a a + 0.\; a a a a + \ldots, \quad \forall a \in \mathbb{N}, \quad 1 \leq a \leq 9
  $

$\frac{a}{9}\left\{n-\frac{1}{9}\left[1-\left(\frac{1}{10}\right)^{n}\right]\right\}$

Summary

The cumulative effect of repeated multiplicative growth or decay can be precisely calculated using the sum of a geometric progression. Its simple yet adaptable formula serves as the foundation for several mathematical and real-world applications in a wide range of fields. Comprehending its characteristics provides us with useful instruments for both conceptual examination and real-world issue resolution

Recommend Video:

Solved Examples Based on the Sum of n term of GP

Example 1: Let $\left\{a_k\right\}$ and $\left\{b_k\right\}, k \in \mathbb{N}$, be two G.P.s with common ratios $\mathrm{r}_1$ and $\mathrm{r}_2$ respectively such that $\mathrm{a}_1=\mathrm{b}_1=4$ and $\mathrm{r}_1<\mathrm{r}_2$. Let $c_{\mathrm{k}}=\mathrm{a}_{\mathrm{k}}+\mathrm{b}_{\mathrm{k}}, \mathrm{k} \in \mathbb{N}$. If $\mathrm{c}_2=5$ and $c_3=\frac{13}{4} \sum_{\text {then } \mathrm{k}=1}^{\infty} \mathrm{c}_{\mathrm{k}}-\left(12 \mathrm{a}_6+8 \mathrm{~b}_4\right)$ is equal to

[JEE MAINS 2023]

Solution:

$
\begin{aligned}
&\begin{array}{ll}
\mathrm{a}_1=4 & \text { GP } 4,4 \mathrm{r}_1, 4 \mathrm{r}_1^2- \\
\mathrm{b}_1=4 & \text { GP } 4,4 \mathrm{r}_2, 4 \mathrm{r}_2^2- \\
\mathrm{C}_2=\mathrm{a}_2+\mathrm{b}_2 \quad \mathrm{C}_3=\mathrm{a}_3+\mathrm{b}_3 \\
5=4 \mathrm{r}_1+4 \mathrm{r}_2 & \frac{13}{4}=4 \mathrm{r}_1^2+4 \mathrm{r}_2^2 \\
\frac{5}{4}=\mathrm{r}_1+\mathrm{r}_2 \ldots(1) \quad \mathrm{r}_1^2+\mathrm{r}_2^2=\frac{13}{16} \ldots \\
\frac{25}{16}=r_1^2+r_2^2+2 r_1 r_2 \\
\frac{25}{16}=\frac{13}{16}+2 r_1 r_2 \\
\Rightarrow r_1 r_2=\frac{12}{16 \times 2}=\frac{3}{8}
\end{array}\\
&\text { (2) }
\end{aligned}
$

$
\begin{aligned}
& \text { Now } r_1+\frac{3}{8 r_1}=\frac{5}{4} \\
& 8 r_1^2+3=10 r_1 \\
& \Rightarrow 8 r_1^2-10 r_1+3=0 \\
& r_1=\frac{3}{4}, r_1=\frac{1}{2} \\
& r_2=\frac{1}{2} r_2=\frac{3}{4} \\
& \because r_1<r_2 \\
& r_1=\frac{1}{2} \\
& \therefore \quad r_2=\frac{3}{4}
\end{aligned}
$

Now $C_k=a_k+b_k$

$
\begin{aligned}
& \sum_{\mathrm{k}=1}^{\infty} \mathrm{C}_{\mathrm{k}}=\frac{4}{1-\mathrm{r}_1}+\frac{4}{1-\mathrm{r}_2} \\
& =\frac{4}{1-\frac{1}{2}}+\frac{4}{1-\frac{3}{4}} \\
& =8+16=24 \\
& \sum_{\mathrm{k}=1}^{\infty} \mathrm{C}_{\mathrm{k}}-\left(12 \mathrm{a}_6+8 \mathrm{~b}_4\right) \Rightarrow 24-\left\{12 \times 4\left(\frac{1}{2}\right)^5+8 \times 4\left(\frac{3}{4}\right)^3\right\} \\
& =24-\left(12 \times \frac{1}{8}+8 \times \frac{27}{16}\right) \\
& =24-\left\{\frac{3}{2}+\frac{27}{2}\right\} \\
& =24-15 \\
& =9
\end{aligned}
$

Hence, the required answer is 9.

Example 2:

The sum of 20 terms of the series $2.2^2-3^2+2.4^2-5^2+2.6^2-\ldots \ldots \ldots$ is equal to [JEE MAINS 2023]

Solution:

$\begin{aligned} & \left(2^2-3^2+4^2-5^2+20 \text { terms }\right)+\left(2^2+4^2+\ldots+10 \text { terms }\right) \\ & -(2+3+4+5+\ldots+11)+4\left[1+2^2+\ldots 10^2\right] \\ & -\left[\frac{21 \times 22}{2}-1\right]+4 \times \frac{10 \times 11 \times 21}{6} \\ & =1-231+14 \times 11 \times 10 \\ & =1540+1-231 \\ & =1310\end{aligned}$

Hence, the required answer is 1310.

Example 3:

Let $A_1$ and $A_2$ be two arithmetic means and $G_1, G_2, G_3$ be three geometric means of two distinct positive numbers. Then $G_1^4+G_3^4+G_3^4+G_3^2 G_3^2$ is equal to

[JEE MAINS 2023]

Solution

$ \begin{aligned}
& \quad a, \mathrm{~A}_1, \mathrm{~A}_2, \text { bareinA.P. } \\
& d=\frac{b-a}{3}: A_1=a+\frac{b-a}{3}=\frac{2 a+b}{3} \\
& A_2=\frac{a+2 b}{3} \\
& A_1+A_2=a+b \\
& a, G_1, G_2, G_{s,} b \text { are in } G \cdot P \\
& r=\left(\frac{b}{a}\right)^{\frac{1}{4}} \\
& G_1=\left(a^3 b\right)^{\frac{1}{4}} \\
& G_2=\left(a^2 b^2\right)^{\frac{1}{4}} \\
& G_5=\left(a b^3\right)^{\frac{1}{4}}
\end{aligned} $

Example 4:

The 4th term of GP is 500 and its common ratio is $\frac{1}{m}, m \in \mathrm{N}_{\text {. }}$ Let $S_{\mathrm{n}}$ denote the sum of the first n terms of this GP. If $\mathrm{S}_6>\mathrm{S}_5+1$ and $\mathrm{S}_7<\mathrm{S}_6+\frac{1}{2}$, then the number of possible values of m is

[JEE MAINS 2023]

Solution

$
\mathrm{T}_4=500 \Rightarrow \mathrm{a}\left(\frac{1}{\mathrm{~m}}\right)^3=500 \Rightarrow \mathrm{a}=500 \mathrm{~m}^3
$
Now $\mathrm{S}_{\mathrm{n}}-\mathrm{S}_{\mathrm{n}-1}=\mathrm{a}\left(\frac{1-\mathrm{r}^{\mathrm{n}}}{1-\mathrm{r}}\right)-\mathrm{a}\left(\frac{1-\mathrm{r}^{\mathrm{n}-1}}{1-\mathrm{r}}\right)$

$
\begin{aligned}
& =\frac{a}{1-r}\left[r^{n-1}(1-r)\right] \\
& =a r^{n-1} \\
& =500 \mathrm{~m}^3\left(\frac{1}{m}\right)^{n-1} \\
& S_n-S_{n-1}=500 \mathrm{~m}^{4-n}
\end{aligned}
$

Now $S_6-S_s>1 \Rightarrow 500 \mathrm{~m}^{-2}>1 \ldots$
$\& S_7-S_6<\frac{1}{2} \Rightarrow 500 \mathrm{~m}^{-3}<\frac{1}{2} \ldots$
$\left.\begin{array}{lc}\text { from(1) } & \mathrm{m}^2<500 \\ \text { from(2) } & \mathrm{m}^3>1000\end{array}\right] 10<\mathrm{m} \leq 22$
The number of possible values of $m$ is $=12$

Example 5:

For $\mathrm{k} \in \mathrm{N}$, if the sum of the series $1+\frac{4}{\mathrm{k}}+\frac{8}{\mathrm{k}^2}+\frac{13}{\mathrm{k}^3}+\frac{19}{\mathrm{k}^4}+\ldots \ldots$ is 10 , then the value of k is:

[JEE MAINS 2023]

Solution

$\begin{aligned} & 10=1+\frac{4}{\mathrm{k}}+\frac{8}{\mathrm{k}^2}+\frac{13}{\mathrm{k}^3}+\frac{19}{\mathrm{k}^4}+\ldots . . \text { upto } \infty \\ & 9=\frac{4}{\mathrm{k}}+\frac{8}{\mathrm{k}^2}+\frac{13}{\mathrm{k}^3}+\frac{19}{\mathrm{k}^4}+\ldots . \text { upto } \infty \\ & \frac{9}{\mathrm{k}}=\frac{4}{\mathrm{k}^2}+\frac{8}{\mathrm{k}^3}+\frac{13}{\mathrm{k}^4}+\ldots . . \text { upto } \infty \\ & \mathrm{S}=9\left(1-\frac{1}{\mathrm{k}}\right)=\frac{4}{\mathrm{k}}+\frac{4}{\mathrm{k}^2}+\frac{5}{\mathrm{k}^3}+\frac{6}{\mathrm{k}^4} \ldots . . \text { upto } \infty \\ & \frac{\mathrm{S}}{\mathrm{k}}=\frac{4}{\mathrm{k}^2}+\frac{4}{\mathrm{k}^3}+\frac{5}{\mathrm{k}^4}+\ldots \ldots \text { upto } \infty \\ & \left(1-\frac{1}{\mathrm{k}}\right) \mathrm{S}=\frac{4}{\mathrm{k}}+\frac{1}{\mathrm{k}^3}+\frac{1}{\mathrm{k}^4}+\frac{1}{\mathrm{k}^5}+\ldots . \infty \\ & \left(1-\frac{1}{\mathrm{k}}\right)^2=\frac{4}{\mathrm{k}}+\frac{\frac{1}{\mathrm{k}^3}}{\left(1-\frac{1}{\mathrm{k}}\right)} \\ & 9(\mathrm{k}-1)^3=4 \mathrm{k}(\mathrm{k}-1)+1 \\ & k=2\end{aligned}$

Hence, the answer is 2.