Syllogism: Topics, Tricks, Examples

Syllogism, derived from the Greek word "syllogismos," meaning "conclusion" or "inference," is a form of logical reasoning that joins two or more premises to conclude. It has been a fundamental element of logic since ancient times and continues to play a crucial role in various fields, including philosophy, mathematics, and computer science.

JEE Main 2025: Sample Papers | Mock Tests | PYQs | Study Plan 100 Days

JEE Main 2025: Maths Formulas  | Study Materials

JEE Main 2025: Syllabus | Preparation Guide | High Scoring Topics 

This Story also Contains

1. Syllogism
2. Tips and Tricks to solve syllogism – questions:
3. Solved Examples Based on Syllogism:Example 1: Mark the answer according to the following conditions:
4. Summary

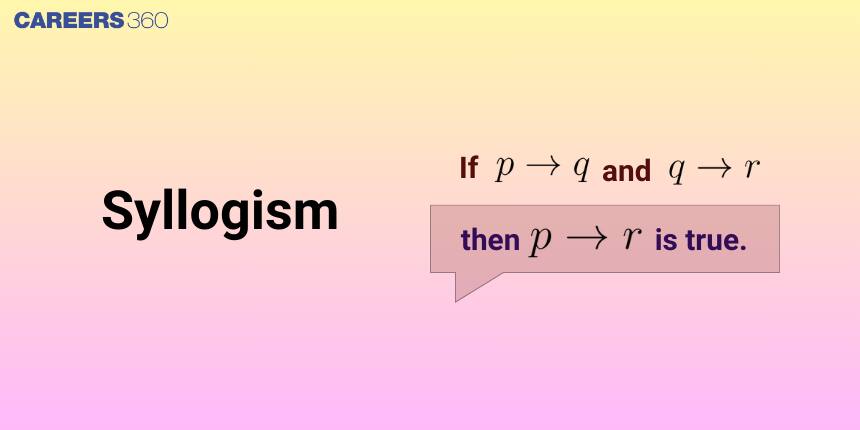
Syllogism

A syllogism has been defined as “A form of reasoning in which a conclusion is drawn from two given or assumed propositions”. It is deductive reasoning rather than inductive reasoning.

Let us begin by taking an example: All girls love to dress up.

There are two sets here, (a) that of girls and (b) that of those who love to dress up. Note that in this case, girls are a part of a bigger set of those who love to dress up. The concept becomes clearer with the help of Venn diagrams.

If the statement had been: Some girls love to dress up, it would mean that a part of the bigger set of girls would love to dress up. The diagrammatical representation would have been like:

In this diagram, the shaded area represents girls who love to dress up.

Now, if we have a statement like Only girls love to dress up, it means that no one else can love to dress up. However, it does not mean that all girls love to dress up. The statement can be diagrammatically represented as:

Let us now move on to situations where there are two statements instead of one. Statements like: All boys love to play football, all girls love to play football. The diagrammatic representation of this will be:

As shown in the Venn diagram, you will see that boys and girls are parts or elements of a larger set of those who love to play football.' And also that boys and girls are two separate sets. As shown in the Venn diagram, you will see that boys and girls are parts or elements of a larger set of 'those who love to play football'. And, also that boys and girls are two separate sets.

Had the statement been, some boys love to dress up and some girls love to dress up, but no boy is a girl and vice versa, it would have been represented as:

Let us now find out whether a third statement can be drawn or concluded from two given statements. All girls love to dress up. Some boys are girls. Let us again take the help of Venn diagrams to represented these.

Tips and Tricks to solve syllogism – questions:

1. Always represent the sentences diagrammatically with the use of Venn diagrams. This will help you be accurate and will save time once you are comfortable using this way to solve questions.
2. Commonly known facts have to be disregarded. So, even if the premise says all blue is red, the conclusion must be derived from the given information.
3. Do not assume any prior knowledge. The conclusion in a syllogism must be derived from the premises given, and not from any prior knowledge on the subject.
4. The conclusion must give some new information based on the two premises, and not simply reiterate the given facts.

5. One syllogism question can test the relationship among three elements only. This will help you in eliminating options and marking the right answers quickly.

6. Use initials to represent H and C respectively. If there are two elements eg. hat and comb, these can be represented by H and C respectively. If there are two elements eg. 'hat' and 'hair' avoid using a single alphabet or representing both. Instead, use the abbreviation 'ht' for hat and 'hr' for 'hair' to avoid confusion while drawing the diagrams.

Recommended Video Based on Syllogism

Solved Examples Based on Syllogism:

Example 1: Mark the answer according to the following conditions:

Statements:

No doctor is enthusiastic.

Some doctors are playful.

Conclusion:

1. All enthusiastic are playful

2. Some playful are not enthusiastic

1) If Only conclusion no. 1 follows

2) If Only conclusion no. 2 follows

3) If either of the two follows

4) If Both conclusions follow

Solution

Syllogism

No doctor is enthusiastic, this can be represented as:

Adding to this premise, the question states, "some doctors are playful". This can be represented in a few ways:

Either way, playful who are doctors, will not be enthusiastic. Hence only option b follows.

Example 2: Negation of the Boolean statement $(\mathrm{p} \vee \mathrm{q}) \Rightarrow((\sim \mathrm{r}) \vee \mathrm{p})$ is equivalent to
1) $\mathrm{p} \wedge(\sim \mathrm{q}) \wedge \mathrm{r}$
2) $(\sim \mathrm{p}) \wedge(\sim \mathrm{q}) \wedge \mathrm{r}$
3) $(\sim \mathrm{p}) \wedge \mathrm{q} \wedge \mathrm{r}$
4) $\mathrm{p} \wedge \mathrm{q} \wedge(\sim \mathrm{r})$

Solution

$
(\mathrm{p} \vee \mathrm{q}) \Rightarrow((\sim \mathrm{r}) \vee \mathrm{p})
$
As negation of $\mathrm{p} \rightarrow \mathrm{q}$ is $\mathrm{p} \wedge \sim \mathrm{r}$
$\therefore$ Negation of given statement is

$
\begin{aligned}
& (\mathrm{p} \vee \mathrm{q}) \wedge \sim((\sim \mathrm{r}) \vee \mathrm{p}) \\
& \equiv(\mathrm{p} \vee \mathrm{q}) \wedge(\mathrm{r} \wedge \sim \mathrm{p})
\end{aligned}
$
Using Venn diagram technique

$
\equiv(\sim \mathrm{p}) \wedge \mathrm{q} \wedge \mathrm{r}
$
Hence answer is option 3

Example 3: Consider the following statements :
$P$ : Ramu is intelligent.
$Q$ : Ramu is rich.
$R$ :Ramu is not honest.
The negation of the statement "Ramu is intelligent and honest if and only if Ramu is not rich" can be expressed as :
1) $
((P \wedge(\sim R)) \wedge Q) \wedge((\sim Q) \wedge((\sim P) \vee R))
$

2) $
((P \wedge R) \wedge Q) \vee((\sim Q) \wedge((\sim P) \vee(\sim R)))
$

3) $
((P \wedge R) \wedge Q) \wedge((\sim Q) \wedge((\sim P) \vee(\sim R)))
$

4) $
((\mathrm{P} \wedge(\sim \mathrm{R})) \wedge \mathrm{Q}) \vee((\sim \mathrm{Q}) \wedge((\sim \mathrm{P}) \vee \mathrm{R}))
$

Solution

Given statement is $(\mathrm{p} \wedge \sim \mathrm{r}) \longleftrightarrow \sim \mathrm{q}$

$
\begin{aligned}
& \equiv((\mathrm{p} \wedge \sim \mathrm{r}) \rightarrow \sim \mathrm{q}) \wedge(\sim \mathrm{q} \rightarrow(\mathrm{p} \wedge \sim \mathrm{r})) \\
& \equiv(\sim(\mathrm{p} \wedge \sim \mathrm{r}) \vee \sim \mathrm{q}) \wedge(\mathrm{q} \vee(\mathrm{p} \wedge \sim \mathrm{r}))
\end{aligned}
$
It's negation is

$
\begin{aligned}
& \sim(\sim(\mathrm{p} \wedge \sim \mathrm{r}) \vee \wedge \mathrm{q}) \vee \sim(\mathrm{q} \vee(\mathrm{p} \wedge \sim \mathrm{r})) \\
& \equiv((\mathrm{p} \wedge \sim \mathrm{r}) \wedge \mathrm{q}) \vee(\sim \mathrm{q} \wedge \sim(\mathrm{p} \wedge \sim \mathrm{r}))
\end{aligned}
$
Hence correct option is 4

Example 4:

If the truth value of the statement $(\mathrm{P} \wedge(\sim \mathrm{R})) \rightarrow((\sim \mathrm{R}) \wedge \mathrm{Q})$ is F , then the truth value of which of the following is F ?
1) $
\mathrm{P} \vee \mathrm{Q} \rightarrow \sim \mathrm{R}
$

2) $
\mathrm{R} \vee \mathrm{Q} \rightarrow \sim \mathrm{P}
$

3) $
\sim(\mathrm{P} \vee \mathrm{Q}) \rightarrow \sim \mathrm{R}
$

4) $
\sim(\mathrm{R} \vee \mathrm{Q}) \rightarrow \sim \mathrm{P}
$

Solution

$\mathrm{P} \wedge \sim \mathrm{R} \rightarrow(\sim \mathrm{R} \wedge \mathrm{Q})$ is false
$\therefore P \wedge \sim R$ is true and $((\sim R) \wedge Q)$ is false
$\Rightarrow P$ is true $R$ is false, $Q$ is false
(A) $: \mathrm{P} \vee \mathrm{Q} \rightarrow \sim \mathrm{R} \Rightarrow \mathrm{T} \rightarrow \mathrm{T} \Rightarrow \mathrm{T}$
(B) : $\mathrm{R} \vee \mathrm{Q} \rightarrow \sim \mathrm{P} \Rightarrow \mathrm{F} \rightarrow \mathrm{F} \Rightarrow \mathrm{T}$
(C) $: \sim(\mathrm{P} \vee \mathrm{Q}) \rightarrow \sim \mathrm{R} \Rightarrow \mathrm{F} \rightarrow \mathrm{T} \Rightarrow \mathrm{T}$
(D) $: \sim(\mathrm{R} \vee \mathrm{Q}) \rightarrow \sim \mathrm{P} \Rightarrow \mathrm{T} \rightarrow \mathrm{F} \Rightarrow \mathrm{F}$
$\therefore$ option (D)

Summary

Syllogism is a fundamental concept in logic that enables rigorous deductive reasoning. By understanding its structure, types, principles, and applications, one can enhance their logical thinking and problem-solving skills.