Fundamental Geometry Concepts for 2D Shapes: Definitions, Properties, and Examples
Comprehending fundamental geometric ideas is essential for candidates getting ready for design aptitude exams such as NID and UCEED. The Understanding of basic geometric concepts is vital for NAT(Numerical Aptitude Test) in various DAT examinations conducted by most reputed D-Schools. To maximize your chances of acing the test, you must understand the fundamentals of dimensions. This will boost your technical expertise as well as your creative capacity to come up with workable design solutions.
Introduction to Basic Geometric Shapes
CIRCLE:
A circle is a round shape where every point along its edge is equidistant from a central point.
Examples: Common examples of circles include coins, wheels, and clocks.
Formula: Area = π × R², where R is the radius.
Major Sector: A circle division with a centre angle larger than 180 degrees.
Minor Sector: A division of the circle distinguished by Less than 180° is the central angle that characterises it.
Let’s understand this with an example of pizza and a slice. Consider the pizza as a full circle, and the slice can be considered a minor sector.
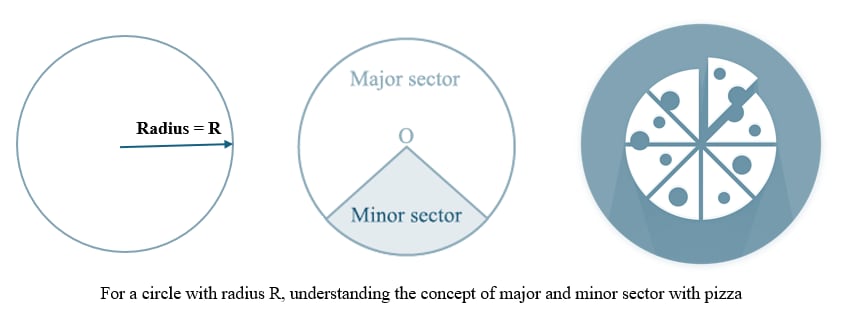
Example: Understanding through an example of a Pizza cut
Calculating the Area of a Sector : (θ/360°) × πr2
Where θ = angle of the sector
r = Radius of circle
PARALLELOGRAM: It is a four-sided polygon with parallel and equal opposite sides.
Properties of a Parallelogram
Understanding the properties of the parallelogram is required for the practical application as asked in diverse Numerical Aptitude and Design Aptitude Sections of the different examinations.
For the above parallelogram, consider the following properties:
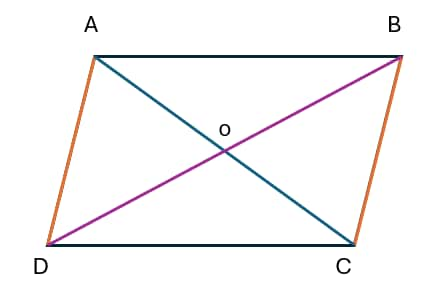
The opposing sides are parallel.
In this case, AB || DC & AD || BC
In a parallelogram, the opposing sides are always equal.
In this case, AB=DC and AD=BC
For a parallelogram, the opposite angles are equal.
In this case, ∠A= ∠C and ∠B=∠D
4. A parallelogram's diagonals cut each other in half.
In this case, AO = OC and BO = OD
5. Interior angles on the same side complement one another.
In this case, ∠ADC + ∠DCB = 180∘, Similarly ∠DCB + ∠CBA = 180∘,
∠CBA + ∠ABD = 180∘, ∠BAD + ∠ADC = 180∘.
6. The parallelogram is divided into two congruent triangles by the diagonals.
Here, ΔADB and ΔDCS are congruent, while ΔADC and ΔABC are congruent.
Classification of Parallelogram: It can be classified mainly into three distinct types as follows :
2a. Square
2b. Rectangle
2c. Rhombus
2a. Square :
Examine the ABCD square and associate it with the subsequent attributes. 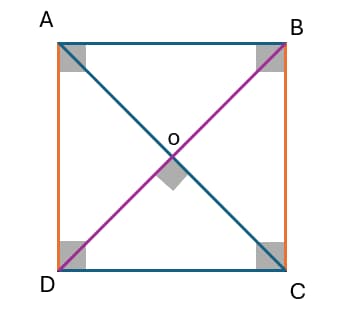
A square possesses the following properties:
Four equal sides. In this case, AB = BC = CD = DA
Four angles to the right. Here, ∠A = ∠B = ∠C = ∠D =90∘
Two sets of sides that are parallel to one another. In this case, AB || DC, AD || BC
A pair of diagonals that are equal. In this case, AC = BD.
Diagonals are perpendicular to one another. In this case, AC⊥BD
Diagonals bisect each other in half
Area of the Square = Side(AB) X Side(BC)
Perimeter of the Square = 4 X Side
2b. Rectangle
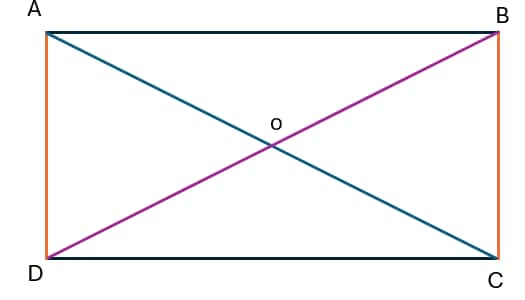
Examine the ABCD rectangle and associate it with the following characteristics. A rectangle possesses:
Two sets of sides that are parallel to one another. In this case, AB || DC, AD || BC
All Four angles are right angles. Here, ∠A = ∠B = ∠C = ∠D = 90∘
Opposite sides are equal in length. This time, AD = BC and AB = DC.
A pair of diagonals that are equal. In this case, AC = BD.
Diagonals that split others into half.
Area of the Rectangle = Length (DC) X Breath (AD)
The perimeter of the Rectangle = 2 (Length + Breadth)
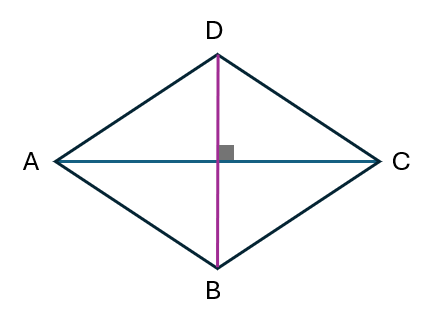
2c. Rhombus
Examine the rhombus EFGH to associate it with the subsequent characteristics. A Rhombus possesses:
Two sets of sides that are parallel to one another. In this case, AB || DC and BC || AD
Four equal sides. Here, AB=BC=CD=DA
Opposite angles are equal, H = F and ∠E = ∠G
Diagonals run parallel to one another. In this case, AC⊥BD
Diagonals(AC & DB) split each other into two halves.
Area of the Rhombus = (Diagonal 1 X Diagonal 2) / 2
Perimeter of the Square = 4 X Side
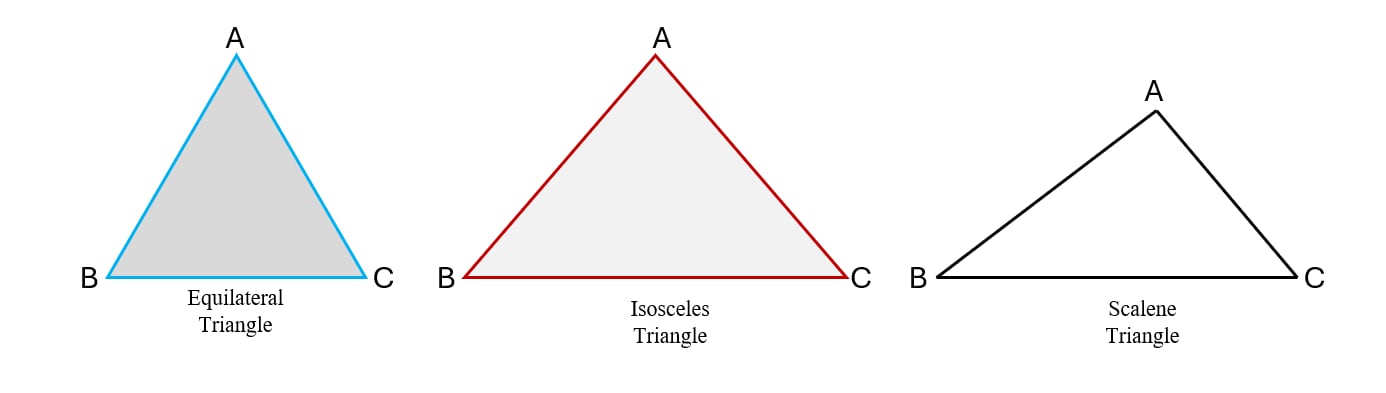
3. TRIANGLES
A triangle, one of the fundamental geometric shapes, is a polygon with three corners and three sides. The sides joining the corners, also known as edges, are one-dimensional line segments, whereas the corners, also known as vertices, are zero-dimensional points.
Classification of Triangles Based on Sides
3a. Isosceles Triangles
3b. Scalene Triangle
3c. Equilateral Triangle
We will cover the fundamentals of the triangles one by one to understand the basics for application:
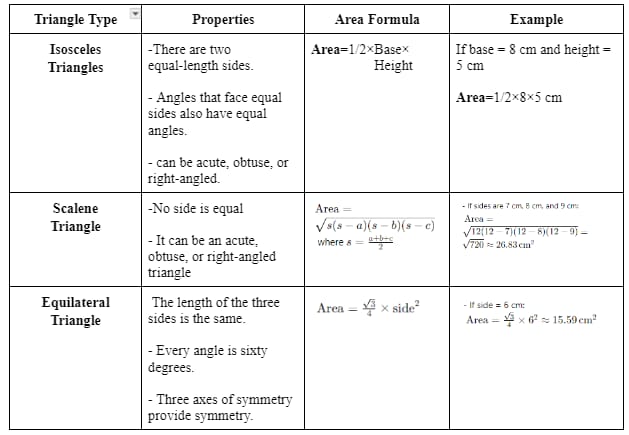
Here in the below table, the properties & Areas of different types of Triangle is covered :
You can also refer to:
Simple Machines (Part-1): Inclined Plane & Lever
Fundamentals of Geometry for 3D Shapes
4. POLYGONS
The term “polygon” is derived from the word ‘poly’ meaning ’many’ and ‘gon’ meaning ‘Angle’.
Polygons are defined as two-dimensional closed shapes formed by joining three or more line segments with each other. We encounter polygons mostly while we learn about geometry. In this lesson, we will learn about polygons' definitions, regular polygons, polygon sides, and the properties of polygons, along with polygon examples and their identification.
Properties of a Polygon
It is composed of three or more sides.
The polygon's angles might or might not be equal.
A polygon's sides could all be the same length or different.
It is a planar shape, meaning that it is composed of straight lines or line segments.
It is a two-dimensional figure, meaning its length and breadth are its only two dimensions. It has no height or depth.
A polygon is a closed shape; it does not have any open ends. It starts and finishes at the exact location.
In this case a Regular Hexagon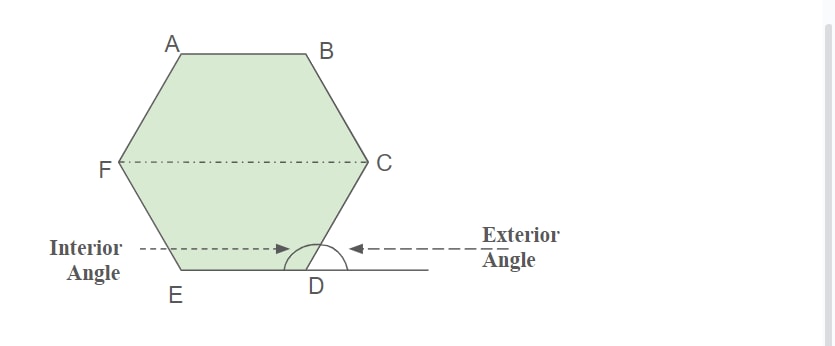
The diagonal is FC.
Every inner angle is equal.
Every external angle is equal.
The hexagon's vertices are A, B, C, D, E, and F.
This regular hexagon has equal sides, which are as follows:
AB = BC = CD = DE = EF = FA.
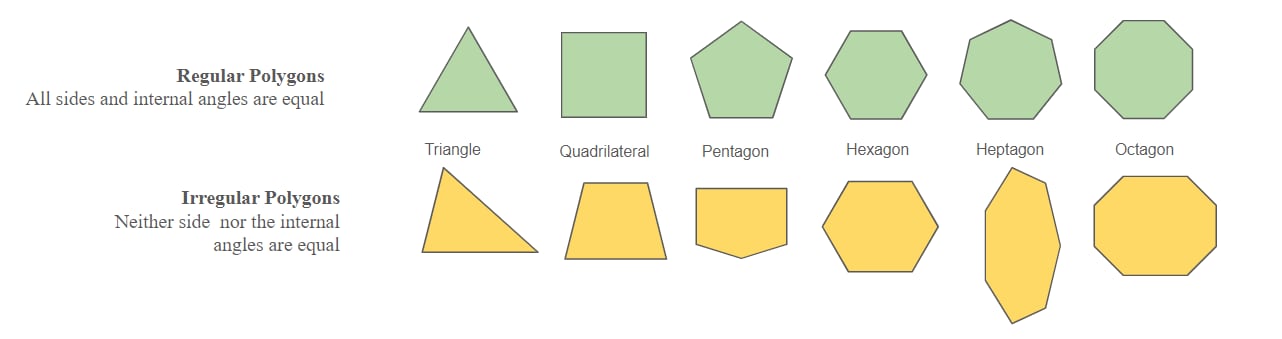
Types of Polygons
There is a range of polygons based on the number of sides from a minimum of 3 sides(Triangle) to even up to 20 (Icosagon)
Based on the length of its sides and the measurement of its angles, a polygon can be classified as either regular or irregular.
A polygon fulfilling all the below conditions is called a Regular polygon when :
The length of all sides is equal.
Measurement of all interior angles is equal
Measurement of all the exterior angles is equal as well.
Perimeter of a Polygon
A polygon's perimeter is its entire boundary measured from end to end. Since polygons are closed-plane shapes, their perimeters fall inside two-dimensional planes.
Q: Calculate the Perimeter of a Regular Pentagon for a side light of 5 cm.
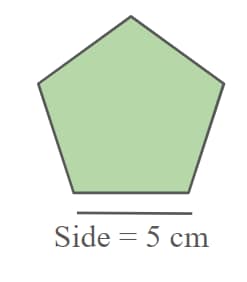
A: Since it’s a regular pentagon, all the sides are equal
Perimeter = Sum of All sides
Perimeter = 5 X (5)
Area of a Polygon
The area of a Polygon is the region or space occupied inside a polygon.
A polygon can be classified as regular or irregular, depending on how long its sides are. Therefore, there is a discrepancy in the area of polygons calculated due to this differentiation. The following are some well-known polygons' areas:
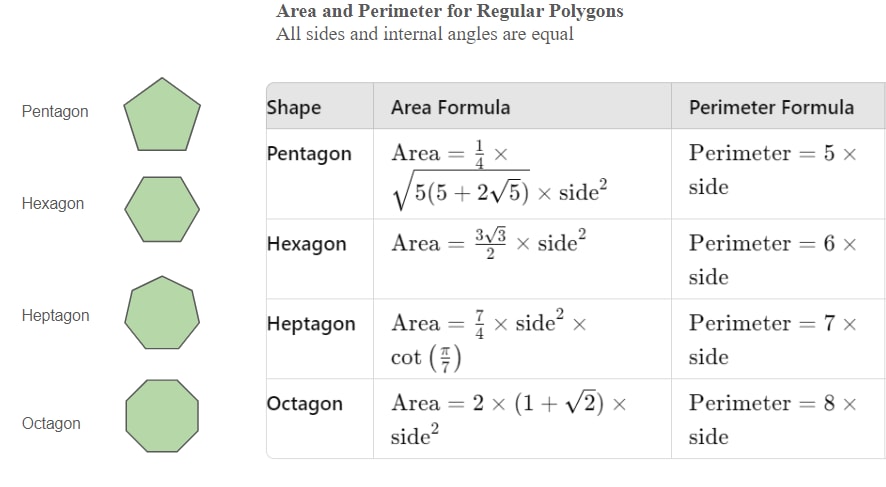
Note: The questions asked in the Design aptitude examination may not ask you to calculate the area of a given figure directly, but mostly the questions asked are application-based.
Sample Question From Previous Year Exams: UCEED/NID- DAT
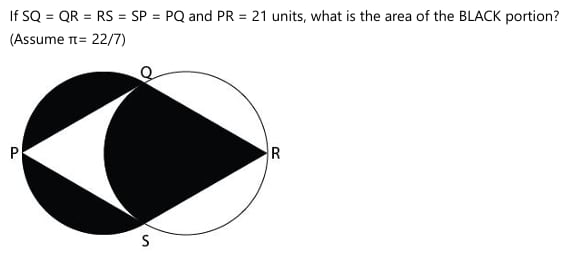
Frequently Asked Questions (FAQs)
Learning how to draw basic geometric shapes such as triangles, parallelograms, and circles is imperative for design tests. These ideas serve as the cornerstone for resolving challenging design issues and improve your capacity to use geometry in original and useful ways.
For design tests, it is essential to comprehend the characteristics of polygons, including their classification, sides, and angles. These characteristics aid in the resolution of application-based queries that assess your aptitude for deriving, analysing, and applying geometric concepts in design contexts.
Understanding circles and major and minor sectors is essential for design tests since it helps with area, proportion, and visual representation challenges. Comprehending these ideas enables you to address inquiries concerning circular forms and their uses in design.