Acceleration - Examples, Meaning, Types, Formula, Faqs

As we shall study, the acceleration of an object is the change in its velocity in each unit of time. In case the change in velocity in each unit of time is constant, the object is said to be moving with constant acceleration and such a motion is called uniformly accelerated motion. On the other hand, if the change in velocity in each unit of time is not constant, the object is said to be moving with variable acceleration, and such a motion is called a non-uniformly accelerated motion.

This Story also Contains

1. What is Acceleration
2. Characteristics Of Acceleration
3. Define Acceleration Formula
4. Important Terms and Equations Related To Acceleration
5. Types of Acceleration
6. Positive, Negative, And Zero Acceleration
7. Real-life Examples Of Acceleration
8. Solved Examples Based on Acceleration

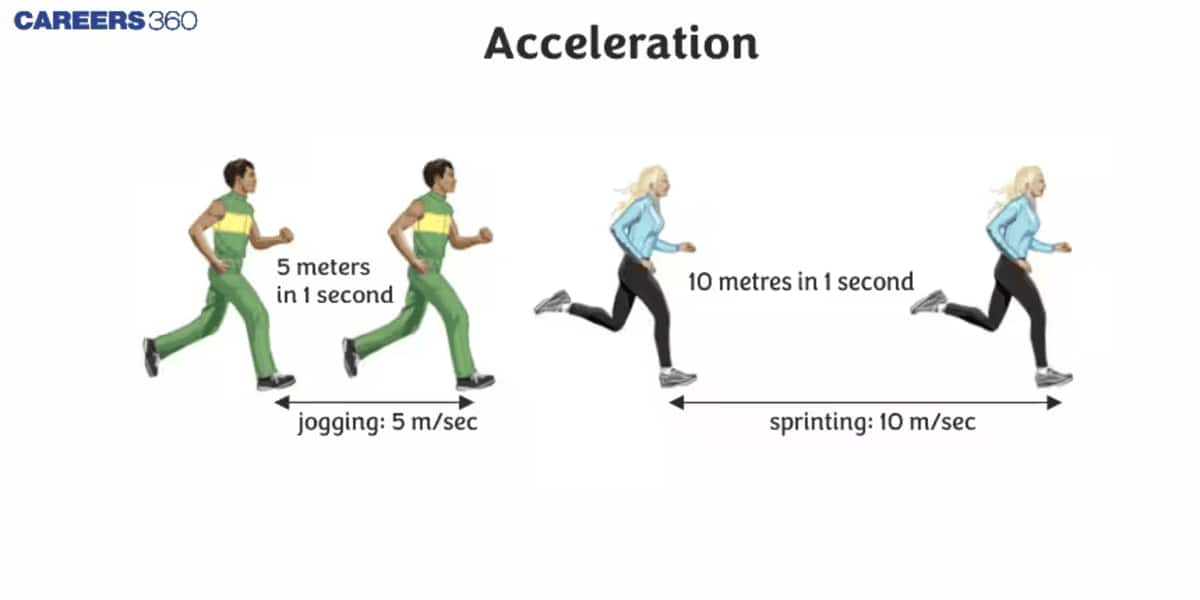
This concept belongs to the chapter Kinematics, which is an important chapter in Class 11 physics. It is not only essential for board exams but also for competitive exams like the Joint Entrance Examination (JEE Main), National Eligibility Entrance Test (NEET), and other entrance exams such as SRMJEE, BITSAT, WBJEE, BCECE, and more. Over the last ten years of the JEE Main exam (from 2013 to 2023), almost seven questions have been asked on this concept. And for NEET three questions were asked from this concept.

What is Acceleration

The rate at which an object's velocity changes in both magnitude and direction over time is called acceleration. A point or object is accelerated if it moves faster or slower in a straight line. On a circle, the direction of motion is constantly changing. Even if the speed is constant, the motion is accelerated. Both effects contribute to the acceleration of all other types of motion. The formula of acceleration is:

$ \vec{a}=\frac{\text { change in velocity }}{\text { time taken }}=\frac{\vec{v}_f-\vec{v}_i}{t}$

Where:

• $\overrightarrow{v_f}=\text { Final velocity, }$
• $\vec{v}_i=\text { initial velocity and }$
• $\mathrm{t}=\text { time }$

Characteristics Of Acceleration

1. The body is said to have undergone acceleration if there is a change in velocity i.e.,

   • Change in speed

   • Change in direction

   • Change in both

2. It is a vector quantity.

3. It has both direction and magnitude.

4. It can either be positive or negative.

5. Dimension of acceleration is $L T^{-2}$

6. The S.I unit of acceleration is: $m s^{-2}$

Explain Acceleration with An Example

Let us understand what is the meaning of acceleration with the help of some examples.

When we are on a roller coaster ride, as it starts, we experience a push backward against our seats, and when it stops, we experience a push forward, and whenever it makes a sharp turn, we experience a push sidewards. This push experienced is all because of acceleration.

When the ride starts or stops, there is a change in its speed. And when it makes a turn, there is a change in its direction.

Define Acceleration Formula

(i) In General

We are familiar that velocity is a vector quantity because it is a speed with direction. The acceleration 'a' is calculated as follows:

$\mathrm{acceleration} = \mathrm{ \frac{Velocity Change}{Time Taken}}$

This acceleration formula indicates that it is the rate of change in velocity, or if an object's velocity changes from its initial value "u" to its final value "v," the formula of acceleration can be expressed as:

$\mathrm{a}= \mathrm{\frac{(v - u)}{t}}$

(ii) In Physics

Acceleration in physics is well-defined as the rate at which an object's velocity changes, regardless of whether it speeds up or slows down. If it accelerates, the acceleration is positive; if it slows, the acceleration is negative. According to Newton's Second Law, it is caused by the object's net imbalanced force. Because it describes the rate of velocity change for time, which is a vector quantity, acceleration is a vector quantity. The letter 'a' stands for acceleration. It has the SI unit of m/s² and the dimensions of M⁰L¹T^-2.

If v_o denotes initial velocity, v_t denotes final velocity, and t denotes time taken.

Acceleration is equal to:

$\frac{v_t-v_0}{t}=a$

Also read :

• NCERT solutions for Class 11 Physics Chapter 3 Motion in a straight line
• NCERT notes Class 11 Physics Chapter 3 Motion in a straight line
• NCERT Exemplar Class 11 Physics Solutions Chapter 3 Motion in a Straight Line

Important Terms and Equations Related To Acceleration

1. Instantaneous acceleration :

It is the acceleration of a body at a certain instant of time.

If r denotes the displacement vector,
$v=\frac{ \mathrm{dr}}{\mathrm{dt}}$ denotes velocity

Acceleration is equal to,
$\mathrm{a}=\frac{\mathrm{dv}}{\mathrm{dt}}=\frac{d^2\mathrm{r}}{ d \mathrm{t}^2}$

2. Average Acceleration:

Average acceleration is the ratio of change in velocity in a certain time interval to the time interval. The average acceleration formula is given as:

$\text { Average Acceleration }\left(a_{\text {avg }}\right)=\frac{v_f-v_i}{t_f-t_i}$

where,

• $v_f$ is the final velocity,
• $v_i$ is the initial velocity,
• $t_f$ is the final time,
• $t_i$ is the initial time

3. Acceleration Due To Gravity

The acceleration produced in the motion of a body under the effect of gravity is called acceleration due to gravity. It is denoted by "g".

$g=\frac{G \cdot M}{r^2}$

where,

• $g$ is the acceleration due to gravity (approximately $9.8 \mathrm{~m} / \mathrm{s}^2$ on Earth's surface),
• $G$ is the universal gravitational constant $\left(6.674 \times 10^{-11} \mathrm{Nm}^2 / \mathrm{kg}^2\right)$,
• $M$ is the mass of the Earth (or the celestial body in question),
• $r$ is the radius or distance from the center of the Earth (or celestial body) to the object.

4. Centripetal Acceleration:

It is the acceleration experienced by the object moving in a circular path directed towards the centre of the circular path. The centripetal acceleration formula is:

$a_c=\frac{v^2}{r}$

Where,

• $a_c$ is the centripetal acceleration,
• $v$ is the tangential velocity of the object,
• $ r$ is the radius of the circular path.

5. Angular Acceleration:

It is the rate of change of angular velocity over time.

$\alpha=\frac{\Delta \omega}{\Delta t}$

Where,

• $\alpha$ is the angular acceleration,
• $\Delta \omega$ is the change in angular velocity,
• $\Delta t$ is the change in time.

6. Tangential Acceleration

It is the rate of change of tangential velocity of an object in a circular path.

$a_t=\frac{d v}{d t}$

Where,

• $a_t$ is the tangential acceleration,
• $v$ is the tangential velocity,
• $t$ is time.

7. Newton’s Second Law Of Motion And Acceleration

According to Newton’s second law of motion, the rate of change of linear momentum of a body is directly proportional to the external force applied on the body, and this change takes place always in the direction of the applied force. The second law of motion gives a measure of force

$F=m \cdot a$

Where,

• $F$ is the net force acting on the object.
• $m$ is the mass of the object
• -$a$ is the acceleration of the object.

Related Topics,

• Average Speed and Average Velocity
• Average Velocity
• Unit of Acceleration
• Uniformly Accelerated Motion
• Angular Acceleration

Types of Acceleration

1. Uniform acceleration

2. Non-uniform acceleration

1. Uniform Acceleration

We say an object has uniform acceleration if its speed (velocity) increases at a constant pace. That is, there is no change in the rate of acceleration.

As the name implies, uniformly accelerated motion refers to an object or a body that accelerates at a consistent rate. Constant velocity does not imply constant acceleration. The definition of uniform acceleration is when a body is in motion, and the amount of variation in velocity in equal intervals of time is constant.

Example of Uniform acceleration

For an example of uniform acceleration, consider the motion of a freely falling body, where the body's acceleration is the sole acceleration attributable to gravity. When we plot velocity vs time on a graph, we get a straight line, and the entire slope equals the required acceleration. Real-life example would be when someone is parachute diving.

2. Non-Uniform Acceleration

Non-uniform acceleration occurs when an object's velocity varies in variable amounts during equal time intervals.

The opposite of uniform acceleration is non-uniform acceleration. We know that uniform acceleration indicates that the rate of change in velocity remains constant across time. In a non-uniform acceleration, the change in velocity is not the same. The magnitude of acceleration and the direction of velocity will change over time.

Example of non-uniform acceleration

Imagine driving a car on the road; there is a recurrent increase or decrease in the vehicle's velocity at unequal intervals of time, causing the vehicle to experience non-uniform acceleration.

Positive, Negative, And Zero Acceleration

• When the velocity of the body increases with time, it experiences positive acceleration. It means the velocity-time graph has a positive slope.

• When the velocity of a body decreases with time, it experiences negative acceleration. It means the velocity-time graph has a negative slope.

• When the velocity of a body is constant, or the body is at rest, it experiences zero acceleration. It means the velocity-time graph slope is zero.

Real-life Examples Of Acceleration

• Driving a car

• Riding a bicycle

• Airplane takeoff and landing

• Amusement park rides

• Lifts

• Moving Vehicles

• Falling objects ( falling under acceleration due to gravity)

in short, acceleration is defined as the rate of change of velocity of an object over time. This happens when an object speeds up, slows down(deceleration), or changes direction. It is the fundamental concept in Newton’s second law of motion. In this article, we discussed what is acceleration, its formula, different types of acceleration, important equations related to acceleration, and daily life examples of acceleration.

Also check-

• NCERT Exemplar Class 11th Physics Solutions
• NCERT Exemplar Class 12th Physics Solutions
• NCERT Exemplar Solutions for All Subjects

Solved Examples Based on Acceleration

Example 1: The displacement Vs time graph is given below which of the following conclusions is correct:

1) Velocity is constant
2) Velocity is continuously increasing

3) Acceleration is positive

4) Acceleration is negative

Solution :

As we know the slope of the displacement-time graph gives us velocity. Hence, a change in slope represents a change in velocity.

In the above figure, the velocity of the particle i.e. slope in the displacement vs time graph is decreasing.

Therefore, acceleration is negative.

Hence, the answer is option (4).

Example 2: Three position Vs time graph is shown in the figure. In which case acceleration is zero

1) I

2) II

3) III

4) None of these

Solution:

Zero Acceleration

When the final velocity is equal to the initial velocity.

For: V₁ = V₂

wherein

When a bus is moving with uniform velocity.

Since velocity is constant in case II hence acceleration = 0

Hence, the answer is option (2).

Example 3: Four velocity time graphs (Namely I, II, III, IV ) are shown in the figure. In which case is the acceleration uniform and positive?

Solution:

$ a=\frac{d v}{d t}$= slope in the V - t graph.

As it is clear II has a uniform and positive slope so it indicates uniform and positive acceleration.

Hence, the answer is option (2).

Example 4: The position of particles as a function of time $t$ is given by $x(t)=a t+b t^2-c t^3$ where $\mathrm{a}, \mathrm{b}$, and $\mathrm{c}$ are constants. When the particle attains zero acceleration, then its velocity will be :

1) $a+\frac{b^2}{4 c}$
2) $a+\frac{b^2}{3 c}$
3) $a+\frac{b^2}{c}$
4) $a+\frac{b^2}{2 c}$

Solution :

Given:

$\begin{aligned}
& x(t)=a t+b t^2-c t^3 \\
& v=\frac{d x}{d t}=a+2 b t-3 c t^2 \\
& a=\frac{d v}{d t}=2 b-6 c t \\
& a=0 \Rightarrow t=\frac{2 b}{6 c}=\frac{b}{3 c}
\end{aligned}$

$\begin{aligned}
& \text { So, Velocity at time } t=\frac{b}{3 c} \\
& V=a+2 b \times \frac{b}{3 c}-3 c \times \frac{b^2}{9 c^2} \\
& V=a+\frac{b^2}{3 c}
\end{aligned}$

Hence, the answer is the option (2).