Addition Of Vectors - Formula, Property, Component

The addition of vectors means putting two or more vectors with each other. In the addition of vectors, we are generally adding two or more vectors by using the mathematical operation of addition to obtaining a new length of the vector that is equal to the sum of two or more vectors. It is necessary for the students to understand all the properties of vectors carefully before they read about how to execute any mathematical operation with the vectors. Vectors can be used to do a large number of mathematical operations, addition is one of these operations. This way of adding two or more vectors is more competitive than scalar addition.

JEE Main 2025: Physics Formula | Study Materials | High Scoring Topics | Preparation Guide

JEE Main 2025: Syllabus | Sample Papers | Mock Tests | PYQs | Study Plan 100 Days

NEET 2025: Syllabus | High Scoring Topics | PYQs

This Story also Contains

1. Details About Addition of Vectors And Vector Addition Formula
2. Necessary Conditions For The Addition Of Vectors
3. Important Properties Of Vector Addition And Vector Addition Formula
4. Vector Addition Formula
5. Or
6. Laws Of Addition Of Vectors

As an example, If an object completes one complete revolution on a circular path perimeter of eighty miles then the total distance covered by an object is eighty miles. Whereas, there is not any displacement of an object. Now, let us know more about the addition of vectors and important details about the addition of vectors.

Details About Addition of Vectors And Vector Addition Formula

The vectors are always written with an alphabet and with an arrow over them and they are represented as a combination of direction and magnitude. The operation of adding two or more vectors together to form a new vector by using a sum is known as the addition of vectors. The addition of vectors can be completed by using two ways, either by using the triangle law or the parallelogram law.

• The two vectors having the same direction, then the sum of their magnitudes will be in the same direction and is always equal to the magnitude of their resultant vector.

• If the two vectors are in opposite directions to each other, then the magnitude of the resultant vector of these vectors is the difference of magnitude between these two vectors and the resultant vector’s direction is in the direction of a greater vector.

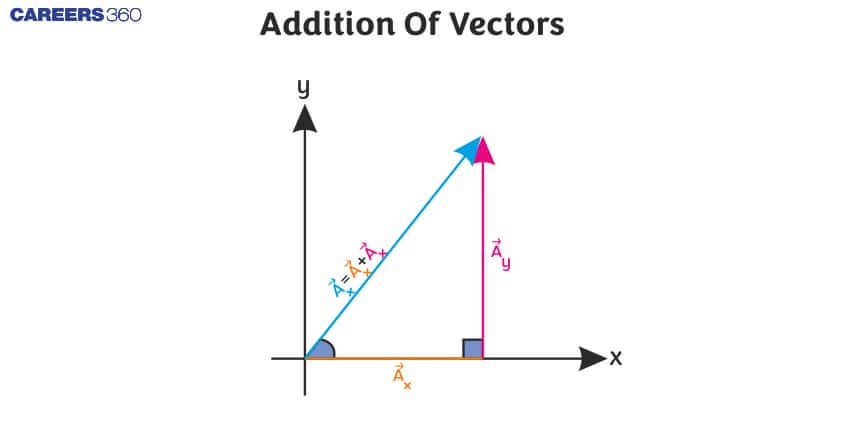
• By using vector addition, if two vectors, \vec{x} 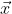and \vec{y} 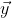have been added together, then their resultant vector can be expressed as \vec{R}= \vec{x}+ \vec{y}.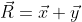

Necessary Conditions For The Addition Of Vectors

The necessary conditions for the addition of vectors are given as follows:-

1. Only vectors of the same kind can be added together. For example, the acceleration should be added only with the acceleration and not with the displacement.

2. We can not be able to add any vectors with any of the scalars, i.e. we can not add 5 with vector \vec{x}.

Important Properties Of Vector Addition And Vector Addition Formula

The addition of the vectors is fully different from the addition of numbers/scalars. There are the important properties which should be seriously considered during adding vectors:

1. Addition of vectors is always commutative which means that the order of vectors never affects the addition, i.e., If there are two vectors \vec{x} and \vec{y} and they are added together, then \vec{x}+ \vec{y}=\vec{y}+ \vec{x}.

2. The addition of vectors is also always associative which means that the mutual grouping of two or more vectors does not have any effect on the result when three or more vectors are added together, For example,

( \vec{x}+ \vec{y})+\vec{z}=\vec{x}+ (\vec{y}+\vec{z}) .

3. The vector addition is always distributive which means that the scalar times of the sum of any two vectors are equal to the sum of the scalar times of these two vectors individually, For example,

m ( \vec{x}+ \vec{y}) =m\vec{x}+ m \vec{y} .

4. Vector addition always satisfies the Existence of Identity property in which for a vector \vec{x}, \vec{x}+ \vec{0} = \vec{x} .

So, here \vec{0} is the additive identity of \vec{x}.

5. Vector addition has the Existence of inverse means that for any vector \vec{x}, \vec{x} + (-\vec{x}) = \vec{0} .

Hence, an additive inverse exists and -\vec{x} is the additive inverse of \vec{x}.

Vector Addition Formula

Or

Laws Of Addition Of Vectors

For the addition of vectors, there are three important formulas or laws or rules which are as follows :

1. Triangle law for addition of vectors:-

The triangle law for the addition of vectors states that if two vectors are represented such as any two sides of a triangle having the same order of magnitude and direction then the direction and magnitude of the resultant vector are denoted by the third side of that triangle.

Let a triangle PQR, such that

PQ= \vec{x} and QR= \vec{y} , then the resultant side RP= \vec{x} + \vec{y}.

If x= magnitude of \vec{x}

y= magnitude of \vec{y}

θ= angle between \vec{x} and \vec{y}

Then, the magnitude of \vec{x} + \vec{y}

is given by using the formula:- \sqrt{\mathrm{x}^2+\mathrm{y}^2+2\mathrm{x}\mathrm{y} \ Cos \theta } .

2. Parallelogram law for the addition of vectors:-

According to the Parallelogram law for the addition of vectors, if two vectors are represented as two adjacent sides of a parallelogram, both vectors should be pointing away from that common vertex then the resultant vector is denoted by the diagonal of that parallelogram passing through that same common vertex.

Here, PQ= \vec{x} and PS= \vec{y} are represented as the two adjacent sides of a parallelogram PQRS, and then their resultant vector is given by the diagonal PR of the parallelogram PQRS which originates through the same common vertex P.

3. Polygon Law of addition of vectors:-

According to the polygon law, if the number of vectors is represented in direction and magnitude as the sides of a polygon that are taken in the same order, then their resultant vector is represented by direction and magnitude such as the closing side of that polygon is taken in the opposite direction.