Centre Of Mass Of Solid Hemisphere

The centre of mass of a solid hemisphere is a fundamental concept in physics and mechanics that plays a crucial role in understanding the balance and stability of objects. A hemisphere, being a three-dimensional object, has its mass distributed unevenly, with a larger mass concentration towards the flat base. The centre of mass is the point where the mass of the hemisphere can be considered to be concentrated for the purpose of analyzing its motion and behaviour under various forces. In real life, this concept is applied in designing stable structures, such as domes or arches, where the centre of mass helps ensure that the weight is evenly distributed, providing stability and strength. For instance, understanding the centre of mass in a solid hemisphere can aid in the design of sports equipment like bowling balls or in the construction of spacecraft, where balance and stability are critical.

JEE Main 2025: Physics Formula | Study Materials | High Scoring Topics | Preparation Guide

JEE Main 2025: Syllabus | Sample Papers | Mock Tests | PYQs | Study Plan 100 Days

NEET 2025: Syllabus | High Scoring Topics | PYQs

This Story also Contains

1. Definition of Centre of Mass
2. Centre of mass for Solid Hemisphere
3. Solved Examples Based on Centre of Mass of Solid Hemisphere
4. Summary

Definition of Centre of Mass

The Centre of mass of a body is defined as a single point at which the whole mass of the body or system is imagined to be concentrated and all external forces are applied there. It is the point where if a force is applied it moves in the direction of the force without rotating.

Centre of Mass of a Continuous Distribution

The centre of mass of a continuous distribution is a key concept in physics that extends beyond simple, discrete systems to more complex, continuous ones. Unlike objects with distinct masses located at specific points, continuous distributions involve mass spread over a region, such as a rod, a plate, or even a fluid. To find the centre of mass in such cases, we consider each infinitesimally small mass element and calculate its contribution to the overall position.

$x_{c m}=\frac{\int x d m}{\int d m}, y_{c m}=\frac{\int y d m}{\int d m}, z_{c m}=\frac{\int z d m}{\int d m}$

Where dm is the mass of the small element. x, y, z are the coordinates of the dm part.

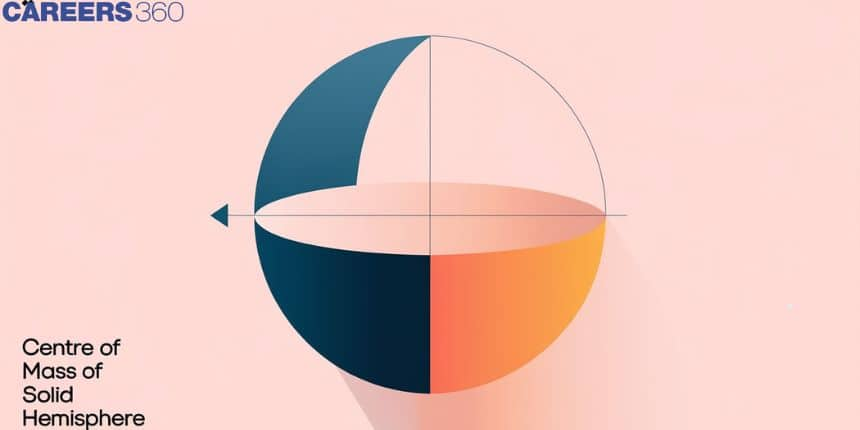
Centre of mass for Solid Hemisphere

Have a look at the figure of solid Hemisphere

Since it is symmetrical about the y-axis

So we can say that its $x_{c m}=0$ and $z_{c m}=0$

Now we will calculate its $y_{c m}$ which is given by

$y_{c m}=\frac{\int y \cdot d m}{\int d m}$

So Take a small elemental hollow hemisphere of mass dm of radius r as shown in figure.

Now have a look on the elemental hollow hemisphere of mass dm of radius r

Since our element mass is a hollow hemisphere its C.O.M is at (r/2)

Now $\quad d m=\rho d v=\rho\left(2 \pi r^2\right) d r$
Where, $\quad \rho=\frac{M}{\frac{2}{3} \pi R^3}$

$
y_{c m}=\frac{\int \frac{r}{2} d m}{M}=\frac{\int_0^R \frac{r}{2} * \frac{3 M}{2 \pi R^3} * 2 \pi r^2 d r}{M}=\frac{3}{2 R^3} * \int_0^R r^3 d r=\frac{3 R}{8}
$
So $y_{\mathrm{cm}}=\frac{3 R}{8}$ from base

Recommended Topic Video

Solved Examples Based on Centre of Mass of Solid Hemisphere

Example 1: A solid hemisphere (A) and a hollow hemisphere (B) Each having mass M are placed as shown in the diagram. What is the y-coordinate of the centre of mass of the system

1) $\frac{R}{16}$
2) $-\frac{R}{16}$
3) $\frac{R}{5}$
4) $-\frac{R}{5}$

Solution

For - A

$
O A_1=r_1=\frac{3 R}{8}
$
For - B

$
O B_1=r_2=\frac{-R}{2}
$
So using,

$
\begin{aligned}
& \text { So using, } y_{c m}=\frac{m_1 y_1+m_2 y_2}{m_1+m_2} \\
& y_{c m}=\frac{m_1 r_1+m_2 r_2}{m_1+m_2}=\frac{M \times \frac{3 R}{8}+M \times\left(\frac{-R}{2}\right)}{2 M}=-\frac{1}{16} R
\end{aligned}
$

Hence, the answer is the option (2).

Example 2: As shown in Figure A solid hemisphere is given Mass M and Radius R and its centre at the origin.

So by increasing its volume uniformly its COM will

1) Shift toward origin

2) Shift away from origin

3) Remains same

4) None of these

Solution

For solid hemisphere

Volume $\propto R^3$
So on increasing its volume uniformly its $R$ will increase
For solid hemisphere
$y_{c m} \propto R$
So on increasing $R$ its $y_{\mathrm{cm}}$ will increase
This means it will Shift away from its origin.

Hence, the answer is the option (2).

Example 3: The centre of mass of a solid hemisphere of radius 8 cm is x cm from the centre of the flat surface. The value of x is _____

1) 3

2) 4

3) 5

4) 6

Solution

$\begin{aligned} & x=\frac{3 R}{8}=3 \mathrm{~cm} \\ & x=3\end{aligned}$

Hence, the answer is the option (1).

Summary

The centre of mass of a solid hemisphere is a crucial concept in physics, helping to understand the distribution of mass and stability of objects. It is calculated using integrals for continuous mass distributions, with the centre of mass for a solid hemisphere located at a distance of 3R8\frac{3R}{8}83R from the flat base. This concept is applied in various real-life scenarios, such as designing stable structures and equipment, where balance is essential.