Compound Microscope

A compound microscope is an essential tool in the field of biology, allowing us to explore the intricate details of microscopic organisms and cellular structures. This optical instrument uses a combination of lenses to magnify small objects, providing a detailed view that is impossible to achieve with the naked eye. In everyday life, compound microscopes play a crucial role in medical laboratories for diagnosing diseases, in forensic science for analyzing evidence, and in research facilities for advancing scientific knowledge. For instance, they enable scientists to study bacteria and viruses, leading to the development of vaccines and treatments that save millions of lives. By unveiling the hidden world of the microscopic, compound microscopes bridge the gap between the unseen and the understood, making them indispensable in both scientific discovery and practical applications. In this article we will discuss the concept of Compound microscope and some solved examples for better clarity.

JEE Main 2025: Physics Formula | Study Materials | High Scoring Topics | Preparation Guide

JEE Main 2025: Syllabus | Sample Papers | Mock Tests | PYQs | Study Plan 100 Days

NEET 2025: Syllabus | High Scoring Topics | PYQs

This Story also Contains

1. What is a Compound Microscope?
2. Case 1: The final image is formed at D:
3. $m_D=\frac{L}{f_o}\left(1+\frac{D}{f_e}\right)$..
4. Case 2: The final image is formed at $\infty$ :
5. Magnification $m_{\infty}=\frac{v_0}{u_0} \cdot \frac{D}{f_e}$ and length of tube $L_{\infty}=v_0+f_e$ In terms of length $m_{\infty}=\frac{\left(L_{\infty}-f_o-f_e\right) D}{f_o f_e}$.
6. Solved Examples Based on Compound Microscope
7. Summary

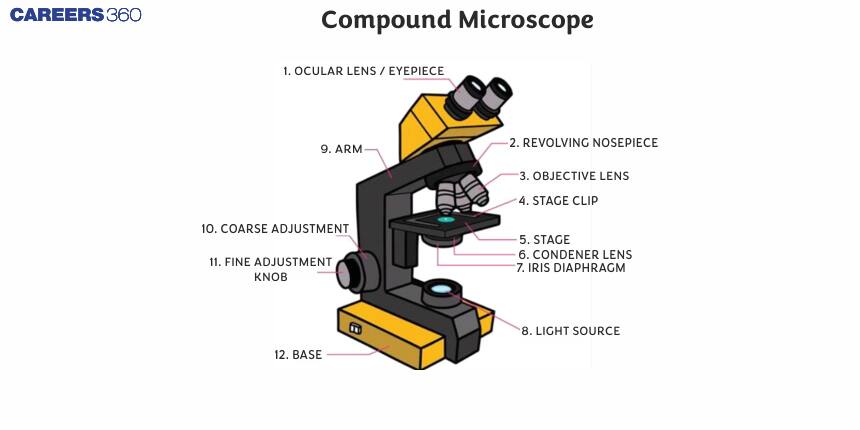
What is a Compound Microscope?

A compound microscope is made up of two converging lenses called objective and eye lenses. It is used to view magnified images of small objects on a glass slide. It can achieve higher levels of magnification than stereo or other low-power microscopes and reduce chromatic aberration.

$f_{\text {eyclens }}>f_{\text {objective }} \text { and }(\text { diameter })_{\text {eyelens }}>(\text { diameter })_{\text {objective }}$
The intermediate image is real and enlarged. The final image is magnified, virtual and inverted.

Here in the diagram

$u_o=$ Distance of object from objective (o),
$v_o=$ Distance of image $\left(A^{\prime} B^{\prime}\right)$ formed by objective from objective,
$u_e=$ Distance of $A^{\prime} B^{\prime}$ from eye lens,
$v_\epsilon=$ Distance of final image from eye lens,
$f_o=$ Focal length of objective,
$f_e=$ Focal length of eye lens.

Here are two cases

Case 1: The final image is formed at D:

Magnification $m_D=\frac{v_o}{u_o}\left(1+\frac{D}{f_e}\right)$ and length of the microscope tube (distance between two lenses) is $L_D=v_e+u_e$
Generally, the object is placed very near to the principal focus of the objective hence $u_o \equiv f_o$ The eyepiece is also of small focal length and the image formed by the objective is also very near the eyepiece.

So $v_o \equiv L_D$ is the length of the tube.
Hence, we can write

$m_D=\frac{L}{f_o}\left(1+\frac{D}{f_e}\right)$..

Case 2: The final image is formed at $\infty$ :

Magnification
$m_{\infty}=\frac{v_0}{u_0} \cdot \frac{D}{f_e}$ and length of tube $L_{\infty}=v_0+f_e$
In terms of length $m_{\infty}=\frac{\left(L_{\infty}-f_o-f_e\right) D}{f_o f_e}$.

- For large magnification of the compound microscope, both $f_o$ and $f_e$ should be small.
- If the length of the tube of the microscope increases, then its magnifying power increases.
- The magnifying power of the compound microscope may be expressed as $M=m_o \times m_e$ where $m_0$ is the magnification of the objective and $m_e$ is magnifying the of eyepiece.

Recommended Topic Video

Solved Examples Based on Compound Microscope

Example 1: The separation L between the objective (f=0.5cm) and the eyepiece (f=5cm) of a compound microscope is 7cm. The angular magnification produced by this microscope when the eye is least strained is:

1) -5

2) -10

3) -15

4) -20

Solution:

Compound Microscope

$m=-\frac{v_o}{u_o} \cdot \frac{D}{u_e}$
wherein

$v_o$ and $u_o$ is the distance from the objective.
$u_e$ Distance from the eyepiece.

Maximum magnification
$
m=-\frac{v_o}{u_0}\left(1+\frac{D}{f_e}\right)
$

Since the eye is least strained hence the final image will form at infinity. In such a case, an image formed by the object should form at the focus of the eyepiece.

$
\begin{aligned}
& v_0=7 \mathrm{~cm}-5 \mathrm{~cm}=2 \mathrm{~cm} \quad f_0=0.5 \mathrm{~cm} \\
& \frac{1}{u}=\frac{1}{v_0}-\frac{1}{f_0}=\frac{1}{2}-\frac{1}{0.5}=\frac{-3}{2} \\
& \text { or }^u=\frac{-2}{3} \mathrm{~cm}
\end{aligned}
$

Angular magnification
$
m=\frac{v_0}{u_0} \cdot \frac{D}{f_e}=\frac{-25}{5}=-15 \mathrm{~cm}
$

Hence, the answer is the option (3).

Example 2: In a compound microscope, the focal length of the objective lens is 1.2 cm and the focal length of the eyepiece is 3.0 cm. When an object is kept at 1.25 cm in front of the objective, the final image is formed at infinity. The magnifying power of the compound microscope should be :

1) 200

2) 100

3) 400

4) 150

Solution:

Maximum magnification
$
m=-\frac{v_o}{u_0}\left(1+\frac{D}{f_e}\right)
$
$
\begin{aligned}
& \quad f_e=3 \mathrm{~cm} \\
& f_0=1.2 \mathrm{~cm} \\
& u_0=1.25 \mathrm{~cm}, v_e=\infty \\
& \Rightarrow \frac{1}{V_0}=\frac{1}{f_0}+\frac{1}{u_0}=\frac{1}{1.2}-\frac{1}{1.25} \\
& V_0=30 \mathrm{~cm} \\
& \qquad \frac{v_0}{u_0} \cdot \frac{D}{f_e}=\frac{30}{1.25} \times \frac{25}{3}=200 \\
& \text { }
\end{aligned}
$

Hence, the answer is the option (1).

Example 3: The focal length of the objective and the eyepiece of the compound microscope are 2cm and 3cm respectively. The distance between the objective and the eyepiece is 15 cm. The final image formed by the eyepiece is at infinity the distance (in cm) of the object and image produced by the objective, measured from the objective lens respectively.

1) 2.4 and 12

2) 2.4 and 15

3) 2.3 and 3

4) 2.3 and 12

Solution:

Length of the compound microscope
$
L=v_o+u_e
$
$v_o=$ Image distance from the objective.
$u_e=$ Object distance from the eyepiece
$
\begin{aligned}
& f_0=2 \mathrm{~cm} \quad f_e=3 \mathrm{~cm} \\
& \mathrm{I}=15 \mathrm{~cm}
\end{aligned}
$

The final image is formed at infinity hence, the image formed by the objective is at the focal point of the eyepiece

$
\begin{aligned}
& u_e=f_e=3 \mathrm{~cm} \\
& v_o=l-f_e=12 \mathrm{~cm}
\end{aligned}
$

For objective:
$
\begin{array}{ll}
v_o=12 c m & f_o=2 \mathrm{~cm} \\
\frac{1}{v_0}-\frac{1}{u_0}=\frac{1}{f_0} & \\
\frac{1}{12}-\frac{1}{u_0}=\frac{1}{2} &
\end{array}
$
or
$
\frac{1}{u_0}=\frac{1}{12}-\frac{1}{2}=\frac{1-6}{12}
$
or,
$
u_0=-2.4 \mathrm{~cm} \quad v_0=12 \mathrm{~cm}
$

Hence, the answer is the option (1).

Example 4: If we used a magnification of 375 from a compound microscope of tube length 150mm and an objective of focal length 5mm, the focal length of the eyepiece should be close to :

1) 12mm

2) 33mm

3) 22mm

4) 2mm

Solution:

The magnification is given by
$
\begin{aligned}
& M=\frac{L}{f_0}\left(1+\frac{D}{f_c}\right) \\
& \Rightarrow 375=\frac{150}{5}\left(1+\frac{25}{f_e}\right) \\
& \Rightarrow f_e=22 \mathrm{~mm}
\end{aligned}
$

Hence the option (3) is correct.

Example 5: A compound microscope consists of an objective lens of focal length 1cm and an eyepiece of focal length 5 cm with a separation of 10 cm. The distance between an object and the objective lens, at which the strain on the eye is minimum is $\frac{n}{40} \mathrm{~cm}$ The value of n is ______.

1) 50

2) 100

3) 150

4) 200

Solution:

Image by objective is formed at the focus of the eye-piece
$\therefore$ For objective, $v=5, u, f=1 \mathrm{~cm}$
$
\begin{array}{r}
\frac{1}{5}-\frac{1}{u}=\frac{1}{1} \Rightarrow \frac{1}{5}-1=\frac{1}{u} \\
\therefore \quad|u|=\frac{5}{4} \mathrm{~cm} \Rightarrow|u|=\frac{50}{40} \mathrm{~cm} \\
\therefore \quad n=50
\end{array}
$

Summary

A compound microscope, utilizing two converging lenses—objective and eyepiece—provides high magnification of small objects. The final image produced is magnified, virtual, and inverted. For maximum magnification, the focal lengths of both lenses should be small, and the microscope's tube length should be optimal. Solved examples illustrate the calculations for determining magnification and focal lengths in different scenarios, emphasizing the importance of precise measurements for accurate results.