Drift Velocity - Meaning, Formula, FAQs

The free electrons in a conductor are always in a continuous random motion due to the thermal energy of the conductor and the average speed at which free electrons 'drift' in the presence of an electric field is called drift velocity. In this article, we will discuss what is drift velocity, the drift velocity formula, the relation between current density and drift velocity, the relation between drift velocity and electric current, and factors affecting drift velocity. Over the last ten years of the JEE Main exam (from 2013 to 2023), nine questions have been asked on this concept.

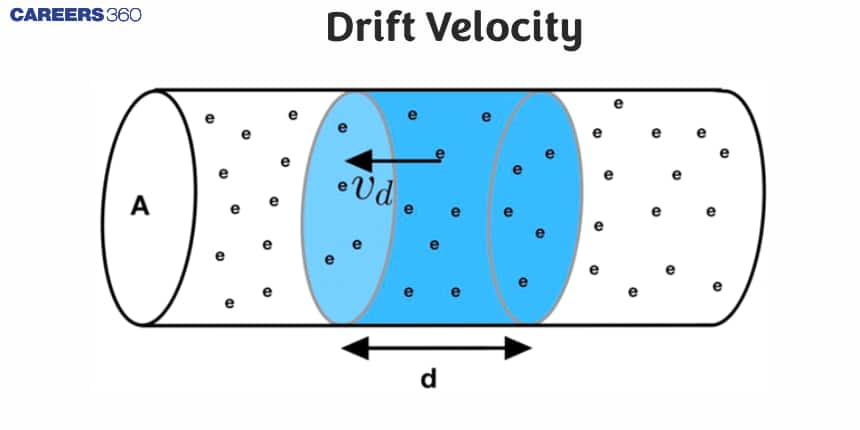
What is Drift Velocity Class 12?

Drift velocity definition: Drift velocity is the average velocity that a particle such as an electron attains in a material due to an electric field.

Drift velocity definition in terms of an electron: The drift velocity of an electron in a conductor is the average velocity an electron acquires in the presence of an electric field.

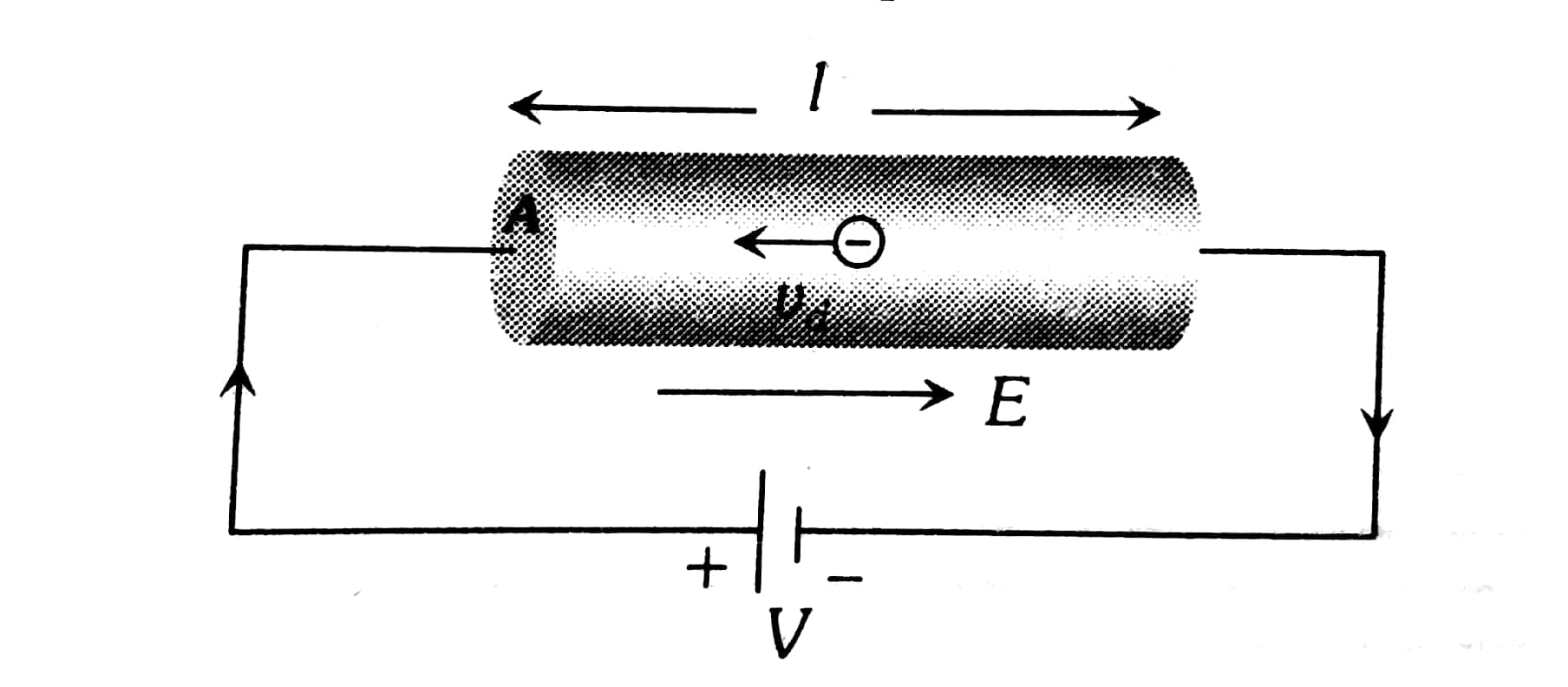
Drift Velocity Formula Class 12

$$V_d=\frac{I}{n A e}$$

where,

• $V_d$ is the drift velocity of the electrons
• $I$ is the electric current flowing through the conductor
• $n$ is the number density of free electrons
• $A$ is the cross-sectional area of the conductor
• $e$ is the charge of an electron

Drift velocity in terms of electric field ($E$) and mobility of electrons ($\mu$)

$$
V_d=\mu E
$$

$V_d$ is directly proportional to $E$:

$V_d \propto E$ when the temperature is constant, the greater the electric field larger the drift velocity.

Drift velocity varies inversely with the area of the cross-section

Also, read

• NCERT Solutions for All Subjects
• NCERT Notes for all subject
• NCERT Exemplar Solutions for All Subjects

SI Unit of Drift Velocity

The SI unit of drift velocity is meters per second ( $\mathrm{m} / \mathrm{s}$ ).

$V_d=\frac{I}{n A e}$

substituting unit of each term

$V_d=\frac{\mathrm{A}}{\left(\mathrm{m}^{-3}\right)\left(\mathrm{m}^2\right) \mathrm{C}}$

$V_d=\frac{\mathrm{A}}{\mathrm{m}^{-1} \cdot \mathrm{C}}$

$V_d=\frac{\mathrm{C} / \mathrm{s}}{\mathrm{m}^{-1} \cdot \mathrm{C}}$

Thus simplifying we get

$$V_d=\frac{\mathrm{m}}{\mathrm{s}}$$

Relation Between Current Density and Drift Velocity

The drift velocity is directly proportional to the current density.

$$
J=n e V_d
$$

where,

$J$ is the Current Density
$n$ is the number density of electrons in a conductor
$e:$ is the charge of an electron
$V_d$ is the drift velocity

Relation Between Drift Velocity and Electric Current

The drift velocity is directly proportional to the electric current.

$$
I=n A e v_d
$$

hence,

$$
I \propto v_d
$$

Factors Affecting Drift Velocity

1. Electric field
2. Charge of the electron
3. The number density of electrons
4. Temperature
5. Mobility of electron

Important Terms Related to Drift Velocity

• Relaxation Time (τ):

The time interval between two successive collisions of electrons with the Positive ions.

• Mean Free Path:

The path between two consecutive collisions is called the free path. The average length of these free paths is called the “Mean Free Path”.

• Mobility Of An Electron:

It is the drift velocity of an electron per unit electric field. It indicates how easily an electron can pass through the material (conductor or semiconductor) in the presence of an electric field.

$$\mu=\frac{v_d}{E}$$

Recommended Topic Video

Solved Examples Based on Drift Velocity

Example 1: Drift speed of electrons, when 1.5 A of current flows in a copper wire of cross-section is v. If the electron density in copper is 9×1028/m3 the value of v in mm/s is close to (Take charge of an electron to be =1.6×10−19C )

1) 0.02

2) 3

3) 2

4) 0.2

Solution:

$\begin{gathered}I=n e A V_d \\ V_d=\frac{I}{n e A}=\frac{9 \times 10^{28} \times 1.6 \times 10^{-19} \times 5 \times 10^{-6}}{1}=0.02 \times 10^{-3} \mathrm{~m} / \mathrm{s}=0.02 \mathrm{~mm} / \mathrm{sec}\end{gathered}$

Hence, the answer is the option (1).

Example 2: A current of 10 A exists in a wire of a cross-sectional area of 5 mm2 with a drift velocity of 2×10−3 ms−1. The number of free electrons in each cubic meter of the wire is

1) 1×1023
2) 625×1025
3) 2×1025
4) 2×106

Solution:

\begin{aligned}
&i=10 \mathrm{~A}, A=5 \mathrm{~mm}^2=5 \times 10^{-6} \mathrm{~m}^2 \text { and } v_d=2 \times 10^{-3} \mathrm{~m} / \mathrm{s}\\
&\text { We know, }\\
&\begin{gathered}
i=n e A v_d \\
10=n \times 1.6 \times 10^{-19} \times 5 \times 10^{-6} \times 2 \times 10^{-3} \Rightarrow n=0.625 \times 10^{28}=625 \times 10^{25}
\end{gathered}
\end{aligned}

Hence, the answer is the Option(2).

Example 3: When a 5V potential difference is applied across a wire of length 0.1 m, the drift speed of electrons is 2.510 ^-4 ms ^-1. If the electron density in the wire is 810²⁸ m ^-3, the resistivity of the material is close to :

1) 1.6×10−8Ωm
2) 1.6×10−7Ωm
3) 1.6×10−6Ωm
4) 1.6×10−5Ωm

Solution:

\begin{aligned}
& I=A n e v_{-} d \\
& \Rightarrow V=R A n e v_{-} d \\
& \Rightarrow V(\rho I / A)=A n e v_{-} d \\
& \Rightarrow V A / \rho I=A n e v_{-} d \\
& \Rightarrow \rho=V / I^*\left(1 / n v_{-} d\right) \\
& \Rightarrow \rho=V I /\left(n e v_{-} d\right) \\
& =50.1 \times\left(8 \times 10^{\wedge} 28\right) \times\left(1.6 \times 10^{\wedge}(-19)\right) \times\left(2.5 \times 10^{\wedge}(-4)\right) \\
& \Rightarrow \rho=1.5625 \times 10^{\wedge}(-5) \Omega \mathrm{m} \approx 1.6 \times 10^{\wedge}(-5) \Omega \mathrm{m}
\end{aligned}

Hence, the answer is the option (4).

Example 4: An electron moving in a zigzag path travels a displaces by 0.2 mm in 10 seconds. Its drift speed is (in m/sec)

1) 2×10^-5

2) 10^-5

3) 2×10^-4

4) 10^-4

Solution:

Drift velocity

Drift velocity is the average velocity that a particle such as an electron attains in a material due to an electric field.

wherein

Drift Velocity = Displacement/time

Displacement $=.2 \mathrm{~mm}=2 \times 10^{-4} \mathrm{~m}$

Time = 10 sec

Drift Velocity $V_d=2 \times 10^{-5} \mathrm{~m} / \mathrm{sec}$

Hence, the answer is option (1).

Example 5: Which of the following is correct regarding relaxation time?

1) Relaxation time increases with increase in temperature

2) Relaxation time decreases with increase in temperature

3) A decrease in relaxation time causes a decrease in resistivity

4) Conductivity is independent of Relaxation time

Solution:

Relaxation time ($\tau$)⟶ The time interval between two successive collisions of electrons with the ions/ atoms.

As with an increase in temperature drift velocity increases which will lead to an increase in the rate of collision and hence relaxation time decreases.

Hence, the answer is the option (2).