Electric Flux Through Cone Or Disc

Imagine you're standing in the rain, holding an umbrella. The amount of water that passes through the surface of the umbrella depends on how it's oriented and how much rain is falling. In the world of electric fields, a similar concept is known as electric flux, which measures how much of the electric field passes through a given surface.

JEE Main 2025: Physics Formula | Study Materials | High Scoring Topics | Preparation Guide

JEE Main 2025: Syllabus | Sample Papers | Mock Tests | PYQs | Study Plan 100 Days

NEET 2025: Syllabus | High Scoring Topics | PYQs

This Story also Contains

1. The electric flux through a cone or disc
2. Solved Examples Based on Electric flux through Cone or Disc
3. Example 1: A cylinder of radius R and length L is placed in a uniform electric field E parallel to the cylinder axis. The total flux for the surface of the cylinder is given by 1) 2πR2E 2) πR2/E 3) (πR2−πR)/E 4) zero
4. Summary

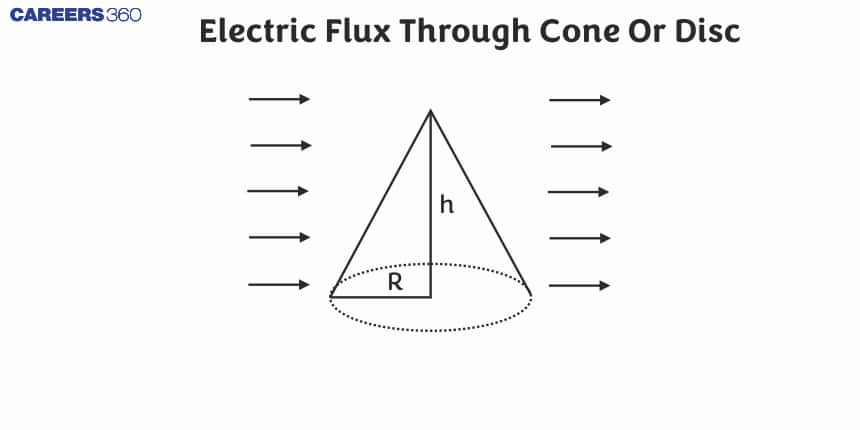
When considering surfaces like a cone or a disc, the electric flux through them depends on the shape of the surface and its orientation relative to the electric field. For a cone, the flux might vary depending on the angle of the cone relative to the field, while for a disc, it depends on whether the disc is perpendicular or at an angle to the field lines. Understanding this concept is essential in many areas of physics, particularly in applying Gauss’s Law, which helps simplify complex electric field calculations. In this article, we'll delve into how electric flux is calculated through a cone or disc and explore some practical examples to help illustrate the concept.

The electric flux through a cone or disc

There are several cases for electric flux calculation. In this concept, we will discuss one very important and complex case which is ''Electric flux through cone or disc''. For this let us consider a point charge at a distance 'a' from a disc of radius R as shown in the given figure.

Let us consider an elemental ring of radius " $y$ " and width "dy". The area of this ring(strip) is $dA=2\pi ydy$.

Electric field due to $q$ at this elemental ring,
$E=\frac{1}{4\pi {\epsilon }_0}\frac{q}{(a^2+y^2)}$

If $d\varphi$ is the flux passing through this elemental ring, we have
$\begin{array}{rl}d\varphi & =EdA\cos \theta \\ & =(\frac{1}{4\pi {\epsilon }_0}\cdot \frac{q}{(a^2+y^2)})(2\pi ydy)\left(\frac{a}{{(a^2+y^2)}^{1/2}}\right)\\ & =\frac{qa}{2{\epsilon }_0}\cdot \left(\frac{ydy}{{(a^2+y^2)}^{3/2}}\right)\end{array}$

To obtain total flux, we should integrate this expression over the whole area of the ring, So the total flux can be given as

$\varphi =\int d\varphi =\frac{qa}{2{\epsilon }_0}{\int }_0^R\frac{ydy}{{(a^2+y^2)}^{3/2}}$

On integration we get,
$\varphi =\frac{q}{2{\epsilon }_0}(1-\frac{a}{\sqrt{a^2+R^2}})$

Recommended Topic Video

Solved Examples Based on Electric flux through Cone or Disc

Example 1: A cylinder of radius $R$ and length $L$ is placed in a uniform electric field $E$ parallel to the cylinder axis. The total flux for the surface of the cylinder is given by
1) $2\pi R^{2}E$
2) $\pi R^2/E$
3) $(\pi R^2-\pi R)/E$
4) zero

Solution:

Electric field E through any area A
$\begin{array}{rl}& \varphi =\overrightarrow{E}\cdot \overrightarrow{A}=EA\cos \mathrm{\Theta }\\ & \text{S.I unit}-(\text{volt})m\text{or}\frac{N-m^2}{c}\end{array}$

wherein

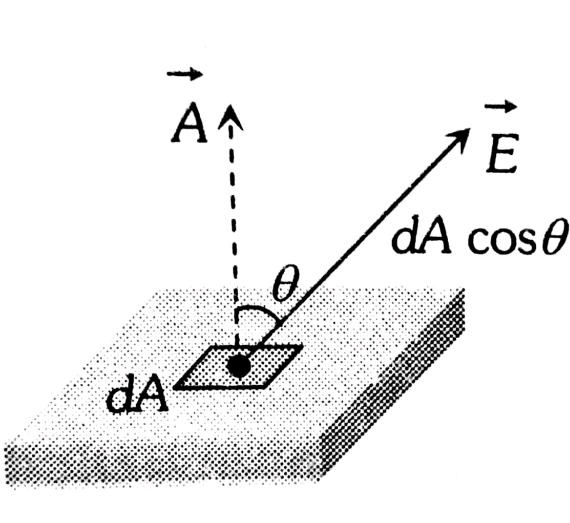
Flux through surface $\mathrm{A}{\varphi }_A=E\times \pi R^2$ and ${\varphi }_B=-E\times \pi R^2$
Flux through curved surface $C=\int Eds=\int Eds\cos {90}^{\circ }=0$
Total flux through cylinder $={\varphi }_A+{\varphi }_B+{\varphi }_C=0$

Hence, the answer is option (4).

Example 2: The Electric field at a point varies as $r^0$ for
1) An electric dipole
2) A point charge
3) A plane infinite sheet of charge
4) A line charge of infinite length

Solution:
Electric field E through any area A
$\varphi =\overrightarrow{E}\cdot \overrightarrow{A}=EA\cos \mathrm{\Theta }$
$\text{S.I unit}-(\text{volt})m\text{or}\frac{N-m^2}{c}$

wherein

$E=\frac{\sigma }{\left(2{\epsilon }_0\right)}$

Hence, the answer is option (3).

Example 3: Shown in the figure are two point charges $+Q$ and $-Q$ inside the cavity of a spherical shell. The charges are kept near the surface of the cavity on opposite sides of the centre of the shell. If ${\sigma }_1$ is the surface charge on the inner surface and ${\mathrm{Q}}_1$ net charge on it and ${\sigma }_2$ the surface charge on the outer surface and $Q_2$ net charge on it then :

$\begin{array}{rl}& \text{1)}{\sigma }_1\ne 0,Q_{1\ne 0}\\ & {\sigma }_2\ne 0,Q_2\ne 0\\ & \text{2)}{\sigma }_1\ne 0,Q_1=0\\ & {\sigma }_2\ne 0,Q_2=0\\ & \text{3)}{\sigma }_1\ne 0,Q_1=0\\ & {\sigma }_2=0,Q_2=0\\ & \text{4)}{\sigma }_1=0,Q_1=0\\ & \text{б}=0,Q_2=0\end{array}$

Solution:

if the Electric field is variable

$\varphi =\int \overrightarrow{E}\cdot d\overrightarrow{A}$

Inside the cavity, the net charge is zero
$\therefore Q_1=0\text{and}{\sigma }_1\ne 0$

There is no effect of point charge and induced charge on the inner surface and the outer surface
$\therefore Q_2=0\text{and}{\sigma }_2=0$

Example 4: If the electric flux entering and leaving an enclosed surface respectively is ${\varphi }_1$ and ${\varphi }_2$ the electric charge inside the surface will be
1) $({\varphi }_1+{\varphi }_2){\epsilon }_0$
2) $({\varphi }_2-{\varphi }_1){\epsilon }_0$
3) $({\varphi }_1+{\varphi }_2)/{\epsilon }_0$
4) $(2{\varphi }_2+{\varphi }_1)/{\epsilon }_0$

Solution:

if the Electric field is variable

$\begin{array}{rl}& \varphi =\int \overrightarrow{E}\cdot d\overrightarrow{A}\\ & {\varphi }_{\text{net}}=1/{\epsilon }_0\times Q_{enc}\Rightarrow Q_{enc}=({\varphi }_2-{\varphi }_1){\epsilon }_0\end{array}$

Hence, the answer is the option (2).

Example 5: The inward and outward electric flux for a closed surface in units of $N{\mathrm{m}}^2/\mathrm{C}$ are respectively $8\times {10}^3$ and $4\times {10}^3$. Then the total charge inside the surface is [where ${\epsilon }_0=$ permittivity constant
1) $4\times {10}^3\mathrm{C}$
2) $-4\times {10}^3\mathrm{C}$
3) $-4\times {10}^3\mathrm{C}/\epsilon$
4) $-4\times {10}^3{\epsilon }_{0}C$

Solution:

if the Electric field is variable

$\varphi =\int \overrightarrow{E}\cdot d\overrightarrow{A}$

By Gauss's law $\varphi =\left(Q_{\text{enclosed}}/{\epsilon }_0\right)$
$Q_{\text{enclosed}}=\varphi {\epsilon }_0=(-8\times {10}^3+4\times {10}^3){\epsilon }_0$

Hence, the answer is the option (4).

Summary

The electric flux through a surface is a measure of the electric field passing through that surface. For a cone or disc, this may be represented by pondering on the number of electric field lines that pierce such shapes. When a uniform electric field is at right angles to the base of a cone or disk, the product of the electric field intensity and the area of the base gives the value of flux.