Enthalpy - Definition, Endothermic, Exothermic Reaction, Units, FAQs

We know what thermodynamics is and how important it is, so therefore one should learn about one of the concepts in thermodynamics. The enthalpy is used to determine the total heat energy present in a system; it tells whether a reaction absorbs energy or releases it. If you watch closely, then you will find out that enthalpy changes are very much related to the phenomena seen in our daily lives, like the warmth caused when burning wood, the cooling effect while melting ice, etc. In the following article, this amazing topic is defined by the types of reactions, units, and commonly asked questions.

JEE Main 2025: Physics Formula | Study Materials | High Scoring Topics | Preparation Guide

JEE Main 2025: Syllabus | Sample Papers | Mock Tests | PYQs | Study Plan 100 Days

NEET 2025: Syllabus | High Scoring Topics | PYQs

Enthalpy Definition

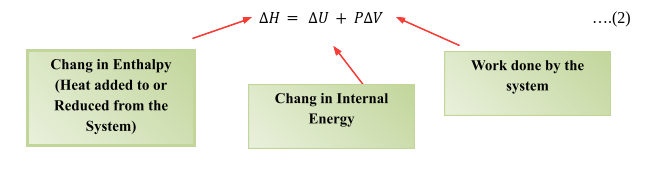
Enthalpy in thermodynamics is defined as the addition of the internal energy of the system and the product of its pressure and volume. Enthalpy meaning also represents the total heat change under constant pressure and temperature. What is meant by enthalpy for the particular thermodynamics system is the total energy of the system including internal energy and flow energy; the thermodynamics system is the system which is under the thermodynamics observation. The term enthalpy is the state function which means it does not depend on the path followed to complete the process on the contrary it only depends upon the final configurations of the pressure, volume and internal energy. Mathematically enthalpy (H), where the symbol of the enthalpy is H can be written as follows

$\Delta H=\Delta U+P \Delta V$

Where,
$\Delta H$ is the change in enthalpy of the system
P is pressure and
$\Delta \mathrm{V}$ is volume
$\Delta U$ is equal to the total internal energy of the system

SI Unit of the enthalpy is Joule.

Also read -

• NCERT Solutions for All Subjects
• NCERT Notes For All Subjects
• NCERT Exemplar Solutions for All Subjects

The second term in the enthalpy equation is related to the work required to establish the physical dimension of the system. The product of pressure P and volume V in enthalpy H is sometimes also called the flow work. Flow work ( PV ) is essentially defined as the work required maintaining an uninterrupted flow through a controlled volume. Flow work can be viewed on the control volume system.

The change in enthalpy for the system can be written as

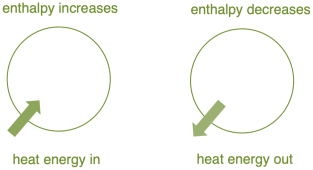
Depending upon the value of $\Delta H$ the Process or the reaction can be of two types
1. If $\Delta H>0$ The process is called the Endothermic Process (Absorbs Heat)
2. If $\Delta \mathrm{H}<\mathrm{O}$, The Process is called the Exothermic Process (Expels Heat)

It can be said from the above change in enthalpy equation that when the heat is added to the system it have twofold effect on the system. First is the increase in internal energy of the system, which is nothing but the sum of potential energy and kinetic energy of atoms and molecules and other is to do flow work i.e expansion of the system against the pressure applied by the atmosphere.

Units of the Enthalpy

As stated above, Enthalpy is nothing but the determination of heat flow at a constant pressure.

In other words:

In other words:
$\Delta \mathrm{H}=\mathrm{Qp}$
Where Q is Heat in Joules.
We can derive this by starting from the following expression:

$$
\Delta H=\Delta U+P \Delta V .
$$

$\qquad$

And $\Delta P V=P \Delta V+V \Delta P+\Delta V \Delta P$. $\qquad$

So, from the first law of thermodynamics,
$\Delta U=Q-W$. $\qquad$
And $W=-P \Delta V$. $\qquad$
$H=$ is enthalpy,
Hence,
$\mathrm{U}=$ internal energy,
$\mathrm{Q}=$ heat flow,

$$
\Delta H=Q-P \Delta V+P \Delta V+V \Delta P+\Delta P \Delta V
$$

$\qquad$
$\mathrm{w}=\mathrm{work}$,
But the pressure is assumed to be constant, so:
$\mathrm{P}=$ pressure and V is volume

$$
\Delta H=Q .
$$

$\qquad$
Hence, as a result; at constant pressure:
$\Delta \mathrm{H}=\mathrm{Qp}$. $\qquad$ (10)

So, enthalpy must then be in Joules, more often kJ is used.
Other units of the enthalpy are Joule $=\mathrm{kg} \cdot \mathrm{m}^2 \mathrm{~s}^2=\mathrm{N} \cdot \mathrm{m}$

Specific Enthalpy (h)

Specific enthalpy is defined as the total enthalpy of the system per unit mass of the system.

$$
h=H / m(\mathrm{~J} / \mathrm{kg})
$$
$$
\mathrm{h}=\mathrm{u}+\mathrm{pv}(\mathrm{~J} / \mathrm{kg})
$$

Enthalpy Change

From the first of thermodynamics, we have

$$
\Delta Q=\Delta U+\Delta W
$$

or, $\Delta U=\Delta Q-\Delta W$
Clearly, we know that Work can also be expressed as, $\Delta \mathrm{W}=\mathrm{P} \Delta \mathrm{V}$
Substituting this in the above equation we obtained,

$$
\Delta U=\Delta Q-P \Delta V-(1)
$$
Now, from the second law of thermodynamics, we have,

$$
\Delta Q=T \Delta S--(\text { expression of entropy) }
$$
On substituting the value of $Q$ from equation (1), we obtain,

$$
\Delta U+P \Delta V=T \Delta S
$$

or, $\Delta \mathrm{U}=\mathrm{T} \Delta \mathrm{S}-\mathrm{P} \Delta \mathrm{V}-(2)$
Now, adding VAP on LHS and RHS of Equation (2), we obtained

$$
\begin{aligned}
& \Delta U+V \Delta P=T \Delta S-P \Delta V+V \Delta P \\
& \Delta U+P \Delta V+V \Delta P=T \Delta S+V \Delta P
\end{aligned}
$$

$$
\begin{aligned}
& \Delta U+\Delta(P V)=T \Delta S+V \Delta P \\
& \Delta(U+P V)=T \Delta S+V \Delta P-(3)
\end{aligned}
$$
Clearly, $\mathrm{H}=\mathrm{U}+\mathrm{PV}$ (definition of enthalpy) -(4)
From equation (3) and (4) we obtained,

$$
\Delta H=T \Delta S+V \Delta P
$$
This is the required expression for the change in enthalpy of the system and is a function of entropy and Pressure.

Enthalpy of Reaction

One way to report the heat absorbed or released is to compile a large number of reference tables listing all possible enthalpy changes of chemical reactions, which will require a lot of effort. Luckily, since enthalpy is a state function, we only need to know the original and finishing state of the reaction. This allows us to use a relatively small set of tabular data to calculate the enthalpy change of almost any possible chemical reaction, for example:

• Enthalpy of combustion ( $\Delta \mathrm{H}_{\text {comb }}$ ): The enthalpy change that occurs during a combustion reaction. Enthalpy change is measured for the combustion of almost all substances burned in oxygen; these values are usually expressed as the enthalpy of combustion per mole of a substance.
• The enthalpy of fusion $\left(\Delta \mathrm{H}_{\text {fus }}\right)$: changes with the enthalpy of the fusion of 1 mole of matter. The enthalpy change that accompanies the fusion or fusion of 1 mole of matter; these values are measured for almost all elements and most simple compounds.
• Enthalpy of Vaporization $\left(\Delta H_{\text {vap }}\right)$: The change in enthalpy associated with the vaporization of 1 mole of a substance. The enthalpy change that accompanies the evaporation of 1 mole of substance; these values are also measured for almost all elements and most volatile compounds.
• Enthalpy of Dissolution $\left(\Delta \mathrm{H}_{\text {soln }}\right)$: The change in enthalpy that occurs when a certain amount of solute is dissolved in a given amount of solvent. When a certain amount of solute is dissolved in a certain amount of solvent, the enthalpy.

Specific Enthalpy

The specific heat enthalpy of the system is defined as the enthalpy per unit mass. It can be mathematically expressed as,

$$
\mathrm{h}=\mathrm{H} / \mathrm{m}
$$
Here,
$\mathrm{h}=$ specific enthalpy of the system
$H=$ enthalpy of the system

$$
\mathrm{m}=\text { Mass }
$$
Are Enthalpy and specific enthalpy the same or is there some difference between them?
Please note that it is the measure of the total heat content of the system, whereas, specific enthalpy is equivalent to the sum of specific Internal energy and the product of pressure and specific volume

$$
\mathrm{h}=\mathrm{u}+\mathrm{pv}
$$
Here,
$\mathrm{h}=$ specific enthalpy
$\mathrm{u}=$ specific Internal energy
$v=$ specific volume

$\mathrm{p}=$ pressure
S.I .unit: Joules per Kg

Dimensional Formula: $\left[M^0 L^2 T^{-2}\right]$

Entropy and Enthalpy

Entropy and enthalpy are regarded as fundamental concepts in thermodynamics, explaining fundamentally different energy properties in a system.

Enthalpy describes the total heat content of a system while keeping attention on the heat exchanged during chemical reactions or physical processes under constant pressure explains whether a process absorbs or releases energy as it does in endothermic and exothermic reactions.

Entropy, on the other hand, is a measure of a degree of disorder or randomness in a system. It, instead, reflects the innate tendency of systems to progress from relatively less random to more random-properly; for example from ice to water and then to the arrangement of random gases.