Faraday's Laws Of Electrolysis

Imagine charging your smartphone or the process of electroplating a piece of jewellery. These everyday activities are governed by the fundamental principles of Faraday's laws of electrolysis. Established by Michael Faraday, these laws explain how electric current can drive chemical reactions. They tell us how much substance is deposited or dissolved at the electrodes during electrolysis, directly impacting industries like electronics, metal plating, and even the production of chemicals. Faraday's laws not only enhance our understanding of these processes but also pave the way for innovations in energy storage and manufacturing. In this article, we will discuss the concept of Faraday's laws of electrolysis and its applications, current Efficiency and solved examples for better understanding.

JEE Main 2025: Physics Formula | Study Materials | High Scoring Topics | Preparation Guide

JEE Main 2025: Syllabus | Sample Papers | Mock Tests | PYQs | Study Plan 100 Days

NEET 2025: Syllabus | High Scoring Topics | PYQs

This Story also Contains

1. Faraday's Laws of Electrolysis
2. What is Current Efficiency?
3. Solved Examples Based on Faraday's laws of Electrolysis
4. Summary

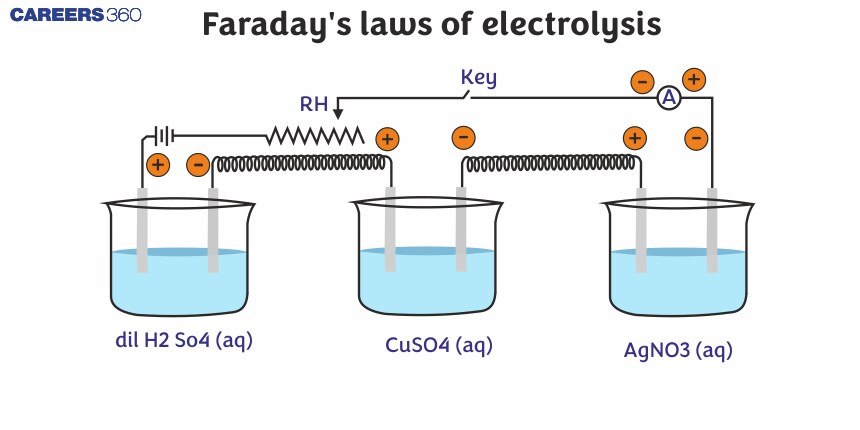
Faraday's Laws of Electrolysis

According to Faraday's first law, The amount of substance or quantity of chemical reaction at the electrode is directly proportional to the quantity of electricity passed into the cell.

$\begin{aligned} & \mathrm{W} \text { or } \mathrm{m} \propto \mathrm{q} \\ & \mathrm{W} \propto \mathrm{It} \\ & \mathrm{W}=\mathrm{ZIt} \\ & \mathrm{Z}=\frac{\mathrm{M}}{\mathrm{nf}} \\ & \mathrm{Z}=\frac{\mathrm{M}}{\mathrm{nf}} \\ & \mathrm{Z}=\text { Electrochemical equivalence } \\ & \mathrm{M}=\text { molarmass } \\ & \mathrm{F}=96500 \\ & \mathrm{n}=\text { Number of electrons transfer } \\ & \mathrm{q}=\text { amount of charge utilized }\end{aligned}$

The electrochemical equivalent is the amount of the substance deposited or liberated by one-ampere current passing for one second (that is, one coulomb, I x t = Q or one coulomb of charge.
One gram equivalent of any substance is liberated by one faraday.

$\begin{aligned} & \text { Eq. Wt. }=\mathrm{Z} \times 96500 \\ & \frac{\mathrm{W}}{\mathrm{E}}=\frac{\mathrm{q}}{96500} \\ & \mathrm{w}=\frac{\mathrm{E} . \mathrm{q}}{96500} \\ & \mathrm{~W}=\frac{\mathrm{Eit}}{96500}\end{aligned}$

As w = a x 1 x d that is, area x length x density
Here a = area of the object to be electroplated
d = density of metal to be deposited
l = thickness of layer deposited
Hence from here, we can predict charge, current strength time, thickness of deposited layer etc.

NOTE: One faraday is the quantity of charge carried by one mole of electrons.

$\begin{aligned} & \mathrm{E} \propto \mathrm{Z} \\ & \mathrm{E}=\mathrm{FZ} \\ & 1 \mathrm{~F}=1.6023 \times 10^{-19} \times 6.023 \times 10^{23} \\ & =96500 \text { Coulombs }\end{aligned}$

According to Faraday's second law, "When the same quantity of electricity is passed through different electrolytes, the amounts of the products obtained at the electrodes are directly proportional to their chemical equivalents or equivalent weights".

As $\frac{W}{E}=\frac{q}{96500}=$ No of equivalents constant
So
$
\frac{\mathrm{E}_1}{\mathrm{E}_2}=\frac{\mathrm{M}_1}{\mathrm{M}_2} \text { or } \frac{\mathrm{W}_1}{\mathrm{~W}_2}=\frac{\mathrm{Z}_1}{\mathrm{Z}_2 \mathrm{It}}=\frac{\mathrm{Z}_1}{\mathrm{Z}_2}
$
$\mathrm{E}_1=$ equivalent weight mass
$\mathrm{E}_2=$ equivalent weight mass
W or $\mathrm{M}=$ mass deposited

From this law, it is clear that 96500 coulomb of electricity gives one equivalent of any substance.

Application of Faraday's Laws

• It is used in the electroplating of metals.
• It is used in the extraction of several metals in pure form.
• It is used in the separation of metals from non-metals.
• It is used in the preparation of compounds

What is Current Efficiency?

It is the ratio of the mass of the products actually liberated at the electrode to the theoretical mass that could be obtained\text { C.E. }=\frac{\text { desired extent }}{\text { Theoretical extent of reaction }} \times 100 \%

Recommended Topic Video

Solved Examples Based on Faraday's laws of Electrolysis

Example 1: The negative Zn pole of a Daniell cell, sending a constant current through a circuit, decreases in mass by 0.13 g in 30 minutes. If the electrochemical equivalent of Zn and Cu are 32.5 and 31.5 respectively, the increase in the mass of the positive Cu pole in this time is

1) 0.180 g

2) 0.141 g

3) 0.126 g

4) 0.242 g

Solution:

Faraday's second law of electrolysis

$
\begin{aligned}
& m=z q \\
& \frac{m_1}{m_2}=\frac{z_1}{z_2}
\end{aligned}
$

According to Faraday's law of electrolysis
$
\frac{m_{z n}}{m_{c u}}=\frac{Z_{z n}}{Z_{c u}}
$
when i and t are the same
$
\begin{aligned}
& \therefore \frac{0.13}{m_{c u}}=\frac{32.5}{31.5} \\
& =m_{c u}=\frac{0.13 \times 31.5}{32.5} \\
& =0.126 \mathrm{~g}
\end{aligned}
$

Hence, the answer is option (3).

Example 2: The mass of a product liberated on an anode in an electrochemical cell depends on

1) $(I t)^{1 / 2}$ (Where $t$ is the time period for which the current is passed).
2) It ( Where $t$ is the time period for which the current is passed).
3) $I / t$ (Where t is the time period for which the current is passed).
4) $I^2 t$ (Where t is the time period for which the current is passed ).

Solution:

Faraday sec law of electrolysis

$\begin{aligned} & m=z q \\ & \frac{m_1}{m_2}=\frac{z_1}{z_2}\end{aligned}$

wherein

The electrochemical equivalent is the mass of ions deposited or liberated during electrolysis.

According to Faraday's law

$
m \propto \mathrm{It}
$

Hence, The correct answer is It.

Hence, The answer is the option (2).

Example 3: Two voltmeters, one of copper and another of silver, are joined in parallel. When a total charge q flows through the voltmeters, an equal amount of metals are deposited. If the electrochemical equivalents of copper and silver are $z_1$ and $z_2$ respectively the charge which flows through the silver voltameter is

1) $q \frac{z_1}{z_2}$
2) $q \frac{z_2}{z_1}$
3) $\frac{q}{1+\frac{z_1}{z_2}}$
4) $\frac{q}{1+\frac{z_2}{z_1}}$

Solution:

Faraday's second law of electrolysis

$\begin{aligned} & m=z q \\ & \frac{m_1}{m_2}=\frac{z_1}{z_2}\end{aligned}$

wherein

The electrochemical equivalent is the mass of ions deposited or liberated during electrolysis.

The voltmeters joined are parallel mass-deposited $z_1 q_1=z_2 q_2$

$\begin{aligned} & \frac{q_1}{q_2}=\frac{z_2}{z_1} \\ & =\frac{q_1+q_2}{q_2}=\frac{z_1+z_2}{z_1} \\ & \Rightarrow \frac{q}{q_2}=\left(1+\frac{z_2}{z^1}\right) \\ & q_2=\frac{q}{1+\frac{z_2}{z_1}}\end{aligned}$

Hence, The answer is the option (4).

Example 4: The negative Zn pole of a Daniell cell, sending a constant current through a circuit, decreases in mass by 0.13 g in 30 minutes. If the electrochemical equivalent of Zn and Cu are 32.5 and 31.5 respectively, the increase in the mass (in grams) of the positive Cu pole at this time is

1) 0.126

2) 0.141

3) 0.180

4) 0.242

Solution:

Faraday's second law of electrolysis

$\begin{aligned} & m=z q \\ & \frac{m_1}{m_2}=\frac{z_1}{z_2}\end{aligned}$

wherein

The electrochemical equivalent is the mass of ions deposited or liberated during electrolysis.

$
\frac{m_{Z n}}{m_{C u}}=\frac{Z_{Z n}}{Z_{C u}}
$

Where $i$ and $t$ are the same.
$
\begin{aligned}
& \frac{0.13}{m_{C u}}=\frac{32.5}{31.5} \\
& m_{C u}=0.126 \mathrm{~g}
\end{aligned}
$

Hence, the answer is the option (1).

Example 5: The electrochemical equivalent of a metal is 3.3 x 10^-7 kg per coulomb. The mass of the metal liberated at the cathode when a 3 A current is passed for 2 second will be

1) 19.8 x 10^-7 kg

2) 9.9 x 10^-7 kg

3) 6.6 x 10^-7 kg

4) 1.1 x 10^-7 kg

Solution:

Faraday's first law of electrolysis

The mass (m) of the substance deposited or liberated at any electrode is directly proportional to the quantity of electricity passed through the electrolyte

$\begin{aligned} m & =z q \\ m & =z i t \\ m & =\left(3.3 \times 10^{-7}\right) \times 3 \times 2 \\ m & =19.8 \times 10^{-7} \mathrm{~kg}\end{aligned}$

Hence, the answer is the option (1).

Summary

Faraday's laws of electrolysis provide a quantitative understanding of how electric current drives chemical reactions in electrolytic cells. They establish that the amount of substance deposited or dissolved at an electrode is directly proportional to the quantity of electricity passed through the electrolyte. These principles have crucial applications in industries such as electroplating, metal refining, and energy storage, offering insights that drive technological advancements and practical applications in everyday life.