Kinetic Friction - Definition, Types, Examples, FAQs

When we try to slide a body over a surface, the motion is opposed by a bonding between the body and the surface. This is caused by the electromagnetic forces between the charged particles at the surface and the body’s surface that is in contact. This resistance/opposition is represented by a force called friction. However our topic of discussion is ‘Kinetic friction’ here. As we go further into the article, we will learn about ‘what is kinetic friction/dynamic friction?’, ’how to define kinetic friction?’, ‘work done by friction’, ‘what is static friction and what are the differences between static and kinetic friction?’ and ‘what are the three types of kinetic friction?’ along with some friction images to understand each concept clearly.

JEE Main 2025: Physics Formula | Study Materials | High Scoring Topics | Preparation Guide

JEE Main 2025: Syllabus | Sample Papers | Mock Tests | PYQs | Study Plan 100 Days

NEET 2025: Syllabus | High Scoring Topics | PYQs

This Story also Contains

1. What is Kinetic Friction?
2. Coefficient of friction (μk)
3. What is Static Friction?
4. Differences Between Static and Kinetic/Dynamic Friction
5. Types of Kinetic Friction
6. Solved Examples Based On Kinetic Friction
7. Summary

Also check-

• NCERT Exemplar Class 11th Physics Solutions
• NCERT Exemplar Class 12th Physics Solutions
• NCERT Exemplar Solutions for All Subjects

What is Kinetic Friction?

Friction is of two types- static and kinetic friction which is also called dynamical friction. The type of friction that acts when a body slides over a surface is called kinetic friction denoted by fk (see figure-1). The two surfaces are moving relative to each other in this case. The magnitude of kinetic friction force increases usually when the normal force increases. That is the reason why it takes more force to slide a box full of books across the floor rather than to slide the same box when it is empty. This principle is also used in braking systems i.e. the harder the brake pads are squeezed against the rotating brake disks, the greater is the braking effect produced (see Figure 2).

(Figure-1)

(Figure-2)

How to Define Kinetic Friction?

We can define kinetic friction as- When two bodies in contact with each other move with respect to each other, rubbing the surfaces in contact, the friction between them is kinetic friction. Direction of kinetic friction is the opposite to the velocity of the body moving on the surface of another body (see figure-3).

(Figure-3)

The magnitude of kinetic friction depends on the normal force or the normal reaction acting between the two bodies. So we can write-

fKαNfK=μKN−−−−−−−(1)fK= the kinetic friction μK= coefficient of kinetic friction is constant N= reaction

Where N is the normal force/normal reaction and k is the proportionality constant used, which is called the coefficient of kinetic friction. The value of this coefficient of kinetic friction depends on the nature of the two surfaces in contact. The more slippery the surface is, the smaller is the coefficient of kinetic friction i.e. if the surfaces are smooth, the coefficient is small and if the surfaces are rough, the value of the coefficient is large. Since this coefficient of kinetic friction is a quotient of two forces, it is a pure number and hence has no units.

NOTE- By equation-1-

1) The coefficient of kinetic friction does not depend on the speed of the sliding bodies. However, this is true only for speeds that are not too large and are generally less than 10m/s.

2) The coefficient of kinetic friction does not depend on the area of the surface in contact as long as the normal force N is the same.

Kinetic friction examples include the friction that comes into play when any body moves along a surface. We will discuss examples of kinetic friction alongside its different types.

Coefficient of friction (μk)

• It is unitless and dimensionless.
• It depends on the nature and material of the two bodies in contact.
• It doesn't depend on the speed of the sliding bodies.

Work Done by Friction

As friction is force acting opposite to external force acting on the surface, this work done by a frictional force is nothing but dot product of force with the displacement the body goes through.

W=-F.ds (equation-2)

In the case of kinetic friction, we put the value of force calculated from equation-1 in the above equation and find the work done by the kinetic friction i.e.

W=-(kN).ds

Also Read:

• NCERT solutions for Class 11 Physics Chapter 5 Laws of Motion
• NCERT Exemplar Class 11 Physics Solutions Chapter 5 Laws of Motion
• NCERT notes Class 11 Physics Chapter 5 Laws of Motion

What is Static Friction?

Imagine we are trying to slide a heavy almirah on a horizontal concrete surface by applying a force F. This concrete surface is not an ideal frictionless surface. So, if this force F is small, the almirah remains stationary and the force that resists or counteracts F , not allowing it to move, is called static friction denoted by fs. If we increase the applied force, the almirah eventually starts moving. When the almirah is on the verge of slipping, the static force of friction is the maximum (limiting friction). At this point F becomes greater than fs which is the maximum static force the surface can exert as a result of which the almirah breaks loose from the bonds between molecules of it and the surface of the floor and it begins to slide.

See figure-3. (a), (b), (c) shows that when there is no relative motion, the magnitude of static friction fs is less than or equal to μsN, where s is the coefficient of static friction and N is the normal force. (d) shows that there is a relative motion when magnitude of kinetic friction fk is equal to kN.

(Figure-3)

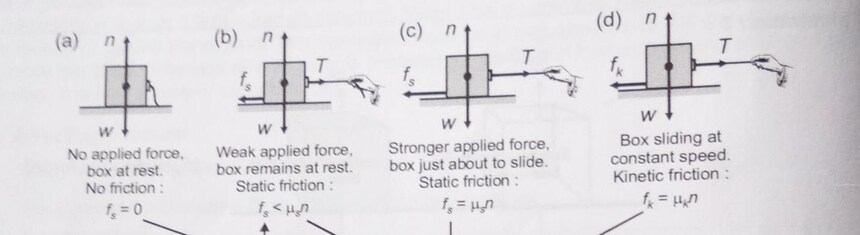
For a pair of given surfaces, the maximum value of fs depends on normal force and experiments show that this maximum value (fs)max is proportional to N and the proportionality constant is μs. In a given situation, the actual static friction can have any magnitude between zero and maximum given by μsN.

Thus, (fs)max= μsN => fs μsN (equation-3)

This maximum value of static friction, (fs)max is called limiting friction. Thus static friction can have any value between zero and its maximum or limiting value.

Differences Between Static and Kinetic/Dynamic Friction

The kinetic friction acts when there is a relative movement between surfaces of 2 bodies or a body and floor. However, static friction acts on a body which remains at rest.

(Figure-4)

2. Kinetic friction is always comparatively less than limiting friction, i.e., fk<fl. Hence, μk<μs.

3. The kinetic friction does not depend on the magnitude of applied force. However, static friction depends on the magnitude of force applied.

4. The value of static friction can be anything from 0 to limiting friction and thus it can be zero but kinetic friction cannot have a zero value.

5. Static friction opposes motion of a body when it’s at rest and then gets max when it starts to slide but kinetic friction is the opposition provided to an already moving body.

NCERT Physics Notes:

• NCERT Notes Class 11th Physics
• NCERT Notes Class 12th Physics
• NCERT Notes For All Subjects

Types of Kinetic Friction

There are three types of kinetic friction generally- i) sliding friction ii) rolling friction and iii) fluid friction. Let’s discuss them one by one-

1. Sliding friction- This is the type of kinetic friction which comes into play when an object slides over a surface. It is of course weaker than the static friction. Formula for sliding friction is the same as the kinetic friction where F is force of sliding kinetic friction and the constant of proportionality is the coefficient of sliding kinetic friction. Examples are- when a block slides up or down an inclined plane, rubbing of hands together, rubbing of a matchstick etc.

Rolling friction- A spherical body or a circular ring rolls over a horizontal surface without slipping and at every instant, the body touches the surface at just one point and this point does not move relative to the surface. The friction experienced by the rolling body is called rolling friction and it is much smaller than sliding friction and hence very less force is required to keep rolling a body even if it is heavy, as compared to sliding it through the floor. That’s why the invention of wheels is a major milestone in the history of mankind.

To further keep things smoother, lubricants are used in ball bearings of many machines which reduces kinetic friction to a great extent. Examples – a suitcase on wheels, cartwheels moving on the floor, a bicycle or any automobile with wheels, etc.

Fluid friction- Both the sliding and rolling friction come into play in the case of solids but in case of liquids and gases, fluid friction comes into play. At least one of the surfaces is that of a fluid. It is the friction between the layers of a fluid that moves on top of each other or relative to each other. This internal opposition is also called viscosity. However if a body flows on top of a liquid or inside a fluid (which can be gas or a liquid), the body also experiences fluid friction (see figure-5).

(Figure-5)

This fluid friction experienced by the body can be minimized by giving streamlined shape to the body. This is why air planes, boats, submarines are made streamlined. In fact, nature has given birds and fishes streamlined bodies, so that they can easily move through fluids. Some examples of fluid friction are-

Recommended Topic Video

Solved Examples Based On Kinetic Friction

Question 1. A block rolled on a rough surface with a velocity of 8m/s comes to rest after travelling 4m. Compute the coefficient of friction (g=10m/s²)

1) 0.8

2) 0.6

3) 0.4

4) 0.5

Solution

Given

The initial velocity of the block, u = 8 m/s,

Distance travelled before the block stops, s = 4 m

Let the coefficient of kinetic friction between the block and the surface be .

F.B.D of the block-

From F.B.D

N=mgfk=μkN⇒fk=μkmg
Assuming the acceleration of the block to be ' a ',

N=mgfk=μkN⇒fk=μkmgFnct=ma−fk=ma⇒a=−μkg
The block stops (v=0) after a travelling displacement of 4m.

Applying 3rd equation of motion-

v2=u2+2as0=u2−2μkgs⇒μk=u22gs=8×82×10×4=0.8

Question 2. The coefficient of static friction between two surfaces of the body depends

1) nature of surface

2) the shape of the surface in contact

3) area of contact

4) all of the above

Solution

Dependence of (µ)

* Depends on material

* Nature of surfaces in contact

wherein

* Does not depend upon the apparent area of contact.

Friction force depends on the material and the nature of the surface in contact.

Hence, the answer is the Option (4).

Question 3. A rocket is fired vertically from the earth with an acceleration of 2g, where g is the gravitational acceleration. On an inclined plane inside the rocket, making an angle θ with the horizontal, a point object of mass m is kept. The minimum coefficient of friction ^µ_min between the mass and the inclined surface such that the mass does not move is :

1) tanθ

2) 2 tanθ

3) 3 tanθ

4) tan2θ

Solution

Kinetic or Dynamic Friction

fKαRfK=μKRfK= kinetic friction μK= coefficient of kinetic friction R= reaction wherein fK<Fl∴μK<μsμK= depends on the nature of the surface in contact.

Since the rocket is moving vertically upwards with an acceleration 2g therefore the apparent acceleration experienced by the point object is g + 2g = 3g

Vertically downwards

Where N=3mgcos⁡θ3mgsin⁡θ=μ3mgcos⁡θμ=tan⁡θ

Hence, the correct option is 1.

Question 4. The upper half of an inclined plane with inclination ϕis perfectly smooth while the lower half is rough._A body starting from rest at the top will again come to rest at the bottom if the coefficient of friction for the lower half is given by

1) 2tan⁡ϕ
2) tan⁡ϕ
3) 2sin⁡ϕ
4) 2cos⁡ϕ

Solution

Kinetic or Dynamic Friction -

fK∝RfK=μKRfK= kinetic friction μK= coefficient of kinetic friction R= reaction - wherein fK<Fl∴μK<μsμK= depends on the nature of the surface in contact.

For the upper half smooth incline, a component of g down the incline =gsin⁡ϕ

∴v2=2(gsin⁡ϕ)l2

For the lower half rough incline, frictional retardation =μkgcos⁡ϕ

∴ Resultant acceleration =gsin⁡ϕ−μkgcos⁡ϕ∴0=v2+2(gsin⁡ϕ−μkgcos⁡ϕ)l2 or 0=2(gsin⁡ϕ)l2+2g(sin⁡ϕ−μkcos⁡ϕ)l2 or 0=sin⁡ϕ+sin⁡ϕ−μkcos⁡ϕ or μkcos⁡ϕ=2sin⁡ϕ or μk=2tan⁡ϕ

Hence, the correct option is 1.

Question 5. A smooth block is released at rest on a 45∘ incline and then slides a distance d. The time taken to slide is n time as much to slide on a rough incline than on a smooth incline. The coefficient of friction is:

1) μs=1−1n2
2) μs=1−1n2
3) μk=1−1n2
4) μk=1−1n2

Solution

Kinetic or Dynamic Friction

fKαRfK=μKRfK= kinetic friction μK=coefficient of kinetic friction R= reaction wherein fK<Fl∴μK<μsμK= depends on the nature of the surface in contact. Component of g down the plane =gsin⁡θ∴ For a smooth plane, d=12(gsin⁡θ)t2….......(i)

For rough planes,

Frictional retardation up the plane =μk(gcos⁡θ)

∴d=12(gsin⁡θ−μkgcos⁡θ)(nt)2∴12(gsin⁡θ)t2=12(gsin⁡θ−μkgcos⁡θ)n2t2 or sin⁡θ=n2(sin⁡θ−μkcos⁡θ) Putting θ=45∘ or sin⁡45∘=n2(sin⁡45∘−μkcos⁡45∘) or 12=n22(1−μk) or μk=1−1n2

Hence, the correct option is 3.

Summary

Kinetic friction, also known as dynamic friction, occurs when two surfaces slide against each other, opposing the relative motion. It depends on the nature of the surfaces and the normal force but is always less than static or limiting friction. Various types of kinetic friction include sliding, rolling, and fluid friction, each with distinct characteristics and applications.

• NCERT Solutions for Class 11 Physics
• NCERT Solutions for Class 12 Physics
• NCERT Solutions for All Subjects