Kirchhoff’s Law - Equation, Example, FAQs

Kirchoff's laws are the basic laws in the current network analysis. There are two Kirchoff's laws- Kirchoff's first law also called Kirchoff's current law and Kirchoff's second law which is also called Kirchoff's voltage law. These are applicable to DC as well as AC currents. In this article, we will discuss state Kirchhoff's current law and Kirchhoff's current law formula, state and explain Kirchhoff's voltage law and Kirchhoff's voltage law formula, advantages of Kirchhoff's law and limitations of Kirchhoff's law.

This Story also Contains

1. Overview Of Gustav Robert Kirchoff and His Circuital Laws
2. State and Explain Kirchhoff’s Law
3. State Kirchhoff’s Current Law (KCL LAW)
4. State and Explain Kirchhoff’s Voltage Law (KVL LAW)
5. Advantages of Kirchhoff’s laws
6. Limitations of Kirchhoff’s laws
7. Solved Example Based on Kirchhoff's Laws

Overview Of Gustav Robert Kirchoff and His Circuital Laws

Kirchhoff's circuit rules are two equalities that deal with the electric current and potential difference in the lumped element model of electrical circuits (often known as voltage).In 1845, a German scientist named Gustav Robert Kirchhoff was the first to describe them. This broadened Georg Ohm's work and came before James Clerk Maxwell's. Kirchhoff's rules, often known as Kirchhoff’s laws, are widely utilized in electrical engineering. These rules apply in both time and frequency domains and serve as the foundation for network analysis.
Kirchhoff’s current law (KCL LAW) and Kirchhoff’s voltage law (KVL LAW) were defined in 1845 after he pursued the notions of Ohm's law and Maxwell's law.
Kirchhoff’s current law, or KCL LAW, is based on the principle of electric charge conservation. The input current to a node must be equal to the node's output current, according to this rule.

State and Explain Kirchhoff’s Law

Gustav Kirchhoff, a German scientist, found the two sets of laws that would help us comprehend the notion of current and energy conservation in a particular electrical circuit in 1845. Kirchhoff’s laws of electrical circuits are the name given to these two rules. Kirchhoff's rules of electrical circuits are useful for assessing and determining the electrical resistance and impedance of any complex alternating current (AC) circuit. To state Kirchhoff’s law, we must also be familiar with the directions of current flow.

Kirchhoff’s laws describe how current flows in a circuit and how voltage varies around a loop.

• Kirchhoff's Current Law: - According to Kirchhoff's First Law, the total current entering a node (or junction) equals the total current leaving it. This principle is based on the conservation of electric charge.
• Kirchhoff's Voltage Law (KVL) states that in any closed loop of an electrical circuit, the algebraic sum of all the potential differences (voltages) across components is zero. This means that the total voltage provided by energy sources (like batteries or generators) is equal to the total voltage drop across the other circuit elements in the loop.

Kirchhoff’s current law or first law is applicable to both alternating current and direct current circuits. It is inapplicable to magnetic fields that change over time.

State Kirchhoff’s Current Law (KCL LAW)

Kirchhoff’s current law definition: - The current coming into a node (or a junction) must match the current flowing out of it, according to Kirchhoff's first law. This is due to charge conservation.

The conventional technique to explain Kirchhoff’s current law is to write Kirchhoff’s equation in which the sum of all currents entering the junction equals the sum of currents exiting the junction.

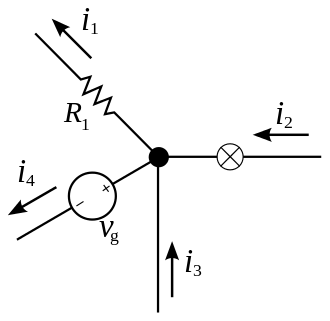
From the figure, the circuit consists of a junction with four branches. Currents $i_1, i_2, i_3$, and $i_4$ flow through these branches, involving a resistor $R_1$, a voltage source $V_g$, and other components. Kirchhoff's Current Law can be applied at the junction to relate the incoming and outgoing currents.

The current that enters a junction equals the current that leaves that junction.

$i_1+i_4=i_2+i_3$

This can be generalized to n wires joined together at a node, so Kirchhoff's current law formula is given as,

$$
\sum_{k=1}^n i_k=0
$$

State and Explain Kirchhoff’s Voltage Law (KVL LAW)

Kirchhoff’s voltage law definition: - The sum of all voltages across components that supply electrical energy (such as cells or generators) in any entire loop inside a circuit must match the sum of all voltages across all other components in the same loop, according to Kirchhoff’s voltage law (2nd Law). This law is the result of both charge and energy conservation.

When there are multiple junctions in a circuit, we must be careful to apply this law to only one loop at a time. In practice, this means selecting only one option at each crossroads. In the diagram given below, the circuit is a closed loop consisting of resistors $R_1, R_2, R_3$, and $R_5$ connected between nodes $a, b, c, d$, along with a voltage source $V_4$ between nodes $a$ and $d$. The currents flow through the resistors and are governed by the voltage drops $v_1, v_2, v_3$, and $v_5$ across each resistor, as determined by Kirchhoff's Voltage Law.

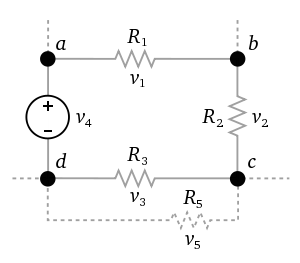
The total of all voltages around a loop equals zero.

$v_1+v_2+v_3+v_4=0$

Kirchhoff’s voltage law formula can be generalized.

$\sum_{k=1}^n V_k=0$

Advantages of Kirchhoff’s laws

Because of the numerous benefits of using Kirchhoff's rules, they are an important element of the fundamentals of circuit theory. For beginners, calculating unknown voltage and the current becomes much simpler. Numerous complex circuits are closed in a structure Kirchhoff’s current law, on the other hand, makes the analysis and calculation of these complex circuits straightforward and comprehensible where circuit analysis is typically difficult. There are numerous other benefits, but these are the most important.

Limitations of Kirchhoff’s laws

Both Kirchhoff’s laws contain a constraint in that they work under the premise that the closed loop has no fluctuating magnetic field. In the presence of a fluctuating magnetic field, electric fields, and emf can be induced, causing Kirchhoff’s loop rule to fail.

Other limitations are:

• Applicable to lumped circuits only
• High-frequency limitations
• Difficult for complex circuits

Recommended Topic Video

Solved Example Based on Kirchhoff's Laws

Example 1: Dimension of spectral emissive power is

1) $\left[M T^{-2}\right]$
2) $\left[M L^2 T^{-3}\right]$
3) $\left[M L^{-1} T^{-3}\right]$
4) $\left[M T^{-3}\right]$

Solution:

As we have learned,

Spectral Emissive Power -

$\begin{aligned}
& e_\lambda=\frac{\text { Energy }}{\text { Area } \times \text { times } \times \text { wavelength }} \\
& \text { unit }=\frac{J}{m^2 \cdot \text { s.A }} \\
& =\frac{\left[M L^2 T^{-2}\right]}{\left[L^2\right][T][L]}=\left[M L^{-1} T^{-3}\right]
\end{aligned}$

Hence, the answer is option (3).

Example 2: Which of the following relation is correct for total emissive power

1) $e=\int_0^{\infty} e_\lambda^2 d \lambda$
2) $e=\int_0^{\lambda_0} e_\lambda d \lambda$
3) $e=\int_0^{\infty} e_\lambda d \lambda$
4) $e=\int_0^{\lambda_0} e_\lambda^2 d \lambda$

Solution:

Total Emissive power is defined as the total amount of thermal energy emitted per unit time, per unit area of the body for all possible wavelengths.

$e=\int_0^{\infty} e_\lambda d \lambda$

Hence, the answer is option (3).

Example 3: For a perfectly black body emissivity is:

1) zero
2) 1
3) $\infty$
4) None of these

Solution:

The emissivity of a body at a given temperature is defined as the ratio of the total emissive power of the body (e) to the total emissive power of a perfectly black body.

$\varepsilon=\frac{e}{E}$

$\varepsilon=1 \text { - for a perfectly black body }$

Hence, the answer is option (2).

Example 4: The emissivity of a perfectly reflecting body is :

1) zero
2) 1
3) $\infty$
4) None of these

Solution:

As we known know,

$\text { Emissivity }=\varepsilon=\frac{e}{E}$

$\varepsilon=0 \text { - for perfectly reflecting body }$

Hence, the answer is option (1).

Also read:

• NCERT Exemplar Solutions for All Subjects
• NCERT Notes For All Subjects
• NCERT Solutions for All Subjects