What is thermal conductivity?

Thermal conductivity is a material property that measures how well heat can flow through it. It quantifies the rate at which heat is transferred through a material when there's a temperature difference across it. Materials with high thermal conductivity allow heat to pass through easily, while those with low thermal conductivity are good insulators.

How does the law of thermal conductivity relate to Fourier's law?

The law of thermal conductivity is essentially Fourier's law of heat conduction. Fourier's law states that the rate of heat transfer through a material is proportional to the negative temperature gradient and the area perpendicular to that gradient. The constant of proportionality in this relationship is the thermal conductivity.

Why do metals generally have higher thermal conductivity than non-metals?

Metals typically have higher thermal conductivity because of their free electrons. In metals, these electrons can move freely throughout the material, carrying both electrical charge and thermal energy. This allows for rapid heat transfer. Non-metals lack these free electrons, relying mainly on vibrations of atoms or molecules for heat transfer, which is less efficient.

How does temperature affect thermal conductivity?

Temperature can affect thermal conductivity, but the relationship varies depending on the material. For many pure metals, thermal conductivity decreases as temperature increases due to increased atomic vibrations interfering with electron movement. For some non-metals and alloys, thermal conductivity may increase with temperature as more energy carriers become available.

What's the difference between thermal conductivity and heat capacity?

Thermal conductivity measures how quickly heat flows through a material, while heat capacity measures how much thermal energy a material can store. A material with high thermal conductivity transfers heat rapidly but doesn't necessarily store much heat. Conversely, a material with high heat capacity can store a lot of thermal energy but may not transfer it quickly.

How is thermal conductivity measured experimentally?

Thermal conductivity can be measured using various methods, including:

How does the atomic/molecular structure of a material influence its thermal conductivity?

The atomic/molecular structure greatly influences thermal conductivity. In crystalline solids, the regular arrangement of atoms allows for efficient heat transfer through lattice vibrations (phonons). In amorphous materials, the irregular structure impedes heat flow. The presence of impurities, defects, or grain boundaries in a material can also reduce thermal conductivity by scattering heat carriers.

How does thermal conductivity affect heat insulation?

Materials with low thermal conductivity make good insulators because they slow down heat transfer. Insulation works by trapping small pockets of air or gas within a material, as gases generally have very low thermal conductivity. The effectiveness of insulation is often measured by its R-value, which is inversely related to thermal conductivity.

How do alloys typically compare to pure metals in terms of thermal conductivity?

Alloys generally have lower thermal conductivity than pure metals. This is because the introduction of different atoms into the crystal structure of a pure metal creates irregularities and impurities. These disrupt the orderly arrangement of atoms and interfere with the movement of electrons and phonons, the primary carriers of thermal energy in metals. The reduced mean free path of these carriers results in lower thermal conductivity.

How does anisotropy affect thermal conductivity in materials?

Anisotropy in materials means that properties vary depending on the direction. Some materials, particularly those with layered or fibrous structures, can have different thermal conductivities in different directions. For example, graphite has much higher thermal conductivity along its planes than perpendicular to them. This anisotropy is important in applications where heat flow direction is critical, such as in heat sinks or thermal management systems.

What's the difference between thermal conductivity and thermal diffusivity?

Thermal conductivity measures a material's ability to conduct heat, while thermal diffusivity measures how quickly a material can change its temperature when heat is applied. Thermal diffusivity is the ratio of thermal conductivity to the product of density and specific heat capacity. It indicates how quickly heat spreads through a material.

What is the role of phonons in thermal conductivity?

Phonons are quantized lattice vibrations in solids and play a crucial role in thermal conductivity, especially in non-metallic materials. They carry thermal energy through the material by propagating these vibrations. The thermal conductivity in many solids is determined by how easily these phonons can travel through the material's crystal structure. Factors that scatter phonons, like impurities or crystal defects, reduce thermal conductivity.

How does thermal conductivity change at very low temperatures?

At very low temperatures (approaching absolute zero), the behavior of thermal conductivity can be complex and material-dependent. In pure metals, thermal conductivity often increases as temperature decreases due to reduced electron-phonon scattering. However, in some materials, especially those where phonons dominate heat transfer, thermal conductivity can peak at a certain low temperature and then decrease as temperature approaches absolute zero due to reduced phonon excitations.

What is the Kapitza resistance and how does it relate to thermal conductivity?

Kapitza resistance, also known as thermal boundary resistance, is the resistance to heat flow that occurs at the interface between two materials. It's particularly noticeable at low temperatures or in nanoscale systems. While thermal conductivity describes heat flow within a material, Kapitza resistance describes the impedance to heat flow across material boundaries. This resistance can significantly affect overall heat transfer in composite materials or nanostructures.

What is the significance of the mean free path in thermal conductivity?

The mean free path is the average distance that a heat carrier (like an electron or phonon) travels before colliding with another particle or defect. It's crucial in determining thermal conductivity. A longer mean free path generally results in higher thermal conductivity because energy carriers can travel further without interruption. Factors that reduce the mean free path, such as impurities, grain boundaries, or increased temperature, typically decrease thermal conductivity.

What is the relationship between thermal conductivity and the speed of sound in a material?

There's a correlation between thermal conductivity and the speed of sound in a material, particularly in non-metallic solids. Both properties depend on how easily vibrations (phonons) can propagate through the material. Materials with strong interatomic bonds tend to have both high speed of sound and high thermal conductivity. This relationship is captured in the Debye model, which relates thermal conductivity to the speed of sound, specific heat capacity, and mean free path of phonons.

What is the role of lattice defects in thermal conductivity?

Lattice defects, such as vacancies, interstitials, or dislocations, generally reduce thermal conductivity in crystalline materials. These defects disrupt the regular crystal structure, scattering phonons and electrons that carry heat. The effect is particularly pronounced in materials where phonons are the primary heat carriers. However, in some cases, controlled introduction of defects can be used to engineer materials with desired thermal properties, such as thermoelectric materials where low thermal conductivity is desirable.

How does thermal conductivity change under extreme pressures?

Under extreme pressures, thermal conductivity generally increases. This is because pressure reduces the interatomic distances, strengthening the bonds between atoms. Stronger bonds allow for more efficient transmission of lattice vibrations (phonons), increasing phonon-mediated thermal conductivity. In metals, pressure can also increase electron density, enhancing electron-mediated thermal conductivity. However, the exact behavior can be complex and material-specific, sometimes showing non-linear changes or even decreases in certain pressure ranges.

What is the concept of phonon-glass electron-crystal (PGEC) in thermoelectric materials?

The phonon-glass electron-crystal (PGEC) concept is an ideal for thermoelectric materials. It describes materials that conduct electricity like a crystalline solid (high electrical conductivity) but conduct heat poorly like a glass (low thermal conductivity). This combination is desirable for efficient thermoelectric materials, which need to maintain a temperature gradient while allowing electrical current flow. PGEC materials often have complex crystal structures that scatter phonons effectively while allowing relatively unimpeded electron movement. This concept guides the search for high-performance thermoelectric materials for energy harvesting and cooling applications.

How does thermal conductivity affect the formation and propagation of thermal waves?

Thermal conductivity plays a crucial role in the formation and propagation of thermal waves. Thermal waves, also known as temperature waves or heat waves, are oscillations in temperature that can propagate through a material. Materials with high thermal conductivity tend to dampen these waves quickly, as heat is rapidly distributed. In contrast, materials with low thermal conductivity allow thermal waves to propagate further. The study of thermal waves is important in non-destructive testing, thermal wave microscopy, and understanding heat transfer

Can thermal conductivity be zero?

In practice, no material has zero thermal conductivity. Even the best insulators conduct some heat. However, in certain conditions, like in a perfect vacuum or at absolute zero temperature, heat transfer by conduction would theoretically cease. The closest to zero conductivity achieved is with aerogels or in carefully designed vacuum insulation panels.

Why do gases generally have lower thermal conductivity than liquids or solids?

Gases typically have lower thermal conductivity because their molecules are far apart and have weak interactions. Heat transfer in gases occurs mainly through collisions between molecules, which are less frequent due to the large spaces between them. In liquids and solids, molecules are closer together, allowing for more efficient energy transfer through molecular vibrations and, in the case of metals, electron movement.

How does porosity affect the thermal conductivity of a material?

Porosity generally decreases thermal conductivity. Pores in a material are often filled with air or another gas, which has low thermal conductivity. These pores interrupt the continuous solid phase, reducing the pathways for heat conduction. This principle is used in many insulating materials like foam insulation or aerogels, where high porosity leads to excellent insulating properties.

How does thermal conductivity change during phase transitions?

Thermal conductivity often changes dramatically during phase transitions. For example, when a solid melts into a liquid, thermal conductivity typically decreases due to the loss of the rigid crystal structure. The change can be even more pronounced when transitioning to or from a gas phase. Understanding these changes is crucial in applications involving heat transfer during melting, boiling, or condensation processes.

What is the concept of minimum thermal conductivity?

The concept of minimum thermal conductivity suggests that there's a lower limit to how low the thermal conductivity of a solid can be. This limit is based on the idea that heat transfer can't be slower than the propagation of individual atomic vibrations. The minimum thermal conductivity is often estimated using models that assume each atom vibrates independently. This concept is important in the search for ultra-low thermal conductivity materials for insulation applications.

How do nanostructures affect thermal conductivity?

Nanostructures can significantly alter thermal conductivity compared to bulk materials. At the nanoscale, surface effects become more prominent, and the mean free path of heat carriers can exceed the dimensions of the structure. This often leads to reduced thermal conductivity due to increased scattering at interfaces and boundaries. However, in some cases, carefully designed nanostructures can enhance thermal conductivity in specific directions, allowing for tailored thermal management solutions.

Law Of Thermal Conductivity

Physics
Properties of Solids and Liquids
Law Of Thermal Conductivity

Law Of Thermal Conductivity

Vishal kumarUpdated on 02 Jul 2025, 07:49 PM IST

Have you ever noticed how quickly a metal spoon is heated up when put in some hot soup, compared to a wooden one? This is caused by a property describing how well a given material conducts heat; it's called thermal conductivity. Understanding the law of thermal conductivity permits us to explain why different materials warm up at different rates and how we can make use of these properties in things like cooking, building homes, or electronic devices.

This Story also Contains

Law of Thermal Conductivity Law Of Thermal Conductivity
Solved Examples Based On Law Of Thermal Conductivity
Summary

In this article, we will cover the concept of the Law Of Thermal Conductivity. This concept falls under the broader category of Properties of Solids and Liquids which is a crucial chapter in Class 11 physics. It is an important topic, last few years many questions asked about this topic in exams like JEE and NEET.

Law of Thermal Conductivity Law Of Thermal Conductivity

Consider a rod of length ' T ', an area of cross-section 'A' whose faces are maintained at temperature $\theta_1$ and $\theta_2$ respectively. In a steady state, the amount of heat flowing from one face to the other face in time t is given by -

$
Q=\frac{K A\left(\theta_1-\theta_2\right) t}{l}
$

$Q=$ Amount of heat transfer
$t=$ Time of heat flow
$K=$ Thermal conductivity of the material
So, from the above equation we can calculate the - Rate of flow of heat i.e. heat current which can be written as -

$
\frac{Q}{t}=H=\frac{K A\left(\theta_1-\theta_2\right)}{l}
$
In the differential form, this heat current can also be written as -

$
\frac{d Q}{d t}=-K A \frac{d \theta}{d x}
$

In the case of a non-steady state or variable cross-section, this is the more general equation that can be used to solve problems.

Relation of Thermal Conductivity of Some Material

$\begin{aligned} & K_{A g}>K_{C u}>K_{A l} \\ & K_{\text {Sold }}>K_{\text {Liquid }}>K_{\text {Gas }} \\ & K_{\text {Metals }}>K_{\text {Non-metals }}\end{aligned}$

Thermal Resistance

$\left(\mathbf{R}_{\mathrm{th}}\right)$: The thermal resistance of a body is defined as the measure of its opposition to the flow of heat through it. It is defined as the ratio of temperature difference to the heat current $\left(\frac{Q}{t}\right)$.

$
R_{t h}=\frac{\theta_1-\theta_2}{H}=\frac{\theta_1-\theta_2}{K A\left(\theta_1-\theta_2\right) / l}=\frac{l}{K A}
$

Solved Examples Based On Law Of Thermal Conductivity

Example 1: A long metallic bar is carrying heat from one of its ends to the other end under steady-state. The variation of temperature $\theta$ along the length $x$ of the bar from its hot end is best described by which of the following figures?

Solution:

The heat Flow rate is given by
$
\begin{aligned}
& \frac{d \theta}{d t}=\frac{K A\left(\theta_1-\theta\right)}{x} \Rightarrow \theta_1-\theta=\frac{x}{K A} \cdot \frac{d \theta}{d t} \\
& \theta=\theta_1-\frac{x}{K A} \cdot\left(\frac{d \theta}{d t}\right) \\
& \text { or } \\
& \theta_1=\text { temperature of the hot end }
\end{aligned}
$

and
$\theta=$ temperature at distance x from hot end
$\theta \rightarrow x$ is a straight line with a -ve slope

Hence, the answer is the option (2).

Example 2: Two thin metallic spherical shells of radii $r_1$ and $r_2\left(r_1<r_2\right)$ are placed with their centres coinciding. A material of thermal conductivity K is filled in the space between the shells. The inner shell is maintained at temperature $\theta_1$ and the outer shell at temperature $\theta_2\left(\theta_1<\theta_2\right) \cdot$ The rate at which heat flows radially through the material is :

1) $\frac{\mathrm{K}\left(\theta_2-\theta_1\right)}{\mathrm{r}_2-\mathrm{r}_1}$
2) $\frac{\mathrm{K}\left(\theta_2-\theta_1\right)\left(\mathrm{r}_2-\mathrm{r}_1\right)}{4 \pi \mathrm{r}_1 \mathrm{r}_2}$
3) $\frac{4 \pi \mathrm{Kr}_1 \mathrm{r}_2\left(\theta_1-\theta_2\right)}{\mathrm{r}_2-\mathrm{r}_1}$
4) $\frac{\pi r_1 r_2\left(\theta_2-\theta_1\right)}{r_2-r_1}$

Solution:

$\begin{aligned} & H=\frac{K\left(4 \pi x^2\right)}{d x}(d \theta) \\ & \frac{H}{4 \pi K} \int_{r_1}^{r_2} \frac{d x}{x^2}=\int_{\theta_2}^{\theta_1} d \theta \\ & \frac{H}{4 \pi K} \times\left[-\frac{1}{x}\right]_{r_1}^{r_2}=[\theta]_{\theta_2}^{\theta_1} \\ & \frac{H}{4 \pi K}\left[\frac{-1}{r_2}+\frac{1}{r_1}\right]=\left(\theta_2-\theta_1\right) \\ & \frac{H\left(r_2-r_1\right)}{4 \pi K\left(r_1 r_2\right)}=\theta_1-\theta_2 \\ & H=\frac{4 \pi K\left(r_1 r_2\right)\left(\theta_1-\theta_2\right)}{\left(r_2-r_1\right)}\end{aligned}$

Hence, the answer is the option (3).

Example 3: Temperature difference of $120^{\circ} \mathbf{C}$ is maintained between two ends of a uniform rod $A B$ of length 2 L. Another bent rod $P Q$, of the same cross-section as $A B$ and length $\frac{3 L}{2}$, is connected across $A B$ (See figure). In steady state, the temperature $\left(\right.$ in $\left.{ }^0 \mathrm{C}\right)$ difference between P and Q will be close to :

1) 45

2) 75

3) 60

4) 35

Solution:

Heat Current
$
I_H=\frac{\theta_1-\theta_2}{R_{t h}}
$

where the Temperature difference between two ends of the conductor.
Thermal current =

$
\begin{aligned}
& \frac{\theta_A-\theta_B}{R_{e q}} \\
\text { Thermal current }= & \frac{\theta_A-\theta_B}{\frac{8 R}{5}}=\frac{5 \times 120}{8 \times R}=\frac{75}{R}
\end{aligned}
$

$\begin{aligned} & \theta_p-\theta_Q=\frac{75}{R} \cdot \frac{3 R}{5} \\ & =45^{\circ} \mathrm{C}\end{aligned}$

Hence, the answer is the option (1).

Example 4: Two plates $A$ and $B$ have thermal conductivities $\underline{84} \mathrm{Wm}^{-1} \mathrm{~K}^{-1}$ and $126 \mathrm{Wm}^{-1} \mathrm{~K}^{-1}$ respectively. They have the same surface area and same thickness. They are placed in contact along their surfaces. If the temperatures of the outer surfaces of A and B are kept at $100^{\circ} \mathrm{C}$ and $0^{\circ} \mathrm{C}$ respectively, then the temperature of the surface of contact in steady state is $\qquad$ ${ }^{\circ} \mathrm{C}$.

1) 40

2) 50

3) 20

4) 35

Solution:

$\begin{aligned} & \frac{K_A A\left(T_A-T\right)}{L}=\frac{K_{\mathrm{B}} A\left(T-T_B\right)}{L} \\ & 84(100-T)=126(T-0) \\ & T=40^{\circ} \mathrm{C}\end{aligned}$

Hence, the answer is the option (1).

Example 5: Three rods are arranged in series combination with their length , area and conductivity given in the figure , then their thermal resistance is

1) $\frac{15 l}{6 K A}$
2) $\frac{19 l}{6 K A}$
3) $\frac{23 l}{6 K A}$
4) $\frac{31 l}{6 K A}$

Solution:

In Series Combination

$R_{e q}=R_1+R_2+R_3-\cdots-\cdots-\cdots R_n$

wherein

R_eq= equivalent thermal resistance

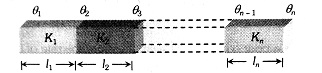
$\begin{aligned} & R=R_1+R_2+R_3 \\ = & \frac{l_1}{K_1 A_1}+\frac{l_2}{K_2 A_2}+\frac{l_3}{K_3 A_3}=\frac{l}{K A}+\frac{2 l}{K A / 2}+\frac{l}{6 K A} \\ & \frac{6 l+24 l+l}{6 K A}=\frac{31 l}{6 K A}\end{aligned}$

Hence, the answer is the option (4).

Summary

The law of thermal conductivity states that the rate of heat transfer through a material is directly proportional to the temperature difference across the material and the area through which the heat is transferred, inversely proportional to the thickness of the material. This law explains the efficiency of heat movement through varied materials. High thermal conductivity will mean quick heat transfers; low thermal conductivity implies slow heat transfers. This principle has generated interest in the design of insulation and heat management in many applications.

Frequently Asked Questions (FAQs)

Q: How do amorphous materials differ from crystalline materials in terms of thermal conductivity?

Amorphous materials generally have lower thermal conductivity than their crystalline counterparts. In crystals, the regular, periodic structure allows for efficient phonon propagation. Amorphous materials lack this long-range order, leading to increased phonon scattering. This disorder limits the mean free path of phonons, reducing thermal conductivity. However, the lack of grain boundaries in amorphous materials can sometimes lead to higher conductivity than polycrystalline samples at very low temperatures. Understanding these differences is crucial in applications ranging from glass manufacturing to semiconductor device design.

Q: What is the significance of the Debye temperature in thermal conductivity?

The Debye temperature is a characteristic temperature of a solid related to its maximum phonon frequency. It's significant in thermal conductivity because it relates to the material's atomic bonding strength and vibrational properties. Materials with higher Debye temperatures typically have stronger bonds and higher phonon frequencies, often correlating with higher thermal conductivities. The Debye temperature also marks a transition in behavior: below it, quantum effects become important in phonon behavior, affecting how thermal conductivity changes with temperature.

Q: What is the role of interface thermal resistance in composite materials?

Interface thermal resistance, also known as thermal boundary resistance or Kapitza resistance, occurs at the boundary between different materials in a composite. It represents an additional barrier to heat flow beyond the bulk thermal resistance of the materials. This resistance arises from differences in phonon spectra and atomic structure at the interface, causing phonon scattering. In composites, especially those with many interfaces like nanocomposites, this resistance can significantly affect overall thermal conductivity, often reducing it compared to the constituent materials.

Q: How does the concept of phonon mean free path relate to the size effect in nanoscale thermal conductivity?

The phonon mean free path is the average distance a phonon travels before scattering. In bulk materials, this path is typically much shorter than the material's dimensions. However, in nanostructures, the material's size can become comparable to or smaller than the mean free path. This leads to the "size effect," where thermal conductivity decreases as the material size approaches the mean free path. This effect is crucial in nanotechnology, allowing for the engineering of materials with tailored thermal properties by controlling their nanoscale structure.

Q: What is the relationship between thermal conductivity and specific heat in determining how quickly a material heats up or cools down?

The rate at which a material heats up or cools down depends on both its thermal conductivity and specific heat, combined in a property called thermal diffusivity. Thermal diffusivity is the ratio of thermal conductivity to the product of density and specific heat. Materials with high thermal conductivity but low specific heat (like metals) tend to heat up and cool down quickly. Conversely, materials with low thermal conductivity but high specific heat (like water) change temperature more slowly. Understanding this relationship is crucial in thermal management and material selection for various applications.

Q: How does thermal conductivity affect the formation of thermal gradients in materials?

Thermal conductivity directly influences how thermal gradients form in materials. Materials with high thermal conductivity tend to have smaller thermal gradients because they quickly distribute heat, equalizing temperatures. Conversely, materials with low thermal conductivity can maintain larger thermal gradients as heat flows more slowly through them. This property is crucial in applications like thermal insulation, where maintaining temperature differences is desirable, or in heat sinks, where rapid heat distribution is needed.

Q: What is the difference between steady-state and transient thermal conductivity?

Steady-state thermal conductivity refers to heat conduction under constant temperature conditions, where the rate of heat flow doesn't change with time. Transient thermal conductivity, on the other hand, deals with situations where temperatures are changing with time. While steady-state measurements are simpler and more common, transient methods are crucial for understanding heat flow in dynamic situations or for materials where steady-state conditions are hard to achieve. Both concepts are important in different applications of heat transfer.

Q: How does thermal conductivity affect the efficiency of heat engines?

Thermal conductivity plays a crucial role in heat engine efficiency. In ideal heat engines, you want good thermal conductivity in heat exchangers to efficiently transfer heat into and out of the working fluid. However, you also want low thermal conductivity in insulating components to minimize unwanted heat loss. The balance between these needs affects the overall efficiency of the engine. Understanding and optimizing thermal conductivity in various components is key to improving heat engine performance.

Q: What is the Wiedemann-Franz law and what are its limitations?

The Wiedemann-Franz law states that the ratio of thermal conductivity to electrical conductivity in metals is proportional to temperature. This relationship arises because free electrons in metals are responsible for both electrical and thermal conduction. However, the law has limitations:

Q: How does thermal conductivity relate to the concept of thermal resistance?

Thermal conductivity is inversely related to thermal resistance. While thermal conductivity is a material property, thermal resistance is a measure of how much a specific object resists heat flow, taking into account both the material's conductivity and its geometry. The thermal resistance of a layer is calculated as the thickness divided by the product of thermal conductivity and area. Understanding this relationship is crucial in heat transfer calculations and thermal management design.

Articles

Gujarat Paramedical Merit List 2025 Revised (Out): Download PDF Rank List

Sep 04, 2025

How Online Degree Exams are Conducted in India?

Sep 01, 2025

BiPC Courses After 12th - Fees, Eligibility, Duration & Top Institutes

Aug 25, 2025

UPTAC Cutoff 2025 Round 3 (Out) - AKTU Opening and Closing Rank

Aug 23, 2025