Mu Naught Value - Definition, Unit, Types, FAQs

Magnetic permeability is a fundamental property of materials that describes how easily they get magnetized when subjected to a magnetic field. To describe the magnetic properties of a given substance, we require some quantities which include a quantity called magnetic permeability denoted as $\mu$ which is pronounced as the mew symbol or mu or meu sign. Hence in magnetism, mu means magnetic permeability. It quantifies the ability of a material to support the formation of the magnetic field within itself. In this article, we will come across three types of magnetic permeability. Also, we will learn about topics like basic principles, mathematical descriptions, and factors affecting magnetic permeability in this article.

JEE Main 2025: Physics Formula | Study Materials | High Scoring Topics | Preparation Guide

JEE Main 2025: Syllabus | Sample Papers | Mock Tests | PYQs | Study Plan 100 Days

NEET 2025: Syllabus | High Scoring Topics | PYQs

This Story also Contains

1. Magnetic Permeability Definition
2. Relation Between μ, B And H
3. Unit and Dimensions of Magnetic Permeability
4. Dimensional Formula For Magnetic Permeability In Free Space
5. Types of Magnetic Permeability
6. Value of μ (Relative Permeability) for Different Types of Materials
7. Relationship Between Magnetic Permeability μr and Magnetic Susceptibility (χm)
8. Factors Affecting Magnetic Permeability
9. Summary

Magnetic Permeability Definition

Magnetic permeability ($\mu$) is the ability to measure the degree of penetration of the magnetic field through the medium, It also measures the capacity of the substance to take magnetism. However, to define magnetic permeability, we must know about magnetic field strength/ magnetic induction denoted by ‘B’, and magnetic field intensity denoted by ‘H’.

• Magnetic intensity (H) is the degree to which a magnetic field can magnetize a material. S.I. unit of H is Ampere/meter (A/m)
• It can also be measured in oersted. Note: 1 A/m is equal to 0.012566 Oersted.
• Magnetic induction (B) also called magnetic flux density is the force experienced by a unit positive charge moving with a velocity perpendicular to the magnetic field. This definition for B has come from the formula of Lorentz force
• S.I. unit of B is Tesla or Weber/m² and C.G.S. unit is Gauss (G) where 1G=10^-4T.

Also, check-

• NCERT Exemplar Class 11th Physics Solutions
• NCERT Exemplar Class 12th Physics Solutions
• NCERT Exemplar Solutions for All Subjects

Relation Between $\mu$, B And H

We know that $\mathrm{B}\propto \mathrm{H}$

• $\mathrm{B}$=$\mu$$\mathrm{H}$, where is $\mu$ the constant of proportionality and stands for magnetic permeability.

Thus, we can define magnetic permeability as the ratio of magnetic field induction (B) to the magnetic intensity (H).

$$\mu =\frac{\text{Total magnetic field inside the material}}{\text{Magnetising field}}$$

$$\mu =\frac{B}{H}$$

where,

$\mu$ is the permeability

$\mathrm{B}$ is the magnetic flux density

$\mathrm{H}$ is the magnetic field strength

Unit and Dimensions of Magnetic Permeability

The S.I. unit of $\mu$

1. Unit for magnetic permeability is $\mathrm{Wb}{\mathrm{A}}^{-1}{\mathrm{m}}^{-1}$ .

• Given

$\mathrm{B}$ $=\mathrm{Wb}/{\mathrm{m}}^2$

$\mathrm{H}$ $=\mathrm{A}/\mathrm{m}$

The unit of magnetic permeability is

$\mu =\frac{B}{H}$

Unit of $\mu$ $=\frac{\mathrm{Wb}/{\mathrm{m}}^2}{\mathrm{A}/\mathrm{m}}$

simplifying

Unit of $\mu$ $=\frac{\mathrm{Wb}}{\text{A m}}$

2. Another unit is Henry per meter (${\mathrm{Hm}}^{-1}$)

$\mathrm{B}$ $=T$

$\mathrm{H}$ $=\mathrm{A}/\mathrm{m}$

Now, the unit of $\mu$ $=\frac{\mathrm{T}}{\mathrm{A}/\mathrm{m}}$

$=\frac{(\mathrm{kg}\cdot \mathrm{m}/({\mathrm{s}}^2\cdot \mathrm{A})}{\mathrm{A}/\mathrm{m}}$

The unit of $\mu$ $=\mathrm{H}/\mathrm{m}$

3. Another unit of $\mu$ is Newton per ampere square (NA^-2) or Telsa meter per ampere(TmA^-1).

$\mathrm{B}$ $=T$

$\mathrm{H}$ $=\mathrm{A}/\mathrm{m}$

Now the unit of $\mu$ $=\mathrm{T}\cdot \mathrm{m}/\mathrm{A}$

OR

$1\mathrm{T}=\frac{1\mathrm{N}\cdot \mathrm{m}}{\mathrm{A}\cdot {\mathrm{m}}^2}=\frac{1\mathrm{N}}{\mathrm{A}\cdot \mathrm{m}}$

Unit of $\mu =\frac{\mathrm{T}}{\mathrm{A}/\mathrm{m}}$

Unit of $\mu =\frac{\frac{1\mathrm{N}}{\mathrm{Am}}}{\mathrm{A}/\mathrm{m}}$

Unit of $\mu =\frac{1\mathrm{N}}{{\mathrm{A}}^2}$

Dimensional Formula For Magnetic Permeability In Free Space

The magnetic permeability in vacuum Charges Magnetism, or free space , ${\mu }_0=4\pi \times {10}^{-7}\mathrm{H}/\mathrm{m}$

Dimensional formula,

$1\mathrm{H}=\frac{1\mathrm{kg}\cdot {\mathrm{m}}^2}{{\mathrm{s}}^2\cdot {\mathrm{A}}^2}$

We know that,

${\mu }_0=\frac{1\mathrm{H}}{\mathrm{m}}=\frac{\frac{1\mathrm{kg}\cdot {\mathrm{m}}^2}{{\mathrm{s}}^2\cdot {\mathrm{A}}^2}}{\mathrm{m}}=\frac{\mathrm{kg}\cdot \mathrm{m}}{{\mathrm{s}}^2\cdot {\mathrm{A}}^2}$

The dimensional formula of magnetic permeability of free space,

$${\mu }_0=\mathrm{M}\cdot \mathrm{L}\cdot {\mathrm{T}}^{-2}\cdot {\mathrm{I}}^{-2}$$

Also read :

• NCERT notes Class 12 Physics Chapter 4 Moving ChargesMagnetism and magnetism
• NCERT solutions for Class 12 Physics Chapter 4 Moving charges and magnetism
• NCERT Exemplar Class 12 Physics Solutions Chapter 4 Moving charges and magnetism

Types of Magnetic Permeability

We will come across three types of magnetic permeability i.e. magnetic permeability in vacuum (μ₀)or permeability of free space, the magnetic permeability of medium (μ) and relative magnetic permeability (μ_r).

1. Magnetic permeability of free space (mu not)- It is the ratio of magnetic induction in free space to magnetic intensity,

i.e. ${\mathrm{B}}_0={\mu }_0\mathrm{H}\Rightarrow >{\mu }_0={\mathrm{B}}_0/\mathrm{H}$

The value of ${\mu }_0$ is $4\pi \times {10}^{-7}{\mathrm{WbA}}^{-1}{\mathrm{m}}^{-1}$

2. Permeability of medium (μ)- It is the ratio of magnetic field induction in the medium to the magnetic intensity.

$$\mu =B/H$$

2. Relative permeability (r)- It is defined as the ratio of the magnetic permeability of a medium ($\mu$) to the magnetic permeability of free space or vacuum.

$${\mu }_{\mathrm{r}}=\mu /{\mu }_0$$

Relative permeability of a material can also be defined as the ratio of the number of magnetic field lines per unit area (flux density i.e. B) in that material to the number of magnetic field lines per unit area that would be present if the material is replaced by vacuum ( flux density in a vacuum i.e. B₀).

As both the quantities involved in the above equations have the same unit, therefore relative permeability has no units and is a dimensionless quantity. It is just a number.

Value of $\mu$ (Relative Permeability) for Different Types of Materials

Based onbased on magnetic property, Faraday classified materials into three categories. These are-

1. Diamagnetic materials

2. Paramagnetic materials

3. Ferromagnetic materials

Let us briefly understand each of these on the basis of their mu values.

1. Diamagnetic materials-

• These materials have atoms that don’t possess their magnetic moment.
• When these materials are placed in an eternal applied field, they get slightly magnetized, and that too in the opposite direction to the applied magnetic field.
• Examples are- diamond, bismuth, gold, silver, copper, water, mercury, alcohol, nitrogen, hydrogen including all inert gases, etc.

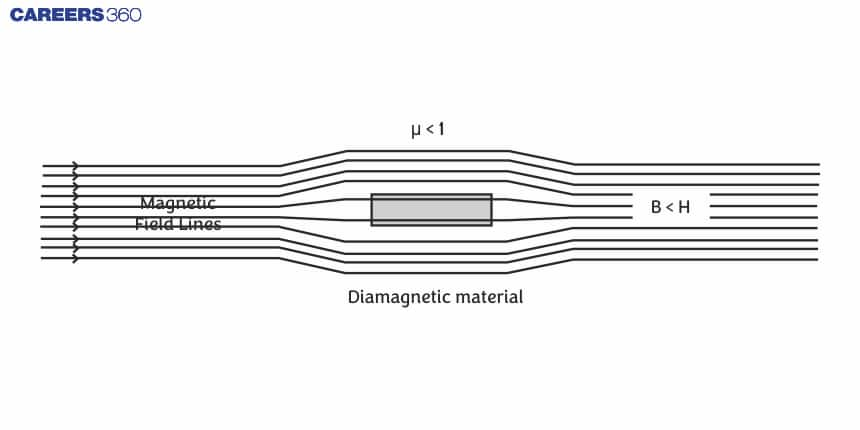
• When we place a diamagnetic specimen th in a magnetizing field, the field lines do not prefer to pass through the specimen material.

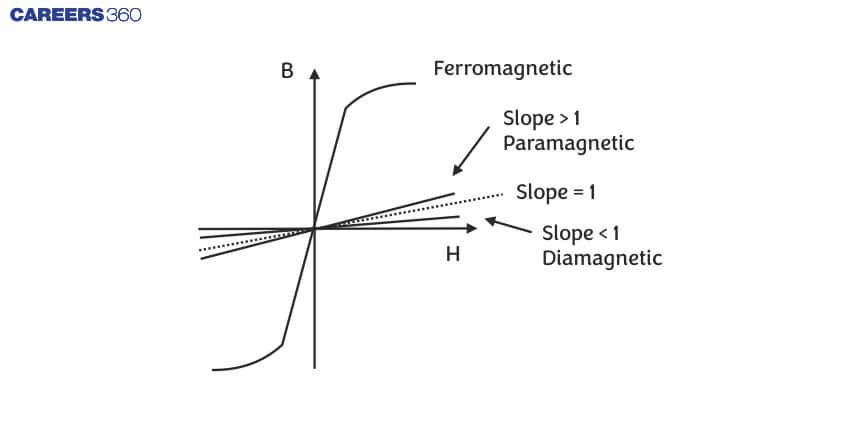
Hence, ${\mu }_r$ =-ve and it is less than 1.

This is because ->

$\begin{array}{rl}& B<B_0\\ & B/B_0<1\end{array}$

$\mu$<1 ($\mu$ value (relative) for diamagnets is always less than 1)

2. Paramagnetic materials-

• These materials have non-zero net magnetic moments of their own.
• When they are placed in an external applied magnetic field, they get magnetized in the same direction as the applied magnetic field.
• In absence of an external magnetic field, paramagnets do not behave like magnets. The reason behind this is that their net magnetic moment per unit volume becomes equal to zero because of their random arrangement of their own magnetic moments of atoms.
• Examples are- Tungsten, oxygen, sodium, aluminium, chromium, manganese, lithium, magnesium, potassium, platinum, etc.

• In the case of paramagnetic materials, the magnetic field lines prefer to pass through the specimen rather than through the air.

Thus, $\begin{array}{rl}& B>B_0\\ & B/B_0>1\end{array}$

${\mu }_r$>1 ( ${\mu }_r$ value for paramagnetic are always greater than 1).

3. Ferromagnetic materials

• These materials have their own permanent magnetic moment like that of paramagnetic materials.
• When they are placed in an external magnetic field, they get strongly magnetized in the direction of the applied external magnetic field. Hence they start behaving as magnets even if the external magnetic field is removed or not present.
• Examples are- iron, cobalt, nickel, gadolinium and other alloys

• Ferromagnetic materials show all the properties of paramagnetic materials but in a better way and to a much greater degree.

Thus, the ${\mu }_r$ value is very high in these materials i.e. ${\mu }_r$=10³ to 10⁵.

Note: there is a B-H graph for all magnetic materials discussed above, the slope of which gives us the curve’s incremental permeability. Hysteresis loops are also plotted in the B-H graph for ferromagnetic materials. The B-H graph for all three magnetic materials is given below.

NCERT Physics Notes:

• NCERT Notes Class 11th Physics
• NCERT Notes Class 12th Physics
• NCERT Notes For All Subjects

Relationship Between Magnetic Permeability ${\mu }_r$ and Magnetic Susceptibility (${\chi }_m$)

• Magnetic susceptibility is a property of a substance that tells us how easily a material can be magnetized when it is placed in an external magnetic field. It is denoted by m (pronounced as kappa).
• It is defined as the ratio of the intensity of magnetization to magnetic intensity

$${\chi }_m=\frac{I}{H}$$

• It has no units as both I and H have the same units.

Now, when a magnetic material is placed in an external magnetic field of magnetic intensity H, it gets magnetized.

Then, total magnetic field induction is-

$B=B_0+B_m$

Therefore, $B={\mu }_0(H+I)$ ------(1)

We know that, $\mathrm{I}={\chi }_{\mathrm{m}}\mathrm{H}$.

Therefore putting this value in equation (1)

$B={\mu }_{0}H({\chi }_m+1)$

But, $B=\mu H$

$\Rightarrow \mu \mathrm{H}={\mu }_0\mathrm{H}({\chi }_{\mathrm{m}}+1)$

$=>\mu /{\mu }_0={\chi }_m+1$

$${\mu }_{\mathrm{r}}={\chi }_{\mathrm{m}}+1$$

This is the relationship between ${\mu }_r$ and ${\chi }_m$.

Also read -

• NCERT Solutions for Class 11 Physics
• NCERT Solutions for Class 12 Physics
• NCERT Solutions for All Subjects

Factors Affecting Magnetic Permeability

• Temperature
• Magnetic Field Strength
• Material Composition
• Position in the medium
• Frequency of the Magnetic Field
• Humidity

Applications Of Magnetic Permeability In Technology

• Used in Transformers and Inductors: Materials with high magnetic permeability are used in the cores of transformers and inductors to improve their efficiency.
• Electric Motors and Generators: The materials with high relative magnetic permeability increase magnetic field interaction and energy conversion. Thus they can be used in the cores of motors and generators.
• Sensors and Instruments: Magnetic field sensors, fluxgate magnetometers, and MRI machines use materials, with specific magnetic permeability for better efficiency

Summary

Magnetic permeability is the ability of a material to allow magnetic lines to pass through it. Relative magnetic permeability (${\mu }_r$) quantifies how a material influences the magnetic field compared to the vacuum. It is used in devices such as electric motors, generators, transformers, etc.. to provide better efficiency. High ${\mu }_r$ materials are used for magnetic shielding, reducing interference in sensitive electronics and magnetic recording media. Understanding and utilizing relative permeability is essential for technological applications.