Relation Between G and g

Edited By Vishal kumar | Updated on Jul 02, 2025 04:34 PM IST

The relation between g (acceleration due to gravity) and G (universal gravitational constant) forms a fundamental concept in gravitational physics. G is a constant that defines the strength of the gravitational force between two masses in the universe, while g represents the acceleration experienced by an object due to Earth's gravitational pull. The relation between the two can be expressed mathematically using Newton's law of gravitation, which helps in understanding how the mass and radius of a celestial body, like Earth, influence the gravitational acceleration experienced at its surface.

Let's read this article to learn about the difference between capital g and small g, and the value of G on different surfaces. This is one of the very important concept for Class 12 Board exams and competitive exams like JEE, NEET and other state engineering exams.

This Story also Contains

Acceleration due to gravity (g) on earth
Universal Gravitational Constant (G)
Relation Between Acceleration Due to Gravity (g) and Gravitational Constant (G)
Variation in the Value of Acceleration Due to Gravity (g)

Relation Between G and g

Acceleration due to gravity (g) on earth

An object which falls under the sole influence of our gravity is called a free-falling object or acceleration due to gravity.
A free-falling object usually has an acceleration of about 9.8 m s−2, downward towards the earth. We usually denote it with the symbol g.

Acceleration due to gravity g on earth formula:

$g=9.8 \mathrm{~m} / \mathrm{s}^2$

Acceleration due to gravity dimensional formula =

$M^0 L^1 T^{-2}$

Also read -

NEET Highest Scoring Chapters & Topics

This ebook serves as a valuable study guide for NEET exams, specifically designed to assist students in light of recent changes and the removal of certain topics from the NEET exam.

Download E-book

Universal Gravitational Constant (G)

The force of attraction which is given between two unit masses that are separated by some unit distance is called the universal gravitational constant. This universal gravitational constant is often denoted by the symbol G (capital G) and is measured in Nm2/kg2.

Value of gravitational constant G $\begin{aligned} & G=6.67 \times 10^{-11} \mathrm{~N} \cdot \mathrm{~m}^2 / \mathrm{kg}^2 \\ & G=6.67 \times 10^{-8} \mathrm{dyne} \cdot \mathrm{cm}^2 / \mathrm{g}^2 \\ & G=6.67 \times 10^{-11} \mathrm{~N} \cdot \mathrm{~m}^2 \mathrm{~kg}^{-2}\end{aligned}$

The dimensional formula for G:

$[G]=M^{-1} L^3 T^{-2}$

Related Topics,

Property	g (Acceleration due to gravity)	G (Gravitational constant)
Definition	The acceleration is experienced by an object due to Earth's gravity.	Universal constant in the law of gravitation.
Value	∼9.8 m/s2	6.674×10−11 N m2/kg2
Unit	meters per second squared (m/s2)	Newton meters squared per kilogram squared (Nm2/kg2)
Formula used In	Used in F=mg, where F is the gravitational force on an object near Earth.	Used in F=Gm1m2r2, where F is the gravitational force between two masses.
Depends On	Earth's gravitational field.	Always constant, independent of location.

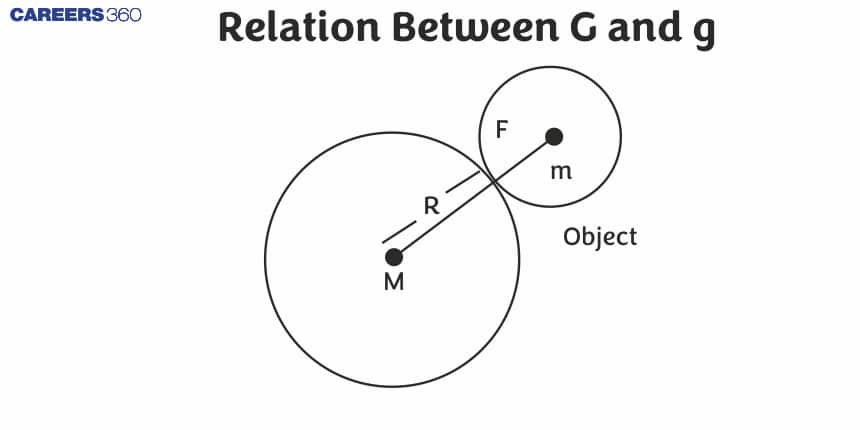
Relation Between Acceleration Due to Gravity (g) and Gravitational Constant (G)

After knowing about the difference between capital g and small g, move to the main part of the article which is the relation between capital g (G) and small g (g).

Let's suppose the mass of the earth is M and its radius is R, then the force of attraction on the given body is equal to the surface of the earth is given by

$$
F=\frac{G M m}{R^2}
$$

Where:

$F$ : Force of attraction (N)
$G$ : Universal gravitational constant $\left(6.67 \times 10^{-11} \mathrm{~N} \cdot \mathrm{~m}^2 / \mathrm{kg}^2\right)$
$M$ : Mass of Earth $\left(6 \times 10^{24} \mathrm{~kg}\right)$
$R$ : Radius of Earth $\left(6.4 \times 10^6 \mathrm{~m}\right)$
$m$: Mass of the object (kg)

Now, from the second law of motion

$
g=\frac{F}{m}
$
Substituting $F=\frac{G M m}{R^2}$ :

$
g=\frac{G M}{R^2}
$ .................(1)

We can see the above equation is free from the mass(m), which means we can say acceleration due to gravity does not depend on the mass. Hence it is equal for all.

Now, to get the value of g we need to put the value of the known parameter in equation (1).

$\begin{gathered}G=6.67 \times 10^{-11} \mathrm{~N} \cdot \mathrm{~m}^2 / \mathrm{kg}^2, M=6 \times 10^{24} \mathrm{~kg}, R=6.4 \times 10^6 \mathrm{~m} \\ g=\frac{\left(6.67 \times 10^{-11}\right)\left(6 \times 10^{24}\right)}{\left(6.4 \times 10^6\right)^2}\end{gathered}$

$\begin{gathered}g=\frac{4.002 \times 10^{14}}{4.096 \times 10^{13}} \\ g \approx 9.8 \mathrm{~m} / \mathrm{s}^2\end{gathered}$

Variation in the Value of Acceleration Due to Gravity (g)

In the above section we have seen the relation between g and G, Now let's move to a little depth of how the g value is changing in different cases.

It may vary due to the following factors

(i) Height above the surface of the earth

(ii) Depth below the surface of the earth

(iii) Shape of the earth

Let's read one by one starting from height above the surface of the earth.

(i) Height above the surface of the earth

Suppose a body of mass m is lying on the surface of the earth and its radius is R. Then its acceleration due to gravity at the earth's surface,

$g=\frac{G M}{R^2}$

Acceleration due to gravity

Height h above the surface, the force of gravity,

$F=\frac{G M m}{(R+h)^2}$

Therefore, the acceleration due to gravity at height h above the surface

$g^{\prime}=\frac{G M}{(R+h)^2}$

Or,

$\begin{gathered}g^{\prime}=\frac{G M}{R^2} \cdot \frac{1}{\left(1+\frac{h}{R}\right)^2} \\ g^{\prime}=g \cdot \frac{1}{\left(1+\frac{h}{R}\right)^2}\end{gathered}$

Thus,

$g^{\prime}<g$

(ii) Depth below the surface of the earth

Now for this situation, let's suppose an object of mass m is kept at a depth of d below the earth's surface. Its distance from the center of the Earth is (R − d). This mass is located on the outer surface of the Inner solid sphere. The gravitational pull on a mass within a spherical shell is always zero. Consequently, the Objects experience gravitational attraction mainly because the Inner solid sphere

Gravitaion due to gravity

Mass of this sphere

$M^{\prime}=M \cdot \frac{(R-d)^3}{R^3}$

Here, M represents the mass of the entire sphere of radius R.

Now, the force of gravity on an object at a depth d

$$
F=\frac{G M^{\prime} m}{(R-d)^2}
$$

Substitute $M^{\prime}=M \cdot \frac{(R-d)^3}{R^3}$ into the equation:

$$
F=\frac{G \cdot\left(M \cdot \frac{(R-d)^3}{R^3}\right) m}{(R-d)^2}
$$
Simplify:

$$
F=\frac{G M m(R-d)}{R^3}
$$
Since,

$g=\frac{G M}{R^2}$-----(1)

After substituting the value in equation (1),

$g^{\prime}=g \cdot\left(1-\frac{d}{R}\right)$

(iii) Shape of the earth

The earth is not a perfect sphere. It is somewhat flat at the two poles. The equatorial radius (R eq ) is approximately 21 km more than the polar radius ( R p ).

$g_p=\frac{G M}{R_p^2}$

Acceleration due to gravity at the equator,

$g_{e q}=\frac{G M}{R_{e q}^2}$

∵Rp<Req

∴gp>geq

In short, by going through this article which starts with the difference between capital g and small g, and covers the relation between G and g along with the last value of g in different surfaces you have a solid understanding of this concept. Solve numerical types of questions based on this concept to boost your confidence before the final board exam or any competitive exams.

Also, check-

Frequently Asked Questions (FAQs)

1. Which Law Defines the Planetary System of the Sun and the Orbit of Satellites Occurring the Planets?

The cosmic law of gravity (cited by Isaac Newton) formed the basis for the description of the planets orbiting the sun and the orbits of satellites orbiting the planets.

2. What is gravity made up of?

Gravity is made up of quantum particles. These quantum particles are called gravitons. These gravitons are lightweight, but they contain energy.

3. Give the formula for the relation between small g and capital G.

g = GM / R²

4. What is small g and capital G

The acceleration of gravity is usually denoted by a small g and the universal gravitational constant is often denoted by a capital G.

5. Can g ever be negative?

g cannot be negative in the usual sense, as it represents the magnitude of gravitational acceleration. However, the direction of g can be considered "negative" when it points upward, such as for an object thrown upwards.

6. How does air resistance affect g?

Air resistance doesn't affect g itself, but it affects the observed acceleration of falling objects. In a vacuum, all objects fall at the same rate (g), but air resistance causes lighter or less aerodynamic objects to fall more slowly in air.

7. How would g change if Earth were hollow?

If Earth were hollow, g would be zero inside the hollow sphere and would decrease as you approach the center from the surface. This is due to the shell theorem in physics, which states that a spherical shell of matter exerts no net gravitational force on objects inside it.

8. How would g change if you were standing on a planet twice the size of Earth but with the same density?

If you were on a planet twice the size of Earth but with the same density, g would be twice as large. This is because g is proportional to the radius for planets of the same density, as mass increases with the cube of radius while g decreases with the square of radius.

9. How does g affect the escape velocity of a planet?

g directly affects escape velocity. The escape velocity is proportional to the square root of g and the planet's radius. A higher g means a higher escape velocity is needed to overcome the planet's gravitational pull.

10. What is the difference between G and g in physics?

G is the universal gravitational constant, while g is the acceleration due to gravity on Earth's surface. G is constant throughout the universe, but g varies depending on location and altitude.

11. How are G and g related mathematically?

G and g are related through the equation g = GM/R², where M is the mass of the Earth and R is the distance from the center of the Earth. This shows that g depends on G, but also on the specific properties of the planet.

12. Why is the value of G so much smaller than g?

G is much smaller than g because it represents the intrinsic weakness of gravity compared to other fundamental forces. The large value of g on Earth is due to the Earth's enormous mass, not the strength of gravity itself.

13. Why don't we use G in everyday calculations involving gravity on Earth?

We don't use G in everyday calculations because it's more convenient to use g, which already incorporates Earth's mass and radius. G is primarily used for astronomical calculations involving other celestial bodies.

14. If you could stand on the surface of the Sun, would g be larger or smaller than on Earth?

g would be much larger on the Sun's surface than on Earth. Despite the Sun's larger radius, its immense mass results in a surface gravity about 28 times stronger than Earth's.

15. Why is G called the universal gravitational constant?

G is called the universal gravitational constant because it has the same value everywhere in the universe. It represents the fundamental strength of gravity and is used in Newton's law of universal gravitation.

16. Does G change in different parts of the universe?

No, G does not change in different parts of the universe. It is a fundamental constant of nature and remains the same everywhere, which is why it's called the universal gravitational constant.

17. How was G first measured?

G was first measured by Henry Cavendish in 1798 using a torsion balance experiment. He measured the tiny gravitational attraction between lead spheres, allowing him to calculate G.

18. Why is it challenging to measure G accurately?

Measuring G accurately is challenging because gravity is the weakest of the fundamental forces. The gravitational attraction between small masses in a laboratory is extremely small and easily affected by external disturbances.

19. How does Einstein's theory of general relativity affect our understanding of G and g?

Einstein's theory of general relativity describes gravity as a curvature of spacetime rather than a force. While it doesn't change G, it provides a more accurate description of gravity in extreme conditions, such as near black holes or in precise GPS calculations.

20. How does g change as you move away from Earth's surface?

As you move away from Earth's surface, g decreases. This is because g is inversely proportional to the square of the distance from Earth's center. The further you are, the weaker the gravitational pull.

21. Can g ever be zero?

Yes, g can be zero at a point where gravitational forces cancel out, such as at the center of the Earth or at a Lagrange point between two massive bodies. However, G is never zero.

22. Why does g vary at different locations on Earth's surface?

g varies slightly at different locations on Earth's surface due to factors like the Earth's rotation, its non-uniform density, and variations in altitude. For example, g is slightly less at the equator than at the poles.

23. How would g change if the Earth's mass doubled but its radius remained the same?

If Earth's mass doubled but its radius remained the same, g would double. This is because g is directly proportional to the mass of the planet, as shown in the equation g = GM/R².

24. How does g on the Moon compare to g on Earth?

g on the Moon is about 1/6 of g on Earth. This is because the Moon has less mass than Earth, resulting in a weaker gravitational pull at its surface.

25. Why don't small objects on Earth gravitationally attract each other noticeably?

Small objects don't noticeably attract each other because the gravitational force depends on mass, and G is very small. The Earth's much larger mass dominates the gravitational effects we observe in everyday life.

26. Is there a relationship between G and other fundamental constants in physics?

While there's no known direct relationship, some theories in physics attempt to link G with other fundamental constants. However, unlike some other constants, G is not yet unified with quantum mechanics or other fundamental forces.

27. Why doesn't G change even though the universe is expanding?

G doesn't change with the expansion of the universe because it's a fundamental constant of nature. The expansion of the universe affects the distances between galaxies but not the strength of the gravitational force itself.

28. How would life on Earth be different if G were significantly larger?

If G were significantly larger, g would increase proportionally. This would lead to a much stronger gravitational field, potentially making it difficult for life as we know it to evolve. Structures and organisms would need to be much stronger to withstand the increased gravitational force.

29. Can g ever be greater than G?

g can be (and usually is) greater than G in terms of numerical value, but this comparison isn't meaningful as they have different units and represent different concepts. G is a universal constant, while g is a local acceleration that depends on mass and distance.

30. How does g affect the period of a pendulum?

The period of a pendulum is proportional to the square root of its length and inversely proportional to the square root of g. A higher g would result in a shorter period, meaning the pendulum would swing faster.

31. Why doesn't doubling the mass of an object double its weight?

Doubling the mass of an object does double its weight because weight is the product of mass and g. Since g remains constant (assuming you're at the same location), doubling mass directly doubles weight.

32. How would g change if Earth were spinning twice as fast?

If Earth were spinning twice as fast, g would decrease slightly, especially at the equator. This is because the increased centrifugal force would counteract gravity more, reducing the effective gravitational acceleration.

33. Is there a maximum possible value for g in the universe?

There's no theoretical maximum for g, but extremely high values of g are found near very dense objects like neutron stars and black holes. However, general relativity becomes important in describing gravity in these extreme conditions.

34. How does g affect the speed of sound in air?

g affects the speed of sound indirectly. While g itself doesn't appear in the equation for the speed of sound, it influences air pressure and density, which do affect sound speed. A higher g would increase air pressure, slightly increasing the speed of sound.

35. Why doesn't g depend on the mass of the falling object?

g doesn't depend on the mass of the falling object due to the equivalence of gravitational and inertial mass. As gravitational force increases with mass, so does the object's resistance to acceleration, resulting in the same acceleration for all masses.

36. How would g change if Earth were perfectly spherical?

If Earth were perfectly spherical, g would be constant over its entire surface. The slight variations in g we observe are partly due to Earth's non-spherical shape (it's an oblate spheroid) and its non-uniform density.

37. Can G be derived from other physical constants?

Currently, G cannot be derived from other physical constants. It is considered a fundamental constant of nature that must be measured experimentally. Some theories attempt to relate G to other constants, but these remain unproven.

38. How does g affect the buoyant force in fluids?

g directly affects the buoyant force as described by Archimedes' principle. The buoyant force is equal to the weight of the displaced fluid, which is proportional to g. A higher g would result in a stronger buoyant force.

39. Why is g considered a vector while G is a scalar?

g is considered a vector because it has both magnitude and direction, pointing towards the center of the Earth. G, on the other hand, is a scalar constant that represents the strength of gravity but doesn't have a direction associated with it.

40. How would g change if you were in a falling elevator?

In a freely falling elevator, you would experience apparent weightlessness, and the effective g would be zero relative to the elevator. This is because both you and the elevator are accelerating at the same rate due to gravity.

41. Can g ever be larger than 9.8 m/s² on Earth?

Yes, g can be slightly larger than 9.8 m/s² in some locations on Earth. For example, at the poles, g is about 9.83 m/s² due to the Earth's shape and rotation. Variations in local geology can also cause slight increases.

42. How does g affect the speed of a satellite in orbit?

g affects satellite speed indirectly. The orbital velocity of a satellite depends on g and the orbital radius. A higher g would require a higher orbital velocity for a satellite at a given altitude to maintain its orbit.

43. Why doesn't G change over time?

Current physics theories and observations suggest that G doesn't change over time because it's a fundamental constant of nature. However, some theories propose that G might have varied in the early universe or might be slowly changing, but no conclusive evidence supports this yet.

44. How would g be affected if Earth had a large moon like Jupiter's Ganymede?

If Earth had a large moon like Ganymede, g on Earth's surface would vary more noticeably with the moon's position. The moon's gravitational pull would cause larger tidal effects, slightly increasing or decreasing the effective g depending on its position relative to an observer on Earth.