Relative Speed - Definition, Formula, FAQs

Edited By Vishal kumar | Updated on Sep 24, 2024 09:47 PM IST

In this article, we will discuss, what is meant by relative? What is the meaning of speed? What is relative speed? What is relative speed formula?/ How to calculate relative speed? What is example of relative speed? How can we check the train speed? So let’s see.
Note: stationary meaning in tamil is நிலையான, relatives meaning in telugu is బంధువులు

JEE Main 2025: Physics Formula | Study Materials | High Scoring Topics | Preparation Guide

JEE Main 2025: Syllabus | Sample Papers | Mock Tests | PYQs | Study Plan 100 Days

NEET 2025: Syllabus | High Scoring Topics | PYQs

Relative Speed - Definition, Formula, FAQs

What is meant by relative?

“Relative” means “in comparison to”. The relative meaning in Tamil is உறவினர் (Uravinar), the Relative meaning in Telugu is చుట్టపువాడు. The stationary meaning in telugu is నిశ్చలమైన (Niscalamaina). Thus, the conceptualization of relative speed is used when two or more bodies in motion with some pace (speed) are contemplated. To make things easier, one body are often made stationary (i.e. Speed = 0) and take the pace of the other body with respect to the stationary body, which is the sum of the speeds if the bodies are moving in the converse i.e., opposite direction and the distinction if relative speed moving in the same direction. This speed of the moving body with reference to the stationary body is known as the relative Speed.

Relative speed of 2 bodies = Sum of their individual pace (speeds) if they are moving in the opposite direction = Distinction of their individual pace if they are moving in the same direction.

Also read -

What is the meaning of speed?

Speed is the ratio of distance travelled by unit time. It is a scalar quantity as it has only magnitude.

Expression for speed of a body travelling in a straight line is given by:

r= d / t

Here r is the speed, d is the distance covered, t is the time.

How to calculate relative speed?

Formula for relative speed: Assume two bodies are moving at a different pace (speed) in the same direction.

Consider the speed of first body occur x km/hr

And the speed of the second body occurs y km/hr .

Therefore, its relative speed is = (x-y)km/hr [x>y]

Next,

The time after which both the bodies meet = distance travelled / relative speed

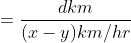
$=\frac{dkm}{(x-y)km/hr}$

Therefore, we already know that relative speed is the speed of one object with reference to another.

So, time after which each of two bodies meet,

Assume, time = t hrs.

So, the distance covered in ‘t’ hours = relative speed × time

= (x – y) km/hr × t hrs.

Related Topics Link,

What is train?

A train may be a kind of rail transport consisting of a series of connected vehicles that generally line a railroad (or railway) track to move passengers or cargo (also referred to as "freight" or "goods").

What is move/define move.

Definition of move: In physics, move/motion is the occurrence in which a body or object changes its position over time. Motion is mathematically found in terms of displacement, distance, velocity, acceleration, speed, and time. As there is no absolute frame of reference, absolute motion cannot be found.

What is train speed formula?

The train speed check can be done using the train Speed Formula.

Here are a few problems on trains for easy and prompt solutions of train-based questions:

Remember some pivotal formulas of train problems for quick solutions.

xkm/hr=x×5 / 18(m/s)
x m/s=x×18 / 5(km/hr)
Time taken by the object (train) of length L to travel a pole or a single post or a standing man is equal to the time taken by the train to cover L distance.
Time taken by the train of length l meters (m) to move an unmoving object of length b meters is the time taken by train to cover (l + b) meters.
Assume that bodies or two trains are travel (moving) in the same direction at

μm/s & v m/s

Where u>v then the relative speed in the same direction is

= (u - v) m/s.

Assume that two objects/bodies or two trains are moving in the opposite direction at u m/s andv m/s, then their relative's speed is = (u + v) m/s.
Let us consider that the two trains of length A,B are moving in opposite direction at u m/s & v m/s, then interval taken by the trains to intersect or cross each other is = (A+B)/(u+v)
Let us consider two trains of length A meters (m) and B meters (m) getting in a same direction at u m /s & v m/ s,

Then the time taken by the faster train to intersect the slower train

= (A+B)/(u-v)

Consider two bodies trains starting at same time from points A and B in the direction (way) of each other and after crossing they take a and b sec in reaching B and A respectively, then

(A’s speed): (B's speed) =(b:a).

Also Read:

What is an example of relative speed?

1. Two trains running in opposite directions cross a man standing on the station in 27 s (Seconds) and 17 s respectively and they intersect (cross) each other in 23 seconds. The ratio of their speed is ____?

Solution:

Let the speeds of the two trains be x m/sec and y m/sec .

So, length of the 1st train = 27x m (metres)

And length of the 2nd train = 17y m (metres).

∴ 27x+17y / x+y=23

Therefore: 27x+17y=23x+23y

4x=6y

So, x / y=3 / 2

Therefore, the ratio of their speeds is 3:2

A body is moving with velocity 30 m/s towards east/a body is moving with velocity 30m s towards east. After 10 s its velocity becomes 40 m/s towards north. The average acceleration of the body is

Solution: We have to formula used

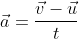
$\vec{a}=\frac{\vec v-\vec u}{t}$

$R=\sqrt{A^2+B^2+2AB cos\theta}$

Acceleration is the change in velocity in one unit of your time. It tells us the speed at which the speed of a given body is increasing or decreasing with reference to time. In other words, what proportion of the speed is increasing or decreasing in one unit time.

Average acceleration is defined because of the difference within the final and initial velocities of the body upon the time taken by the body to succeed in the ultimate velocity from its initial velocity.

i.e. a=v-u / t……..(1)

Where a = acceleration of the body,

v = initial velocity and u = final velocity respectively and

t = time taken by the body.

Since, velocity and acceleration are vector quantities. Therefore we can write the Eq.(i) is,

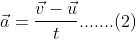
$\vec a=\frac{\vec v-\vec u}{t}.......(2)$

To determine the average acceleration (a) of the given body, first determine the value of v, u and t.

Initial velocity is

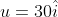
$u=30\hat{i}$

Final velocity is

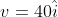
$v=40\hat{i}$

Change in the velocity

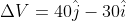
$\Delta V=40\hat{j}-30\hat{i}$

Magnitude is

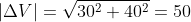
$\left |\Delta V \right |=\sqrt{30^2+40^2}=50$

Average acceleration is

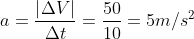
$a=\frac{\left | \Delta V \right |}{\Delta t}=\frac{50}{10}=5m/s^2$

Also check-

NCERT Physics Notes:

Frequently Asked Questions (FAQs)

1. Relative Speed in Opposite Direction.

The object or body relative speed in a distinct direction is shown:

Let us say a man is traveling from Ajmer to Delhi, and the second man is traveling from Delhi to Ajmer in opposite directions. Then we can find out the relative speed as (a+b) or the sum of two velocities.

2. A car is moving with a speed of 30m/s on a circular path on a radius 500m. It is increasing at the speed (rate) of 2m/sec2. Find out the acceleration of the car?

The object or body relative speed in a distinct direction is shown:

3. A train of length 200m traveling at a speed of 30m/s over takes second one having 300 m train length that is traveling at a pace of 20ms −1in the similar direction. The time taken by the 1st train to pass the second train is:

If we see in question, then both two trains are moving in same direction,

Therefore the relative speed = 30-20 = 10m/s

Total distance = 200 + 300 = 500m

Therefore, the time taken = 500 / 10

=50s

4. 2nd sitting in train means?

2nd refers to second seating or second seater in train seats. The seats are present within the sort of benches. This coach doesn't have the power for the passengers to sleep since there are not any berths and it's a sitting arrangement.

5. What is the opposite of fast and opposite of moving?

Opposite of fast-Slow

Opposite of moving- Stationary

6. What is the speed of light in kmph?

1 speed of light = 1.079e⁺⁹