Series LCR Circuit

A Series LCR Circuit consists of an inductor (L), capacitor (C), and resistor (R) connected in series with an alternating current (AC) source. This circuit is fundamental in understanding AC circuit behaviour, particularly resonance, where the inductive and capacitive reactances cancel each other out, resulting in a purely resistive circuit. In real life, series LCR circuits are widely used in various applications such as radio receivers and filters, where they help in tuning to specific frequencies and eliminating unwanted signals. In this article, we will explore the principles of series LCR circuits, we gain insights into how electronic devices manage signal processing and energy transfer efficiently.

JEE Main 2025: Physics Formula | Study Materials | High Scoring Topics | Preparation Guide

JEE Main 2025: Syllabus | Sample Papers | Mock Tests | PYQs | Study Plan 100 Days

NEET 2025: Syllabus | High Scoring Topics | PYQs

This Story also Contains

1. Series LCR Circuit
2. Solved Examples Based on Series LCR Circuit
3. Summary

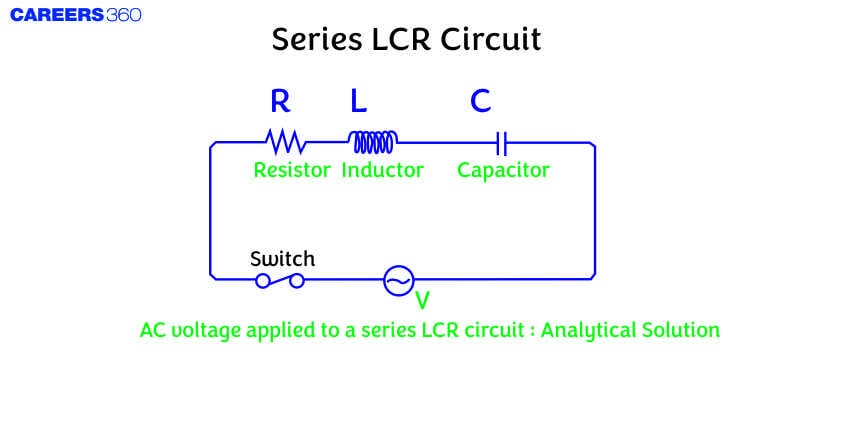
Series LCR Circuit

A Series LCR Circuit consists of an inductor (L), a capacitor (C), and a resistor (R) connected in series with an alternating current (AC) source. The behaviour of this circuit is governed by the interplay of inductive reactance (XL), capacitive reactance (XC), and resistance (R), which together determine the overall impedance (Z) of the circuit.

The Figure given above shows a circuit containing a capacitor, resistor and inductor connected in series through an alternating/sinusoidal voltage source.

As they are in series the same amount of current will flow in all three circuit components and for the voltage, the vector sum of potential drop across each component would be equal to the applied voltage.

Let 'i' be the amount of current in the circuit at any time and V_L, V_C and V_R the potential drop across L, C and R respectively then

${\mathrm{v}}_{\mathrm{R}}=\mathrm{iR}\to$ Voltage is in phase with i
${\mathrm{v}}_{\mathrm{L}=\mathrm{i}\omega \mathrm{L}}\to$ voltage is leading i by ${90}^{\circ }$
${\mathrm{v}}_{\mathrm{c}}=\mathrm{i}/\omega \mathrm{c}\to$ voltage is lagging behind i by ${90}^{\circ }$

By all these, we can draw a phasor diagram as shown below

One thing that should be noticed is that we have assumed that V_L is greater than V_C which makes i lag behind V. If V_C > V_L then I lead V. So as per our assumption, their resultant will be (V_L -V_C). So, from the above phasor diagram V will represent the resultant of vectors V_R and (V_L -V_C). So the equation becomes

$\begin{array}{rl}V& =\sqrt{V_R^2+{(V_L-V_C)}^2}\\ & =i\sqrt{R^2+{(X_L-X_C)}^2}\\ & =i\sqrt{R^2+{(\omega L-\frac{1}{\omega C})}^2}\\ & =iZ\end{array}$
where,
$Z=\sqrt{R^2+{(\omega L-\frac{1}{\omega C})}^2}$

Here, Z is called the Impedance of this circuit.

Now come to the phase angle. The phase angle for this case is given as

$\tan \phi =\frac{V_L-V_C}{V_R}=\frac{X_L-X_C}{R}=\frac{\omega L-\frac{1}{\omega C}}{R}$

Now from the equation of the phase angle, three cases will arise. These three cases are

(i) When, $\omega L>\frac{1}{\omega C}$ then, tanφ is positive i.e. φ is positive and voltage leads the current i.
(ii) When $\omega L<\frac{1}{\omega C}$ then, tanφ is negative i.e. φ is negative and voltage lags behind the current i.
(iii) When $\omega L=\frac{1}{\omega C}$ then tanφ is zero i.e. φ is zero and voltage and current are in phase. This is called electrical resonance.

Recommended Topic Video

Solved Examples Based on Series LCR Circuit

Example 1: Current in resistance is 1 A, then:

1) ${\mathrm{V}}_{\mathrm{s}}=5\mathrm{V}$
2) The impedance of the network is $5\mathrm{\Omega }$
3) The power factor of the given circuit is 0.6 lagging (current is lagging)
4) All of the above

Solution:

$\begin{array}{rl}& {\mathrm{V}}_{\mathrm{s}}^2=(3{)}^2+(8-4{)}^2;{\mathrm{V}}_{\mathrm{s}}=5\mathrm{V}\\ & \text{Now,}\mathrm{Z}=\frac{{\mathrm{V}}_{\mathrm{s}}}{\mathrm{I}}=\frac{5}{1}=5\mathrm{\Omega }\text{Also,}{\mathrm{V}}_{\mathrm{R}}=\mathrm{IRor}\mathrm{R}=\frac{3}{1}=3\mathrm{\Omega }\text{So,}\\ & \mathrm{PF}=\frac{\mathrm{R}}{\mathrm{Z}}=\frac{3}{5}=0.6\text{as}{\mathrm{V}}_{\mathrm{L}}>{\mathrm{V}}_{\mathrm{C}}\Rightarrow \mathrm{I}\text{lagsV,}\end{array}$
so this is a lagging nature.

Hence, the answer is the option (4).

Example 2: Which of the following statements is correct regarding the AC circuit shown in the adjacent figure?

1) The RMS value of current through the circuit is $i_{\mathrm{rms}}=5\sqrt{2}\mathrm{A}$
2) The phase difference between source emf and current is
$\varphi ={\cos }^{-1}\left(\frac{1}{3}\right)$
3) The average power dissipated in the circuit is 500 W
4) None of the above

Solution:

$\begin{array}{rl}& {\mathrm{X}}_{\mathrm{C}}={\mathrm{X}}_{\mathrm{L}}\\ & \therefore \mathrm{Z}=\mathrm{R}=2\mathrm{\Omega }\Rightarrow {\mathrm{I}}_{\mathrm{rms}}=\frac{{\mathrm{V}}_{\mathrm{mms}}}{\mathrm{Z}}=\frac{100/\sqrt{2}}{2}=25\sqrt{2}\mathrm{A}\end{array}$

The phase difference will be zero.
$\text{Average power}={\mathrm{V}}_{\mathrm{rms}}{\mathrm{I}}_{\mathrm{mms}}\cos \varphi =\frac{100}{\sqrt{2}}\times 25\sqrt{2}\times \cos 0^{\circ }=2500\mathrm{W}$

Hence, the answer is the option (4).

Example 3: An inductor L, a capacitor of $20\mu \mathrm{F}$ and a resistor of $10\mathrm{\Omega }$ are connected in series with an AC source of frequency 50 Hz. If the current is in phase with the voltage, then the inductance of the inductor is:

1) 2.00 H

2) 0.51 H

3) 1.5 H

4) 0.99 H

Solution:

In an L-C-R circuit, the current and the voltage are in phase $(\varphi =0)$, when

or
$\begin{array}{rl}& \tan \varphi =\frac{\omega \mathrm{L}-\frac{1}{\omega \mathrm{C}}}{\mathrm{R}}=0\\ & \text{or}\\ & \omega \mathrm{L}=\frac{1}{\omega \mathrm{C}}\text{or}\mathrm{L}=\frac{1}{{\omega }^2\mathrm{C}}\\ & \text{Here,}\omega =2\pi \mathrm{f}=2\times 3.14\times 50{\mathrm{s}}^{-1}=314{\mathrm{s}}^{-1}\\ & \mathrm{C}=20\mu \mathrm{F}=20\times {10}^{-6}\mathrm{F}\\ & \therefore \mathrm{L}=\frac{1}{{\left(314{\mathrm{s}}^{-1}\right)}^2\times (20\times {10}^{-6}\mathrm{F})}=0.51\mathrm{H}\end{array}$

Hence, the answer is the option (2).

Example 4: An L-C-R series circuit consists of a resistance of $10\mathrm{\Omega }$ a capacitor of reactance $60\mathrm{\Omega }$ and an inductor coil. The circuit is found to resonate when put across a 300 V, 100 Hz supply. The inductance of the coil is (take, $\pi =3$)

1) 0.1 H

2) 0.01 H

3) 0.2 H

4) 0.02 H

Solution:

Angular velocity,

$\begin{array}{rl}& {\omega }_0=2\pi \mathrm{v}=2\pi \times 100\\ & {\omega }_0=2\times 3\times 100\\ & =600{\mathrm{rads}}^{-1}\end{array}$

Further, ${\omega }_0=\frac{1}{\sqrt{\mathrm{LC}}}$
$\begin{array}{rl}& {\mathrm{X}}_{\mathrm{C}}=\frac{1}{\mathrm{C}{\omega }_0}=60\mathrm{\Omega }\\ & \Rightarrow \mathrm{C}=\frac{1}{{\omega }_0\times 60}=\frac{1}{600\times 60}\Rightarrow \mathrm{C}=\frac{1}{36\times {10}^3}\mathrm{F}\end{array}$

So, put values in eq. (i), we get
$\begin{array}{rl}& 600=\frac{1}{\sqrt{L\left(\frac{1}{36\times {10}^3}\right)}}\\ & \Rightarrow 36\times {10}^4=\frac{36\times {10}^3}{L}\Rightarrow \mathrm{L}=\frac{36\times {10}^3}{36\times {10}^4}=\frac{1}{10}=0.1\mathrm{H}\end{array}$

Hence, the answer is the option (1).

Example 5: In the series L-C-R circuit, the voltmeter and ammeter readings are:

1) V = 100 V, I = 2 A

2) V = 100 V, I = 5 A

3) V = 1000 V, I = 2A

4) V = 3000 V, I = 1 A

Solution:

$\begin{array}{rl}& \mathrm{V}=\sqrt{{\mathrm{V}}_{\mathrm{R}}^2+{({\mathrm{V}}_{\mathrm{L}}-{\mathrm{V}}_{\mathrm{C}})}^2}\\ & \text{As}{\mathrm{V}}_{\mathrm{L}}={\mathrm{V}}_{\mathrm{C}}=400\mathrm{V}\\ & \therefore \mathrm{V}={\mathrm{V}}_{\mathrm{R}}\\ & \text{Reading of voltmeter}=100\mathrm{V}\\ & \text{Reading of ammeter,}{\mathrm{I}}_{\mathrm{rms}}=\frac{{\mathrm{V}}_{\text{rms}}}{\mathrm{Z}}=\frac{100}{50}=2\mathrm{A}\end{array}$

Hence, the answer is the option (1).

Summary

A Series LCR Circuit, composed of an inductor (L), capacitor (C), and resistor (R), connected in series with an AC source, demonstrates critical principles of AC circuit behaviour. At resonance, the inductive and capacitive reactances cancel each other, resulting in a purely resistive circuit. This phenomenon is essential in various applications, such as tuning radio frequencies and filtering signals. By understanding the impedance and phase angles of series LCR circuits, we gain insights into efficient signal processing and energy management in electronic devices.