Stopping Of Block Due To Friction

Friction is the opposing force to the motion of one body over the surface of another body. As in the case of a ball rolling freely on the ground, the ball ultimately comes to rest, due to the frictional force that exists between the ball and the ground. Friction is a force that is around us all of the time, and it opposes relative motion between systems in contact and also allows us to move. It is parallel to the surface and opposite to the direction of the intended motion.

JEE Main 2025: Physics Formula | Study Materials | High Scoring Topics | Preparation Guide

JEE Main 2025: Syllabus | Sample Papers | Mock Tests | PYQs | Study Plan 100 Days

NEET 2025: Syllabus | High Scoring Topics | PYQs

This Story also Contains

1. On the Horizontal Road
2. On the Inclined Road
3. Solved Examples Based on Stopping of Block Due to Friction
4. Summary

In this article, we will cover the concept of stopping Block Due To Friction. This concept falls under the broader category of law of motion which is a crucial chapter in Class 11 physics. It is not only essential for board exams but also for competitive exams like the Joint Entrance Examination (JEE Main), National Eligibility Entrance Test (NEET), and other entrance exams such as SRMJEE, BITSAT, WBJEE, BCECE and more. Over the last ten years of the JEE Main exam (from 2013 to 2023), a total of three questions have been asked on this concept and no direct question was asked in NEET.

On the Horizontal Road

A block of mass m is moving initially with velocity u on a rough surface and due to friction, it comes to rest after covering a distance S.

• Distance travelled before coming to rest (S):

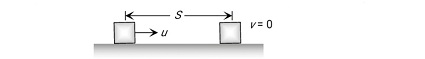
F=ma=μRma=μmga=μgV2=u2−2asS=u22μg=P22μm2g

Where:

a= acceleration μ= coefficient of friction S= distance travelled g= gravity u= initial velocity V= finally velocity P= initial mometum=mu

• Time taken to come to rest:

From equation, v=u−at⇒0=u−μgt[ As v=0,a=μg]
∴t=uμg

• The force of friction acting on the body:

F=maF=m(v−u)tF=mut[ As v=0]F=μmg[ As t=uμg]

On the Inclined Road

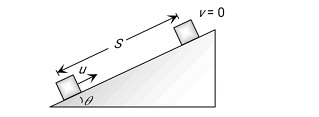
a=g[sin⁡θ+μcos⁡θ]V2=u2−2aS0=u2−2g[sin⁡θ+μcos⁡θ]SS=u22g(sin⁡θ+μcos⁡θ)

Where:

S= distance travelled μ= coefficient of friction V= Final velocity u= Initial velocity

Recommended Topic Video

Solved Examples Based on Stopping of Block Due to Friction

Example 1: A 2 kg mass starts from rest on an inclined smooth surface with an inclination 30∘ and a length 2 m. How much will it travel (in meters) before coming to rest on a surface with a coefficient of friction of 0.25?

1) 4

2)6

3)8

4)2

Solution:

v2=u2+2as=0+2×gsin⁡30×2

Let it travel a distance ' S ' before coming to rest on a horizontal surface
S=v22μg=202×0.25×10=4 m

Hence, the answer is option (1).

Example 2: A body is moving with a speed of 12m/s and the coefficient of friction between the ground and the body is 0.4. The distance travelled by the body (in meters) before coming to rest is.

1) 18

2)10

3)15

4)20

Solution:

f=μmg
due to retardation
μmg=ma⇒a=μg=0.4∗10=4 m/s2
now for V=0
V2=u2−2as⇒0=u2−2asS=u22×a⇒12∗122∗4=18 m

Hence, the answer is option (1).

Example 3: A block of mass 10 kg starts sliding on a surface with an initial velocity of 9.8 ms−1. The coefficient of friction between the surface and block is 0.5. The distance covered by the block before coming to rest is:
[ use g=9.8 ms−2]

1) 4.9 m
2) 9.8 m
3) 12.5 m
4) 19.6 m

Solution:

f=μN=μmga=μg=4.9 m/s2V2=u2+2as0=(9.8)2+2(−4.9)ss=9.8 m
The distance covered by the block before coming to rest is

Hence, the correct option is (2)

Example 4: A bag is gently dropped on a conveyor belt moving at a speed of 2 m/s. The coefficient of friction between the conveyor belt and bag Initially, the bag slips on the belt before it stops due to friction. The distance travelled by the bag on the belt during the slipping motion, is : [Take g=10 m/s−2 ]

1) 2 m
2) 0.5 m
3) 3.2 m
4) 0.8 ms

Solution:

f=μmga=fm=μg

The bag comes to rest when the speed of the bag is the same as the conveyor belt ie., 4 m/s
v2=u2+2 as (4)2=0+2(0.4×10)sS=2 m

The distance travelled by the bag on the belt during slipping motion is 2 m

Hence 1 is the correct option.

Example 5: A block of mass M slides down on a rough inclined plane with constant velocity. The angle made by the inclined plane with horizontal is θ. The magnitude of the contact force will be:

1) Mg
2) Mgcos⁡θ
3) Mgsin⁡θ+Mgcos⁡θ
4) Mgsin⁡θ1+μ

Solution:

For block moving at constant velocity

∑Fx=0−mgsin⁡Θ+μMgcos⁡Θ=0tan⁡Θ=μf=Mgsin⁡Θ=μMgcos⁡Θ→(1) Contact force =f2+N2(Mgsin⁡Θ)2+(Mgcos⁡Θ)2 Contact force =Mg

Hence, the answer is option (1).

Summary

When a moving block encounters friction, the force of friction opposes its motion, gradually reducing its speed until it comes to a complete stop. The stopping distance depends on the initial speed of the block and the coefficient of friction between the block and the surface. Understanding this interaction is crucial for applications requiring precise control of motion and stopping.