Work Done Against Gravity

In our everyday lives, we frequently encounter situations where work is done against gravity, often without even realizing it. Whether lifting groceries into a car trunk or climbing stairs, these common activities involve exerting force to overcome gravity's pull. Understanding the concept of work done against gravity not only enriches our grasp of basic physics but also helps us appreciate the energy involved in our daily tasks. This article explores this fundamental concept, illustrated with real-life scenarios and a solved example to demonstrate how physics applies to everyday experiences. By delving into the mathematics and principles underlying such actions, we gain a clearer insight into the forces at play in our routine movements.

JEE Main 2025: Physics Formula | Study Materials | High Scoring Topics | Preparation Guide

JEE Main 2025: Syllabus | Sample Papers | Mock Tests | PYQs | Study Plan 100 Days

NEET 2025: Syllabus | High Scoring Topics | PYQs

This Story also Contains

1. Work Done Against Gravity
2. Solved Examples Based on Work Done Against Gravity
3. Summary

Work Done Against Gravity

The gravitational potential energy at height 'h' from the earth's surface Is given by

$U_h=-\frac{mgR}{1+\frac{h}{R}}$

So at the surface of the earth put $\mathrm{h}=0$
We get $U_s=-mgR$

So if the body of mass m is moved from the surface of the earth to a point at height h from the earth's surface

Then there is a change in its potential energy.

This change in its potential energy is known as work done against gravity to move the body from the earth's surface to height h.

$W=\mathrm{\Delta }U=GMm[\frac{1}{r_1}-\frac{1}{r_2}]$

Where $W\to$ work done
$\mathrm{\Delta }U\to$ change in Potential energy
$r_1,r_2\to$ distances
Putting $r_1=R$, and $r_2=R+h$

So

$W=\mathrm{\Delta }U=GMm[\frac{1}{R}-\frac{1}{R+h}]$

when ' $h$ ' is not negligible

$W=\frac{mgh}{1+\frac{h}{R}}$

when ' $h$ ' is very small

$W=\frac{mgh}{1+\frac{h}{R}}$
But $h$ is small compared to Earth's radius

$\frac{h}{R}\to 0$

$\mathrm{So}W=mgh$
If $\mathrm{h}=\mathrm{nR}$ then

$W=mgR\ast \frac{n}{n+1}$
Recommended Topic Video

Solved Examples Based on Work Done Against Gravity

Example 1: A satellite is revolving in a circular orbit at a height ‘h’ from the earth’s surface (radius of earth R; h<<R). The minimum increase in its orbital velocity required, so that the satellite could escape from the earth’s gravitational field, is close to : (Neglect the effect of atmosphere.

1) $\sqrt{2gR}$
2) $\sqrt{gR}$
3) $\sqrt{gR/2}$
4) $\sqrt{gR}(\sqrt{2}-1)$

Solution:

Orbital velocity of the satellite

$\begin{array}{rl}V& =\sqrt{\frac{GM}{r}}\\ r& =R+h\end{array}$

$r\to$ Position of the satellite from the centre of the earth

$V\to \text{Orbital velocity}$

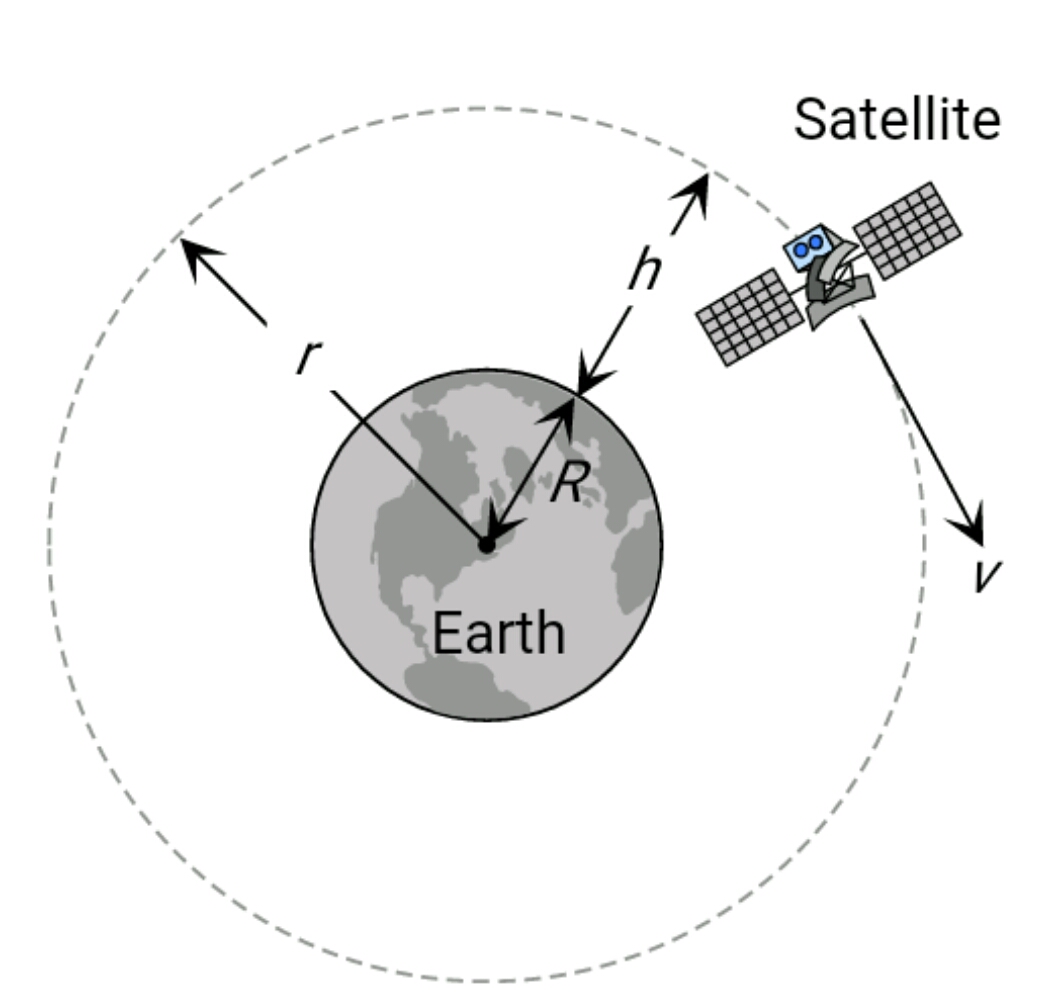
wherein
The velocity required to put the satellite into its orbit around the earth.
So

$v_0=\sqrt{\frac{Gm}{R+h}}$

For escape velocity
Apply energy conservation

$\frac{1}{2}m{v}_e^2=\frac{Gmm}{R+h}\Rightarrow v_e=\sqrt{\frac{2Gm}{R+h}}=\sqrt{\frac{2Gm}{R}}(\because h<<R)$

required increment in the orbital velocity

$\begin{array}{rl}& v_e-v_0=\sqrt{\frac{2Gm}{R}}-\sqrt{\frac{Gm}{R}}=\sqrt{\frac{Gm}{R}}(\sqrt{2}-1)\\ & v_e-v_0=\sqrt{gR}(\sqrt{2}-1)\end{array}$

Example 2: The gravitational potential energy of a body of mass ' $m$ ' at the earth's surface is $-{\mathrm{mgR}}_{\mathrm{e}}$. Its gravitational potential energy at a height $R_e$ from the earth's surface will be ( $R_e$ is the radius of the earth)
1) $-2mg{R}_e$
2) $2{\mathrm{mgR}}_{\mathrm{e}}$
3) $1/2{\mathrm{mgR}}_{\mathrm{e}}$
4) $-1/2{\mathrm{mgR}}_{\mathrm{e}}$

Solution:

Work done against gravity
$W=\mathrm{\Delta }U=GMm[\frac{1}{r_1}-\frac{1}{r_2}]$

$W\to$ work done
$\mathrm{\Delta }U\to$ change in Potential energy
$r_1,r_2\to$ distances
wherein
If the body is moved from the surface of the earth to a point $h$ above the surface of the earth then use the given formula

$\begin{array}{rl}& \mathrm{\Delta }U=U_2-U_1=\frac{mgh}{1+\frac{h}{R_e}}\\ & \text{as}\mathrm{h}={\mathrm{R}}_{\mathrm{e}}\\ & \mathrm{\Delta }U=\frac{mg{R}_e}{2}\\ & U_2-(-mg{R}_e)=\frac{mg{R}_e}{2}\\ & U_2=\frac{mg{R}_e}{2}+(-mg{R}_e)=\frac{-1}{2}mg{R}_e\end{array}$

Hence, the answer is the option 4.

Example 3: If $g$ is the acceleration due to gravity on the earth's surface, the gain in the potential energy of an object of mass $m$ raised from the surface of the earth to a height equal to the radius $R$ of the earth is :
1) $2mgR$
2) $\frac{1}{2}mgR$
3) $\frac{1}{4}mgR$
4) $mgR$

Solution:
Work done against gravity when ' $h$ ' is not negligible -

$\begin{array}{rl}& W=\frac{mgh}{1+\frac{h}{R}}\\ & W=\text{workdone}\\ & h\to \text{height above the surface of the earth}\\ & R\to \text{Radius of earth}\end{array}$

if $h=R$
$\begin{array}{rl}& \text{if}h=R\\ & \begin{array}{rl}W& =\frac{1}{2}mgR\\ \text{if}h& =nR\\ W& =mgR\left(\frac{n}{n+1}\right)\\ n& \to \text{times}\\ n& =1,2,3\cdots \end{array}\end{array}$
So here
$F=\frac{Gmm}{x^2}$ at distance $x$ from centre of earth

$dw=\int \frac{Gmm}{x^2}dx=Gmm{\int }_{2R}^R\frac{dx}{x^2}$

$\therefore$ Potential energy gained $=\mathrm{Gmm}|-\frac{1}{x}|$

$=\frac{Gmm\times 1}{2R}$

$\therefore$ Gain in potential energy $=\frac{1}{2}mR\left(\frac{Gm}{R^2}\right)$
$=\frac{1}{2}mR\left(\frac{GM}{R^2}\right)=\frac{1}{2}mgR[\because g=\frac{GM}{R^2}]$

$\therefore$ Gain in potential energy $=\frac{1}{2}mR\left(\frac{Gm}{R^2}\right)=\frac{1}{2}mgR$
Hence, the answer is the option (2).

Example 4: If a body of mass m is moved from the earth's surface at height ‘h’ above the earth work is done against gravity. When (h<<<R) where R is the radius of the earth.

1) -mgR

2) -mgh

3) mgR

4) mgh

Solution:

Work done against gravity when ' $h$ ' is very small

$\begin{array}{rl}& W=\frac{mgh}{1+\frac{h}{R}}\\ & W=mgh\end{array}$
W= work done
$h\to$ height
wherein

$\frac{h}{R}\to 0$

$h$ is small compared to the earth

Work done against gravity
$\begin{array}{rl}& w=\frac{mgh}{1+\frac{h}{R_e}}\\ & \text{if}h\ll \ll <R_{\mathrm{e}}\\ & \frac{h}{R_e}\ll <<<<1\end{array}$

hence $w=\mathrm{mgh}$
Hence, the answer is the option 4.

Summary

This is how the work that the gravitational force does may be determined and calculated. To effectively apply the formula in the exercise questions, be aware of how it was developed. Make sure you utilise the units of these physical quantities appropriately as you practise applying this calculation.