Zener diode as a voltage regulator

Imagine you're on a roller coaster that suddenly has a mechanism to keep the ride smooth, regardless of the ups and downs. This is similar to how a Zener diode functions in an electrical circuit. A Zener diode is designed to maintain a constant voltage level, acting as a voltage regulator. When the voltage in a circuit fluctuates, the Zener diode steps in to stabilize it, ensuring that the connected electronic devices receive a consistent voltage. This regulation is crucial for the reliable operation of various electronic systems, much like how the mechanism in a roller coaster ensures a safe and steady ride for passengers. By maintaining a stable voltage, Zener diodes protect sensitive electronic components from damage and ensure they perform optimally.

This Story also Contains

1. Zener Diode
2. Zener Diode as a Voltage Regulator
3. Solved Examples Based on Zener diode as a Voltage Regulator
4. Summary

Zener Diode

It is invented by C. Zener. A Zener diode is a p-n junction semiconductor device designed to operate in the reverse breakdown region. It is a highly doped p-n junction which is not damaged by high reverse current. It can operate continuously, without being damaged in the region of reverse background voltage. It forms a very thin depletion region and an extremely high electric field across the junction even for a small reverse bias voltage (~5 V).

In the forward bias, the Zener diode acts as an ordinary diode.

Symbol of Zener Diode

The symbol of the Zener diode is shown in the below figure.

VI characteristics of Zener Diode

• Zener Breakdown

When a reverse bias is increased the electric field at the junction also increases. At some stage, the electric field becomes so high that it breaks the covalent bonds creating electron-hole pairs. Thus a large number of carriers are generated. This causes a large current to flow. This mechanism is known as Zener breakdown.

• Avalanche breakdown

At high reverse voltage, due to the high electric field, the minority charge carriers, while crossing the junction acquire very high velocities. These by collision break down the covalent bonds, generating more carriers. A chain reaction is established, giving rise to a high current. This mechanism is known as Avalanche breakdown.

The VI characteristics of a Zener diode are shown in the below figure.

When forward-biased voltage is applied to the Zener diode, it works like a normal diode. When reverse-biased voltage is applied to a Zener diode, it allows only a small amount of leakage current until the voltage is less than the Zener voltage (V_z). As the reverse bias voltage (V) reaches the breakdown voltage of the Zener diode (Vz), there is a large change in current. Also, note that for a negligible change in the reverse bias voltage, a large change in current is produced.

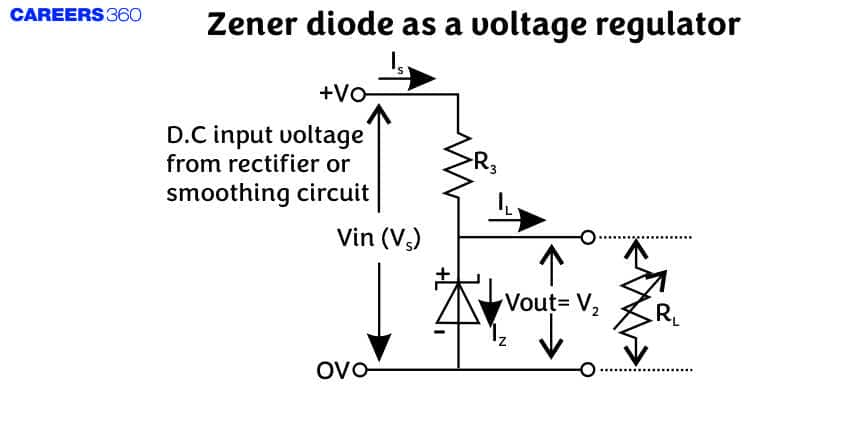
Zener Diode as a Voltage Regulator

A Zener diode is used to get constant DC voltage from a DC unregulated output of a rectifier. The circuit diagram of a voltage regulator using a Zener diode is shown in the below figure.

Here the unregulated DC output of a rectifier is connected to the Zener diode through a series of resistance (R_s) such that the Zener diode is reverse biased. Let's see how it works. If the input voltage increases, the current through R_s and Zener diode also increases. This increases the voltage drop across R_s. But the voltage across the Zener diode does not change, because, in the breakdown region, Zener voltage remains constant despite the change in current.

Similarly, if the input voltage decreases, the current through R_s and Zener diode also decreases. This decreases the voltage drop across R_s . However the voltage across the Zener diode does not change. Hence, a change of voltage drop across the R_s does not change the voltage across the Zener diode.

Hence, the Zener diode acts as a voltage regulator.

Recommended Topic Video

Solved Examples Based on Zener diode as a Voltage Regulator

Example 1: For LEDs to emit light in the visible region of electromagnetic light, they should have an energy band gap in the range of :

1) 0.1 eV to 0.4 eV

2) 0.5 eV to 0.8 eV

3) 0.9 eV to 1.6 eV

4) 1.7 eV to 3.1 eV

Solution:

For emitting visible light $\lambda$ should lie between $4000 A^{\circ}$ to $7600 A^{\circ}$
$
\begin{aligned}
& \therefore E^{\min }=\frac{12400}{7600}=1.7 \mathrm{eV} \\
& \therefore E^{\max }=\frac{12400}{4000}=3.1 \mathrm{eV}
\end{aligned}
$

Hence, the answer is the option (4).

Example 2: Symbolic representation of photodiode is

1)

2)

3)

4)

Solution:

• The photodiode is a special type of PN junction diode made up of photosensitive semiconducting material that generates current when exposed to light.
• It operates in reverse-biased mode and converts light energy into electrical energy.
• The diode has a transparent window to allow light to fall on the diode

Hence, the answer is option (4).

Example 3: Assertion: A P-N photodiode is made from a semiconductor for which E_g = 2.8 eV. This photodiode will not detect the wavelength of 6000 nm.

Reason: A PN photodiode detects wavelength if $\frac{h c}{\lambda}>E_g$

1) both assertion and reason are true and the reason is the correct explanation of the assertion.

2) both assertion and reason are true but the reason is not the correct explanation of the assertion.

3) assertion is true but reason is false.

4) the assertion and reason both are false.

Solution:

For detection of a particular wavelength $(\lambda)$ by a $P N$ photo diode, energy of incident light $>E_g \Rightarrow \frac{h c}{E_g}>\lambda$ For
$
E_g=2.8 \mathrm{eV}, \frac{h c}{E_g}=\frac{6.6 \times 10^{-34} \times 3 \times 10^8}{2.8 \times 1.6 \times 10^{-19}}=441.9 \mathrm{~nm}
$
i.e., $\frac{h c}{E_q}<6000 \mathrm{~nm}$, so diode will not detect the wavelength of 6000 A .

Hence, the answer is the option (1).

Example 4: If a semiconductor photodiode can detect a photon with a maximum wavelength of 400 nm, then its band gap energy (in eV ) is :
Planck's constant $h=6.63 \times 10^{-34} \mathrm{~J} . \mathrm{s}$.
Speed of light $c=3 \times 10^8 \mathrm{~m} / \mathrm{s}$

1) 3.1

2) 2.0

3) 1.5

4) 1.1

Solution:

For the photodiode to detect
$
\begin{aligned}
& E=\frac{h c}{\lambda}>(\text { band gap energy }) \\
& \Rightarrow(\text { band gap energy })_{\max }=\mathrm{hc} / \lambda_{\max } \\
& =\frac{6.63 \times 10^{-34} \times 3 \times 10^8}{400 \times 10^{-9}}=5 \times 10^{-19} \mathrm{~J}=3.1 \mathrm{eV}
\end{aligned}
$

Hence, the answer is option (1).

Example 5: The electrical conductivity of a semiconductor increases when electromagnetic radiation of a wavelength shorter than 2480 nm is incident on it. The band gap in (eV) for the semiconductor is

1) 0.5

2) 0.7

3) 1.1

4) 2.5

Solution:

Electrical conductivity increases when more electrons jump from the valence band to the conduction band. Hence the light must provide energy equal to or more than the band gap.

Band gap $\frac{h c}{\lambda}=\frac{12400}{24800}=0.5 \mathrm{eV}$

Hence, the answer is option (1).

Summary

P-N junction diodes have been designed with specialized uses in mind, other than just rectifying current. Some of these uses include. The first diode is a Zener diode which is used for voltage regulation by maintaining constant voltage across it even if we increase its current. The second diode is known as the Light Emitting Diode (LED) which emits light when current flows through it, they are most appropriate in displays and indicators. Photodiodes are the third kind of diodes and they change light into electricity; it is important to mention that they are commonly applied in optical communication and sensing devices.