Surface Area and Volume of a Prism: Formula, Calculator
The surface area and volume of a Prism is an important chapter of 3d geometry. Prisms can be of many different types such as triangular, rectangular, or pentagonal etc. We will discuss 'Surface Area and Volume of a Prism', 'Surface area and volume of a prism formula', 'Surface area and volume of a prism worksheet', 'Surface area and volume of a prism calculator', 'Surface area and volume of a rectangular prism', 'Surface area and volume of a triangular prism', 'Surface area and volume of a triangular prism calculator', 'Surface area and volume of a rectangular prism formula', and 'Surface area and volume of a hexagonal prism' etc.
CAT 2024: 20 Free Mock Test | 10 Year PYQs | 60 Day Study Material | Most Scoring Concepts
XAT 2025: Section-wise Preparation Tips | Sample Paper
Don't Miss: SNAP 2024 Sample Papers | NMAT 2024 Sample Papers | MAT 2024 Sample Papers
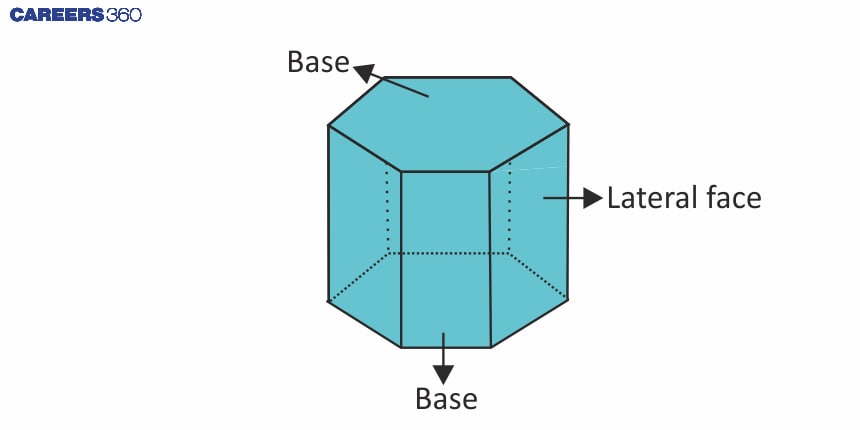
Prism: An Overview
A prism is a three-dimensional object with two parallel, congruent bases covered by rectangular or parallelogram faces. The bases can be of any shape. According to the shapes of their bases, prisms are called triangular, rectangular, and hexagonal.
Examples of Prism
Triangular Prism: A tent with triangular faces.
Rectangular Prism: A common brick or a shoebox.
Pentagonal Prism: A cross-section of certain complex buildings.
Hexagonal Prism: Some nuts and bolts.
What is the Surface area of a Prism?
The surface area of a prism is the total area of all of its faces, including the base, top, and lateral surfaces. It helps in determining how much material is needed to cover the prism.
The formula for the surface area of a Prism
There are two types of formulas for the surface area of a prism, one is the lateral surface area and the other is the total surface area of a prism.
Lateral Surface Area of a Prism
The lateral surface area (LSA) is the sum of the areas of all the lateral faces (the faces excluding the bases).
So, LSA = Perimeter of the Base × Height
The Total surface area of a Prism
The total surface area (TSA) includes the lateral surface area and the area of the two bases.
So, TSA = LSA + (2 × Area of Base)
What is the Volume of a Prism?
The volume of a prism measures the space it occupies. It is found by multiplying the area of the base by the height (the perpendicular distance between the bases).
The formula for the Volume of a Prism
Volume of a Prism = Area of the Base × Height
List of Formulas
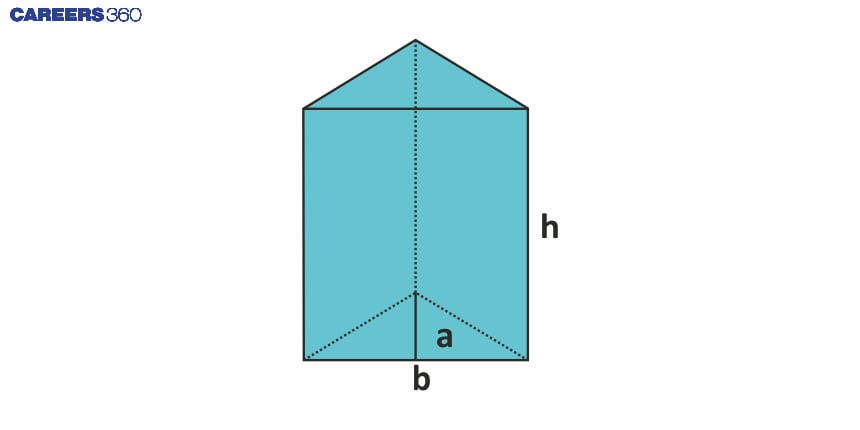
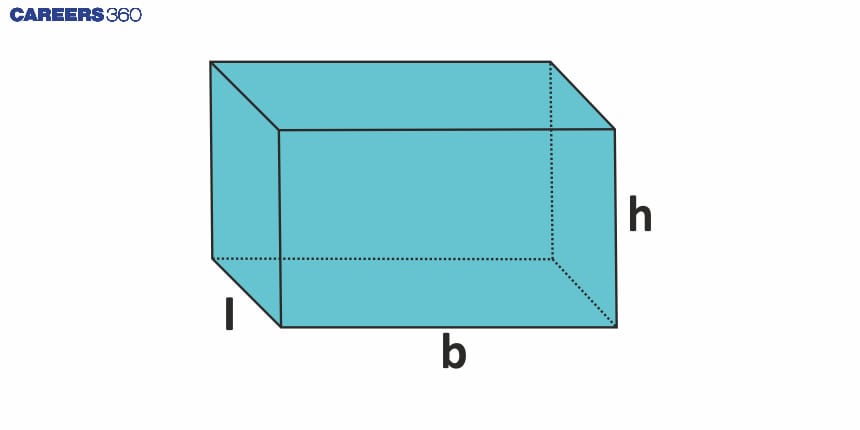
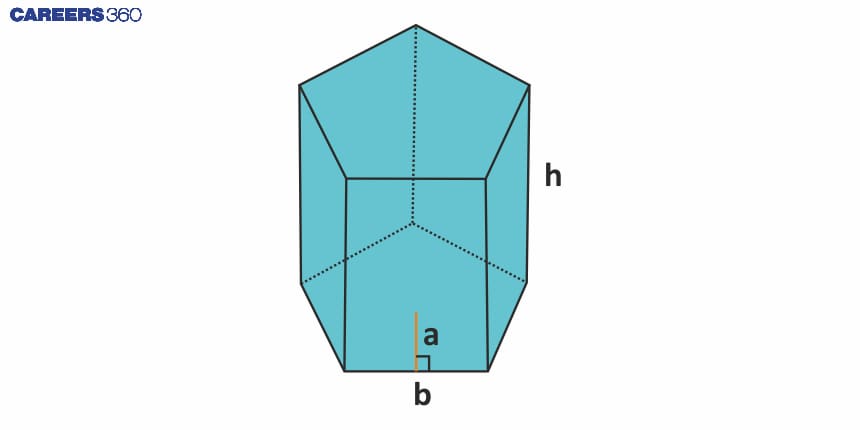
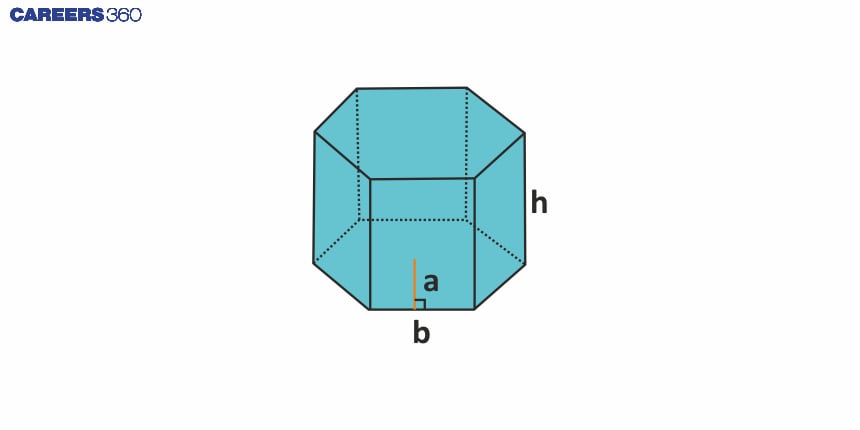
Lateral Surface Area | Base Area | Total Surface Area | Volume | Diagram | |
Triangular Prism | $3bh$ | $\frac{1}{2}ab$ | $ab+3bh$ | $\frac{1}{2}abh$ |
|
Rectangular Prism | $2(lh+hb)$ | $lb$ | $2(bl+lh+hb)$ | $lbh$ |
|
Pentagonal Prism | $5bh$ | $\frac{5}{2}ab$ | $5ab+5bh$ | $\frac{5}{2}abh$ |
|
Hexagonal Prism | $6bh$ | $3ab$ | $6ab+6bh$ | $3abh$ |
|
Important points
The base shape determines the type of prism.
The height is the perpendicular distance between the bases.
The lateral surface area accounts for the sides excluding the bases.
The total surface area includes all faces of the prism.
Volume is the product of the base area and the height.
Practice Questions/Solved Examples
Q.1.
A prism has a regular hexagonal base with a side of 6 cm. If the total surface area of the prism is 216$\sqrt3$ cm2, then what is the height (in cm) of the prism?
$3\sqrt3$
$6\sqrt3$
$3$
$6$
Hint: By using the formula: Total curved surface area of prism = 2 × (Area of base) + 6 × (Side of the base) × height
Solution:
Given: Side, $a$ = 6 cm
Let the height be $h$.
Area of the base of the hexagonal prism $=6 \times \frac{\sqrt3}{4}a^2=6 \times \frac{\sqrt3}{4} \times 6^2 = 54\sqrt3$
Total curved surface area of prism = 2 × (Area of base) + 6 × (Side of the base) × height
⇒ $216\sqrt3 = 2 \times 54\sqrt3 + 6 \times 6 \times h$
⇒ $108\sqrt3 = 36h$
$\therefore h = 3\sqrt3\ \text{cm}$
Hence, the correct answer is $3\sqrt3$.
Q.2.
The base of a prism is a right-angled triangle with two sides 5 cm and 12 cm. The height of the prism is 10 cm. The total surface area of the prism is:
360 sq cm
300 sq cm
300 sq cm
325 sq cm
Hint: Total surface area of a prism = curved surface area + 2 (area of base)
Solution:
Given: Height = 10 cm
Two sides = 5 cm and 12 cm
$\therefore$ Area of the base of the right prism = $\frac{1}{2}×5×12$ = 30 sq cm
Other side of the right-angled triangle $=\sqrt{5^2+12^2}=\sqrt{25+144} = 13$
Curved surface area = perimeter of base × height = (5 + 12 + 13) × 10 = 300 sq cm
$\therefore$ Total surface area = curved surface area + 2 (area of base) = 300 + 2 × 30 = 360 sq cm
Hence, the correct answer is 360 sq cm.
Q.3.
A right prism stands on a base 6 cm equilateral triangle and its volume is $81\sqrt3$ cm3. The height (in cm) of the prism is:
9
10
12
15
Hint: Use the formulas:
Area of equilateral triangle of side $a$ = $\frac{\sqrt{3}a^2}{4}$
Volume of a right prism = Area of base × Height
Solution:
The base of a right prism = 6 cm
Volume $= 81\sqrt3$ cm3
Area of base of prism $= \frac{\sqrt{3}}{4}×6×6 = 9\sqrt{3}$ cm2
Volume of a right prism = area of base × height
⇒ $81\sqrt3=9\sqrt{3}$ × height
⇒ Height = 9 cm
Hence, the correct answer is 9.
Q.4.
If the altitude of a right prism is 10 cm and its base is an equilateral triangle of side 12 cm, then its total surface area (in cm2) is:
$(5+3\sqrt3)$
$36\sqrt3$
$360$
$72(5+\sqrt3)$
Hint: Use the formulas:
Area of equilateral triangle of side $a =\frac{\sqrt3a^2}{4}$
The perimeter of an equilateral triangle of side $a = 3a$
Total surface area of prism = Perimeter of base × Height + 2 × Base area
Solution:
Use the formulas:
Area of equilateral triangle of side $a =\frac{\sqrt3a^2}{4}$
The perimeter of an equilateral triangle of side $a = 3a$
Total surface area of prism = Perimeter of base × Height + 2 × Base area
Height of a right prism = 10 cm
Side of an equilateral triangle, $a$ = 12 cm
Base area $= \frac{\sqrt3a^2}{4} = \frac{\sqrt3}{4}×12×12 = 36\sqrt3$cm2
Perimeter of base $= 3a = 3 × 12 = 36$ cm
Total surface area of prism = (Perimeter of base × Height + 2 × Base area)
$=36×10 + 2×36\sqrt3$
$= 360+72\sqrt3$
$= 72(5+\sqrt3)$ cm2
Hence, the correct answer is $72(5+\sqrt3)$.
Q.5.
The base of a right prism is a right-angled triangle whose sides are 5 cm, 12 cm, and 13 cm. If the area of the total surface of the prism is 360 cm2, then its height is:
10 cm
10 cm
10 cm
10 cm
Hint: Use the formulas:
Area of an equilateral triangle = $\frac{1}{2}$ × Base × Perpendicular
The perimeter of a right triangle = Base + Hypotenuse + Perpendicular
Total surface area of prism = (Perimeter of base × Height + 2 × Base area)
Solution:
The area of the total surface of the prism = 360 cm2
The base of the right-angled triangle = 5 cm
The perpendicular of the right-angled triangle = 12 cm
Hypotenuse of the right-angled triangle = 13 cm
Perimeter of base of prism = (5 + 12 + 13) = 30 cm
Base area = $\frac{1}{2}$ × base × perpendicular
= $\frac{1}{2}$ × 5 × 12
= 30 cm2
Total surface area of prism = (Perimeter of base × Height + 2 × Base area)
⇒ 360 = 30 × height + 2 × 30
⇒ Height = $\frac{360 - 60}{30}$ = 10 cm
Hence, the correct answer is 10 cm.
Q.6.
The base of the right prism, whose height is 2 cm, is a square. If the total surface area of the prism is 10 cm2, then its volume (in cm3) is:
3
1
2
4
Hint: Use the formulas:
The total surface area of the prism = curved surface area + 2 × area of base
The volume of a prism = base area × height
Solution:
Height = 2 cm
The total surface area of the prism = 10 cm2
Let the side of the base be $a$ cm.
The total surface area of the prism = curved surface area + 2 × area of base
10 = perimeter of base × height + 2 × area of base
⇒ (4 × $a$ × 2) + (2 × $a^2$) = 10
⇒ $a^2 + 4a -5$ = 0
⇒ $(a+5)(a-1)$ = 0
Since $a$ cannot be negative,
$a$ = 1
Volume = base area × height
= $(1)^2 × 2$
= 2 cm3
Hence, the correct answer is 2 cm3.
Q.7.
Let ABCDEF be a prism whose base is a right-angled triangle, where sides adjacent to 90$^{\circ}$ are 9 cm and 12 cm. If the cost of painting the prism is INR 151.20, at the rate of 20 paise per sq cm then the height of the prism is:
17 cm
18 cm
15 cm
16 cm
Hint: Use the formula:
Total surface area = perimeter of base × height + 2 × area of base
Solution:
Total surface area of prism = $\frac{\text{Total cost of painting}}{\text{Cost per sq cm}}=\frac{151.2}{0.2}$ = 756 cm2
Hypotenuse of the triangular base = $\sqrt{9^2 + 12^2}$ = $\sqrt{81 + 144}$ = $\sqrt{225}$ = 15 cm
Perimeter of base = 9 + 12 + 15 = 36 cm
Let h be the height of the prism.
Total surface area = perimeter of base × height + 2 × area of base
756 = 36 × h + 2 × $\frac{1}{2}$ × 9 × 12
⇒ 756 = 36h + 108
⇒ h = $\frac{756-108}{36}$ = $\frac{648}{36}$ = 18 cm
Hence, the correct answer is 18 cm.
Q.8.
The base of a solid right prism is a triangle whose sides are 9 cm, 12 cm, and 15 cm. The height of the prism is 5 cm. Then, the total surface area (in cm2) of the prism is:
180
234
288
270
Hint: The total surface area of a prism = 2 × The area of the base + The perimeter of the base × The height of the prism
Solution:
Given that the base of a solid right prism is a triangle whose sides are 9 cm, 12 cm, 15 cm and the height of the prism is 5 cm.
The total surface area of a prism = 2 × The area of the base + The perimeter of the base × The height of the prism
The area of the base = $\frac{1}{2}$ × 9 × 12 = 54 cm2
The perimeter of the base = 9 + 12 + 15 = 36 cm
The total surface area of a prism = 2 × 54 cm2 + 36 cm × 5 cm = 108 cm2 + 180 cm2 = 288 cm2
Hence, the correct answer is 288 cm2.
Frequently Asked Questions (FAQs)
No, a cone is not a prism. A prism has two parallel and congruent bases connected by rectangular faces, whereas a cone has a single circular base and a curved surface that tapers to a point (the apex).
Volume of a Prism = Area of the Base × Height
A prism has two parallel, congruent bases connected by rectangular faces, and its cross-sections parallel to the bases are the same. A pyramid has a single base and triangular faces that converge to a single apex, with cross-sections parallel to the base decreasing in size as they approach the apex.
Yes, a parallelepiped is a type of prism where the bases are parallelograms. It is a specific form of a prism where all six faces are parallelograms.
The surface area of a rectangular prism is calculated using the formula $2(bl+lh+hb)$, where $l,b,$ and $h$ are the lengths of the prism's edges.