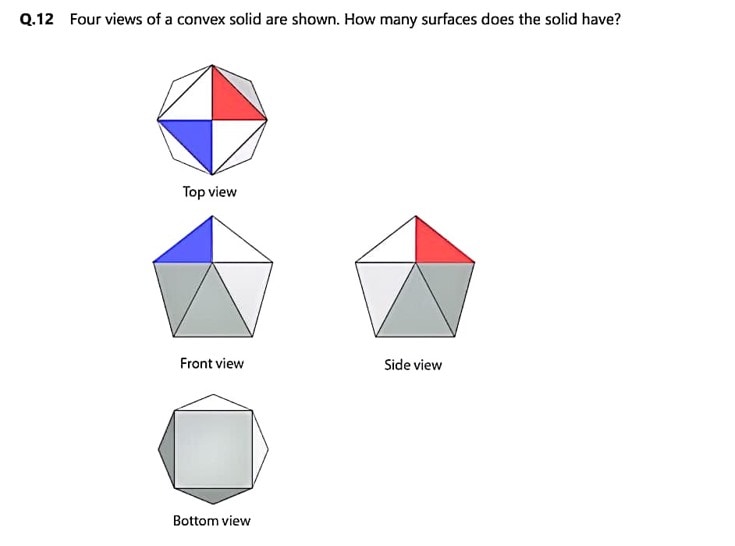
Surface Counting: Techniques and Practice for Design Entrance Exams

Surface counting is a visualization exercise that involves determining the number of distinct surfaces in a three-dimensional object. This skill is frequently tested in design entrance exams such as NID, UCEED, CEED, and NIFT, where candidates are required to analyze and interpret complex geometric structures. Mastering surface counting enhances spatial reasoning and analytical abilities, which are essential for success in these examinations.

This Story also Contains

1. INTRODUCTION TO SURFACE COUNTING
2. Understanding the Concept of Continuity and Discontinuity :
3. Practise Questions for Surface Counting:

INTRODUCTION TO SURFACE COUNTING

Surface counting involves counting the number of visible and invisible surfaces for a given three-dimensional arrangement. A three-dimensional arrangement could be a single entity or a combination of more than one three-dimensional volume with continuity or discontinuity in the surfaces, which we would go through next :

1.Discontinuous Surfaces : Cube and Cuboid

To understand the fundamentals of Surface Counting for 3D volumes, we will begin with the primary volumes like cubes and cuboids.

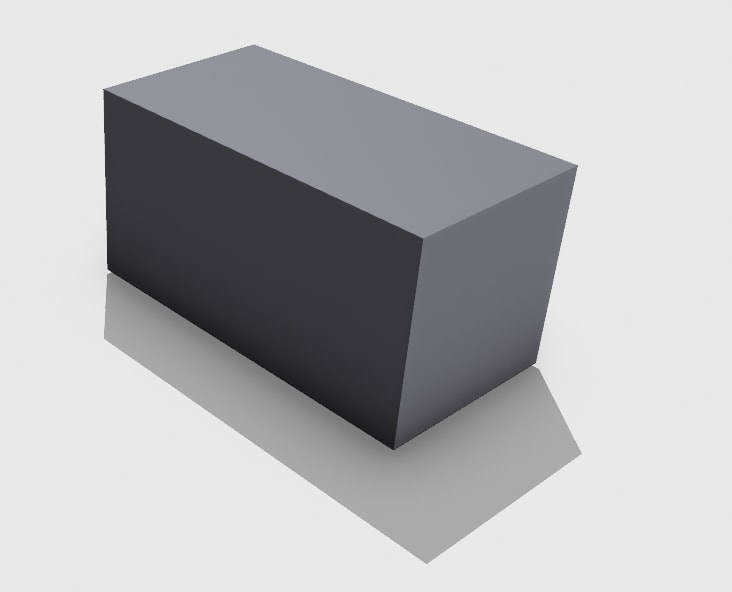
Cube (all sides are equal)

Both cube and cuboid have 06 surfaces, considering the volumes length, width and height.

Top: 01

Bottom: 01

Sides: 04

2. Continuous Surface : Sphere

A sphere, even with an equal/smaller or greater volume than a cube, has one continuous surface.

Activity : How to Count the Surfaces ?

Trick: Suppose an ant starts travelling across a surface, at point A, it meets an edge while travelling the surface. From Here(point A), the surface would be counted as the second surface.

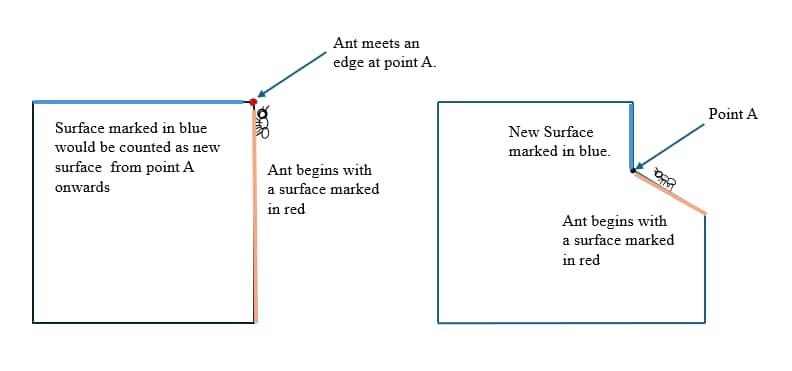
Concept 01: The Ant Rule

Imagine an ant travelling along the edges of a wall.

For the above figure, Count the Number of surfaces for the given volume, as inferred from the two given views using the “Ant Rule”.

Solution: 06 Surfaces

Method: Ant travelling across the edges

Description: As per the Ant Rule, at every encounter of an edge, the following surface count would be a new surface.

To Start counting, we need to understand the logic for solving such questions.

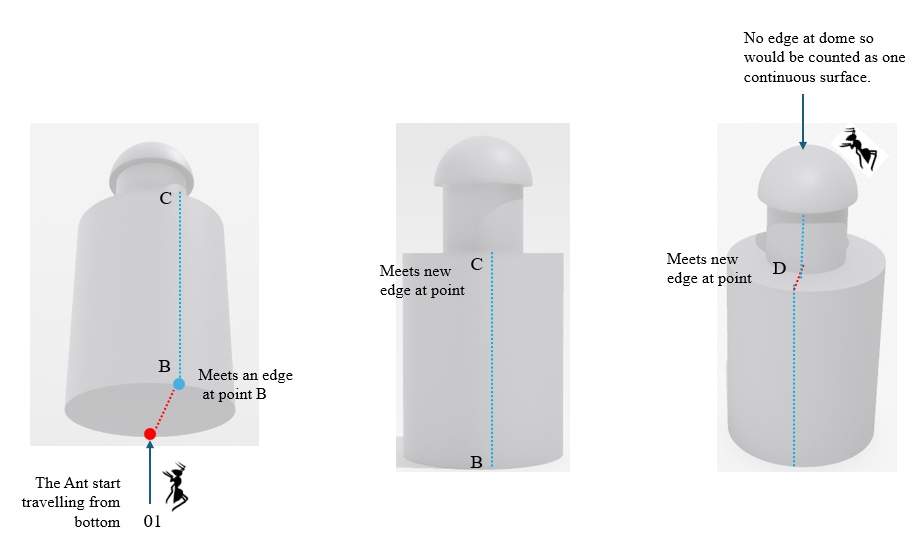
Logic 01: Continuity or discontinuity of a surface, marked by edge. In a question, the figure may contain two or more geometric volumes combined, as simple as a combination of cubes to a complex combination of multiple volumes, as shown in the above figure, which combines cylinders and hemispheres. In such cases, the edge serves as a partition between two surfaces.

Understanding the Concept of Continuity and Discontinuity :

Logic 02: Direction

It is a good practice always to count all the surfaces visible from a given direction(like the top) out of 06 possible directions.

For example, all the surfaces visible from the top should be counted at a time in the figure, i.e., T1 & T2.

How To: Select a Direction to begin counting the surfaces.

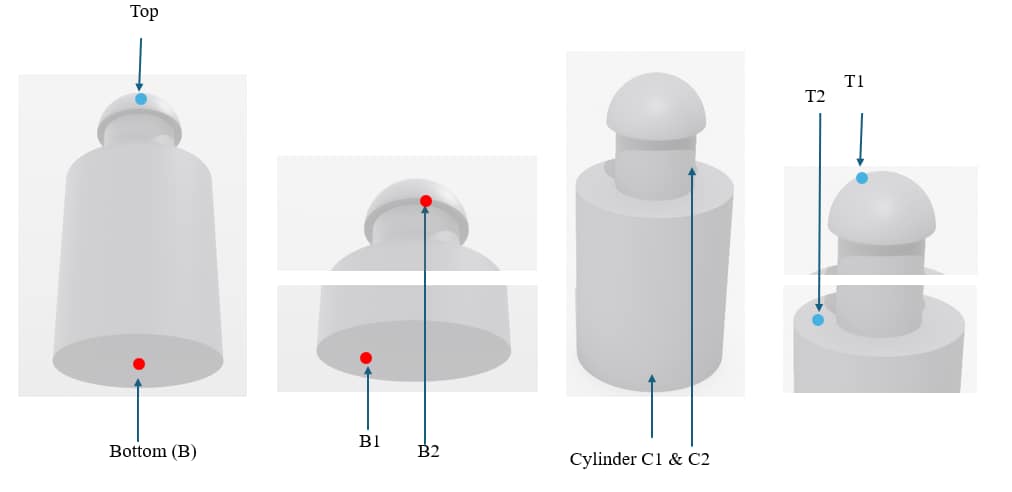
Step-by-Step Approach to Solution

• Step 01: Choose any one direction, only the Top or Only the bottom, to begin. In the given figure, we start with the bottom approach.
• Step 02: Count all the visible surfaces from the bottom, as shown in Figure 02
• Step 03: Count all the Right-side surfaces. In this case of cylindrical surfaces, only C1 & C2
• Step 04: Count all the visible surfaces from the top, in this case, T1 & T2

TOTAL SURFACES

B1+B2+C1+C2+T1+T2 = 6 surfaces

Practise Questions for Surface Counting:

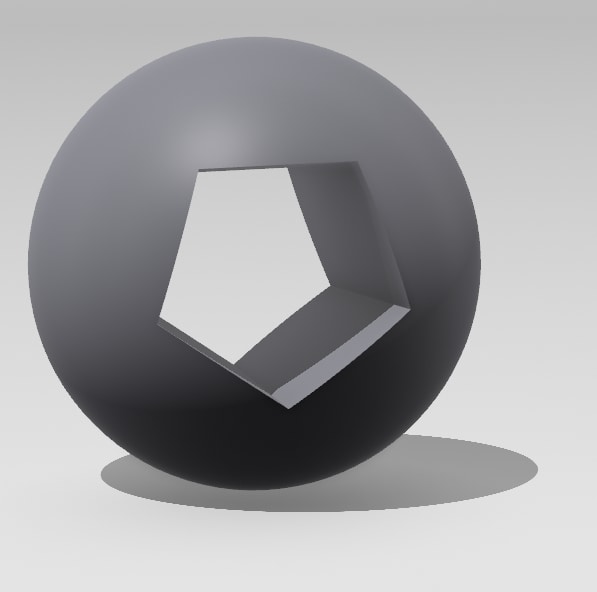
Q 2 : A pentagonal shape is cut out from the sphere for the given figure. Count the number of surfaces in the newly formed 3D volume.

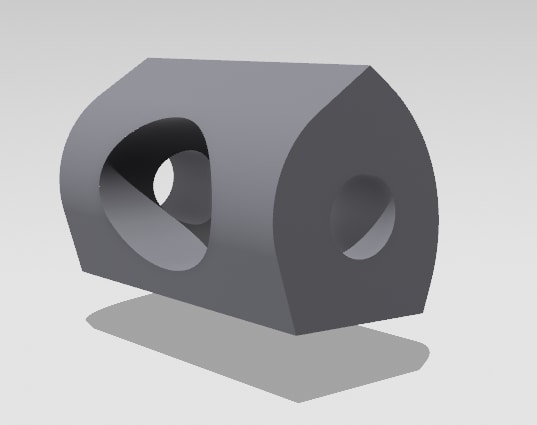
Q 3 : From the Front, Right side and Perspective views for the given volume, Count the number of faces in the figure.

Hint: Count all the faces from one side in one go, e.g. counting all the visible surfaces from the right side view.