Law of Equivalence: Definition, Formula, Questions and Examples

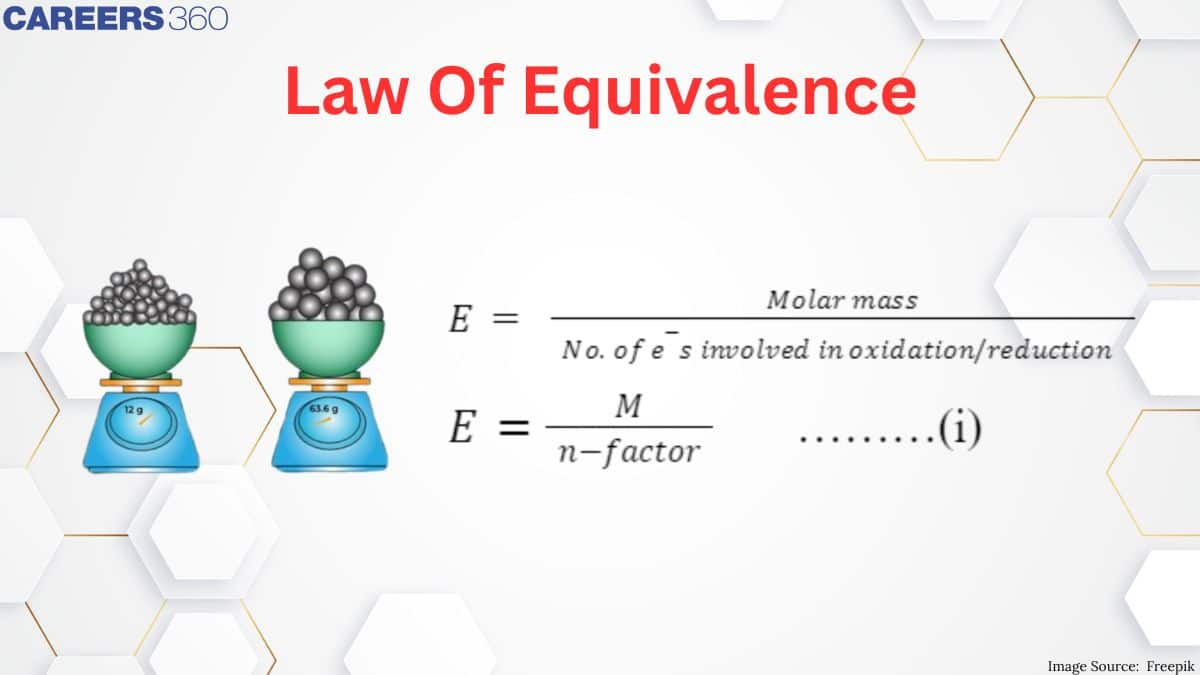
Laws of Equivalence

According to the law of equivalence, for each and every reactant and product,
Equivalents of each reactant reacted = Equivalents of each product formed.

Example,
Suppose the reaction is taking place as follows:
$$$$$\mathrm{\$P+Q\; \backslash rightarrow\; R+S\$}$$$$$

$P+Q \rightarrow R+S$

According to the law of equivalence,

Equivalents of P reacted = Equivalents of Q reacted = Equivalents of R produced = Equivalents of S produced

Equivalents of any substance = (Weight of substance (in g)) / (Equivalent weight)
= Normality (N) x Volume (V) (In liter)
Normality (N) = n-Factor x Molarity (M)

Law of Equivalence finds great importance in Acid-base Neutralisation Reactions as well as Redox Titrations.

Here we shall be mainly covering the Acid-Base neutralization reactions in detail.

Types of Equivalents

1. General Equivalent:

An equivalent is the amount of a substance that reacts with or is equivalent to one mole of another in a specific reaction—such as supplying one mole of H⁺ ions in acid-base reactions or one mole of electrons in redox reactions.

2. Acid–Base Equivalents:

• For acids an equivalent is based on how many H⁺ ions the acid can donate.

• For bases, it's the number of OH⁻ ions the base can supply.
  For example, 1 mole of H₂SO₄ can donate 2 H⁺ ions—thus it’s equivalent to 2 equivalents.

3. Redox Equivalents:

In redox reactions, equivalents refers to how many electrons are transferred. Forexample, in acidic solutions, one mole of KMnO₄ (Mn from +7 to +2) corresponds to 5 equivalents because it accepts 5 electrons.

$\mathrm{MnO}_4^{-}+5 \mathrm{Fe}^{2+}+8 \mathrm{H}^{+} \rightarrow \mathrm{Mn}^{2+}+5 \mathrm{Fe}^{3+}+4 \mathrm{H}_2 \mathrm{O}$

4. Equivalent Weight (Equivalent Mass):
This is the mass of a substance that corresponds to one equivalent.

• Calculated by dividing molecular weight by the valency factor (n-factor)

5. Electrochemical Equivalent :
Used in electrochemistry, this measures the mass of a substance deposited or liberated per unit of electricity—typically grams per coulomb. Values vary by element (e.g., copper, silver, etc.)

6. Normality (Equivalent Concentration):
Normality (N) is the number of equivalents per liter of solution.

• In acid-base chemistry, it reflects equivalents of H⁺ or OH⁻.

• In redox reactions, it corresponds to electrons transferred.

Application in Real Life and its Implication

The law of equivalence is very essential in practical chemistry. In titrations, it confirms accurate measurements by comparing reactive equivalents of acids and bases. It is also helpful in redox reactions and volumetric analysis, empowering precise calculations of reactants and products. Industries depends on it for tasks such as water treatment—monitoring chlorine or oxygen levels—and food and pharmaceutical testing, such as measuring vitamin C or iron content. The principle bridges theoretical stoichiometry with real lab and industrial practices, enhancing reliability, safety, and efficiency in chemical processes.

Also Read:

• Oleum And Its % Labelling
• Some Basic Concept In Chemistry Formula

Recommended topic video on(Law of Equivalence)

Some Solved Examples

Example 1:In this equation:

$\mathrm{}$$\mathrm{}$
$\mathrm{}{\mathrm{}}_{}$$2 \mathrm{KClO}_3 \rightarrow 2 \mathrm{KCl}+3 \mathrm{O}_2$

What will be the mass (in g) of O₂ produced from 1.23g of KClO₃?

1) 0.482

2) 0.545

3) 0.758

4) 0.345

Solution:

According to the equation,

2 moles of KClO₃ will produce 3 moles of O₂.

Thus moles of KClO₃ = 1.23 / 123= 0.01 moles

Therefore, 0.01 moles of KClO₃ will produce = (3 x 0.01) / 2= 0.015 moles

thus mass of O₂ = 0.015 x 32
= 0.48g

Hence, the answer is the option (1).

Example 2:
50 mL of 0.5 M oxalic acid is needed to neutralize 25 mL of sodium hydroxide solution. What is the mass (in g) of NaOH in 50 mL of the given sodium hydroxide solution?

1) 20

2) 4

3) 10

4) 40

Solution

$\begin{aligned} & \mathrm{H}_2 \mathrm{C}_2 \mathrm{O}_4+2 \mathrm{NaOH} \rightarrow \mathrm{Na}_2 \mathrm{C}_2 \mathrm{O}_4+2 \mathrm{H}_2 \mathrm{O} \\ & \text { meq of } \mathrm{H}_2 \mathrm{C}_2 \mathrm{O}_4=\text { meq } \mathrm{NaOH} \\ & 50 \times 0.5 \times 2=25 \mathrm{MNaOH} \times 1 \\ & 1000 \mathrm{ml} \text { solution }=2 \times 240 \text { gramNaOH } \\ & \therefore 50 \mathrm{ml} \text { sol }=4 \mathrm{gNaOH}\end{aligned}$

$\begin{array}{rl}& {\mathrm{}}_{}\end{array}$$\begin{array}{r}\end{array}$


Hence, the answer is the option (2).$\begin{array}{r}{\mathrm{}}_{}\end{array}$

Example 3:
The volume of 0.1 N dibasic acid sufficient to neutralize 1 g of a base that furnishes 0.04 mol of OH− in aqueous solution is:

1) 200mL

2) 400mL

3) 600mL

4) 800mL

Solution

Dibasic acids mean acids have two ionizable hydrogens.

gram eq. of $\mathrm{OH}^{-}=$mole $\times$n-factor $=0.04 \times 1=0.04$

The Law of equivalence we get,
milli equivalents of acid = milli equivalents of base

N₁ X V₁ (ACID) = ( meq. of base)

0.1×V = ( meq. of base)

0.1 X V = ( meq. of base) = 0.04$ \times 1000 $

V x 0.1 = 40
V = 400ml

Hence, the answer is an option (2).

Example 4:
How much NaOH is required to neutralize 1500 mL of 0.1 M HCl?

1) 40g

2) 4g

3) 6g

4) 60g

Solution

According to the law of Equivalence,

meq. of HCl = meq. of NaOH

$1500 \times 0.1=$ moles of $\mathrm{NaOH}($ as $n$-factor of $\mathrm{NaOH}=1)$
moles of $\mathrm{NaOH}=150$
Weight of $\mathrm{NaOH}=6 \mathrm{~g}$

Hence, the answer is the option (3).

Example 5:
To neutralize 20 mL of M10 sodium hydroxide, the volume of M20 hydrochloric acid required is:

1) 10ml

2)15ml

3) 20ml

4) 40ml

Solution

According to the law of equivalence,

meq. of HCl = meq. of NaOH

$\begin{aligned} & \therefore 20 \times \frac{1}{10}=\times \frac{1}{20} \\ & =40 \mathrm{ml}\end{aligned}$

$\begin{array}{r}\end{array}$$\begin{array}{r}\end{array}$
$\begin{array}{r}\\ \end{array}$
Hence, the answer is the option (4).

Practice more Questions From the Link Given Below:

Conclusion

The Law of Equivalence expresses that in any chemical reaction, the number of equivalents of reactants is always equal to the number of equivalents of products. It makes it easy to perform calculations in titrations and redox reactions, even without a balanced equations. Law of equivalence links chemistry theory to practical use, enhancing accuracy, safety, and efficiency in laboratory and industrial processes by ensuring proper reactant usage and reliable outcomes.